Lee K.K. Lectures on Dynamical Systems, Structural Stability and Their Applications
Подождите немного. Документ загружается.


(ii)
if
G·x
and
G·y
are
any
two
orbits
of
the
non-wandering
set
then
W
8
(G·
x)
,:j\
Wu
(G·
y)
•
Thus
the
non-wandering
set
of
a
2-dim.
flow
consists
of
at
most
five
types
of
orbits,
nmely,
hyperbolic
source,
sinks,
saddle
points,
and
hyperbolic
expanding
and
contracting
closed
orbits.
Peixoto
shows
that
Theorem
6.3.1
also
holds
for
vector
fields
on
compact
orientable
2-manifolds
and
for
diffeomorphisms
of
S
1
•
That
is:
Theorem
6.3.3
[Peixoto
1962]
A
vector
field
on
a
compact
orientable
2-dim.
manifold
M
(resp.
a
diffeomorphism
of
S
1
)
is
C
1
structurally
stable
iff
it
is
Morse-Smale.
Morse-Smale
systems
are
open
and
dense
in
r•(M)
(resp.
Diff•s
1
)
for
1
!>
r
!>
oo.
The
theorem
for
non-orientable
2-manifolds
is
still
an
open
question.
Although
the
results
above
failed
to
generalize
to
higher
dimensions,
it
is
fortunate
to
find
that
for
all
dimensions:
Theorem
6.3.4
A
Morse-Smale
system
on
a
compact
manifold
is
structurally
stable.
In
fact,
it
gets
even
better.
Since
every
compact
manifold
admits
a
Morse-Smale
system
[Smale
1961],
thus
every
compact
manifold
admits
a
structurally
stable
system
[Palis
and
Smale
1970].
Theorem
6.3.4
and
the
above
statement
are
corollaries
of
the
following
theorem
due
to
Palis
and
Smale
[1970]:
Theorem
6.3.5
Let
M
be
a
compact
CU
manifold
without
boundary,
and
for
r
~
1
let
Diff(M)
be
the
set
of
c•
diffeomorphisms
of
M
with
the
uniform
c•
topology.
For
f £
Diff(M)
we
denote
by
n(f)
the
set
of
nonwandering
points
of
f.
If
f £
Diff(M)
satisfies:
(a)
n(f)
is
finite,
(b)
n(f)
is
hyperbolic,
and
(c)
transversality
condition,
then
f
is
structurally
stable.
One
can
extend
the
result
to
flows
or
vector
fields.
Using
the
above
theorem
and
a
converse
known
for
some
time,
we
have:
Theorem
6.3.6
Let
f £
Diff(M),
with
n(f)
being
finite.
254

Then
f
is
structurally
stable
iff
f
satisfies
(a)-(c)
in
Theorem
6.3.5.
This
result
gives
a
good
characterization
of
structural
stability
in
the
n-finite
case
and
leads
to
the
question
of
finding
a
similar
characterization
for
the
general
case.
Palis
and
Smale
also
proposed
the
following
conjecture.
f e
Diff(M)
is
structurally
stable
iff
f
satisfies:
(a)
axiom
A,
i.e.,
n(f)
is
hyperbolic
and
the
set
of
periodic
points
off
is
dense
in
n(f);
(b)
strong
transversality
condition,
i.e.,
for
all
x,
y e
n(f),
W
5
and
Wu
intersect
transversally.
Armed
with
these
results,
Afraimovich
and
Sil'nikov
[1974]
considered
the
system:
dxjdt
=
f(x),
(6.3.1)
with
f e
c•,
r
~
1,
in
some
region
D c R".
Let
G
be
a
bounded
region
which
is
homeomorphic
to
a
ball
and
has
a
smooth
boundary.
Then
they
considered
the
system,
dxjdt
=
f(x),
d6jdt
=
1,
(6.3.2)
defined
in
G X 5
1
•
They
have
the
following
theorem.
Theorem
6.3.7
If
system
(6.3.1)
is
a
Morse-Smale
system
in
G,
then
for
sufficiently
small
6
in
c
1
,
the
a-neighborhoods
of
system
(6.3.2)
will
be
everywhere
dense
Morse-Smale
systems.
From
Palis
and
Smale
[1970]
and
Robbin
[1971]
one
obtains
the
following
useful
result.
Corollary
6.3.8
If
system
(6.3.1)
is
structurally
stable
in
G
and
does
not
have
periodic
motions,
then
system
(6.3.2)
is
also
structurally
stable.
In
the
following,
we
shall
briefly
discuss
perturbation
problems
for
the
two
dimensional
system
dxjdt
f(x).
By a
perturbation
of
the
system,
we
mean
a
system
dx/dt
=
f(x)
+
~g(x,t),
(6.3.3)
where
x =
(u,v)
e R
2
,
~
<<
1.
Equivalently,
we
have
the
suspended
system:
dxjdt
=
f(x)
+
~g(x,B),
d6jdt
=
1,
(x,6)
E R
2
X 5
1
•
(6.3.4)
Here
f(x)
is
a
Hamiltonian
vector
field
and
~g(x,t)
is
a
small
perturbation
which
need
not
even
be
Hamiltonian
255

itself.
We
want
to
discuss
the
bahavior
of
the
orbits
of
(6.3.3)
for
(x,~)
in
a
neighborhood
of
rx{O}
where
r
is
the
periodic
orbit
of
the
unperturbed
system.
The
basic
idea
is
due
to
Melnikov
[1963].
It
is
to
make
use
of
the
globally
computable
solutions
of
the
unperturbed
integrable
system
in
the
computation
of
perturbed
solutions.
In
order
to
do
this,
we
must
first
ensure
that
the
perturbation
calculations
are
uniformly
applicable
on
arbitrarily
long
time
intervals.
Let
us
make
the
assumptions
precise.
consider
the
system
(6.3.4)
which
is
sufficiently
smooth,
c•,
r
~
2,
and
bounded
on
the
bounded
region
G,
and
g
is
periodic
in
t
with
period
T,
and
f =
(f
1
(x),f
2
(x))t,
and
g =
(g
1
(x,t),g
2
(x,t))t.
For
simplicity,
we
assume
that
the
unperturbed
system
is
Hamiltonian
with
f
1
=
aHjav,
f
2
=
-aH;au.
Furthermore,
we
assume
the
unperturbed
flow
is:
(a)
For~
=
0,
the
system
(6.3.4)
possesses
a
homoclinic
orbit
q
0
(t),
to
a
hyperbolic
saddle
point
Po;
(b)
Let
ro =
{q
0
(t)
I
t € R}U{p
0
},
the
interior
of
ro
is
filled
with
a
continuous
family
of
periodic
orbits
~(t),
a €
(-1,0).
Letting
d(x,r
0
)
=
infqtrolx
-
ql
we
have
lim,....
0
supttR
d(~(t)
,r
0
)
=
0.
(c)
Let
h
..
=
H(~(t))
and
T
..
be
the
period
of
~(t).
Then
T
..
is
a
differentiable
function
of
her
and
dT
..
/dhcz > 0
inside
ro.
Before
we
state
any
results,
we
would
like
to
remark
that
many
of
them
can
be
proved
under
less
restrictive
assumptions.
Note
that
assumption
(a)
implies
that
the
unperturbed
Poincare
map P
0
has
a
hyperbolic
saddle
point
p
0
and
that
the
closed
curve
ro =
Wu(P
0
)
n W
8
(p
0
)
is
filled
with
nontransverse
homoclinic
points
for
P
0
•
Finally,
the
Melnikov
function
is
defined
as:
M(t
0
)
=
J_.,"'
f(q
0
(t
- t
0
))
A
g(q
0
(t
- t
0
)
1
t)
dt
(6.3.4)
Then
we
have
the
following
important
theorem
which
allows
us
to
test
for
the
existence
of
transverse
homoclinic
orbits
for
specific
systems:
Theorem
6.3.9
If
M(t
0
)
has
simple
zeros
and
is
independent
of
~'
then
for
sufficiently
small
~
>
O,
Wu(P~)
and
W
8
(p~)
intersect
transversely.
If
M(t
0
)
remains
away
from
zero
then
Wu
(pi') n W
8
(p~)
=
0.
256

Remarks:
(i)
M(t
0
)
is
periodic
with
period
T.
(ii)
If
the
perturbation
g
is
derived
from
a
time-dependent
Hamiltonian
function
G(u,v)
such
that
g
1
=
aG;av,
g
2
=
-aG;au;
then:
M(t
0
) =
J_.,"'
(H(q
0
(t-
t
0
)),
G(q
0
(t-
t
0
),t)}
dt,
(6.3.5)
where
(H,G}
denotes
the
Poisson
bracket
(Section
2.8).
(iii)
If
g =
g(x),
not
explicitly
time
dependent,
then
using
Green's
theorem
we
obtain:
M(t
0
)
=
Lnt
r"
trace
Dg(x)
dx.
(iv)
By
changing
variables
t
~
t + t
0
,
we
have
a
more
convenient
Melnikov
integral:
M(t
0
) =
J..,"'
f(q
0
(t))
A
g(qO(t)
,t+t
0
)dt.
Now,
let
us
return
to
the
more
general
case,
where
g =
g(x,t;8).
Theorem
6.3.10
Let
the
system
dxjdt
=
f(x)
+
~g(x,t;B),
8 E R,
satisfies
the
assumptions
(a)-(c)
before
we
defined
the
Melnikov
function.
Suppose
that
the
Melnikov
function
M(t
0
,8)
has
a
quadratic
zero
M(r,Bb) =
(aM;at
0
)
(r,8b)
= 0
but
ca·
Mjato')
(T
,Bb) + 0
and
(aM;aB)
(T
,Bb) +
0.
Then
BB
=
Bb
+
0(~)
is
a
bifurcation
value
for
which
quadratic
homoclinic
tangencies
occur
in
the
family
of
systems.
If
we
rewrite
system
(6.3.3)
as:
dxjdt
=
f(x,~),
where
x
=
(x
1
,x
2
),
and
for~=
o,
the
system
becomes
dxjdt
=
f(x,O)
has
a
periodic
orbit
r
with
period
T.
Let
us
define
a
0
=
tr[af(O,O)/ax}
+
0.
Theorem
6.3.11
The
homoclinic
orbit
r
is
asymptotically
stable
if
a
0
< 0
and
unstable
if
0
0
>
0.
There
can
be
at
most
one
periodic
orbit
bifurcating
from
r
and
it
is
asymptotically
orbitally
stable
if
a
0
< 0
and
unstable
if
a
0
>
0.
Thus,
if
a
0
=
O,
one
can
have
either
periodic
orbits
in
any
neighborhood
of
r,
or
r
can
be
asymptotically
stable
or
unstable.
To
illustrate
this,
consider:
dxjdt
=
2y,
dyjdt
12x
-3X
2
•
This
system
has
a
potential
V(x,y)
= x
3
-
6x'
+
y• ,
and
the
solution
curves
are
given
as
257

y
Clearly,
the
origin
0
is
a
hyperbolic
saddle
and
point
A =
(4,0)
is
a
center.
There
is
a
periodic
orbit
in
any
neighborhood
of
the
homoclinic
orbit
r
and
a
0
=
o.
Take
note
that
r c
((x,y):
V(x,y)
=
0}.
Consider
the
perturbed
system
to
be:
dx/dt
=
2y-
~V(x,y)
(12x-
3x
2
)
=
f
1
(x,y,~),
dyjdt
=
12x-
Jx•
+
~V(x,y)2y
=
f
2
(x,y,~).
It
is
easy
to
show
that
a
0
(~)
=
tr
a(f
1
(0,0,~),f
2
(0,0,~))/a(x,y)
= 0
for
all~·
The
perturbed
system
has
only
the
equilibrium
points
0
and
A
with
0 a
hyperbolic
saddle
and
A a
hyperbolic
focus
for
~
+
0
sufficiently
small.
A
is
stable
for
~
> 0
and
unstable
for
~
< o.
The
solution
curves
in
(x,y)-space
defined
by
V(x,y)
= 0
is
invariant
under
the
perturbation.
Thus,
r
is
again
a
homoclinic
orbit
for
the
perturbed
system
for
every
~.
Noting
that
the
perturbed
system
is
obtained
from
the
original
system
by
a
rotation
through
an
angle
arctan(~V(x,y)),
it
follows
that
no
curve
V(x,y)
=constant
inside
the
curve
r
can
be
tangent
to
the
vector
field
of
the
perturbed
system.
Thus,
there
can
be
no
periodic
orbits
of
the
perturbed
system
inside
r
for
~
f
0.
Since
~
> 0
(~
<
0)
implies
the
focus
A
is
stable
(unstable),
it
follows
that
r
is
unstable
(asymptotically
stable)
for~>
0
(~
<
0).
Chan
[1987]
considered
the
equation
d
2
X/dt
2
+
g(x)
=-
adxjdt
+
~f(t),
where
xis
real,
g
is
smooth,
f
is
periodic,
i.e.,
f(t+l)
f(t),
and
a
and~
are
small
parameters.
By
using
periodic
Melnikov
functions
and
the
method
of
Liapunov,
all
the
258

Floquet
multipliers
of
the
bifurcating
subhamonic
solutions
are
determined.
Recently,
Ling
and
Bao
[1987]
have
deviced
a
numerical
implementation
of
Melnikov•s
method.
The
procedure
is
based
on
the
convergence
of
the
integral
and
the
uniqueness
of
the
boundary
of
the
horseshoe
region
in
the
parameter
space
under
the
conditions
that
it
be
inverse
symmetric,
i.e.,
f(x)
=
-f(-x),
and
there
exists
more
than
one
homo(hetero)clinic
orbits.
Meanwhile,
Salam
[1987]
presented
explicit
calculations
which
extended
the
applicability
of
the
Melnikov's
method
to
include
a
general
class
of
highly
dissipative
systems.
The
only
required
condition
is
that
each
system
of
this
class
possesses
a
homo(hetero)clinic
orbit.
Furthermore,
it
was
shown
that
sufficiently
small
time-sinusoidal
perturbation
of
these
systems
resulted
in
transversal
intersection
of
stable
and
unstable
manifolds
for
all
but
at
most
discretely
many
frequencies.
For
further
details
for
bifurcations
of
autonomous
or
periodic
planar
equations,
and
their
applications,
see,
e.g.,
Chapter
4
of
Guckenheimer
and
Holmes
[1983]:
Chapter
9-11
of
Chow
and
Hale
[1982]:
Chapter
7
of
Lichtenberg
and
Lieberman
[1983]:
and
for
applications,
see,
e.g.,
Smeller
[1983].
Recently,
Wiggins
[1988]
has
developed
a
global
perturbation
technique
similar
to
that
of
Melnikov
[1963]
for
detecting
the
presence
of
orbits
homoclinic
to
hyperbolic
periodic
orbits
and
normally
hyperbolic
invariant
tori
in
a
class
of
ordinary
differential
equations.
This
technique
is
more
general
then
Melnikov's
because
it
applies
to
systems
undergoing
large
amplitude
excitation
at
low
frequencies
and
to
systems
undergoing
quasiperiodic
excitation.
When
the
paper
by
Palis
and
Smale
appeared,
it
was
known
that
there
were
other
structurally
stable
systems
beside
Morse-Smale
systems.
One
of
them
was
the
toral
automorphisms
(see
Section
4.1).
These
are
structurally
stable,
but
their
non-wandering
sets
are
the
whole
of
the
tori,
so
they
259

certainly
fail
condition
(i)
of
Marse-smale.
Next,
we
shall
very
briefly
discuss
the
Anosov
systems.
6.4
Anosov
systems
A
diffeomorphism
f:
M
~
M
of
a
manifold
M
is
Anosov
if
M
has
a
hyperbolic
structure
with
respect
to
f.
Recall
that
this
means
that
the
tangent
bundle
TM
splits
continuously
into
a
Tf-
invariant
direct-sum
decomposition
TM
= E
8
+
Eu
such
that
Tf
contracts
E
5
and
expands
Eu
with
respect
to
some
Riemannian
matric
on
M.
Trivially,
hyperbolic
linear
maps
f
possess
this
property,
since
one
has
the
identification
TR"
=
R"
x
R"
and
Tf(x,v)
=
(f(x),f(v)).
In
the
case
of
toral
automorphisms,
this
splitting
is
carried
over
to
the
tours
when
the
identification
was
made.
So,
toral
automorphisms
are
Anosov.
Similarly,
a
vector
field
on
M
is
Anosov
if
M
has
a
hyperbolic
structure
with
respect
to
it.
As
examples
of
such,
we
have
all
suspensions
of
Anosov
diffeomorphisms.
Theorem
6.4.1
[Anosov
1962]
Anosov
systems
on
compact
manifolds
are
c
1
structurally
stable.
There
are
some
unsolved
problems
about
Anosov
diffeomorphisms.
For
instance,
is
their
non-wandering
set
always
the
whole
manifold?
Do
they
always
have
a
fixed
point?
Since
not
all
manifolds
admit
Anosov
diffeomorphism,
do
all
n-dim.
manifolds
which
do
admit
them
have
R"
as
universal
covering
space?
6.5
Characterizing
structural
stability
Realizing
the
diagonal
differences
of
systems
such
as
Morse-Smale
and
Anosov
systems,
it
is
a
challenging
problem
to
characterize
structural
stability.
The
essential
link
comes
about
when
Smale
recognizes
the
fact
that
by
replacing
the
term
"closed
orbits"
in
Morse-Smale
definition
by
"basic
sets"
(to
be
defined
shortly),
then
Anosov
and
other
systems
are
also
encompassed.
260

A
dynamical
system
has
an
n-decomposition
if
its
non-
wandering
set
n
is
the
disjoint
union
of
closed
invariant
sets
n
1
,
n
2
,
•••
,
nk.
If
the
system
is
topologically
transitive
on
n;
(i.e.,
n;
is
the
closure
of
the
orbit
of
one
of
its
points)
for
all
i,
we
say
that
n;u
....
unk
is
a
spectral
decomposition,
and
that
the
n;
are
basic
sets.
One
can
also
define
a
basic
set
individually
by
saying
that
a
closed
invariant
set,
A c n
is
basic
if
the
system
is
topologically
transitive
on
A
but
A
does
not
meet
the
closure
of
the
orbit
of
njA.
Note
that
a
basic
set
is
indecomposable
since
it
is
not
the
disjoint
union
of
two
non-empty
closed
invariant
sets.
A
dynamical
system
satisfies
Axiom
A
if
its
non-wandering
set
(a)
has
a
hyperbolic
structure,
and
(b)
is
the
closure
of
the
set
of
closed
orbits
of
the
system.
It
was
conjectured
that
(a)
implies
(b).
Newhouse
and
Palis
[1970]
have
shown
that
it
is
true
for
diffeomorphisms
of
2-dim
manifolds,
but
false
for
higher
dimension
manifolds
[Danberer
1977].
Theorem
6.5.1
(Spectral
Decomposition
Theorem)
The
non-
wandering
set
of
an
Axiom
A
dynamical
system
on
a
compact
manifold
is
the
union
of
finitely
many
basic
sets.
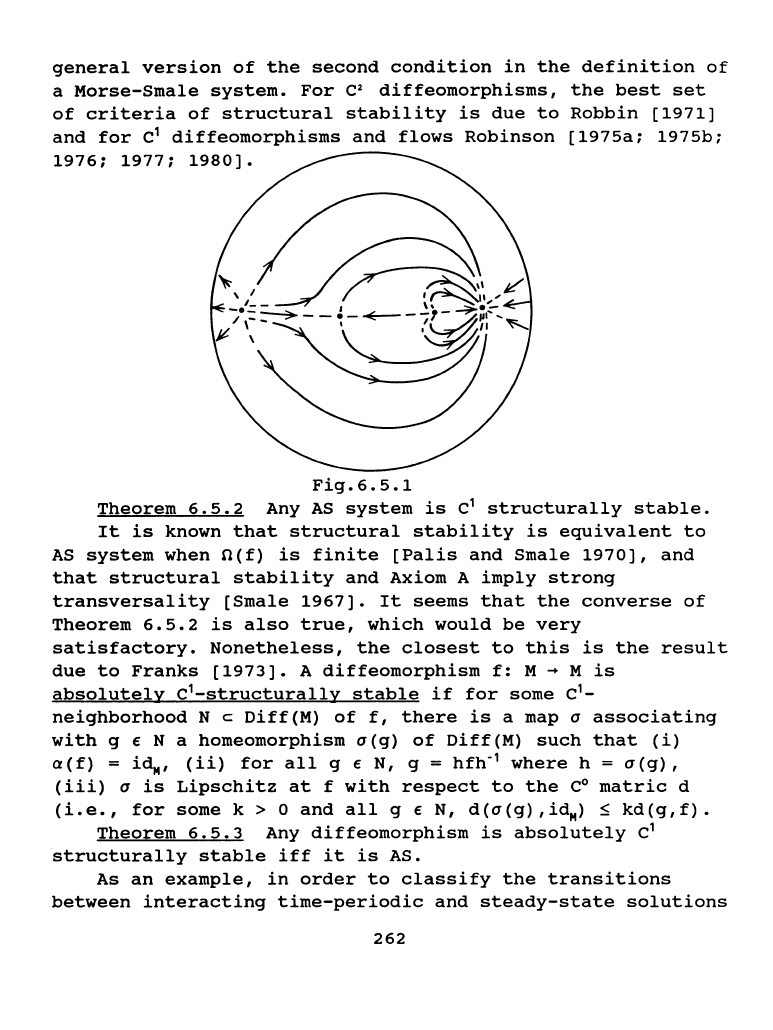
In
order
to
visualize
an
Axiom
A
system,
one
thinks
of
a
system
of
2-dim
manifold
with
finitely
many
fixed
points
and
periodic
orbits,
all
hyperbolic,
such
as
the
gradient
of
the
height
function
on
the
torus,
or
a
Morse-Smale
system
for
s•
(see
Fig.
6.5.1).
But
in
higher
dimensions,
one
replace
the
fixed
points
and
periodic
orbits
by
more
general
basic
sets.
It
should
be
pointed
out
that
there
is
no
need
for
a
basic
set
to
be
a
submanifold.
Such
basic
sets
are
termed
strange
or
exotic.
Following
Smale,
we
say
that
a
system
is
AS
if
it
satisfies
both
Axiom
A
and
the
strong
transversality
condition,
which
is,
for
all
x
and
y
in
the
non-wandering
set
of
the
system,
the
stable
manifold
of
the
orbit
of
x
and
the
stable
manifold
of
the
orbit
of
y
intersect
transversally.
The
strong
transversality
c
~ition
is
the
261
general
version
of
the
second
condition
in
the
definition
of
a
Morse-Smale
system.
For
c•
diffeomorphisms,
the
best
set
of
criteria
of
structural
stability
is
due
to
Robbin
[1971]
and
for
c
1
diffeomorphisms
and
flows
Robinson
[1975a;
1975b;
1976; 1977:
1980].
Fig.6.5.1
Theorem
6.5.2
Any
AS
system
is
c
1
structurally
stable.
It
is
known
that
structural
stability
is
equivalent
to
AS
system
when
n(f)
is
finite
[Palis
and
Smale
1970],
and
that
structural
stability
and
Axiom
A
imply
strong
transversality
[Smale
1967].
It
seems
that
the
converse
of
Theorem
6.5.2
is
also
true,
which
would
be
very
satisfactory.
Nonetheless,
the
closest
to
this
is
the
result
due
to
Franks
(1973].
A
diffeomorphism
f:
M
~
M
is
absolutely
c
1
-structurally
stable
if
for
some
c
1
-
neighborhood
N c
Diff(M)
of
f,
there
is
a
map
a
associating
with
g E N a
homeomorphism
a(g)
of
Diff(M)
such
that
(i)
a(f)
=
i~,
(ii)
for
all
g
EN,
g =
hfh"
1
where
h =
a(g),
(iii)
a
is
Lipschitz
at
f
with
respect
to
the
C
0
matric
d
(i.e.,
for
some
k > 0
and
all
g
EN,
d(a(g),idM)
S
kd(g,f).
Theorem
6.5.3
Any
diffeomorphism
is
absolutely
C
1
structurally
stable
iff
it
is
AS.
As
an
example,
in
order
to
classify
the
transitions
between
interacting
time-periodic
and
steady-state
solutions
262

of
nonlinear
evolution
equations
by
using
the
normal
forms
of
the
imperfect
bifurcation
theory
(Section
6.6),
two
types
of
bifurcation
of
solutions
of
general
evolution
equations
are
fundamental:
the
bifurcation
of
a
steady
state
with
amplitude
x
and
the
Hopf
bifurcation
of
a
time-periodic
solution
with
amplitude
y
from
a
stationary
one.
Interactions
between
them
lead
to
secondary
bifurcations
of
periodic
solutions
and
to
tertiary
bifurcations
of
double-periodic
motions
lying
on
tori
and
eventually
to
chaotic
motions.
such
interactions
occur
if
a
control
parameter
~
in
the
evolution
equation
crosses
some
critical
values.
By
Liapunov-Schmidt
reduction
we
can
show
that
x
and
y
satisfy
two
algebraic
normal
form
equations
a(x,~,y
2
)
= 0
and
yb(x,~,y
2
)
=
o.
The
solution
of
the
first
is
a
two
dimensional
multisheeted
surface
y =
~(x,~)
in
(x,y,~)-space,
and
that
of
the
second
equation
is
another
surface
y =
p(x,~).
The
lines
along
which
both
surfaces
intersect
are
the
bifurcation
diagrams
of
the
evolution
equations
from
which
the
behavior
of
the
system
can
be
inferred
as
~
varies.
The
intersection
of
the
two
surfaces
may
be
transversal
so
that
any
perturbation
of
a
and
b,
i.e.,
a
slight
deformation
or
shifting
of
the
surfaces,
causes
no
new
type
of
intersections.
In
this
case
structural
stability
of
the
bifurcation
diagram
is
ensured
at
the
outset.
Nonetheless,
if
the
two
surfaces
intersect
with
tangential
contact,
or
just
touching,
then
a
slight
deformation
or
shifting
of
them
produces
new
intersections
and
gives
rise
to
new
bifurcation
diagrams
which
then
are
stable
against
any
further
perturbations.
These
perturbations
can
be
thought
to
be
induced
by
variations
of
system-imminent
imperfection
parameters
(such
as
impurity
of
material
parameters
etc.)
in
the
original
evolution
equations.
Since
the
forms
of
the
perturbed
polynomials
a
and
b
can
be
classified
into
a
finite
set
by
imperfect
bifurcation
theory,
the
problem
of
interacting
spatial
and
temporal
patterns
has
thus
been
reduced
to
linking
together
the
possible
basic
bifurcation
diagrams.
Then
a
variety
of
263
