Labelle P. Supersymmetry DeMYSTiFied

Подождите немного. Документ загружается.

Supersymmetry Demystified

Let us write the two types of dot products explicitly in terms of the components

of the spinors. It is conventional to write the components of a left-chiral Weyl spinor

with lower indices, namely,

χ ≡





in terms of which the ﬁrst invariant of Eq. (2.50) is

χ ·χ = χ

− χ

At ﬁrst sight, this may seem to be identically zero, but recall that the χ

are

fermionic quantum ﬁelds, which anticommute. This is clear if we write the ﬁelds

in terms of creation and annihilation operators. But what if we use the path integral

approach, where quantum ﬁelds are not represented by operators? In that case, as

you surely remember, the fermionic degrees of freedom must be represented by

Grassmann variables, which also anticommute. In either case, we have

=−χ

,(χ

)

= (χ

)

= 0

Using the ﬁrst of these two relations, we get three equivalent expressions for the

dot product χ ·χ :

χ ·χ = χ

− χ

= 2 χ

=−2 χ

(2.51)

Consider now the second invariant of Eq. (2.50). It is simply given by

¯χ · ¯χ = χ

†

− χ

†

= 2 χ

†

=−2 χ

†

(2.52)

This is it. This is all we are going to introduce in terms of new notation in this

section. In the next chapter, a different way to represent these dot products will be

introduced, but for now, you should think of χ ·χ and ¯χ · ¯χ purely as shorthand

notations for the expressions appearing on the right of Eq. (2.50), nothing more.

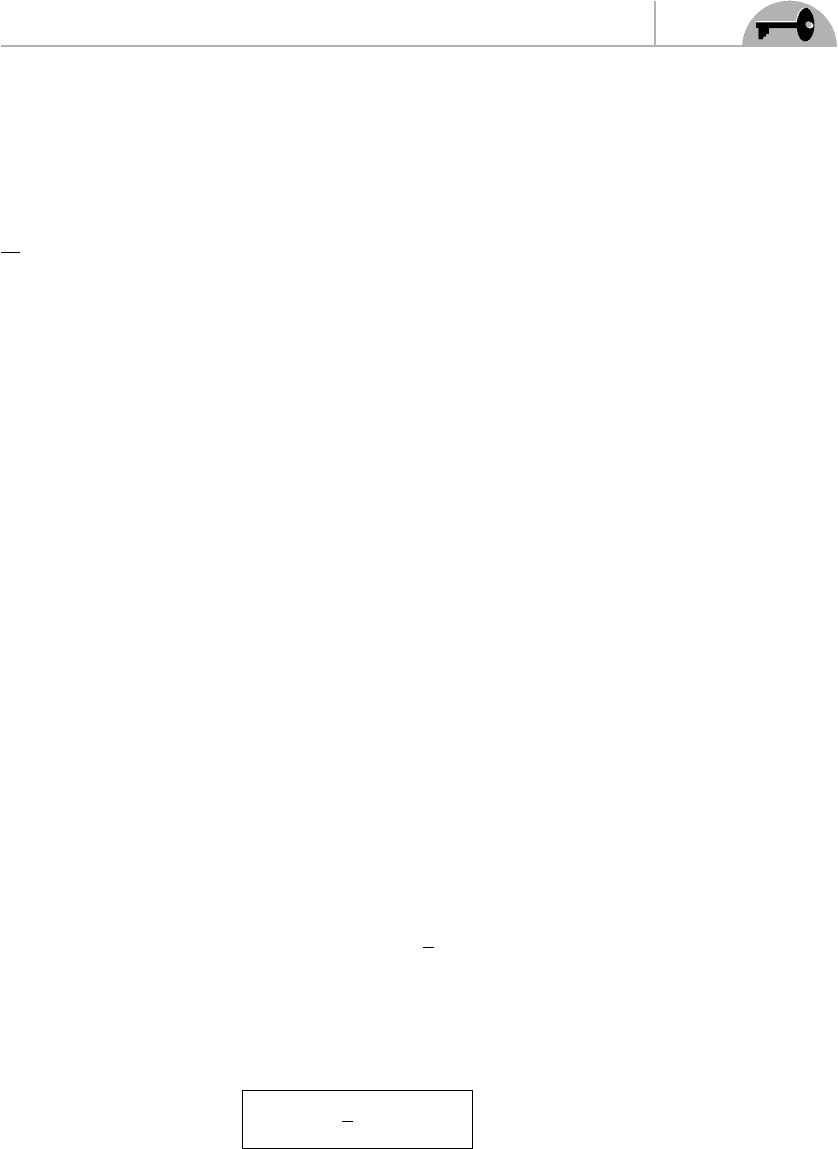
CHAPTER 2 A Crash Course on Weyl Spinors

To be honest, SUSY afﬁcionados usually don’t write any dot symbol (·) between

the spinors, but it makes expressions more clear to include it, so that’s what we will

do for the rest of this book. However, even the notation used here may cause some

confusion, so let us issue a few warnings.

First, note that the bar appearing over the Weyl spinors has nothing whatsoever

to do with the bar used to represent Dirac conjugation for a four-component spinor,

 = 

†

. We will discuss the bar notation for Weyl spinors in more depth later.

For now, consider the bars in the second line of Eq. (2.50) to be simply a notation

to distinguish the two types of dot products.

Second, those “spinor dot products” have nothing to do with the usual dot prod-

ucts between four-vectors or three-vectors familiar from introductory physics. It

may be tempting to think of χ ·χ, say, as χ

+ χ

, but this, of course, would be

incorrect (and equal to zero!). If we want to calculate these dot products explicitly

in terms of components, we need to go back to their explicit deﬁnitions given in

Eq. (2.50).

Third, it’s very important to keep in mind that the difference between the two

types of dot products is not only that one involves the hermitian conjugate of the

spinors, whereas the other does not; there is also a sign difference between the two

deﬁnitions, as is shown explicitly in Eqs. (2.51) and (2.52).

However, the two types of dot products obviously can be written in terms of one

another using

¯χ · ¯χ =−χ

†T

· χ

†T

(2.53)

The right-hand side is admittedly rather ugly, which is why the bar notation of the

left-hand side is preferred. The point of showing this relation is that the bar notation

is redundant; we could write everything in terms of unbarred spinors, at the price

of sometimes getting awkward expressions.

From Eq. (2.51), we see that the product of the two components of a spinor is

related to the dot product of that spinor with itself in a very simple way:

=−χ

=−

χ ·χ

whereas, of course, χ

= χ

= 0. These four relations can be summarized into a

very useful identity:

=−

(iσ

)

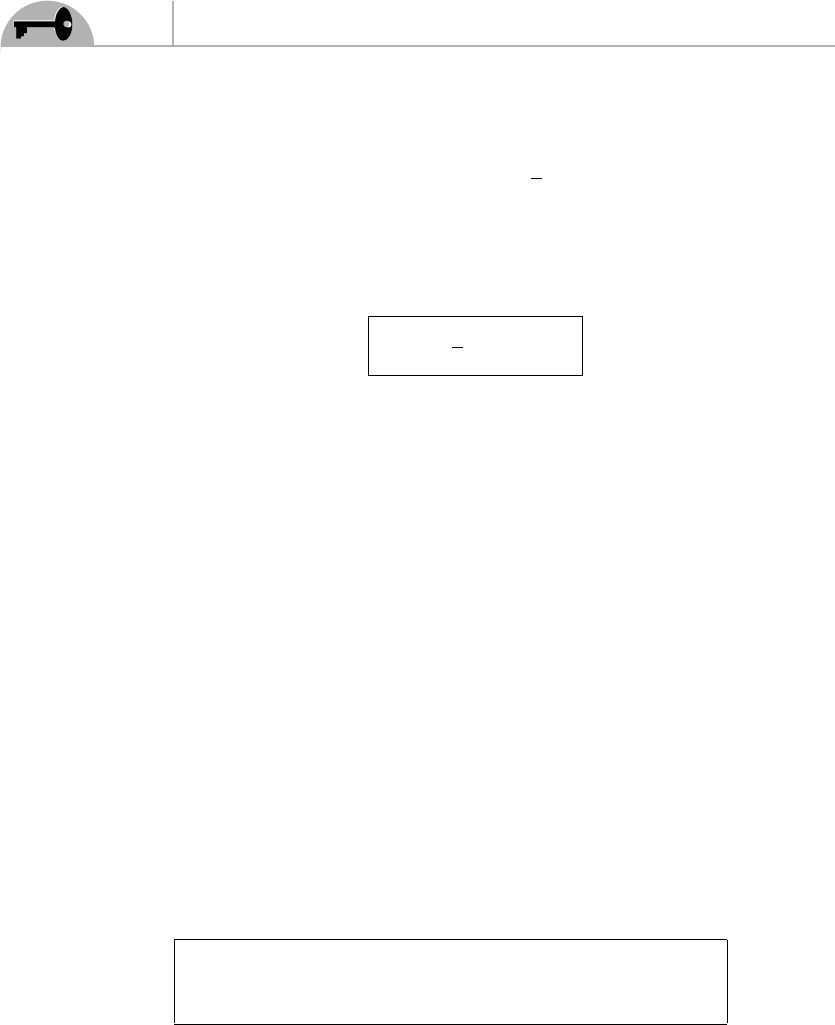
χ ·χ (2.54)

Supersymmetry Demystified

Similarly, we have

†

=−χ

†

¯χ · ¯χ

whereas (χ

†

)

= (χ

†

)

= 0, so the equivalent of Eq. (2.54) for the hermitian con-

jugated components is

†

(iσ

)

¯χ · ¯χ (2.55)

There is something important to repeat here: The hermitian conjugate in the last

two equations is applied to the components of the spinor, not to the spinor itself. In

that context, the dagger necessarily means the hermitian conjugate in the quantum

ﬁeld operator sense. If the components were simple complex numbers, it would

make no sense to write, say, χ

†

. We would simply write χ

∗

. Keep in mind that

whenever we write components of a spinor with a dagger applied, as in χ

†

, the

quantum ﬁeld operator sense is always implied.

There is another simple relation between the two types of products [much more

elegant than Eq. (2.53)], but before demonstrating it, we ﬁrst have to deal with a

tricky issue. Consider taking the hermitian conjugate of χ ·χ :

(χ ·χ)

†

= (2χ

)

†

We face a subtle problem. We know that the hermitian conjugate of the product of

two matrices exchanges their order, but what do we do when we have Grassmann

quantum ﬁelds (in other words, spinor quantum ﬁelds)? Do we include an extra

minus sign owing to the exchange? The convention is to deﬁne the hermitian con-

jugate to exchange their order without introducing a factor of minus one. This is so

important that we cannot resist the urge to put it in a box:

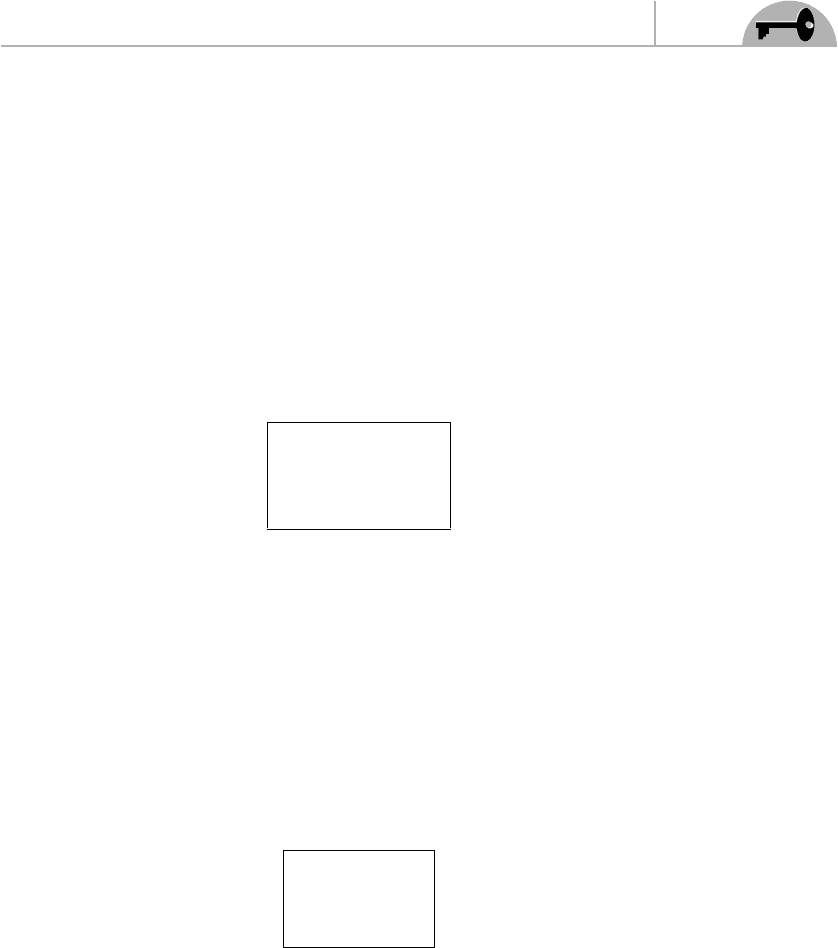
The hermitian conjugate of the product of two spinor ﬁelds

exchanges their order without introducing a minus sign

(2.56)

Again, an ambiguity arises if we use the path integral approach and write the

fermion ﬁelds as Grassmann variables. In that case, instead of the hermitian con-

jugate of a product of ﬁelds, we would simply take the complex conjugate of that

product. But, in order to be consistent with Eq. (2.56), we must deﬁne the complex

CHAPTER 2 A Crash Course on Weyl Spinors

conjugate of a product of Grassmann variables to change their order without intro-

ducing a minus sign! In other words, we deﬁne

(αβ)

∗

= β

∗

(2.57)

where α and β are some arbitrary Grassmann variables.

With this convention, we get

(χ ·χ)

†

= 2(χ

)

†

= 2χ

†

(2.58)

which is precisely ¯χ · ¯χ! So we have the following two important identities:

(χ ·χ)

†

= ¯χ · ¯χ



¯χ · ¯χ



†

= χ ·χ

(2.59)

where the second line is simply the hermitian conjugate of the ﬁrst line.

So far we have only written invariants built out of a single left-chiral spinor. Of

course, we also will need to consider invariants made of two different left-chiral

spinors, let’s say χ and λ. In this case, the two possible invariants mixing the two

spinors are obviously

χ ·λ ¯χ ·

At ﬁrst sight it seems that we also should consider λ · χ and

λ · ¯χ, but it turns

out that the order of the spinors in spinor dot products does not matter, i.e.,

λ · χ = χ · λ

λ · ¯χ = ¯χ ·

(2.60)

We have emphasized these identities by placing them in a box because they will

be used repeatedly. They probably look wrong to you because the components of

spinors anticommute, so shouldn’t we add a minus sign when we switch their order?

The answer is no because the dot products used here involve the matrix σ

, which

is antisymmetric. This introduces an extra minus sign that cancels the minus sign

arising from the exchange of the spinor components. The following exercise will

make this more explicit.

Supersymmetry Demystified

EXERCISE 2.6

Prove Eq. (2.60). Hint: Use the deﬁnitions (2.50) and the identity (2.48).

The generalization of Eq. (2.59) to the products between two different spinors

is easily found to be

(λ · χ)

†

λ · ¯χ

(

λ · ¯χ)

†

= λ · χ

(2.61)

Consider now three left-chiral spinors χ, α, and β. The following identities are

easy to prove:

χ ·αχ · β =−

χ ·χα· β

¯χ · ¯α ¯χ ·

β =−

¯χ · ¯χ ¯α ·

(2.62)

We see that the net effect of these identities is to swap the spinors appearing

in the dot products. Such identities are generically referred to as Fierz identities.

These particular examples of Fierz identities will prove to be very useful later in

this book.

EXERCISE 2.7

Prove Eq. (2.62). Hint: You may simply expand out completely both sides in terms

of the components of the spinors and then move some of them around, or you may

use Eqs. (2.54) and (2.55).

In the next chapter we will introduce the shorthand notation of dotted and undot-

ted indices mentioned in the Introduction, but as also mentioned there, we will not

make use of it until Chapter 11. So feel free to go directly to Chapter 4 and come

back to Chapter 3 later.

2.10 Quiz

1. Write ¯χ ·

λ explicitly in terms of the components of the spinors.

2. Under what condition does a Weyl spinor have a deﬁnite helicity? Under what

condition does it have a deﬁnite chirality?

CHAPTER 2 A Crash Course on Weyl Spinors

3. Do left- and right-chiral spinors transform the same way or differently under

rotations? What about their transformations under boosts?

4. Write down the expression for a Dirac mass in terms of Weyl spinors.

5. Consider the two components χ

and χ

of a Weyl spinor. What is (χ

)

†

equal to ?

This page intentionally left blank

CHAPTER 3

New Notation for

the Components

of Weyl Spinors

As mentioned at the end of Chapter 2, you may safely skip this chapter on a ﬁrst

reading and come back to it before you tackle Chapter 11.

What we would like to do now is to introduce a notation for the components

of the spinors that will make it obvious how to construct Lorentz invariants. Our

notation will be inspired from the summation convention of relativity, where upper

and lower indices can be contracted to form invariant quantities such as A

(or,

more generally, to form quantities that have deﬁnite transformation properties, such

as tensors). We want our notation to be such that we simply contract the indices

of the spinors to form invariants, without having to take hermitian conjugates or to

insert iσ

matrices. This will force us to introduce more than one type of index, as

we will soon ﬁnd out.

Supersymmetry Demystified

Since in this section we will be showing the indices of the spinors explicitly in

most equations, it would be very awkward to include the L or R labels as well. We

will therefore drop these indices with the understanding that χ will always represent

a left-chiral spinor, whereas η will consistently be used to represent a right-chiral

spinor. When other spinors will be needed, their chirality will be deﬁned when they

are introduced.

As we have seen previously, we assign lower indices to the components of left-

chiral spinors. There is no particular reason for assigning a lower index instead of

an upper index and no particular reason to pick left-chiral spinors, but hey, we have

to start somewhere! We will use indices taken from the ﬁrst few letters of the Latin

alphabet for the spinor components. So let us make the deﬁnition

Components of χ ≡ χ

What we mean by this is that in all the expressions we have written so far, the χ

have to be considered as having lower indices, as is shown explicitly in Eqs. (2.51)

and (2.52).

Next, we would like to introduce a notation for the components of right-chiral

spinors. This is simple if we recall that a Lorentz invariant containing χ and the

right-chiral ﬁeld is η

†

χ. In order to follow the usual convention of Lorentz invari-

ants being built out of matched upper and lower indices, we deﬁne this invariant

to be

†

χ ≡ η

(3.1)

You are probably wondering where the hermitian conjugate went! The point is that

we choose the deﬁnition (3.1) to make the result as similar as possible to what

we encounter in relativity, but the price to pay is that the quantities η

are not the

components of the spinor η themselves but their hermitian conjugate. It’s important

to keep this in mind, so let’s emphasize it:

≡ hermitian conjugate of the components of η (3.2)

The obvious question is then: What notation should we use to represent the com-

ponents of the spinor η themselves? Well, we would like to keep an upper index,

but it has to be different from a Latin letter, and we surely don’t want to introduce a

Greek letter, which could cause confusion with four-vector and tensor indices. The

solution that has been chosen is to use an upper dotted Latin letter to represent the

CHAPTER 3 New Notation for Weyl Spinors

components of a right-chiral spinor, e.g., η

˙a

. This notation in terms of dotted and

undotted indices is often referred to as the van der Waerden notation.

Actually, this is not the end of the story. The notation η

˙a

is ﬁne as it is, as long

as the indices are shown explicitly. But let’s say that we want to write expressions

without including explicit indices. It would be very confusing if, after dropping the

indices, we were to write both η

and η

˙a

as η, so we introduce another layer of

notation: We will include a bar symbol over spinors with dotted indices. Our ﬁnal

notation for the components of a right-chiral spinor is then

Components of η ≡ ¯η

˙a

(3.3)

When the indices are shown, the bar notation is redundant, but it is conventional

to include the bar whether or not the indices are explicitly written.

Again, what we mean by Eq. (3.3) is that in all the equations we wrote before

this section, the η has to be thought of as having upper dotted indices. For example,

Eq. (3.2) may be written as

≡ (¯η

˙a

)

†

(3.4)

Of course, this also implies

¯η

˙a

= (η

)

†

(3.5)

or, to be more explicit,

¯η

= (η

)

†

¯η

= (η

)

†

We see that the effect of taking the hermitian conjugate is to change a dotted index

into an undotted index (and to add or remove a bar) without changing the position

of the index. Actually, we have only shown this for upper indices on right-chiral

spinors, but as we will soon see, it will work for lower indices and for components

of left-chiral spinors as well.

In case you are wondering, the bar in Eq. (3.3) is indeed the same bar that appears

in the spinor dot product in Eq. (2.50), and again, this bar has nothing to do with

Dirac conjugation. You may not like this notation at ﬁrst (I sure didn’t) because

it may seem to create a potential source of confusion with Dirac conjugation, but

Bartel Leendert van der Waerden was a Dutch mathematician who made important contributions to several ﬁelds

of mathematics, most notably to group theory.