Labelle P. Supersymmetry DeMYSTiFied

Подождите немного. Документ загружается.

Supersymmetry Demystified

where δ

is the Kronecker delta, and 

ijk

is the totally antisymmetric Levi-Civita

symbol. To be explicit,



123

= 

231

= 

312

= 1 and 

321

= 

213

= 

132

=−1

with all the other components being zero. The Levi-Civita symbol makes it possible

to write the components of a cross-product between vectors in a compact manner.

For example, the kth component of the vector



A ×



B may be written in either of

the following two forms:

(



A ×



= 

ijk

= 

kij

(2.7)

Note that we are using Einstein’s summation convention throughout this entire

book: Any repeated index is implicitly summed over. However, we will require

only Lorentz indices to satisfy the rule that they must appear in the upper and lower

positions to be contracted.

From Eq. (2.6) one can calculate the commutator of two Pauli matrices to be

[σ

,σ

] ≡ σ

− σ

= 2i

ijk

(2.8)

The anticommutator is also easily computed:

{σ

,σ

}≡σ

+ σ

= 2δ

1 (2.9)

From this anticommutator, we directly obtain

=−σ

for i = j (2.10)

(σ

)

= 1

We noted earlier that σ

stands apart from the other two matrices when we

consider complex conjugation or transposition. Let us focus on σ

again, for which

we have

=−σ

= σ

CHAPTER 2 A Crash Course on Weyl Spinors

the last one being, of course, trivial. What is interesting is that we can combine

these results with Eq. (2.4) to get

σ

=−σσ

(2.11)

σ

∗

=−σσ

(2.12)

Using the fact that (σ

)

= 1, we get for free

σσ

=−σ

∗

σ

= σ

σ

∗

=−σ (2.13)

where the second line is trivially obtained from the ﬁrst line by sandwiching the

latter between two σ

matrices. These identities will come in handy later on.

Consider now a vector



A whose components are numbers, not matrices. We will

soon encounter an expression of the form



A ·σσ

. It will prove useful to rewrite

this as



A ·σσ

= A

+ A

[σ

,σ

]

= A

+ 2i

ijk

= A

− 2i

jik

= σ



A ·σ − 2i(



A ×σ)

(2.14)

2.2 Weyl versus Dirac Spinors

Let us now write the four-component Dirac spinor in terms of two-component

spinors:

 =





(2.15)

In this book we will use capital Greek letters for four-component spinors and

lowercase Greek letters for two-component spinors. There is no standard notation

for the symbols used for the upper and lower two-component spinors; some authors

switch the η and the χ or use different Greek letters altogether.

Supersymmetry Demystified

The two-component spinors appearing in Eq. (2.15) are called Weyl spinors. The

reason it makes sense to decompose a Dirac spinor this way is that, as we will

see in more detail in Section 2.4, η and χ transform independently under Lorentz

transformations; i.e., they do not mix. The technical way to express this is to say

that a Dirac spinor forms a reducible representation of the Lorentz group, whereas

Weyl spinors form irreducible representations. This implies that from a purely

mathematical point of view, Weyl fermions may be considered more “fundamental”

than Dirac spinors. The reason why one has to put together two Weyl spinors to

form a Dirac spinor will be discussed in Chapter 4.

Note that setting either η or χ equal to zero in a Dirac spinor yields eigenstates

of γ













=−





The eigenvalue of γ

is called the chirality of the spinor. By a slight abuse of

language, we will say that the upper two-component spinor η has a chirality of +1,

whereas χ has a chirality of −1 (this is an abuse of language because it is actually

the four-component spinor with two components set to zero that has a deﬁnite

chirality, not the two-component spinors themselves).

A Weyl spinor with positive chirality is sometimes referred to as a right-chiral

spinor, and a Weyl spinor with negative chirality is referred to as a left-chiral

spinor. However, some references replace the adjectives right-chiral and left-chiral

by right-handed and left-handed, which is quite unfortunate because, in general,

knowing the chirality does not tell us the handedness of a particle! We will soon

discuss how the handedness or, to be more technical, the helicity of a spinor is

deﬁned, but the key point to make here is that the notions of chirality and helicity

of a spinor coincide only when a particle is massless. For a massive particle, both

the left-chiral and the right-chiral states are, in general, linear combinations of

left-handed and right-handed states. We will be careful about consistently using

left-chiral and right-chiral to describe the eigenstates of the chirality operator. As

we just mentioned, these two terms are synonyms of left-handed and right-handed

only in the massless case.

Why has it become common to use left-handed and right-handed to describe

the states of deﬁnite chirality, even for massive particles? We don’t know for sure,

but if we had to bet, our guess would be that people got so used to working with

massless neutrinos in the standard model and talking about left-handed neutrinos

and right-handed antineutrinos that they kept using this nomenclature even when

CHAPTER 2 A Crash Course on Weyl Spinors

they started considering massive neutrinos, and this habit has made its way into

SUSY, where Weyl spinors are ubiquitous. However, we would not bet a lot of

money.

But why not forget about chirality and just work with helicity? The answer is

that, as already mentioned, it is the chirality that tells us how a spinor behaves under

Lorentz transformations! When a spinor is massive, it is its chirality that speciﬁes

what representation of the Lorentz group it belongs to, not its helicity. Obviously,

it is the behavior under Lorentz transformations that matters when constructing

theories, so we will care much more about chirality than about helicity. Indeed, we

will only discuss helicity in a few paragraphs in the entire book, whereas chirality

will be omnipresent from the beginning to the end. We will discuss in detail the

Lorentz transformations of the chiral states in Section 2.4.

We will therefore show explicit subscripts R and L on the spinors η and χ for

the remainder of the chapter to indicate their chirality. Of course, if the spinors

are massless, the labels also tell us the helicity. When we start constructing super-

symmetric theories, we will be working exclusively with left-chiral spinors, so

subscripts won’t be necessary.

Be warned that when it comes to the different types of spinors, there is no

standard notation. Most books or papers do not show explicitly the indices L

and R, and there is no general consensus on what Greek letters to use for left-

and right-chiral spinors. Some switch the χ and η symbols for left- and right-

chiral spinors, whereas others use different Greek letters altogether. In addition,

some equations and expressions will be different if other representations of the

gamma matrices are used. On top of that, some authors place the right-chiral

spinor in the lower half of the Dirac spinor rather than at the top. Always check

the notation and representation used when consulting a new reference. Caveat

emptor!

It is convenient to introduce the operators P

and P

, which, when applied to a

full Dirac spinor, project out the right-chiral and left-chiral Weyl spinors. In other

words,

 = P









 = P









(2.16)

which imply







= P

γ







=−P

 (2.17)

Supersymmetry Demystified

The explicit representations of these operators are obviously



1 0





0 1



It is easy to verify that with the representation of γ

given in Eq. (2.3), we may

write these projection operators as

1 + γ

1 − γ

(2.18)

where now the 1 obviously represents the 4 × 4 identity matrix as opposed to the

2 × 2 identity matrix that it has represented so far. Most references are even less

discerning than we are here and simply use the numeral 1 to represent the identity

matrix in any dimension.

It is also easy to check that these two operators satisfy the following properties:

)

= P

)

= P

= 0

= P

=−P

The ﬁrst three identities are, of course, typical for projection operators.

If we substitute Eq. (2.15) into the Dirac equation, we obtain two coupled equa-

tions for the Weyl spinors:

(E 1 −σ ·p)η

= m χ

(E 1 +σ ·p)χ

= m η

(2.19)

Let us pause to introduce some new notation that will prove to be very useful

later on. Let us deﬁne σ

to be the following set of four matrices:

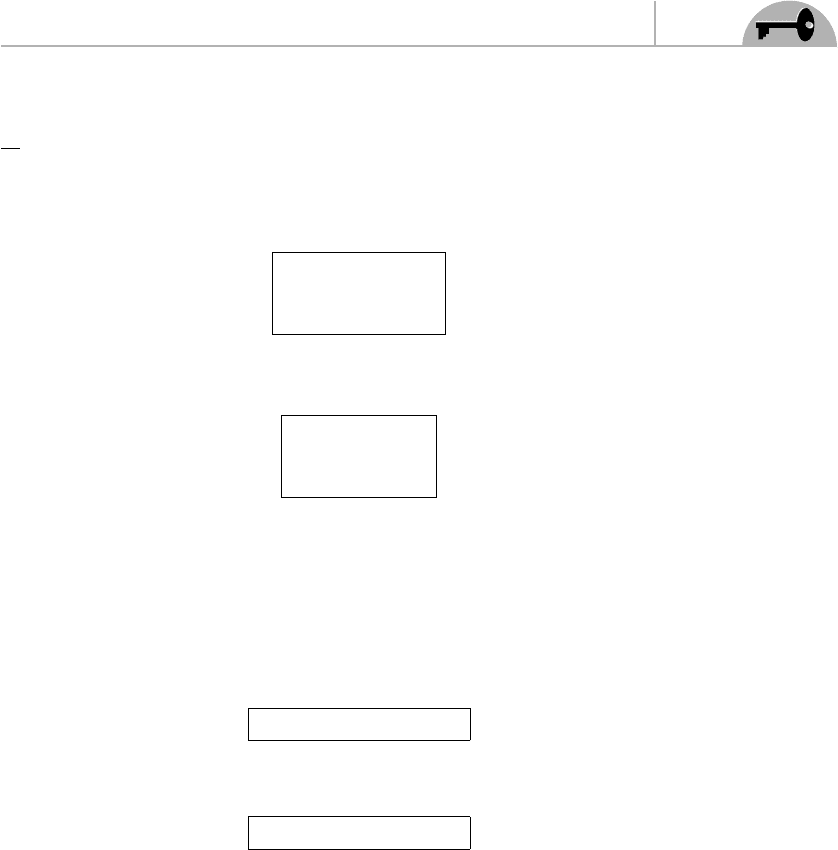
≡ (1, σ) (2.20)

We also will need to introduce

¯σ

≡ (1, −σ) (2.21)

CHAPTER 2 A Crash Course on Weyl Spinors

It’s important to emphasize that the bar used here has nothing whatsoever to do

with the one used to denote Dirac conjugation of a four-component spinor ﬁeld,

 = 

†

. A different symbol over σ

—maybe a tilde—would have been more

appropriate, but this is standard notation, and we are stuck with it.

Equation (2.13) imply the following identities:

(σ

)

= ¯σ

( ¯σ

)

= σ

(2.22)

If we take the transpose of both equations (and use (σ

)

=−σ

), we also get

= ¯σ

μT

¯σ

= σ

μT

(2.23)

Since (σ

)

∗

= (σ

)

, these equations are still valid with the transpose replaced by

a complex conjugation.

The four identities in Eqs. (2.22) and (2.23) will be so useful to us that they

have been included in Appendix A, which lists the most important identities used

throughout this book.

An identity that is easy to prove (see Exercise 2.1) is

¯σ

+ σ

¯σ

= 2η

μν

1 (2.24)

Actually, doing the proof makes it obvious that the following also holds true:

¯σ

+ ¯σ

= 2η

μν

1 (2.25)

EXERCISE 2.1

Prove Eq. (2.24). Hint: Consider separately the cases μ = ν = 0, μ = 0 and ν = i,

ν = 0 and μ = i and ﬁnally μ = i and ν = j (where it is understood that a Latin

index may take a value of 1, 2, or 3 only).

Using the deﬁnitions (2.20) and (2.21), the two coupled equations (2.19) may

be written as

= m χ

¯σ

= m η

(2.26)

Supersymmetry Demystified

We also could have obtained Eq. (2.26) directly from the Dirac equation by

noting that γ

may be written as



0¯σ



(2.27)

We also can write the Dirac lagrangian in terms of the Weyl spinors by substi-

tuting Eqs. (2.15) and (2.27) in (2.2):

Dirac

= η

†

i∂

+ χ

†

¯σ

i∂

− m η

†

− m χ

†

(2.28)

Note one important point: Despite the fact that σ

and ¯σ

carry a Lorentz index,

they are, of course, not themselves four-vectors (for the same reason that γ

not a four-vector). However, we can form quantities with well-deﬁned Lorentz

properties by sandwiching them between Weyl spinors, as we will discuss in more

detail below.

2.3 Helicity

Equation (2.26) shows clearly that the Dirac equation mixes η

and χ

. We cannot

have one without the other. For example, if we set η

= 0, the ﬁrst equation yields

0 = mχ

, which implies that χ

must be zero as well, unless the mass is zero.If

the mass is zero, the two equations for η

and χ

decouple to give

σ ·p

|p|

= η

σ ·p

|p|

=−χ

(2.29)

where we have set E =|p|, which is valid for a massless particle (recall that in

natural units, c = 1).

So, for a massless particle, the Dirac equation actually breaks up into two in-

dependent equations acting on two different two-component spinors. These two

equations are known as the Weyl equations.

As we will discuss more later, this implies that when the mass is zero, we should

think of η

and χ

as ﬁelds describing two different particles instead of two states

of a single particle. In fact, one can even build theories in which only one of the

CHAPTER 2 A Crash Course on Weyl Spinors

two is present, whereas the second one is absent altogether (this is of course the

case in the standard model which contains only left-handed massless neutrinos).

If we multiply those equations by

/2 (showing explicitly  for the moment)

and use the fact that

 σ/2 is the spin operator



S,weget



S ·

pη





S ·

pχ

=−



The operator



S ·

p is called the helicity operator, and the corresponding eigen-

value is the helicity of the state. This eigenvalue corresponds to the component of

the spin along the direction of the motion of the particle. We see that in the massless

limit, the Weyl spinors are eigenstates of the helicity operator.

The fact that η

has an helicity of /2 when m = 0 tells us that its spin is aligned

with its direction of motion. If spin were a classical angular momentum, this would

correspond to a particle following the path of a right-handed helix, which is why

we refer to the two upper components of a massless Dirac spinor as a right-handed

Weyl spinor. Obviously, χ

corresponds to a left-handed Weyl spinor.

There is a simple physical argument to explain why the two helicity states do

not decouple for a massive state. To visualize, consider a particle with a classical

angular momentum vector. Let’s say that the particle moves to the right while

tracing a right-handed helix around the positive x axis. If we boost ourselves (the

observers) to a frame that is moving faster than the particle, we will now observe it

moving to our left while tracing a left-handed helix. Therefore, boosting to a frame

moving faster than the particle changes its helicity state from right-handed to left-

handed, and vice versa. This has the implication that both states must be included to

describe a massive spinor. The argument clearly fails when the particle is massless

because it is impossible to boost to a frame moving faster than the particle. In that

case, the two helicity states can never get mixed under Lorentz transformations,

and they can be thought of as different particles altogether (as opposed to two states

of a single particle in the massive case).

It’s important to keep in mind that the chiral spinors χ

and η

only have well-

deﬁned helicities in the massless limit. Let’s call ψ

and ψ

−

the states of positive and

negative helicities (i.e., the right-handed and left-handed states). Then, in general,

we have

= C

−

= C

∗

−C

∗

−

(2.30)

Supersymmetry Demystified

which can, of course, be inverted to give

∗

+|C

−

∗

−C

+|C

(2.31)

In other words, chirality and helicity offer two different bases on which the spinors

may be expanded. The massless limit corresponds to C

being identically 0 and

= 1.

Helicity, the projection of the spin on the direction of motion of the particle, is

easier to picture physically than the abstract concept of chirality. But we care more

about the chiral eigenstates χ

and η

because, as mentioned earlier, these are the

states that have well-deﬁned Lorentz transformation properties, as we will discuss

next.

2.4 Lorentz Transformations and Invariants

Our next step is to look at the Lorentz transformations of spinors. Knowing how

quantities transform under rotation and boosts is obviously crucial to building

invariant lagrangians. The inﬁnitesimal transformations of the right-chiral and left-

chiral Weyl spinors are

→ η





1 +

 ·σ −



β ·σ



→ χ





1 +

 ·σ +



β ·σ



(2.32)

where  is the inﬁnitesimal rotation vector (the direction of  gives the axis of

rotation, and its magnitude gives the amount of rotation), and β is the inﬁnitesimal

boost parameter.

The transformations [2.32] show explicitly that, as we mentioned earlier, the

left-chiral and right-chiral spinors transform independently under Lorentz transfor-

mations. They are therefore inequivalent irreducible representations of the Lorentz

group and are the fundamental “building blocks” from which any other spinor

representation can be constructed.

Because the Lorentz algebra is equivalent to the algebra su(2) × su(2), all rep-

resentations can be labeled by two numbers that are multiples of one-half. The two

CHAPTER 2 A Crash Course on Weyl Spinors

fundamental representations then are described by the quantum numbers (

, 0)

and (0,

), which describe, respectively, the left-chiral and right-chiral spinors. We

won’t use this language in this book, but it is important to be aware of this notation

because many SUSY references use it. By the way, even though the algebra acting

on the Weyl spinors is su(2) × su(2), the group under which they transform is not

SU(2) × SU(2) but instead SL(2, C), the universal covering group of the Lorentz

group SO(3, 1). This might be useful to know if you want to impress your friends.

For the sake of comparison, consider the corresponding transformations for a

four-vector, e.g., the four-momentum P

. The zeroth component P

= E and the

vector components p transform under rotations and under boosts in the following

way:

E → E



= E −



β ·p

p →p



=p − ×p −



β E

(2.33)

Now, let us ask: What Lorentz-invariant quantity can we build out of spinors?

We can use the Lorentz transformations (2.32) to ﬁgure this out or simply use the

Dirac lagrangian Eq. (2.2) as a guide. Let us ﬁrst focus on the mass term. The Dirac

lagrangian tells us that combination 

†

 is invariant. Writing this in terms of

Weyl spinors, we ﬁnd that

 = 

†

 = η

†

+ χ

†

is invariant under Lorentz transformations. Actually, it turns out that each term on

the right-hand side is separately invariant.

EXERCISE 2.2

Show that η

†

is invariant under Lorentz transformations. Note that the Lorentz

transformations of the spinors are given to ﬁrst order in  and β so that all terms of

higher order may be dropped.

Now consider the kinetic energy term of the Dirac lagrangian

γ

. Since

this is invariant and P

is a four-vector, the quantity γ

 must transform as a four-

vector (as a contravariant four-vector, to be precise). If we rewrite this expression

in terms of Weyl spinors and use Eq. (2.27), we ﬁnd that

γ

 = η

†

+ χ

†

¯σ

transforms as a four-vector under Lorentz transformations. Actually, it turns out

that each term on the right separately transforms as a four-vector. As noted earlier,

although σ

and ¯σ

carry a Lorentz index, they are not themselves four-vectors.