Labelle P. Supersymmetry DeMYSTiFied

Подождите немного. Документ загружается.

Supersymmetry Demystified

1.3 Effective Field Theories, Naturalness,

and the Higgs Mass

Let’s get back to the issue of the naturalness problem brieﬂy mentioned in

Section 1.1. The fact that the problem disappears completely in a supersymmetric

theory is one of the most appealing aspect of supersymmetry (SUSY), so it deserves

further elaboration.

In the last 30 years, our understanding of renormalization in the context of

particle physics has undergone a radical shift (or a paradigm shift to use the oft-

abused expression). To explain the difference between the “old” school of thought

and the “modern” point of view, it’s convenient to consider for regulator a “hard”

cutoff  on the integration of momenta,



∞

dk →





dk (1.1)

Of course, such a regulator breaks almost all the symmetries usually encountered

in particle physics and therefore is not practical, but for the purpose of building phys-

ical intuition about renormalization, nothing beats a good old momentum cutoff.

The old view of the cutoff was that it had no physical meaning, that it was

a purely mathematical sleight of hand introduced to make the intermediate steps

of the calculations mathematically well-deﬁned and that, by consequence, it had

to be taken to inﬁnity at the end of the calculation. A renormalizable theory is,

by deﬁnition, a theory in which any result for physical processes can be made

cutoff-independent in the limit  →∞by redeﬁning, i.e., renormalizing, the ﬁnite

number of parameters appearing in the lagrangian (the so-called bare parameters).

By contrast, the modern point of view is that all the quantum ﬁeld theories

we know (possibly with the exception of M theory) are to be treated as effective

ﬁeld theories, i.e., theories that are approximations of more fundamental quantum

theories, and in that context, the cutoff must be viewed as a physically meaningful

parameter whose value should not be taken to inﬁnity. Instead, the ﬁnite value of

the cutoff corresponds to the energy scale at which the effects of the “new physics”

beyond the effective ﬁeld theory become important. From that point of view, there

is nothing wrong with nonrenormalizable theories. As long as the processes that are

computed involve particles with a typical external (nonloop) momentum p much

smaller than the scale of the new physics 

, the nonrenormalizable contributions

appear in the form of an expansion of the form







CHAPTER 1 Introduction

where the coefﬁcients c

are of order one. As long as the momentum p is much

smaller than the scale of new physics, one can neglect all but the ﬁrst few terms to

a given precision. Of course, the desired accuracy also dictates the number of loops

used in the calculation, so there are two expansions involved in any calculation.

Obviously, as p approaches 

, the whole effective ﬁeld theory approach breaks

down, and one needs to uncover the more fundamental theory (which might itself

be an effective theory valid only up to some higher scale of new physics). In some

sense, effective ﬁeld theories are the “quantum equivalents” of Taylor expansions

in calculus. The expression

1 − x + x

/2 − x

is a good approximation to exp(−x) as long as x (the analogue of p/

) is much

smaller than 1. If we use x = 2, we are outside of the radius of convergence of the

expansion, and we must replace the “effective” description 1 − x + x

/2 ... by

the more fundamental expression exp(−x).

However, things are not as obvious in the context of a quantum theory because

even if the external momenta are small relative to the scale of new physics, the loop

momenta have to be integrated all the way to that scale. So it might seem at ﬁrst sight

that no matter how small the external momenta are, since the loops will be sensitive

to the new physics, the very concept of an approximate theory does not make sense

in the context of quantum physics. However, the diagrams with external momenta

much smaller than 

and internal momenta on the order of the scale of new

physics are highly off-shell and, because of the Heisenberg uncertainty principle,

are “seen” by the external particles as local interactions with coefﬁcients suppressed

by powers of the scale of new physics. A detailed and very pedagogical discussion

of this point is presented in the paper by Peter Lepage,

who has pioneered the use

of effective ﬁeld theories in bound states and in lattice quantum chromodynamics

(QCD). An excellent introduction to effective ﬁeld theories is the paper by Cliff

Burgess.

Let us get back to the problem of naturalness. The key point to retain from the

preceding discussion is that we must think of the cutoff as representing the scale

of new physics, not as a purely mathematical artifact that must be sent to inﬁnity

at the end of the calculation.

Consider, then, an effective ﬁeld theory that is to be regarded as an approximation

of a more general theory, for energies much smaller than the scale of new physics.

The question is whether it is natural to have dimensionful parameters in the effective

theory, masses in particular, that are much smaller than 

. Of course, at tree

level, there is never any naturalness problem; we may set the parameters of the

effective theory to any value we wish. It’s the loops that may cause some trouble

(as always!). There is a naturalness problem if the dimensionful parameters of the

effective ﬁeld theory are driven by the loops up to the scale of new physics. In that

Supersymmetry Demystified

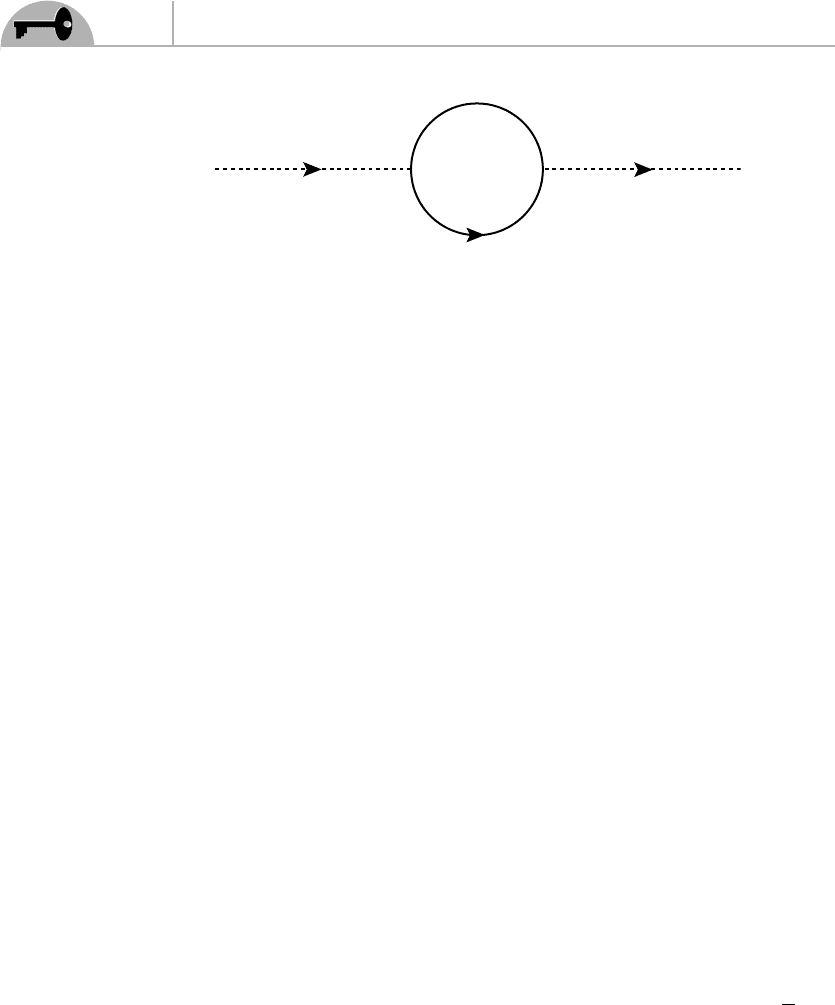
Figure 1.1 One-loop correction to a fermion propagator.

case, the only way to keep these parameters at their small values is to impose an

extreme ﬁne-tuning of the bare parameters to cancel the loop contributions.

To be more speciﬁc, consider the electron mass in quantum electrodynamics

(QED), viewed as effective ﬁeld theory of the electroweak model. The scale of

new physics is therefore of order 

≈ 100 GeV, which is much larger than the

electron mass, m

≈ 0.511 GeV. The question is whether, if we introduce loop

corrections, it is natural for the renormalized electron mass to remain so small. The

one-loop correction to the electron mass, depicted in Figure 1.1, is roughly

δm

 α



/k + m

− m



αm

4π





(1.2)

Naive power counting would lead us to expect a linear divergence, δm

 α

but the linear term in k in the numerator actually vanishes on integration, leaving

us with a much milder logarithmic divergence. Even if we set 

 100 GeV,

we ﬁnd that the correction to the bare mass is of order δm

≈ 19% relative to

its tree-level value. This is a small correction, and therefore, a small electron mass

is natural in the context of QED as an effective ﬁeld theory.

Let’s look at the masses of gauge bosons. This time, the two one-loop diagrams

of Figure 1.2 contribute if the gauge group is nonabelian, whereas only the ﬁrst

diagram exists if the gauge group is abelian. Schematically, the two diagrams give

a correction to the gauge boson mass of the form

δm

= α



+ m

− m

)

(1.3)

Figure 1.2 One-loop corrections to a nonabelian gauge ﬁeld propagator.

CHAPTER 1 Introduction

where m is the mass of whatever is circulating in the loop, and α

is the usual

/4π, where e

is the gauge coupling constant. Simple power counting predicts

this time a quadratically divergent result, but again, this contribution actually van-

ishes on integration. If the gauge symmetry is unbroken, the correction to the gauge

boson mass actually vanishes identically, δm

= 0. If the gauge symmetry is spon-

taneously broken, the correction is not zero, but the quadratic divergence is still

absent, and the leading correction is only logarithmically divergent,

δm

 α





(1.4)

Once more, the correction is small even if the scale of new physics is much larger

than the physical mass of the gauge boson.

It is not fortuitous if the leading divergences in both our examples cancel out,

leaving a milder logarithmic divergence. In both cases, a massless theory has more

symmetry than the massive theory. The theory of a massless fermion has a chiral

symmetry that ensures that the fermion will remain massless to all orders of per-

turbation theory. If we start with a massive fermion, the loop corrections no longer

vanish, but they must go to zero in the massless limit. This is why the one-loop

correction to the fermion mass could not be of the form δm

 

because this

would not vanish as the limit m

goes to zero. It is therefore chiral symmetry that

“protected” us from the linear divergence.

In the gauge boson case, it is obviously the gauge symmetry that is restored when

the gauge boson mass goes to zero. Therefore, all corrections to the gauge boson

mass must vanish as the limit m

goes to zero. This is why we did not generate a

quadratic divergence, which would not have satisﬁed this criterion.

This deﬁnition of naturalness has been formalized by ’t Hooft

A theory is natural if, for all its parameters p which are small with respect to the fundamental

scale , the limit p → 0 corresponds to an enhancement of the symmetry of the system.

Obviously, his  corresponds to our 

Let us now turn our attention to the mass of a scalar particle, e.g., the Higgs

boson in the standard model. One one-loop correction arises from the fermion loop

shown in Figure 1.3, and it corresponds to the integral

δm

 α



+ m

− m

)

≈ α

(1.5)

This time, taking the mass of the scalar to zero does not lead to any enhancement

of symmetry, and we obtain a quadratically divergent correction. If we take the

mass of the Higgs to be of order 10

GeV and the scale of new physics to be of

Supersymmetry Demystified

Figure 1.3 One-loop correction to the propagator of a scalar. The dotted lines represent

the scalar particle propagator, and the loop is a fermion loop.

order the usual grand uniﬁcation scale of about 10

GeV, we see that the one-loop

correction is roughly 24 orders of magnitude larger than the tree-level value of m

(taking α ≈ 10

−2

). This means that the bare squared mass of the Higgs would have

to be ﬁne-tuned to 24 digits to keep the renormalized mass equal to its tree-level

value! In other words, the mass of the Higgs is given by m

= C

−C

, where

both C

and C

are of order 10

GeV

and have the same ﬁrst 24 digits!

This is a bit as if you were to throw a needle up in the air and watch it fall on

a marble ﬂoor exactly on its tip and stay upright in that position! You certainly

would be ﬂabbergasted! It would not be impossible that it did land in exactly

the right way to have it be in equilibrium and then be undisturbed by any air

current after it landed, but it would seem so unlikely as to be preposterous. You

certainly would look for some explanation for why this happened. Maybe this part

of the ﬂoor is not marble, and the needle penetrated the surface? Maybe there is a

magnet under the surface, and the needle is magnetized in such a way as to stay

upright?

We are in a similar situation when pondering the mass of the Higgs in the standard

model. There has to be some way to obtain a reasonable mass of the Higgs without

invoking a mind-boggling accidental cancellation between two huge numbers!

So why not simply say that the mass of the Higgs could be huge, of the order of

GeV? Because there are other constraints that this time put an upper bound

on the Higgs mass. For example, unitarity of the S-matrix leads to the constraint

< 780 GeV (see Ref. 8 for more details).

This leaves us with only one possible way out: The scale of new physics must be

much smaller than 10

GeV. As a criterion for absence of ﬁne-tuning, it is natural

to demand that a one-loop correction must be smaller than the tree-level value.

Applying this to the Higgs mass in the standard model leads to 

≤ m

√

α ≈ 1

TeV. This makes the scale of new physics very low and accessible experimentally.

What could happen at this new scale? It could be revealed that the Higgs boson

is not an elementary particle but instead a bound state, as in technicolor theories.

Or maybe there are extra dimensions that can affect physics at the TeV scale, or

again, maybe particles are not pointlike but rather are excitations of strings.

CHAPTER 1 Introduction

But let us assume that the Higgs still would be present as an elementary point

particle in some new, more fundamental, four-dimensional theory superseding the

standard model in the TeV region. In that case, it would seem that the naturalness

problem still would be present. The scalar masses now would be driven to the scale

of new physics beyond this new theory, and we would be back to our starting point.

Unless this new theory would possess some symmetry that would protect even

scalar masses from large corrections. And this is where supersymmetry makes it tri-

umphant entrance! In theories with unbroken supersymmetry, quadratic divergences

are altogether absent, so there is no naturalness problem! This makes supersymme-

try a very attractive candidate for the new physics lurking around 1 TeV.

1.4 Further Reading

After you have ﬁnished this book, you will have the necessary background (and

hopefully, the hunger) to consult more advanced references. Here are some sugges-

tions.

We should ﬁrst mention the ground-breaking papers that gave birth to super-

symmetry. Supersymmetry was independently discovered in the west

16,33,39

and in

the former Soviet Union.

17,46

This was followed by the seminal work of Wess and

Zumino, who constructed explicit four-dimensional supersymmetric quantum ﬁeld

theories.

For more details, the ﬁrst chapter of Ref. 47 is highly recommended.

If you are interested in the phenomenology of the MSSM, the best book to read

after the present volume is the one by Aitchison.

It is presented at the same level

as the present volume and complements it nicely.

The following books are slightly more advanced but should offer no difﬁculty

after you have worked your way through Supersymmetry Demystiﬁed. Baer and

Tata

offer a large number of cross sections and decay-rate calculations. The book

by Bin´etruy

is quite exhaustive (with almost 400 references!) and discusses cos-

mologic implications as well as the MSSM in great depth. Weinberg’s book

supersymmetry is, as is all his work, a model of clarity and a great source of insights.

It covers not only the MSSM but also supergravity, Seiberg-Witten, supergraphs,

and several other topics.

Bailin and Love

focus on supersymmetry in the context of superstring theory.

A book by Dine

also covers supersymmetry and its applications to superstring

theory, but is more recent and has a wider scope (it covers Seiberg-Witten, cosmo-

logy, supergravity, and many other topics).

Although old and, unfortunately, hard to ﬁnd, the book by M¨uller-Kirsten and

Wiedemann

is a real gem because of the large number of derivations presented in

great detail. A classic is the book by Wess and Bagger,

which is a good resource

Supersymmetry Demystified

for learning about supergraphs and supergravity but is more advanced than the

previous references.

In a different category, Ref. 15 contains a large number of the most important

early research papers on supersymmetry and is an invaluable resource. In the same

vein, Ref. 25 contains all the earlier Physics Reports on supersymmetry and remains

a very useful reference.

In addition to books, a large number of excellent review papers or lecture notes on

supersymmetry are available. For more details concerning the phenomenological

aspects of the MSSM, some excellent resources are Refs. 10, 29, 32, 36, and

38. If you are interested in the Seiberg-Witten theory, Refs. 47 and 3 are good

places to start. A couple of more formal reviews are Refs. 27 and 51. Reference

43 focuses on supersymmetry breaking. Reference 13 explains in great detail how

to do calculations with Weyl spinors and provides a very large number of detailed

calculations relevant to the MSSM.

This is far from being an exhaustive list (Ref. 8 alone contains almost 400

references!). In particular, very few of the original research papers are cited in the

References, and for that we apologize to the large number of researchers who have

discovered and reﬁned supersymmetry over the years. You are invited to consult the

bibliographies of the books and review papers mentioned here for a more complete

list of references.

CHAPTER 2

A Crash Course on

Weyl Spinors

As mentioned in the Introduction, supersymmetric theories deal with Weyl or

Majorana spinors, not the more familiar Dirac spinors. The ﬁrst step to under-

stand supersymmetry (SUSY) is therefore to become at ease with those two types

of spinors. This chapter will focus on Weyl spinors and their connection with Dirac

spinors. Along the way, we will work out many identities that will be used repeat-

edly throughout this book and will deﬁne some notation that will prove very handy

in the building of supersymmetric theories.

This won’t be glamorous stuff—only in Chapter 5 will we construct our ﬁrst

supersymmetric theory—but it is crucial to become proﬁcient with the notation

established in the ﬁrst few chapters before tackling SUSY. Indeed, most physicists

working in SUSY (whom we like to refer to as “superphysicists”) will tell you

that the brunt of the difﬁculty in learning SUSY is not understanding the theory

itself but rather becoming at ease with Weyl and Majorana spinors and the daunting

notation used to describe them. It is therefore imperative to ﬁrst spend some time

Supersymmetry Demystified

familiarizing ourselves with this notation; once this is achieved, calculations in

SUSY will be a breeze (well, almost).

2.1 Brief Review of the Dirac Equation and of

Some Matrix Properties

Let’s start by reviewing a few basic relations and deﬁning our convention for the

Dirac matrices. The Dirac equation is

 = m (2.1)

or, using Feynman’s “slash” notation,

/P = m

Here, P

represents the differential operator i∂

. Throughout this book we use the

so-called natural units, in which c =

 = 1, unless stated otherwise.

It is also useful to recall the Dirac lagrangian:

Dirac

= (γ

− m) (2.2)

where the bar over the spinor denotes the Dirac adjoint, deﬁned as

 ≡ 

†

Equation (2.2) is actually not a lagrangian but a lagrangian density (because it must

be integrated over spacetime to yield the action), but we will follow the incorrect

but widespread convention of dropping the adjective density most of the time.

There are several different representations for the Dirac matrices γ

and γ used

in the literature. We choose the representation



0 1

1 0



γ =



0 −σ

σ 0



where, of course, 1 stands for the 2 ×2 identity matrix. These can be used to deﬁne

= (γ

, γ)

The corresponding quantity with a covariant index is deﬁned as

= η

μν

= (γ

, −γ)

CHAPTER 2 A Crash Course on Weyl Spinors

where η

μν

is the ﬂat spacetime metric for which we use the form

μν

= diag(1, −1, −1, −1)

This is sometimes referred to as the mostly minus convention as opposed to the

mostly plus convention (−1, 1, 1, 1). Usually, particle physicists adopt the mostly

minus convention, whereas the mostly plus metric is preferred by general relativists.

It is always important to check the convention used when consulting a new reference.

For the sake of completeness, let us also give the matrix γ

, which will be needed

shortly. We choose the following representation:



1 0

0 −1



(2.3)

This is often called the chiral or Weyl representation.

Recall that the Pauli matrices are given by







0 −i

i 0





0 −1



We will make extensive use of the Pauli matrices, so it would be useful to recall

some of their properties (which all can be checked using the explicit matrix repre-

sentations). First, they are hermitian, i.e., (σ

)

†

= σ

. However, they do not behave

so nicely under the separate operations of complex conjugation and transposition:

(σ

)

∗

= σ

(σ

)

∗

=−σ

(σ

)

∗

= σ

(σ

)

= σ

(σ

)

=−σ

(σ

)

= σ

(2.4)

Obviously,

σ

∗

=σ

(2.5)

The fact that σ

is singled out will play a key role later on.

The product of any two Pauli matrices is given by

= 1δ

+i 

ijk

(2.6)