Labelle P. Supersymmetry DeMYSTiFied

Подождите немного. Документ загружается.

Supersymmetry Demystified

Using dot products, the six Lorentz invariants built out of a left-chiral and a

right-chiral spinor of Eq. (3.22) therefore are written as

= η · χ

¯χ

˙a

¯η

˙a

= ¯χ · ¯η

= χ ·χ

¯χ

˙a

¯χ

˙a

= ¯χ · ¯χ

= η · η

¯η

˙a

¯η

˙a

= ¯η · ¯η

We now understand the choice of the order of indices in Eq. (3.22). They all have

been written with undotted indices going diagonally downward and dotted indices

going diagonally upward so that when the time would come to write them as dot

products, we would not have to switch the order of anything and introduce minus

signs.

It’s important to repeat that we could write absolutely everything in this entire

book in terms of the components with lower undotted indices and their hermitian

conjugates only. Most equations would be more cumbersome because there usually

would be a bunch of σ

matrices around and hermitian conjugate symbols all over

the place, but this is not the main motivation for the introduction of the notation.

The key point in using the van der Waerden notation is that it makes it easy to

see how expressions transform under Lorentz transformations, which is of major

importance in building lagrangians.

EXERCISE 3.4

Write down χ ·χχ · η in terms of components with lower undotted indices.

3.3 Invariants Built Out of

Two Left-Chiral Spinors

In SUSY, it is conventional to work exclusively in terms of left-chiral spinors, so

let’s restrict our discussion to this type of spinor from now on. If we have only one

such spinor, say, χ, we may write only two invariants: χ ·χ and ¯χ · ¯χ.

CHAPTER 3 New Notation for Weyl Spinors

If we add a second left-chiral spinor, let’s call it λ, then we now can write six

Lorentz invariants:

= λ · χ

˙a

¯χ

˙a

λ · ¯χ

= χ ·χ

¯χ

˙a

¯χ

˙a

= ¯χ · ¯χ

= λ · λ

˙a

λ ·

λ (3.27)

Again, the identities (3.26) apply:

λ · χ = χ · λ

λ · ¯χ = ¯χ ·

3.4 The  Notation for ±iσ

Writing the matrices ±iσ

all the time makes the calculations very awkward, espe-

cially when indices are explicitly shown. To avoid this, we will therefore introduce

one last layer of notation that is fairly common in SUSY and that consists of using

the symbol  to denote the matrices ±i σ

, following

(iσ

)

≡ 

(iσ

)

˙a

≡ 

˙a

(−iσ

)

≡ 

(−iσ

)

˙a

≡ 

˙a

We see that 

= 

˙a

and 

= 

˙a

. It greatly simpliﬁes the notation to use these

deﬁnitions, but one must be careful about issues of signs. To be explicit, the notation

implies



= 

= 1



= 

=−1

Supersymmetry Demystified

whereas the expressions with lower indices have the opposite signs:



= 

=−1



= 

= 1

Although the  with upper indices can be seen as two-dimensional versions of

the familiar Levi-Civita symbol 

ijk

, it is important to keep in mind that  with

lower indices has the opposite sign.

Now it is a simple matter to raise or lower indices on spinors using this new

notation. We have, for example,

= 

¯χ

˙a

= 

˙a

¯χ

(3.28)

and, of course, similar expressions for the right-chiral spinor.

We see that the  matrices act as a metric on the spinor indices. However, it is

important to keep in mind that in contrast with the metrics of special or general

relativity, the  are antisymmetric under the exchange of their indices:



=−



˙a

=−

b ˙a



=−



˙a

=−

b ˙a

In particular, note that the order of the indices in Eq. (3.28) must be respected: The

second index of the  must match the index of the spinor with which it is contracted;

otherwise, a minus sign must be present. For example,



=−

=−ψ

CHAPTER 3 New Notation for Weyl Spinors

In our new notation, Eqs. (2.54), (2.55), and (3.25) take the simpler form



χ ·χ

¯χ

˙a

¯χ

=−



˙a

¯χ · ¯χ

=−



χ ·χ

¯χ

˙a

¯χ



˙a

¯χ · ¯χ

(3.29)

Obviously, the order in the indices of  in Eq. (3.29) also must be respected.

Because of this need to pay very close attention to the order of the indices, the

use of the  is quite a bit more tricky than the use of the metric tensor g

μν

general relativity. Still, it is worthwhile to use the  notation for the computational

simplicity it offers.

Since −iσ

is the inverse of iσ

, we also have that



= 



= δ



˙a



b˙c

= 

˙c



b ˙a

= δ

˙a

˙c

(3.30)

where the two δ are the usual Kronecker deltas. We may use the antisymmetry of

 to generate several new identities, e.g.,



=−



=−



= 



= δ

Note again that the order of the indices is crucial: We get a positive Kronecker

delta when the index that is common to the two symbols appears in different posi-

tions in the two  (ﬁrst and second or second and ﬁrst positions).

Supersymmetry Demystified

Let us repeat the proof that η · χ = χ ·η [see Eq. (3.19)] to illustrate the power

of our new notation:

η · χ = η

= 

=−

= 

= χ

= χ ·η

where the ﬁrst minus sign came from the exchange of the two spinor components,

and the second minus sign arose from the exchange of the  indices.

3.5 Notation for the Indices of σ

and

Now let’s ﬁnd out what kind of indices we should assign to those two matrices.

Recall the results in Eqs. (2.43) and (2.44), which we repeat here for convenience:

i∂

η transforms like a left-chiral spinor (3.31)

whereas

¯σ

i∂

χ transforms like a right-chiral spinor (3.32)

Let’s start with Eq. (3.32). Since we assigned a lower undotted index to χ and we

assigned an upper dotted index to quantities transforming like a right-chiral spinor,

we see that the matrix ¯σ

converts a lower undotted index into an upper dotted

index, so it must carry two mixed upper indices as follows:

Indices of ¯σ

= ( ¯σ

)

˙ab

(3.33)

Note that the order is important; the dotted index comes ﬁrst. As for σ

, Eq. (3.31)

tells us that it converts an upper dotted index into a lower undotted index, so

Indices of σ

= (σ

)

(3.34)

CHAPTER 3 New Notation for Weyl Spinors

We have seen that the matrices  may be used to raise or lower indices on the

spinors. It is natural to wonder how they may be used to move the indices of σ

and ¯σ

. The key relations are provided in Eq. (2.23). Consider the ﬁrst of these

identities, which we’ll repeat here:

= ¯σ

μT

(3.35)

According to Eqs. (3.33) and (3.34), σ

has two lower indices, whereas ¯σ

has two

upper indices. We therefore need matrices to raise the two indices of σ

; i.e., we

need two matrices iσ

. We simply multiply both sides of Eq. (3.35) by i

to get

iσ

=−¯σ

μT

(3.36)

Let’s take advantage of the  notation. Assigning lower indices a

b to σ

,asin

Eq. (3.34), we must write the left-hand side of Eq. (3.36) as

iσ

= 

(σ

)



(3.37)

Note that we do not have any choice on the positions of the indices; since Eq. (3.36)

involves the product of three matrices, we must have the second index of the ﬁrst 

be the same as the ﬁrst index of the matrix σ

and the second index of the σ

equal to the ﬁrst index of the second . And since Eq. (3.37) carries upper indices c

(in that order), we must assign the same indices to the right-hand side of Eq. (3.36).

We then obtain that, with all indices explicitly shown, Eq. (3.36) corresponds to



(σ

)



=−( ¯σ

μT

)

Since a transpose switches the order of indices, we ﬁnally get



(σ

)



=−( ¯σ

)

Pay close attention to the order of the indices in this expression. This can be written

in a more elegant form by using 

=−

to give us



(σ

)

= ( ¯σ

)

(3.38)

This is what we were looking for: an expression showing us how the  matrices can

be used to raise the two indices of σ

Note two important points about this identity. First, the indices of σ

that are

raised appear in the second position of both . Second, note how the order of the

Supersymmetry Demystified

dotted and undotted indices changes in going from σ

to ¯σ

, obeying the deﬁnitions

in Eqs. (3.33) and (3.34).

The identity (3.38) will be very useful shortly. Of course, we can invert it [or, if

you prefer, start from the second relation of Eq. (2.23)] to show that



d ˙a

( ¯σ

)

˙ab

= (σ

)

(3.39)

Let us turn our attention to the ever-important issue of building quantities with

deﬁnite Lorentz transformation properties (invariants or tensors). The index assig-

nations (3.33) and (3.34) make it easy to write down such quantities. For example,

what contravariant four-vectors can we write down if we have two left-chiral Weyl

spinors χ and λ at our disposal? Simply by matching indices, we get the following

combinations:

¯χ

˙a

( ¯σ

)

˙ab

˙a

( ¯σ

)

˙ab

(σ

)

(σ

)

¯χ

(3.40)

However, only two of those four expressions are independent, as we will demon-

strate below. Before doing that, let’s introduce an index-free notation for these

expressions. Dropping the indices, we get, for example,

¯χ

˙a

( ¯σ

)

˙ab

= ¯χ ¯σ

λ (3.41)

As always when we drop indices, some ambiguity might arise. If we were to see

the right-hand side appearing in some formula, how would we know if we are to

assign upper or lower dotted indices to ¯χ? The answer is that we have to remember

that ¯σ

carries two upper indices. If we keep this in mind, then it is clear that the

right-hand side of Eq. (3.41) corresponds to the expression on the left-hand side.

Similarly, we must remember that σ

carries two lower indices. If we remember

this, no ambiguity is possible.

We now can rewrite the four terms of Eq. (3.40) unambiguously as

¯χ ¯σ

λ ¯σ

χχσ

λλσ

¯χ (3.42)

Now let’s show that these expressions are not all independent. Let’s start with

the ﬁrst one and use  matrices to express the spinors in terms of components with

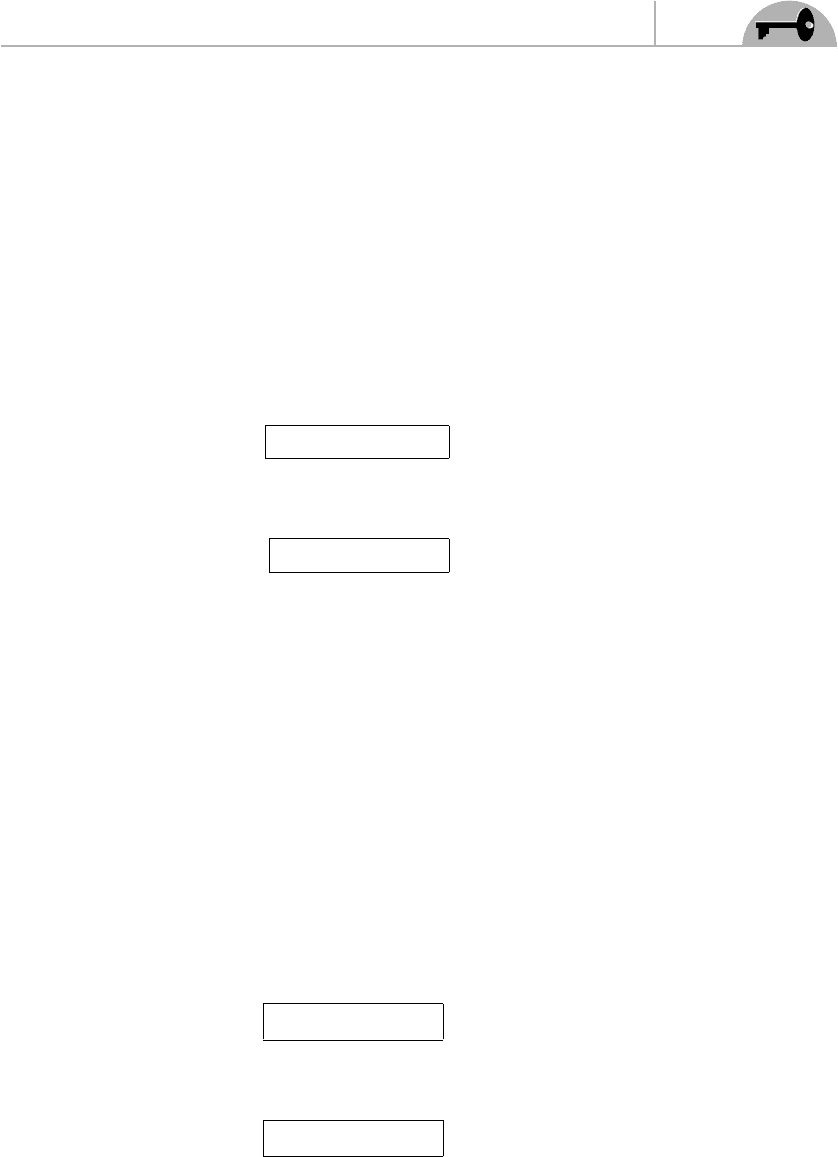
upper indices:

¯χ ¯σ

λ = ¯χ

˙a

( ¯σ

)

˙ab

= 

˙a ˙c

¯χ

˙c

( ¯σ

)

˙ab



CHAPTER 3 New Notation for Weyl Spinors

Now we contract the two  with the ¯σ

using Eq. (3.39) and see where it leads us:



˙a ˙c

¯χ

˙c

( ¯σ

)

˙ab



= 

˙c ˙a



( ¯σ

)

˙ab

¯χ

˙c

= (σ

)

d ˙c

¯χ

˙c

[using Eq. (3.39)]

=−λ

(σ

)

d ˙c

¯χ

˙c

=−λσ

¯χ

where in the ﬁrst step we have switched the indices of both , which produces a

factor of (−1)

= 1.

To summarize, we thus have shown that

¯χ ¯σ

λ =−λσ

¯χ (3.43)

By simply renaming λ ↔ χ, this, of course, also implies

χσ

λ =−

λ ¯σ

χ (3.44)

This conﬁrms the claim made earlier that only two of the four expressions in Eq.

(3.42) are independent.

Let’s now look at what happens if we take the hermitian conjugate of one of

these expressions, let’s say ¯χ ¯σ

λ. Keeping in mind the rule that when we take the

hermitian conjugate we switch the order of the components of the spinors without

introducing a minus sign, we get

( ¯χ ¯σ

λ)

†

= λ

†

( ¯σ

)

†

( ¯χ)

†

= λ

†

¯σ

( ¯χ)

†

(3.45)

using the fact that Pauli matrices are hermitian. But since taking the hermitian

conjugate on the components of a spinor simply changed undotted indices into

dotted indices, the right-hand side of Eq. (3.45) is simply

λ ¯σ

χ. We therefore have

established that

( ¯χ ¯σ

λ)

†

λ ¯σ

χ (3.46)

Similarly, it is easy to show that

(χ σ

λ)

†

= λσ

¯χ (3.47)

Supersymmetry Demystified

We’ll close this chapter with the derivation of (yet another!) identity (by the way,

all these identities we are proving will be useful at some point, I promise!). This

one starts with

(λ σ

¯χ)(λ σ

¯χ) (3.48)

The goal is to rewrite this as something proportional to (λ · λ)( ¯χ · ¯χ). At ﬁrst sight,

this might seem impossible. How can we get the spinors of the same type dotted

together when they appear in different matrix products in Eq. (3.48)? What could

bring about this kind of magic? The answer lies in the identities in Eq. (3.29).

To see how this works, we ﬁrst write Eq. (3.48) in terms of components and

move the components of the same type next to one another (recall that we pick up

a minus sign when we move a spinor component through another one and that the

components of the σ

matrices are plain numbers):

(λ σ

¯χ)(λ σ

¯χ) = λ

(σ

)

¯χ

(σ

)

¯χ

=−(σ

)

(σ

)

¯χ

Now, using Eq. (3.29), we may write this as

−(σ

)

(σ

)

¯χ

(σ

)

(σ

)



λ · λ ¯χ · ¯χ (3.49)

Switching the order of the indices in the two  matrices and using Eq. (3.38), we get

(σ

)

(σ

)



λ · λ ¯χ · ¯χ =



(σ

)

(σ

)

λ · λ ¯χ · ¯χ

( ¯σ

)

(σ

)

λ · λ ¯χ · ¯χ

By deﬁnition, we have

( ¯σ

)

(σ

)

= Tr( ¯σ

)

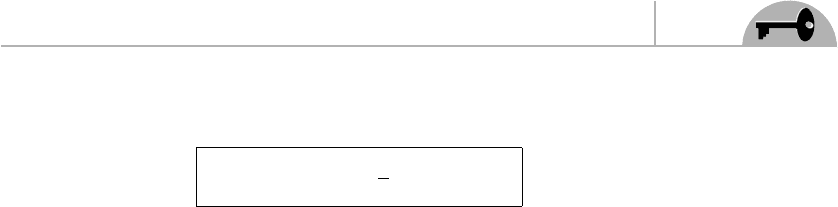
This trace turns out to be equal to 2η

μν

(see Exercise 3.5). Our ﬁnal result therefore is

(λ σ

¯χ)(λ σ

¯χ) =

μν

λ · λ ¯χ · ¯χ (3.50)

an identity which will be used several times.

CHAPTER 3 New Notation for Weyl Spinors

It’s clear that the same steps also lead to

( ¯χ ¯σ

λ)( ¯χ ¯σ

λ) =

μν

λ · λ ¯χ · ¯χ (3.51)

EXERCISE 3.5

Show that Tr( ¯σ

) = 2η

μν

. Hint: Use Eq. (2.24).

3.6 Quiz

1. Write the invariant ¯η

˙a

¯χ

˙a

in an index-free notation (two answers are accept-

able).

2. What are 

and 

equal to?

3. What can the expression 



(σ

)

be simpliﬁed to?

4. What is 

˙a



˙a ˙c

equal to?

5. What is the relationship between components with dotted indices and com-

ponents with undotted indices in the same position?

6. What matrix may be used to lower both dotted and undotted indices?

7. If we contract all the indices in 



, what do we get?