Labelle P. Supersymmetry DeMYSTiFied
Подождите немного. Документ загружается.


196
Supersymmetry Demystified
This is simply 4m times the expression we would have in a boson propagator, so
we may write
Tr[S(z − z)] = 4mD(z − z) (9.56)
Equation (9.54) is therefore
8g
2
m
2
d
4
zd
4
wD
B
(x −w )D
B
(y − w)D
A
(z − w)D(z − z)
AM
FT
=−8ig
2
I
d
(9.57)
where once more we have used Eq. (9.44).
Contribution from L
2
I4
The integral is [see Eqs. (9.39) and (8.71)]
d
4
wd
4
z0|T
"
B(x)B(y)
g
2
2
B(z)
(z)γ
5
(z) B(w)(w)γ
5
(w)
#
|0
(9.58)
Obviously, B(x) must be contracted with either B(w) or B(z), and B(y) must
be contracted with the remaining B. This gives two contributions. We get, after
contracting the B fields only,
g
2
d
4
wd
4
zD
B
(x − z)D
B
(y − w)0|T
α
(z)γ
5αβ
β
(z)
δ
(w)γ
5δρ
ρ
(w)
|0
(9.59)
where the spinor indices are shown explicitly.
If we contract the two spinors at z with each another and the two spinors at w
with each other, we get a disconnected graph. We therefore must contract the two
spinors at w with the two spinors at z. At this point, we must keep in mind that we
are dealing with Majorana fermions, so we have more possibilities than for a Dirac
CHAPTER 9 Some Explicit Calculations
197
fermion. Keeping this in mind, we get
0|T
α
(z)γ
5αβ
β
(z)
δ
(w)γ
5δρ
ρ
(w)
|0
=0|T
α
(z)γ
5αβ
β
(z)
δ
(w)γ
5δρ
ρ
(w)
|0
+0|T
α
(z)γ
5αβ
β
(z)
δ
(w)γ
5δρ
ρ
(w)
|0 (9.60)
Consider the second term first. This is a term we also would get for Dirac
fermions. Recall that in the fermion propagator (9.18), the appears to the left of
the Dirac conjugate field
, so we must move
ρ
(w) to the left of
α
(z). Every
time we move a fermion field passed a or a
, we pick up a minus sign, so we get
0|T
α
(z)γ
5αβ
β
(z)
δ
(w)γ
5δρ
ρ
(w)
|0
=−0|T
ρ
(w)
α
(z)γ
5αβ
β
(z)
δ
(w)γ
5δρ
|0 (9.61)
Now we can replace the contractions by fermion propagators, which gives us that
the second term of Eq. (9.60) is finally equal to
−S
ρα
(w − z)γ
5αβ
S
βδ
(z − w)γ
5δρ
=−Tr
S(w − z)γ
5
S(z − w)γ
5
(9.62)
We obtain a trace of the matrices because all the indices appear next to one another
except for the index ρ, which appears at the very beginning and at the very end.
Let us now turn our attention to the first term of Eq. (9.60). This is a contribution
that we would not have if we were considering Dirac fermions. In order to use the
propagators (9.25) and (9.26), we need to move the two next to one another and
the two
next to one another as well. In principle, we could use any order between
the two and between the two
, but the calculation will be much simpler if we
arrange the order so that we get a trace. Unfortunately, we can’t quite make it work.
For example, if we move the
δ
(w) to the left of
α
(z) and
ρ
(w) to the right of
β
(z),weget
0|T
α
(z)γ
5αβ
β
(z)
δ
(w)γ
5δρ
ρ
(w)
|0
=0|T
δ
(w)
α
(z)γ
5αβ
β
(z)
ρ
(w)γ
5δρ
|0 (9.63)
198
Supersymmetry Demystified
We see that the indices are not in order: There is a mismatch because of the indices
of the γ
5
matrix on the far right. The way out is of course to reverse the indices of
that matrix. This can be done by using the identity (valid for any matrix)
γ
5δρ
= (γ
T
5
)
ρδ
(9.64)
However, because γ
T
5
= γ
5
, we see that we are free to switch the order of its indices,
γ
5δρ
= γ
5ρδ
(9.65)
which allows us to write Eq. (9.63) as
0|T
δ
(w)
α
(z)γ
5αβ
β
(z)
ρ
(w)γ
5ρδ
|0
= C
T
δγ
S
γα
(w − z)γ
5αβ
S
βμ
(z − w)C
T
μρ
γ
5ρδ
= Tr
C
T
S(w − z)γ
5
S(z − w)C
T
γ
5
Now, it turns out that there is always a way to get rid of the C
T
matrices. The
trick is to use that C
T
=−C = C
−1
so that (C
T
)
2
=−1. In order to use this,
though, we need to get the two C
T
matrices next to one another. We will do this
in two steps. First, we use the invariance of the trace under cyclic permutations,
Tr(ABC D) = Tr(DABC) to write
Tr
C
T
S(w − z)γ
5
S(z − w)C
T
γ
5
= Tr
S(w − z)γ
5
S(z − w)C
T
γ
5
C
T
(9.66)
Next, we use the fact that γ
5
and C
T
commute; i.e., γ
5
C
T
= C
T
γ
5
(which can be
easily checked using their explicit representations), so we finally obtain
Tr
S(w − z)γ
5
S(z − w)C
T
γ
5
C
T
= Tr
S(w − z)γ
5
S(z − w)C
T
C
T
γ
5
=−Tr
S(w − z)γ
5
S(z − w)γ
5
(9.67)
which is equal to what we had obtained for the second term of Eq. (9.60) [see also
Eq. (9.62)]. Therefore, Eq. (9.60) is equal to
0|T
α
(z)γ
5αβ
β
(z)
δ
(w)γ
5δρ
ρ
(w)
|0=−2Tr
S(w − z)γ
5
S(z − w)γ
5
(9.68)
CHAPTER 9 Some Explicit Calculations
199
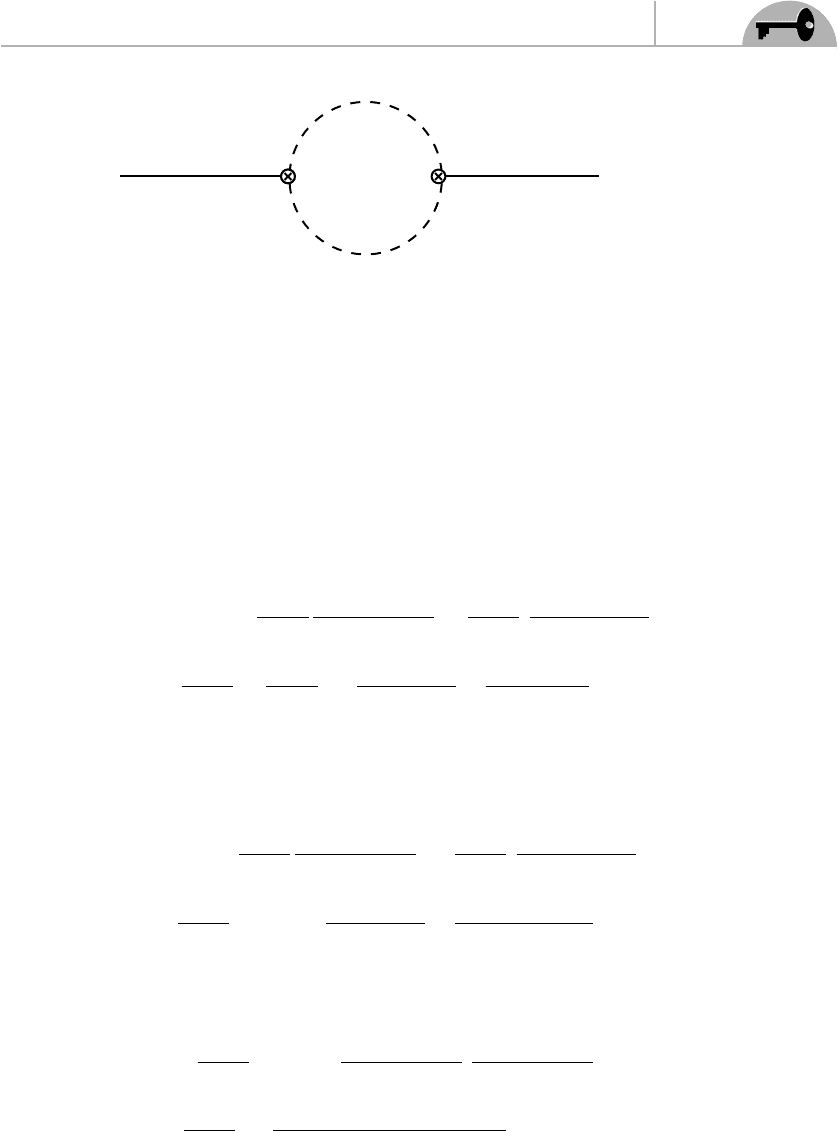
BB
Figure 9.11 Diagram corresponding to Eq. (9.69). The vertices drawn as a cross inside a
circle represent interactions containing a γ
5
matrix.
Plugging this back into the expression for the diagram, i.e., Eq. (9.59), we get
that the contribution of L
2
14
is
−2g
2
d
4
wd
4
zD
B
(x − z)D
B
(y − w)Tr
S(z − w)γ
5
S(w − z)γ
5
(9.69)
which is shown in Figure 9.11 and corresponds to the integral
−2g
2
d
4
wd
4
z
d
4
p
(2π)
4
ie
−ip·(x−z)
p
2
− m
2
+i
d
4
q
(2π)
4
ie
−iq·(y−w )
q
2
− m
2
+i
d
4
k
(2π)
4
d
4
l
(2π)
4
Tr
ie
−ik·(z−w )
/k − m +i
γ
5
ie
−il·(w−z)
/l − m + i
γ
5
(9.70)
Doing the integration over z gives a factor (2π)
4
δ
4
( p − k +l), which we may
use to integrate over d
4
l.Weget
−2g
2
d
4
w
d
4
p
(2π)
4
ie
−ip·x
p
2
− m
2
+i
d
4
q
(2π)
4
ie
−iq·(y−w )
q
2
− m
2
+i
d
4
k
(2π)
4
e
ip·w
Tr
i
/k − m +i
γ
5
i
/k − /p − m + i
γ
5
(9.71)
Doing the integral over w and then over q,weget
2g
2
d
4
p
(2π)
4
e
−ip·(x−y)
i
p
2
− m
2
+i
i
p
2
− m
2
+i
d
4
k
(2π)
4
Tr
"
(/k + m)γ
5
(/k − /p +m)γ
5
(k
2
− m
2
)[(k − p)
2
− m
2
]
#
200
Supersymmetry Demystified
To carry out the traces, we simply need to use (γ
5
)
2
= 1, γ
5
γ
μ
=−γ
μ
γ
5
,Tr(/a) = 0,
Tr(/aγ
5
/bγ
5
) =−4a · b, and obviously, Tr(1) = 4. This leads us to
8g
2
d
4
p
(2π)
4
e
−ip·(x−y)
i
p
2
− m
2
+i
i
p
2
− m
2
+i
×
d
4
k
(2π)
4
k · p − k
2
+ m
2
(k
2
− m
2
)[(k − p)
2
− m
2
]
(9.72)
This contains a quadratic divergence, but it is not easy to isolate it in that form. A
standard trick is to write the numerator as
k · p − k
2
+ m
2
=
−( p − k)
2
+ k
2
+ p
2
− 2k
2
+ 2m
2
2
=
−( p − k)
2
+ m
2
− k
2
+ m
2
+ p
2
2
(9.73)
so that the integral is
−4g
2
d
4
p
(2π)
4
e
−ip·(x−y)
i
p
2
− m
2
+i
i
p
2
− m
2
+i
×
d
4
k
(2π)
4
"
1
(k − p)
2
− m
2
+
1
k
2
− m
2
−
p
2
(k
2
− m
2
)[(k − p)
2
− m
2
]
#
In this form, it is clear that the quadratic divergence is isolated in the first two
terms. Ignoring the last term and making the change of variable k → k + p in the
first integral, we can finally see that the quadratically divergent contribution of this
diagram is
−8g
2
d
4
p
(2π)
4
e
−ip·(x−y)
i
p
2
− m
2
+i
i
p
2
− m
2
+i
d
4
k
(2π)
4
1
k
2
− m
2
AM
FT
= 8ig
2
I
d
(9.74)
As an aside, the change of variable we just performed is fine as long as we are only
interested in isolating the quadratic divergent contribution of the diagram. If we
were interested in extracting the finite contribution, we could not be as cavalier.

CHAPTER 9 Some Explicit Calculations
201
The Remaining Terms
There are still three terms we haven’t calculated: the terms in L
2
I 3
, L
I 2
L
I 4
, and
L
I 3
L
I 4
. However, none of these terms contribute connected diagrams to the one-
loop B propagator as you can easily verify.
9.5 Putting It All Together
Let us summarize our results. We will group the contributions into two groups:
the tadpole diagrams and the nontadpole diagrams. Our goal is to demonstrate that
quadratic divergences cancel out, so we don’t take into consideration the diagram
of Figure 9.7 which contains a logarithmic divergence.
For the tadpole diagrams, we found
A tadpole = 6ig
2
I
d
B tadpole = 2ig
2
I
d
Fermion tadpole =−8ig
2
I
d
Sum = 0 (9.75)
The results for the nontadpole diagrams are
A loop =−2ig
2
I
d
B loop =−6ig
2
I
d
Fermion loop = 8ig
2
I
d
Sum = 0 (9.76)
As advertised, all quadratic divergences cancel out!
The choice of grouping the contributions into tadpole versus nontadpole diagrams
to show the cancellation may seem arbitrary in this example. For example, we
could have grouped instead the fermion and scalar contributions separately and still
obtained a cancellation. However, there is an accidental symmetry here that obscures
the structure of the cancellations. For this reason, it is instructive to calculate as well
the one-loop quadratic divergent contributions to the A propagator. We will only
quote the results here, which you are invited to double-check (all the tricks needed
to do the calculation have been introduced in the calculation of the B propagator).

202
Supersymmetry Demystified
The one-loop quadratic divergent contributions to the propagator of A are, for
the tadpole diagrams,
A tadpole = 18ig
2
I
d
B tadpole = 6ig
2
I
d
Fermion tadpole =−24ig
2
I
d
Sum = 0 (9.77)
and for the non-tadpole diagrams, they are
A loop =−6ig
2
I
d
B loop =−2ig
2
I
d
Fermion loop = 8ig
2
I
d
Sum = 0 (9.78)
Again, there is one diagram that is only logarithmically divergent and therefore was
not included.
By looking at the results for the A propagator, it is now clear that the tadpole
diagrams cancel separately from the nontadpole contributions. These cancellations
are highly nontrivial. They obviously require a very subtle “conspiracy” between
all the parameters appearing in the lagrangian. Obviously, SUSY imposes very
stringent conditions on these parameters (not only at tree level, as we will discuss
in the next section). This is striking when we realize that there are three particles
and seven interaction terms but only one mass and one coupling constant in the
theory! Thus there are only two parameters, where we could have, in principle, 10
different parameters (three masses and seven coupling constants).
However, if we are only interested in having the quadratic divergences cancel, it
turns out that SUSY offers more constraints than what is necessary. For example,
consider leaving the dimensionless coupling constants in all the interactions the
same, as in SUSY, but let’s assign different symbols to the four different parameters
with a dimension of mass: the masses of the three particles m
A
, m
B
, and m
and
the dimensionful coupling constant that appears in the interaction L
I 2
, which we
will denote by m
C
.
The important point to notice is that the scalar masses m
A
and m
B
do not appear
at all in the quadratically divergent corrections to the propagator! On the other
hand, if we go over the calculation of the contribution from the fermion tadpole,
we can see that this diagram is actually proportional to m
C
/m
, and therefore, for
the quadratic divergences to cancel, it is essential that m
C
= m
.

CHAPTER 9 Some Explicit Calculations
203
The conclusion is that we can change the masses of the scalar particles with-
out disrupting the cancellation of quadratic divergences! This would clearly break
SUSY because the fermion and the scalar would no longer be degenerate in mass,
but it would not spoil the cancellation of the quadratic divergences. Of course, we
have only shown this for the very specific case of the one-loop corrections to the B
propagator. But a more detailed analysis shows that it is in fact valid to all orders
of perturbation theory and for all processes!
In addition, we also may modify some of the coupling constants of the various
interactions without spoiling the cancellations of the quadratic divergences. Thus,
as long as we are motivated only by the desire to cancel these divergences, we
have actually more leeway with the parameters of the theory than SUSY allows.
We could therefore add terms to the lagrangian that would break explicitly the
invariance under SUSY but which would preserve the cancellation of quadratic
divergences in the theory. Such terms are said to break SUSY softly. We will list all
the possible terms that break SUSY softly in Chapter 14. They will play a crucial
role in Chapters 15 and 16 because they are required to break SUSY in the MSSM.
9.6 A Note on Nonrenormalization Theorems
Unfortunately, we don’t have enough space to explore more in-depth the perturba-
tive properties of supersymmetric theories. The very simple examples we did had
for their only goal to give you a peek at the types of cancellations of ultraviolet
divergences that occur owing to SUSY.
However, this very short chapter does not do justice to the power of SUSY. Given
more space, we would discover not only that all quadratic divergences cancel to
all orders of perturbation theory but also that the only renormalization required
in the Wess-Zumino model is a common wavefunction renormalization of all the
fields (which involves only a logarithmic divergence). The mass m and the coupling
constant g do not get renormalized at all to any order in perturbation theory!
The theorems demonstrating that certain parameters in supersymmetric theories
do not get renormalized at all are known as nonrenormalization theorems. A proof
using a diagrammatic approach was worked out in Ref. 22. Seiberg
42
has derived
the same result using a very nifty trick that makes the proof extremely simple. The
basic idea is that the parameters of the theory, such as m and g in the Wess-Zumino
model, are treated as the expectation values of some new fields. Of course, these are
not physical fields but rather simply mathematical tricks to carry out the proof and
therefore are sometimes referred to as spurious fields or spurions. The introduction
of these spurious fields enhances the symmetry of the lagrangian, introducing an
additional U(1) symmetry. Using the superspace approach that we will cover in
later chapters and the holomorphicity of the potential, it is a simple matter to prove

204
Supersymmetry Demystified
that some interactions cannot get renormalized to any order in perturbation theory.
A simple illustration of this technique can be found in Section 3 of Ref. 43, and
more details are given in Section 8.2 of Ref. 8.
Let us also add that calculations of Feynman diagrams in SUSY are simplified
greatly by the use of so-called supergraphs. These graphs involve the propaga-
tors (or superpropagators) of the superfields that we will introduce in Chapter 11.
The end result is that the effect of all the field members of a supermultiplet are
taken into account simultaneously instead of having many diagrams with the var-
ious fields. This obviously simplifies the calculations greatly, and is a powerful
tool to build directly into the Feynman diagrams the highly nontrivial relations be-
tween the various terms imposed by SUSY. The supergraph approach therefore not
only simplifies calculations but also facilitates the proof of many results, including
nonrenormalization theorems. This is unfortunately beyond the scope of this book,
but if you are interested, you can find a nice introduction in Ref. 47 and a more
technical presentation in Ref. 49.
9.7 Quiz
1. What is the difference between the propagator of a Dirac fermion and the
propagator of a Majorana fermion?
2. How many different parameters are there in the Wess-Zumino model (for a
single multiplet)?
3. Are supersymmetric theories completely free of all divergences?
4. Give one example of a term we could add to the lagrangian that would break
SUSY but which would not spoil the cancellation of quadratic divergences.
5. What is meant by saying that an interaction breaks SUSY softly?
CHAPTER 10
Supersymmetric
Gauge Theories
The only theory we have built so far contained a spinor and a scalar field. If we are
to apply supersymmetry (SUSY) to real life, we need to deal with gauge theories.
As we saw in Section 7.4, a vector (massless) supermultiplet contains states with
helicities h = 1/2 and h = 1. Together with the corresponding CPT conjugates,
we therefore have four states with h =±1/2, ±1. The particle content is therefore
a massless fermion and a massless spin 1 particle. If we consider an abelian U (1)
gauge theory, this is all the particle content we need to construct the supersymmetric
version because there is only one gauge field. For nonabelian gauge theories, we
will, of course, need to introduce as many fermions as there are gauge fields, and
these fermions will have to transform in the same representation as the gauge fields.
But let’s not get ahead of ourselves, and let’s start by building a supersymmetric
abelian gauge theory. By the way, the term gauge multiplet is often used to denote
a vector multiplet, and we will use both terms interchangeably.
