Labelle P. Supersymmetry DeMYSTiFied

166

Supersymmetry Demystified

which, when used in Eq. (8.18), yields

W

11

=

∂W

1

∂φ

(8.20)

We have canceled all the terms except terms I and VII, i.e.,

I + VII =

∂W

1

∂φ

ζ · χ F − W

11

F χ · ζ

=



∂W

1

∂φ

− W

11



F ζ · χ (8.21)

where we have used ζ · χ = χ ·ζ . Obviously, this vanishes because of Eq. (8.20)!

We therefore have constructed a supersymmetric interaction lagrangian

L

int

= W

1

(φ) F −

1

2

W

11

(φ) χ ·χ + h.c.

= W

1

(φ) F −

1

2

∂W

1

(φ)

∂φ

χ ·χ + h.c. (8.22)

where we made explicit the fact that W

1

and W

11

may only depend on φ.

To have a renormalizable lagrangian, W

1

must be at most a quadratic function

of the scalar ﬁeld. The most general form is therefore

W

1

(φ) = mφ +

1

2

yφ

2

+C (8.23)

where m has dimension of a mass, y is dimensionless, and the factor of 1/2is

purely conventional.

The model we considered in this section is extremely simple. In the next section

we will generalize to a set of n multiplets with ﬁelds φ

i

,χ

i

, and F

i

, where i runs

from 1 to n. Most of what we did here will apply

verbatim, but there will be a few

nontrivial complications owing to the presence of several ﬁelds. We will see that it

proves convenient to introduce a new function of the scalar ﬁeld denoted by W(φ)

and related to W

1

by

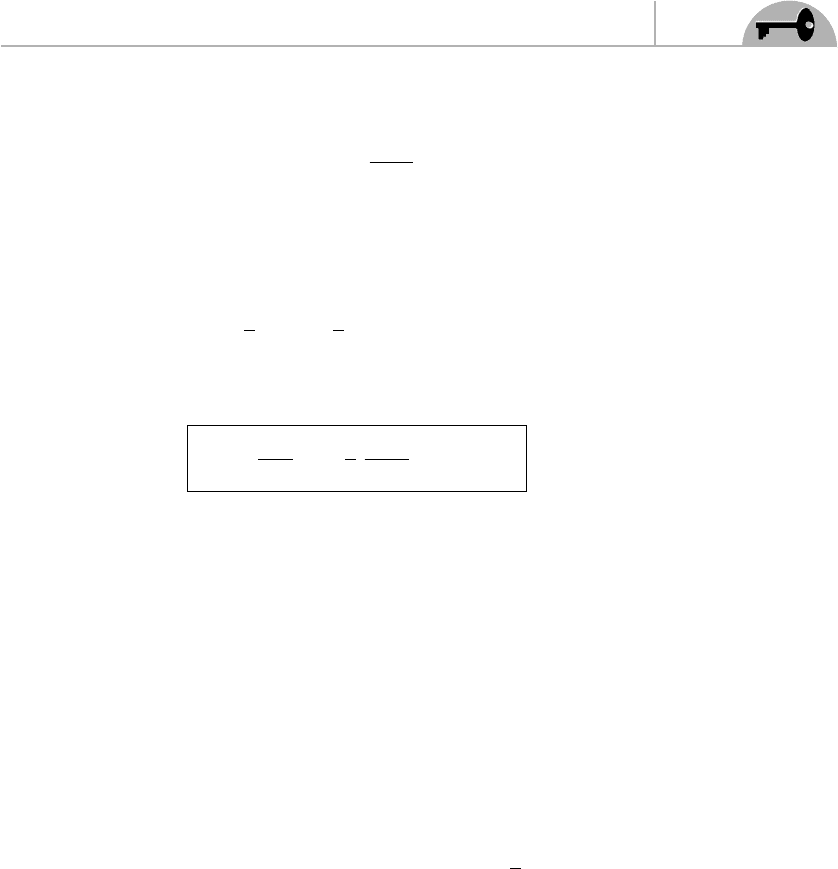
W

1

≡

∂W

∂φ

(8.24)

CHAPTER 8 The Wess-Zumino Model

167

which, using Eq. (8.20), implies

W

11

=

∂

2

W

∂φ

2

W is called the superpotential because it also will appear as a function of the

superﬁelds that will be introduced in Chapter 11. The superpotential corresponding

to the W

1

given in Eq. (8.23) is obviously

W =

1

2

m φ

2

+

1

6

y φ

3

+C φ + f (φ

†

) (8.25)

In terms of the superpotential, the interaction lagrangian (8.22) is given by

L

int

=

∂W

∂φ

F −

1

2

∂

2

W

∂φ

2

χ ·χ + h.c. (8.26)

The function f (φ

†

) appearing in the superpotential plays no role because only

derivatives of W with respect to φ appear in the lagrangian, so we may drop it and

take the superpotential to be holomorphic in φ. The linear term in φ usually is not

included but plays a role in the spontaneous symmetry breaking of SUSY, as we

will see in Chapter 14.

8.2 A More General Lagrangian

We now generalize the free lagrangian to a set of n copies of the ﬁelds. Such

a generalization will be useful when we consider the minimal supersymmetric

standard model (MSSM). The obvious generalization of the lagrangian is

L

WZ

= ∂

μ

φ

†

i

∂

μ

φ

i

+ χ

†

i

i ¯σ

μ

∂

μ

χ

i

+ F

†

i

F

i

+



W

i

F

i

−

1

2

W

ij

χ

i

· χ

j

+ h.c.



(8.27)

where the indices i and j are summed from 1 to n. We won’t require that this

index appear in the upper and lower positions to be summed over because it is not

a spacetime index. The functions W

i

and W

ij

are a priori arbitrary functions of all

the scalar ﬁelds φ

i

and their hermitian conjugate

W

i

= W

i

(φ

1

,φ

†

1

,... ,φ

n

,φ

†

n

) (8.28)

and similarly for W

ij

.

168

Supersymmetry Demystified

We take the ﬁelds of each multiplet to transform exactly as in Eq. (8.2):

δφ

i

= ζ · χ

i

=−ζ

T

iσ

2

χ

i

δφ

†

i

= ¯χ

i

·

¯

ζ = χ

†

i

iσ

2

ζ

∗

δχ

i

=−iσ

μ

iσ

2

ζ

∗

∂

μ

φ

i

+ F

i

ζ

δF

i

=−i ζ

†

¯σ

μ

∂

μ

χ

i

(8.29)

Note that even though there are n ﬁelds of each type in the lagrangian, there is still

only one spinor ζ appearing in the transformations.

The variation of the functions W

i

is generalized to

δW

i

(φ, φ

†

) =

∂W

i

∂φ

k

δφ

k

+

∂W

i

∂φ

†

k

δφ

†

k

(8.30)

=

∂W

i

∂φ

k

ζ · χ

k

+

∂W

i

∂φ

†

k

¯

ζ · ¯χ

k

(8.31)

with a similar expression for the variation of W

ij

.

We therefore have

δ



W

i

(φ, φ

†

) F

i

+ h.c.



=

∂W

i

∂φ

k

ζ · χ

k

F

i

+

∂W

i

∂φ

†

k

¯

ζ · ¯χ

k

F

i

+ W

i

δF

i

+ h.c.

=

∂W

i

∂φ

k

ζ · χ

k

F

i

+

∂W

i

∂φ

†

k

¯

ζ · ¯χ

k

F

i

−iW

i

ζ

†

¯σ

μ

∂

μ

χ

i

+ h.c. (8.32)

and, similarly,

δ



−

1

2

W

ij

χ

i

· χ

j

+ h.c.



=−

1

2

∂W

ij

∂φ

k

ζ · χ

k

χ

i

· χ

j

−

1

2

∂W

ij

∂φ

†

k

¯

ζ · ¯χ

k

χ

i

· χ

j

−

1

2

W

ij

δ(χ

i

· χ

j

) + h.c. (8.33)

We are looking for conditions on W

i

and W

ij

such that the sum of Eqs. (8.32)

and (8.33) vanishes, modulo total derivatives. We will focus on the terms written

out explicitly and not worry about their hermitian conjugates because if they cancel

out, their hermitian conjugates will too.

CHAPTER 8 The Wess-Zumino Model

169

Terms with Four Spinors

Consider the ﬁrst term of Eq. (8.33):

−

1

2

∂W

ij

∂φ

k

ζ · χ

k

χ

i

· χ

j

(8.34)

There is no other term of this form. When the three indices are equal, i = j = k,

it is identically zero because it contains the square of spinor components. What

happens when the indices are different? Do we have to set W

ij

equal to a constant?

Actually, this would be too restrictive. To see this, consider identity (A.53). In order

to use this identity, we must keep in mind that the indices i, j, and k are summed

over. Consider, for example, the three terms

∂W

12

∂φ

3

ζ · χ

3

χ

1

· χ

2

+

∂W

31

∂φ

2

ζ · χ

2

χ

3

· χ

1

+

∂W

23

∂φ

1

ζ · χ

1

χ

2

· χ

3

(8.35)

Identity (A.53) tells us that

ζ · χ

3

χ

1

· χ

2

+ ζ · χ

2

χ

3

· χ

1

+ ζ · χ

1

χ

2

· χ

3

= 0 (8.36)

so the three terms of Eq. (8.35) will cancel out at the condition that

∂W

12

∂φ

3

=

∂W

31

∂φ

2

=

∂W

23

∂φ

1

(8.37)

It’s clear that if we assume cyclic symmetry of ∂ W

ij

/∂φ

k

under the permutation of

the three indices i, j, and k, we will have automatic cancellation of Eq. (8.34), all

the terms canceling in groups of three.

As for the second term of Eq. (8.33), there is no identity to save us this time, so

we must impose

∂W

ij

∂φ

†

k

= 0 (8.38)

that is, W

ij

must be a holomorphic function of the φ

k

.

In order for W

ij

χ

i

· χ

j

to be of dimension 4 or less, the most general holomorphic

W

ij

we can write down is

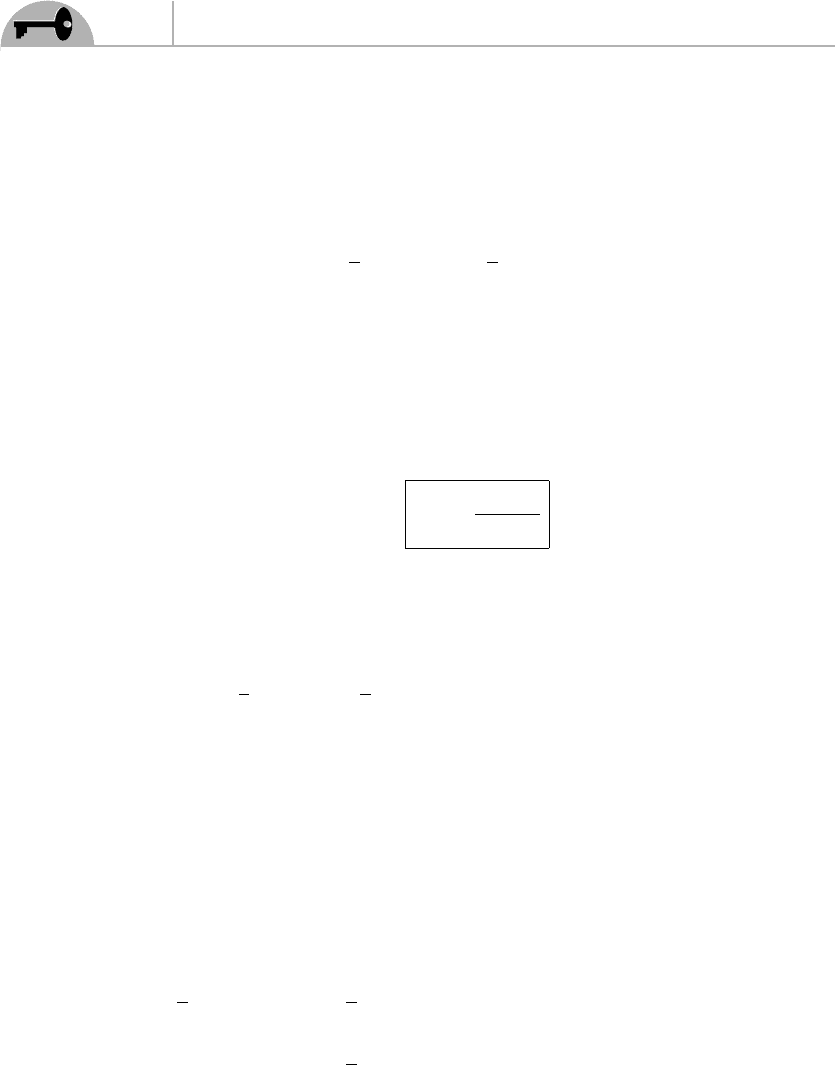
W

ij

= m

ij

+ y

ijk

φ

k

(8.39)

170

Supersymmetry Demystified

If we impose that ∂ W

ij

/∂φ

k

be invariant under cyclic permutation of the indices, we

must take the couplings y

ijk

to have cyclic symmetry, whereas there is no required

symmetry on the constants m

ij

. The end result is that the part of L

int

containing

W

ij

is given by

−

1

2

m

ij

χ

i

· χ

j

−

1

2

y

ijk

φ

k

χ

i

· χ

j

(8.40)

But because χ

i

· χ

j

is symmetric under the exchange of i and j , we can always

make m

ij

symmetric too. Thus, from now on, we take m

ij

to be symmetric. With

this choice, W

ij

is symmetric under the exchange of its indices.

Now comes the key trick: We can automatically make W

ij

symmetric if we write

it as the second derivative of another function of the scalar ﬁelds, i.e.,

W

ij

≡

∂

2

W

∂φ

i

∂φ

j

(8.41)

where W is, of course, the superpotential we encountered previously. Its most

general form is

W =

1

2

m

ij

φ

i

φ

j

+

1

6

y

ijk

φ

i

φ

j

φ

k

+ c

ij

φ

†

i

φ

j

+ c

i

φ

i

+ f (φ

†

i

) (8.42)

where f is an arbitrary function of the φ

†

i

(at most cubic). We will show below that

the constants c

ij

actually must be equal zero. The term linear in φ cannot be ruled

out, and it is actually considered in certain forms of SUSY breaking.

Last Term of Eq. (8.33)

This term is

−

1

2

W

ij

δ(χ

i

· χ

j

) =

1

2

W

ij

δ



χ

T

i

iσ

2

χ

j



=

1

2

W

ij



−



iσ

μ

iσ

2

ζ

∗

∂

μ

φ

i



T

iσ

2

χ

j

+ F

i

ζ

T

iσ

2

χ

j

−χ

T

i

iσ

2

iσ

μ

iσ

2

ζ

∗

∂

μ

φ

j

+ F

j

χ

T

i

iσ

2

ζ



(8.43)

CHAPTER 8 The Wess-Zumino Model

171

Now we use identity (A.36) applied to the ﬁrst term. For an arbitrary matrix A, this

identity allows us to write

(A ζ)

T

(iσ

2

χ) =−(iσ

2

χ)

T

A ζ =−χ

T

(iσ

2

)

T

A ζ = χ

T

iσ

2

A ζ (8.44)

Therefore, the ﬁrst term may be written as



iσ

μ

iσ

2

ζ

∗

∂

μ

φ

i



T

iσ

2

χ

j

= i χ

T

j

iσ

2

σ

μ

iσ

2

ζ

∗

∂

μ

φ

i

(8.45)

Substituting this into Eq. (8.43), we get

−

1

2

W

ij

δ(χ

i

· χ

j

) =−

i

2

W

ij

χ

T

j

iσ

2

σ

μ

iσ

2

ζ

∗

∂

μ

φ

i

+

W

ij

2

F

i

ζ

T

iσ

2

χ

j

−

i

2

W

ij

χ

T

i

iσ

2

σ

μ

iσ

2

ζ

∗

∂

μ

φ

j

+

W

ij

2

F

j

χ

T

i

iσ

2

ζ (8.46)

We see that the ﬁrst and third terms are identical (since the indices i and j are

summed over).

It turns out that the second and fourth terms of Eq. (8.46) are also identical. This

is obvious if we recall that

ζ

T

iσ

2

χ

j

=−ζ · χ

j

(8.47)

whereas χ

T

i

iσ

2

ζ =−χ

i

· ζ and ζ · χ = χ ·ζ .

Using this, we obtain

−

1

2

W

ij

δ(χ

i

· χ

j

) =−iW

ij

χ

T

j

iσ

2

σ

μ

iσ

2

ζ

∗

∂

μ

φ

i

− W

ij

F

i

ζ · χ

j

(8.48)

This can be simpliﬁed even further using Eq. (A.5) and then Eq. (A.36) to write

χ

T

j

σ

2

σ

μ

σ

2

ζ

∗

= χ

T

j

( ¯σ

μ

)

T

ζ

∗

=−ζ

†

¯σ

μ

χ

j

so that Eq. (8.48) simpliﬁes to

−

1

2

W

ij

δ(χ

i

· χ

j

) =−iW

ij

ζ

†

¯σ

μ

χ

j

∂

μ

φ

i

− W

ij

F

i

ζ · χ

j

(8.49)

172

Supersymmetry Demystified

Remaining Terms

We are left with the terms of Eqs. (8.32) and (8.49):

−iW

ij

ζ

†

¯σ

μ

χ

j

∂

μ

φ

i

− W

ij

F

i

ζ · χ

j

+

∂W

i

∂φ

k

ζ · χ

k

F

i

+

∂W

i

∂φ

†

k

¯

ζ · ¯χ

k

F

i

−iW

i

ζ

†

¯σ

μ

∂

μ

χ

i

(8.50)

All these must cancel out, modulo total derivatives.

Consider ﬁrst the terms that contain derivatives acting on some ﬁeld, which are

the ﬁrst and last terms. These are very similar. To show even more clearly their

relationship, we may use the fact that

W

ij

=

∂

2

W

∂φ

i

∂φ

j

(8.51)

to write

W

ij

∂

μ

φ

i

=

∂

2

W

∂φ

i

∂φ

j

∂

μ

φ

i

= ∂

μ



∂W

∂φ

j



(8.52)

So the sum of the ﬁrst and last terms of Eq. (8.50) is

−i ∂

μ



∂W

∂φ

j



ζ

†

¯σ

μ

χ

j

−iW

i

ζ

†

¯σ

μ

∂

μ

χ

i

(8.53)

This is a total derivative at the condition that

W

i

=

∂W

∂φ

i

(8.54)

We see that both W

ij

and W

i

are given in terms of the superpotential [see Eq. (8.41].

Now we are left with three terms in Eq. (8.50):

−W

ij

F

i

ζ · χ

j

+

∂W

i

∂φ

k

ζ · χ

k

F

i

+

∂W

i

∂φ

†

k

¯

ζ · ¯χ

k

F

i

(8.55)

Note that Eqs. (8.54) and (8.41) imply that

W

ij

=

∂W

i

∂φ

j

(8.56)

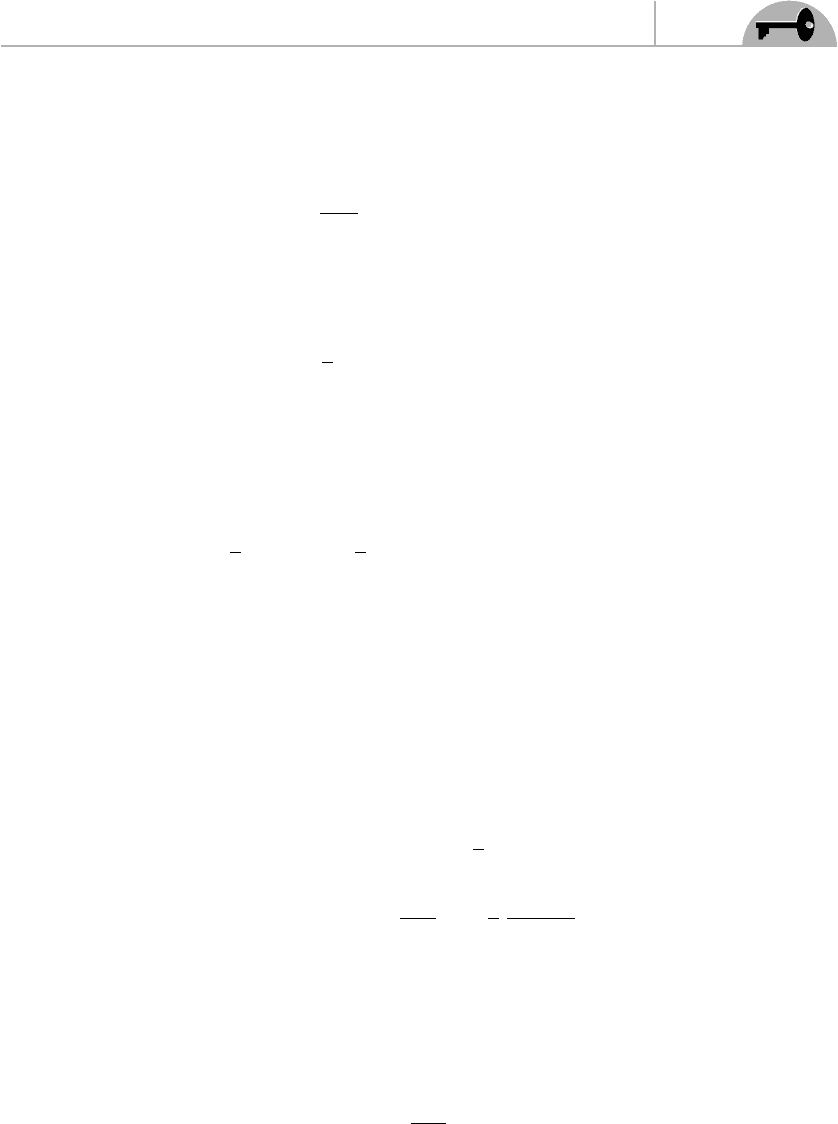
CHAPTER 8 The Wess-Zumino Model

173

so the ﬁrst two terms of Eq. (8.55) cancel out. We are left with the last term.

Since we can’t cancel this with anything and it is not a total derivative, W

i

must be

independent of φ

†

, i.e.,

∂W

i

∂φ

†

j

= 0 (8.57)

If we plug the most general form of the superpotential Eq. (8.42) into Eq. (8.54),

we get

W

i

= m

ij

φ

j

+

1

2

y

ijk

φ

j

φ

k

+ c

ij

φ

†

j

+ c

i

(8.58)

We see that Eq. (8.57) implies that c

ij

= 0.

Since the lagrangian contains only derivatives of the superpotential with respect

to the ﬁelds φ

i

, we also may set the function f (φ

†

i

) = 0 in Eq. (8.42) so that the

most general superpotential is the holomorphic function

W =

1

2

m

ij

φ

i

φ

j

+

1

6

y

ijk

φ

i

φ

j

φ

k

+ c

i

φ

i

(8.59)

And we are done! We have obtained a supersymmetric interacting theory. We

will summarize our results in the next section.

8.3 The Full Wess-Zumino Lagrangian

We have found that the following lagrangian, referred to as the Wess-Zumino la-

grangian, is supersymmetric:

L

WZ

= ∂

μ

φ

†

i

∂

μ

φ

i

+ χ

†

i

i ¯σ

μ

∂

μ

χ

i

+ F

†

i

F

i

+



W

i

F

i

−

1

2

W

ij

χ

i

· χ

j

+ h.c.



= ∂

μ

φ

†

i

∂

μ

φ

i

+ χ

†

i

i ¯σ

μ

∂

μ

χ

i

+ F

†

i

F

i

+



∂W

∂φ

i

F

i

−

1

2

∂

2

W

∂φ

j

∂φ

i

χ

i

· χ

j

+ h.c.



(8.60)

where we have used Eqs. (8.54) and (8.41).

Let us now eliminate the auxiliary ﬁelds. Using the equation of motion for F

i

,

∂L/∂ F

i

= 0, we get

F

i

=−W

i

=−

∂W

∂φ

i

174

Supersymmetry Demystified

On the other hand, the equation of motion for F

†

i

gives

F

i

=−W

†

i

=−



∂W

∂φ

i



†

so the two terms containing the auxiliary ﬁelds combine to form

F

†

i

F

i

+

∂W

∂φ

i

F

i

+



∂W

∂φ

i



†

F

†

i

=−



∂W

∂φ

i



2

(8.61)

Substituting this expression in the lagrangian, we get a different representation

for the Wess-Zumino lagrangian:

L

WZ

= ∂

μ

φ

†

i

∂

μ

φ

i

+ χ

†

i

i ¯σ

μ

∂

μ

χ

i

−



∂W

∂φ

i



2

−

1

2



∂

2

W

∂φ

j

∂φ

i

χ

i

· χ

j

+ h.c.



(8.62)

We see that the potential of the scalar ﬁelds is given by (recall L = K − V )

V (φ

i

) =



∂W

∂φ

i



2

(8.63)

Here, we are including the mass terms of the scalar ﬁeld in the potential.

Using the general superpotential Eq. (8.59), we have

W

i

= m

ij

φ

j

+

1

2

y

ijk

φ

j

φ

k

+ c

i

(8.64)

and W

ij

= m

ij

+ y

ijk

φ

k

so that the lagrangian is

L

WZ

= ∂

μ

φ

†

i

∂

μ

φ

i

+ χ

†

i

i ¯σ

μ

∂

μ

χ

i

−



m

ij

φ

j

+

y

ijk

2

φ

j

φ

k

+ c

i



2

−

1

2

(m

ij

χ

i

· χ

j

+ y

ijk

φ

k

χ

i

· χ

j

+ h.c.)

The coefﬁcients c

i

are usually set to zero, although they play a role in SUSY

breaking, as we will discuss in Chapter 14.

We see that the spinor and scalar masses are equal as expected. Also, we see

that there are three types of interactions: a cubic scalar interaction, a quartic scalar

CHAPTER 8 The Wess-Zumino Model

175

interaction, and a scalar-fermion interaction whose coupling constants are related

in a very speciﬁc way in order to ensure invariance under SUSY.

8.4 The Wess-Zumino Lagrangian

in Majorana Form

In Chapter 9 we will carry out explicit calculations in the Wess-Zumino model.

Although it is, of course, possible to carry out calculations with Weyl spinors (see

in particular Ref. 13), most references use Majorana spinors for calculations of

scattering amplitudes, as well as real scalar ﬁelds. We will therefore rewrite the

Wess-Zumino model in terms of Majorana spinors and real ﬁelds. Let’s consider

for simplicity the case of a single multiplet, for which (setting the linear term cφ

in the superpotential equal to zero)

W =

m

2

φ

2

+

y

6

φ

3

W

1

= m φ +

y

2

φ

2

W

11

= m + y φ (8.65)

If we don’t get rid of the auxiliary ﬁelds, the lagrangian is

L

WZ

= ∂

μ

φ

†

∂

μ

φ +χ

†

i ¯σ

μ

∂

μ

χ + FF

†

+ m φ F +

1

2

y φ

2

F +m φ

†

F

†

+

y

2

(φ

†

)

2

F

†

−

m

2



χ ·χ + ¯χ · ¯χ



−

y

2



φχ ·χ + φ

†

¯χ · ¯χ



(8.66)

where we have taken the parameters m and y to be real. If we use the equations of

motion of F and F

†

to get rid of them, we get

L

WZ

= ∂

μ

φ

†

∂

μ

φ +χ

†

i ¯σ

μ

∂

μ

χ −m

2

φφ

†

−

my

2



φ

2

φ

†

+ φφ

†2



−

y

2

4

(φ φ

†

)

2

−

m

2



χ ·χ + ¯χ · ¯χ



−

y

2



φχ· χ +φ

†

¯χ · ¯χ



(8.67)

It is conventional to rewrite the complex scalar ﬁeld in terms of two real scalars

conventionally named A and B according to

φ ≡

1

√

2

(A + iB) (8.68)

Labelle P. Supersymmetry DeMYSTiFied

Подождите немного. Документ загружается.