Kounadis A.N., Gdoutos E.E. (Eds.) Recent Advances in Mechanics

Подождите немного. Документ загружается.

Scaling of Strength and Lifetime Distributions of Quasibrittle Structures 49

= n

+...+p



(1)



∏

i=1

−1



...dy

(9)

where

(S) is the feasible region of stresses in all the ﬁbers, which deﬁnes an n

dimensional space, and

(1) is the corresponding feasible region of normalized

stresses, y

/S.

Thus, regardless of the post-peak slope E

of each ﬁber, it is proven that, if each

ﬁber strength has a cdf with a power-law tail, then the cdf of bundle strength will also

have a power-law tail, and the power-law exponent will be the sum of the exponents

of the power-law tails of the cdf’s of all the ﬁbers in the bundle.

The reach of power-law tail of the strength cdf of softening bundle was shown to

be another important consideration [13]. It can be calculated from Eq. 9. However,

for large bundles, it is difﬁcult to handle the integral in Eq. 9 numerically. Previous

studies [12, 13] showed that the reach of power-law tail decreases with the num-

ber n

of elements rapidly as P

∼ (P

)

−(P

/3n

)

for brittle bundles, or

)

for plastic bundles, where P

= failure probability at the terminal point of

the power-law tail of one ﬁber. Since the behavior of softening bundles is bounded

between these two extreme cases, the rate of shortening of power-law tail of strength

cdf of the softening bundles must lie between them; i.e.

∼ (P

)

−(P

/3n

)

(10)

The series coupling, by contrast, was shown to extend the power-law tail [13]—

roughly by one order of magnitude for each ten-fold increase in the number links.

Another important asymptotic property is the type of cdf of strength of large bun-

dles. For brittle bundles, Daniels derived a recursive equation for the strength cdf of

a bundle with n

ﬁbers and showed that the strength cdf of large bundles approaches

the Gaussian (or normal) distribution [20]. This property is obviously also true for

plastic bundles; it is a natural consequence of the Central Limit Theorem since the

strength of a plastic bundle is the sum of strengths of all the ﬁbers.

To prove that this asymptotic property applies to all the bundles regardless of

their post-peak softening stiffness E

, consider a bundle of 3n

ﬁbers (or elements).

The load carried by the bundle is given by F(

)=max



∑

j=2

(



,where

A = cross section area of each ﬁber,

= stress in jth element, and

= strain in

each element. The mechanical behavior of each ﬁber can be random. This causes

the randomness of the critical value

∗

of strain

,atwhichF reaches its maximum

value. We label the 3n

elements by j = 2, 3,4, ...3n

+1, arrange them according to

their breaking order, and divide them into two groups with different load resultants:

(

∑

i=3k

(

)A, F

(

∑

i=3k±1

(

)A (k = 1,2,3,...n

). (11)

The maximum load carried by the bundle is:

max

= F

(

∗

)+F

(

∗

) (12)

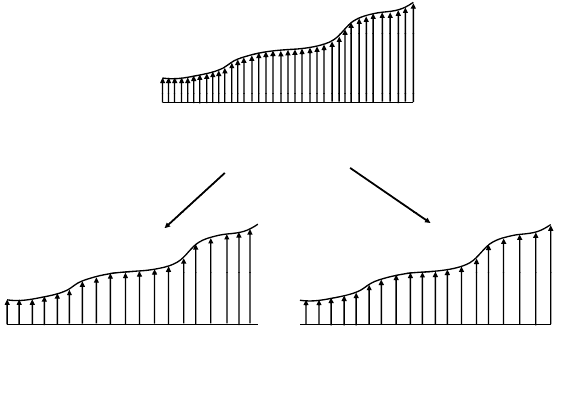
50 Z.P. Baˇzant and J.-L. Le

…

Stress distribution in the bundle

…

Stress distribution in

sub

bundle

Stress distribution in

sub

bundle

sub

bundle

sub

bundle

Fig. 3. Stress distribution of ﬁbers within a large bundle.

If n is large, then the stress distribution over the elements in these two groups will be

similar to that in the bundle (Fig. 3). It follows that the cdf of F

max

(i.e. the strength

of bundle) and the cdf’s of F

(

∗

) and F

(

∗

) are of the same type. Then, to satisfy

Eq. 12, the only possible distribution of F

max

is the Gaussian distribution (note that

this argument would not apply if the we divided the bundle into 2 groups with the

same number of elements and the same resultant for large n

However, the rate of convergence depends on the post-peak softening stiffness E

of the elements. The slowest convergence, of the order of O(n

−1/3

(logn

)

) [48],

occurs for brittle bundles. The fastest convergence, of the order of O(n

−1/2

), occurs

for plastic bundles [13].

To ﬁgure out the type of cdf of strength of one RVE, one must specify the me-

chanical behavior of the bundles in the hierarchical model. Although different as-

sumptions yield about the same results, here the following assumption is made: For

the bundles at the lowest scale, the elements are connected between two rigid plates,

and thus they are subjected to the same deformation. For the bundles at higher scales,

they follow the equal-load sharing mechanism.

Note that one should calculate the strength cdf in a hierarchical manner, at the

lowest scale, each element represents a nano-structure whose strength cdf is a power-

law function. One can then calculate the strength cdf of the sub-chain that connects

these elements. At the next scale, the strength cdf of the sub-bundle, which consists

of these sub-chains, can be calculated based on the strength cdf of these sub-chains.

Following in this manner, one could move up through the scales, and ﬁnally obtain

the strength cdf of one RVE.

Scaling of Strength and Lifetime Distributions of Quasibrittle Structures 51

The strength cdf of all the sub-chains follows from the joint probability theorem.

For the bundles at the lowest scale, where all the elements are subjected to a roughly

uniform deformation, the strength cdf will depend on the mechanical behavior of

each element, which is given by Eq. 8, and for the bundles at higher scales, equal-

load sharing is assumed. Regardless of the mechanical behavior of each element,

the strength of a bundle with the equal-load sharing mechanism is given by:

= max



−1

,...,



(13)

where

,...

are the strength values ordered by the sequence of their break-

ing. In fact, the strength distribution of such a bundle is exactly the same as the

strength distribution of the brittle bundle. Therefore, the strength distribution of

the equal-load sharing bundle can be calculated by Daniels’ recursive equation

[20, 28, 13].

As an example, we calculate the strength cdf of the hierarchical model shown in

Fig. 1. Every element in the hierarchical model represents one nanoscale element,

which has a power-law cdf of strength. Three cases are considered:

1) Each element has an elastic-brittle behavior.

2) Each element exhibits a linear post-peak softening, where the softening

modulus magnitude is 40% of the elastic modulus of the element.

3) Each element has an elastic-plastic behavior.

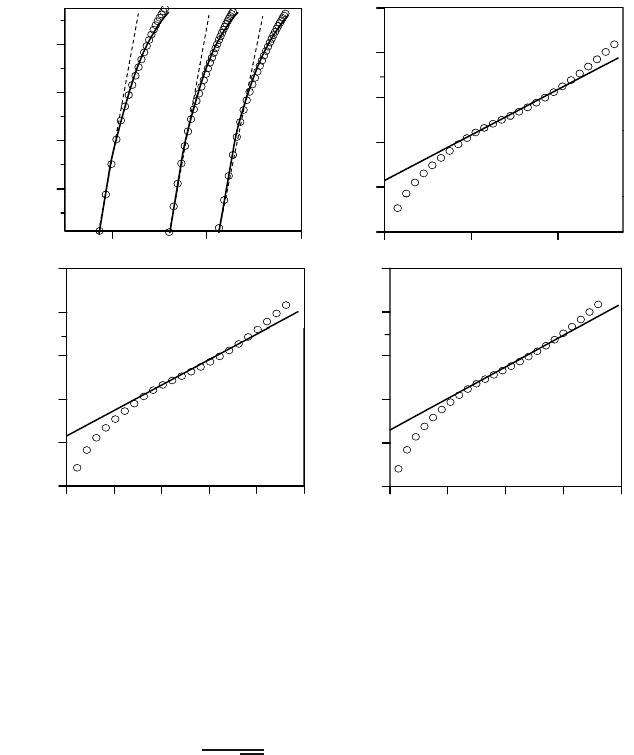
Fig. 4a shows the calculated strength cdf’s of the hierarchical model for these three

cases on the Weibull scale. For all these cases, the lower portion of the calculated

strength cdf is a straight line on the Weibull plot, which indicates that it follows the

Weibull distribution (a power-law tail). Such a property is expected since the power-

law tail of the cdf of strength is indestructible in the chain and bundle models.

For the upper portion, the strength cdf deviates from the straight line in Fig. 4a.

Among the three cases considered, case 1 (i.e., elements with brittle behavior) gives

the shortest Weibull tail, which terminates at the probability of about 0.7 ×10

−4

while case 3 (elements with plastic behavior) gives the longest Weibull tail, which

terminates at the probability of about 0.7 ×10

−3

To identify the type of distribution for the upper portion of the cdf, the cdf’s

of strengths are plotted on the normal probability paper; see Figs. 4b-d where the

upper portion of the cdf’s is seen to be ﬁtted quite closely by a straight line. The

straight line not is not too close for case 1 and for P

≥ 0.8. For the cases 2 and 3,

the straight line ﬁts quite closely, with a slight deviation occurring only for P

≥

0.99. This means that, the upper portion of the strength cdf can be approximated as

the Gaussian distribution. Since, for real quasibrittle structures, the nano-element is

expected to have a softening behavior, the strength cdf of one RVE should be close

to case 2.

In general, the strength distribution of one RVE can be approximately described

by a Gaussian distribution with a Weibull tail grafted on the left at the probability of

52 Z.P. Baˇzant and J.-L. Le

0.999968

)

]}

-4

0.5

0.9772

/(1



)

-12

-8

6.22u10

-16

3.16u10

-5

ln{ln[1

Brittle

Softening

-4 -2 0

00.020.04

Brittle

1.77u10

-33

Softening

Plastic

0.999968

316

0.5

0.9772

0.5

0.9772

Sft i

6.22u10

-16

6.22u10

-16

3.16u10

-5

0 0.05 0.1 0.15 0.2 0.25

0 0.2 0.4 0.6 0.8

Plastic

1.77u10

-33

1.77u10

-33

Fig. 4. a) Calculated cdf of strength of one RVE on the Weibull scale, b)–d) Calculated cdf

of strength of one RVE on the normal distribution paper.

about 10

−4

−10

−3

. The grafted cdf of strength of one RVE may be mathematically

described as [12, 13]:

(

)=1 − e

−(

)

(

≤

) (14)

(

)=P

√



−(



−

)



(

) (15)

where

= nominal strength, which is a maximum load parameter of the dimension

of stress. In general,

= c

max

/bD or c

max

for two- or three-dimensional

scaling (P

max

= maximum load of the structure or parameter of load system, c

parameter chosen such that

represents the maximum principal stress in the struc-

ture, b = structure thickness in the third dimension, D = characteristic structure di-

mension or size). Furthermore, m (Weibull modulus) and s

are the shape and scale

parameters of the Weibull tail, and

and

are the mean and standard deviation of

the Gaussian core if considered extended to −∞; r

is a scaling parameter required

to normalize the grafted cdf such that P

(∞)=1, and P

= grafting probability =

1 −exp[−(

)

]. Finally, continuity of the probability density function at the

grafting point requires that (dP

=(dP

−

Scaling of Strength and Lifetime Distributions of Quasibrittle Structures 53

4 Lifetime Distribution of One RVE

It has recently been shown [8, 33, 5] that one can derive the lifetime cdf of one

RVE by using the power law for creep crack growth, which has been empirically

described as [23, 51, 24, 15, 14, 4, 37]:

˙a = Ce

−Q

/kT

(16)

where C,n = empirical constant, Q

= activation energy, k = Boltzmann’s constant,

T = absolute temperature, K = stress intensity factor. Recent studies [8, 33] showed

that, under certain plausible assumptions, the power law for creep crack growth can

be physically justiﬁed on the basis of atomistic fracture mechanics and a multi-scale

transition framework of fracture kinetics.

Now consider one RVE undergoing strength and lifetime tests, where a linearly

ramped load is applied in the strength test and a constant load is applied in the

lifetime test. By applying Eq. 16 to these two cases, one ﬁnds the relation between

the strength and lifetime of one RVE as:

βσ

n/(n+1)

1/(n+1)

(17)

where

= nominal strength of RVE,

= P/l

= applied nominal stress in the

lifetime test (P = applied load), l

= RVE size,

= lifetime of RVE,

=[r(n +

1)]

1/(n+1)

,andr = rate of loading in the strength test. Since the distribution of RVE

strength is given by Eqs. 14 and 15, the lifetime distribution of one RVE can be

easily obtained by substituting Eq. 17 for

of Eqs. 14 and 15:

for

: P

(

)=1 −exp[−(

)

¯m

]; (18)

for

≥

: P

(

)=P

√



γλ

1/(n+1)

γλ

1/(n+1)

−(



−

)



(19)

where

βσ

n/(n+1)

−(n+1)

−n

n+1

N,gr

, s

= s

n+1

−(n+1)

−n

,and ¯m =

m/(n + 1). Similar to the strength distribution of one RVE, the lifetime cdf of one

RVE also has a Weibull tail (power-law tail). However, the rest of the lifetime cdf

of one RVE does not follow the Gaussian distribution. Note that the grafting proba-

bility P

for the lifetime distribution of one RVE is the same as that for the strength

cdfofoneRVE.

5 Finite Weakest Link Model and Optimum Fits of Histograms

To analyze softening damage and failure, the RVE must be deﬁned as the smallest

material volume whose failure triggers the failure of entire structure [12, 13]. There-

fore, the structure can be statistically represented by a chain of RVEs. By virtue of

the joint probability theorem, and under the assumption of independence of random

strengths or lifetimes of the links in a ﬁnite weakest-link model, one can calculate

the strength or lifetime cdf of a structure as:

54 Z.P. Baˇzant and J.-L. Le

(x)=1 −

∏

i=1

[1 −P

(x)] (20)

where x =

for strength distribution and x =

for lifetime distribution, P

strength or lifetime cdf of one RVE given by Eqs. 14 and 15 or Eqs. 18 and 19.Here

we assume that the principal stresses in one RVE are fully statistically correlated to

the maximum one, which seems realistic. If they were uncorrelated, each principal

stress would require one element in the chain.

For large size structures, what matters for P

is only the tail of the strength or life-

time cdf of one RVE, i.e P

)

or P

)

¯m

. By taking the logarithm

of Eq. 20 and setting ln(1 −P

) ≈−P

for small P

, one can easily show that the

strength and lifetime distributions for large size structure converge to the Weibull

distribution:

(

)=1 −exp[−N

eq,

(

)

] (21)

(

)=1 −exp[−N

eq,

(

)

¯m

] (22)

where N

eq,

are the equivalent numbers of RVEs for the strength and lifetime

distributions, which can be calculated based on the elastic stress distribution in the

structure [13, 5]. The equivalent number of RVE physically means that a chain that

has N

eq,

or N

eq,

RVEs subjected to a uniform stress gives the same strength or

lifetime cdf as Eq. 20. The Weibull modulus of lifetime distribution is much smaller

than the Weibull modulus of strength distribution. They are related by:

¯m = m/(n + 1) (23)

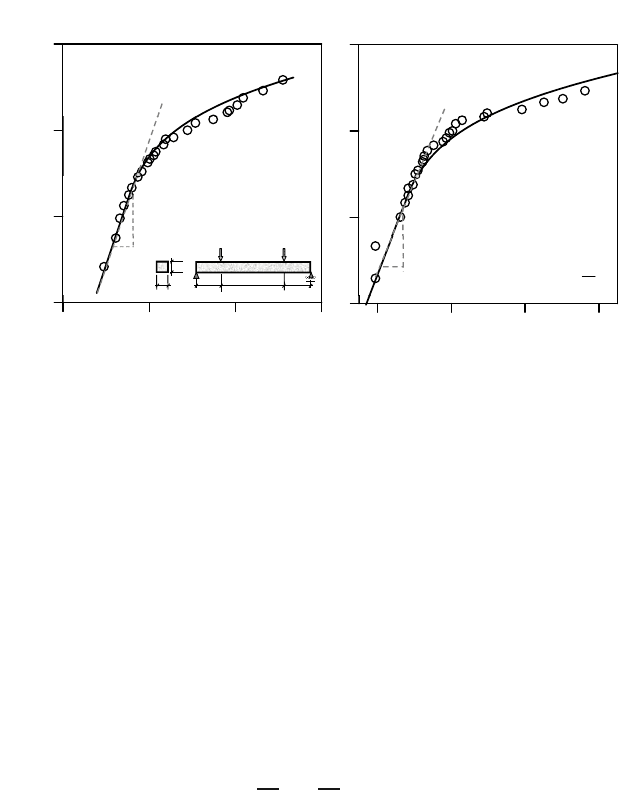
Fig. 5 presents the optimum ﬁts of the strength and lifetime histograms of 99.9

%Al

beam under four point bend test [25]. For each histogram, a total of 30

specimens were tested. Obviously, on the Weibull scale, both histograms do not

appear to be straight lines. Instead, there is a kink separating the histogram into two

parts where the lower part is a straight line and the upper part is curved. Such a

pattern cannot be explained by the two-parameter Weibull distribution.

Fig. 5 shows that the present theory gives excellent ﬁts of both the strength and

lifetime histograms. The location of kink actually corresponds to the grafting prob-

ability, which measures the degree of quasibrittleness of the structure.

From the data ﬁts, it is further observed that the grafting probabilities of the

strength and lifetime cdf’s are about the same. This agrees well with the present

theory, in which the grafting probability can be calculated as: P

= 1−[1−P

gr,1

]

Since the grafting probabilities P

gr,1

of strength and lifetime cdf’s for one RVE is

the same and the equivalent number of RVE for strength cdf is almost identical to

the equivalent number of RVE for lifetime cdf, then the grafting probabilities for

strength and lifetime cdf’s must be approximately the same.

By optimum ﬁtting, the Weibull moduli for strength and lifetime distributions are

estimated to be about 30 and 1.1, respectively. From Eq. 23 one can get exponent n

of the power law for creep crack growth for 99.9 % Al

, which is about 26.

Scaling of Strength and Lifetime Distributions of Quasibrittle Structures 55

99 6% Al

Strength Distribution Lifetime Distribution

)]}

(1

{

ln[1

-2

{

3.5

12 5

Constant load

78.0

5.2 5.4 5.6 5.8

-4

-8 -4 0 4

-4

Fig. 5. Optimum ﬁts of strength and lifetime histograms of 99.9 % Al

[25].

6 Size Effect on Mean Structural Strength and Lifetime

With the grafting probability distributions of strength and lifetime of one RVE, Eq.

20 directly implies the size effects on the strength and lifetime cdf’s. One can further

compute the size effects on the mean strength and lifetime. Based on the calibrated

strength and lifetime distributions of 99.9 % Al

(Fig. 5), one can calculate the

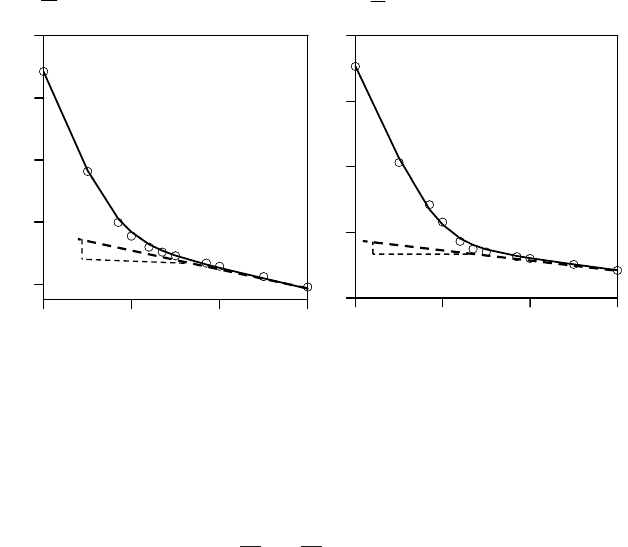
corresponding size effects on the mean structural strength and lifetime as shown in

Fig. 6.

Although a close-form expression for such size effects is impossible, one can ob-

tain the approximate form for the mean strength and lifetime by asymptotic match-

ing. It has been proposed that the size effect on mean strength can be approximated

by[2,3,11]:









(24)

where parameters N

, N

and m are to be determined by asymptotic properties of

the size effect curve.

It has been shown that such a size effect curve agrees well with the predictions

by other mechanical models such as the nonlocal Weibull theory [9, 10], as well

as with the experimental observations on concrete [16] and ﬁber composites [18];

m = Weibull modulus of the strength distribution, which can be determined by the

slope of the left tail of the strength histogram plotted on the Weibull scale, or more

accurately by size effect tests. The other three parameters, N

, N

,andr, can be

determined by solving three simultaneous equations based on three asymptotic con-

ditions, [

]

D→l

, [d

/dD]

D→l

,and[

1/m

]

D→∞

56 Z.P. Baˇzant and J.-L. Le

0.6

ean

rengt

ean L

0.4

0.2

99.6% Al

-0.2

1.1

0246

Dln

Fig. 6. Calculated size effects on mean structural strength and lifetime.

One can approximate the size effect on the mean structural lifetime by an equa-

tion with the same form as Eq. 24:









(n+1)/

(25)

where m is the Weibull modulus of the cdf of strength, and n = exponent of the

power law for subcritical creep crack growth rate. Similar to the size effect on mean

strength, C

, C

,and

can be obtained from three asymptotic conditions: [

]

D→l

/dD]

D→l

,and[

(n+1)/m

]

D→∞

It is obvious that the size effect on the mean structural lifetime is much stronger

than that on the mean strength. This is physically plausible. Consider two geometri-

cally similar beams, with size ratio 1:8. Let the nominal strength of the small beam

. Due to the size effect on the mean strength, the nominal strength of the large

beam is about

/2. If one applied a nominal load

/2 to both beams, the large beam

will fail within standard laboratory testing period (i.e. about 5 minutes) while the

small beam is expected to survive at that load for decades if not forever.

7Conclusion

The present theory shows that the types of strength and lifetime distributions depend

on the structure size and geometry. This has important implications for the safety

factors to be used in reliability assessment for the design of many engineering struc-

tures, such as large prestressed concrete bridges, large aircraft or ships made of ﬁber

composites, and various micro- and nano-electronic devices.

Scaling of Strength and Lifetime Distributions of Quasibrittle Structures 57

The present theory indicates that the safety factors guarding against the uncer-

tainties in strength and lifetime cannot be empirical, and cannot be constant. They

must be calculated as a function of the size, geometry of structures and environment.

Acknowledgement. The theoretical development was partially supported under

Grant CMS-0556323 to Northwestern University (NU) from the U.S. National Sci-

ence Foundation. The applications to concrete and composites were partially sup-

ported under a Grant to NU from the U.S. Department of Transportation through

the NU Infrastructure Technology Institute, and Grant N007613 to NU from

Boeing, Inc.

References

1. Basquin, O.H.: The Exponential Law of Endurance Tests. In: Proc. Amer. Soc.Testing

and Mater., ASTEA, vol. 10, pp. 625–630 (1910)

2. Baˇzant, Z.P.: Scaling theory of quaisbrittle structural failure. Proc. Nat’l. Acad. Sci.,

USA 101(37), 13397–13399 (2004)

3. Baˇzant, Z.P.: Scaling of Structural Strength, 2nd edn. Elsevier, London (2005)

4. Baˇzant, Z.P., Jir´asek, M.: R-curve modeling of rate and size effects in quasibrittle frac-

ture. Int. J. of Frac. 62, 355–373 (1993)

5. Baˇzant, Z.P., Le, J.-L.: Nano-mechanics based modeling of lifetime distribution of qua-

sibrittle structures. J. Engrg. Failure Ana. 16, 2521–2529 (2009)

6. Baˇzant, Z.P., Le, J.-L.: Size effect on strength and lifetime distributions of quasibrittle

structures. In: Proc. (CD), ASME 2009 Int. Mech. Engrg. Congress (IMECE 2009), Lake

Buena Vista, Florida, pp. 1–9 (2009)

7. Baˇzant, Z.P., Le, J.-L., Bazant, M.Z.: Size effect on strength and lifetime distribution

of quasibrittle structures implied by interatomic bond break activation. In: Proc. of 17th

European Conference on Fracture, Brno, Czech Rep., pp. 78–92 (2008)

8. Baˇzant, Z.P., Le, J.-L., Bazant, M.Z.: Scaling of strength and lifetime distributions of

quasibrittle structures based on atomistic fracture mechanics. Proceeding of National

Academy of Sciences 106(28), 1484–11489 (2009)

9. Baˇzant, Z.P., Nov´ak, D.: Probabilistic nonlocal theory for quasibrittle fracture initiation

and size effect. I. Theory. J. of Engrg. Mech. 126(2), 166–174 (2000)

10. Baˇzant, Z.P., Nov´ak, D.: Probabilistic nonlocal theory for quasibrittle fracture initiation

and size effect. II. Application. J. of Engrg. Mech. ASCE 126(2), 175–185 (2000)

11. Baˇzant, Z.P., Nov´ak, D.: Energetic-statistical size effect in quasibrittle failure at crack

initiation. ACI Mater. J. 97(3), 381–392 (2000)

12. Baˇzant, Z.P., Pang, S.-D.: Mechanics based statistics of failure risk of quasibrittle

structures and size effect on safety factors. Proceeding of National Academy of Sci-

ences 103(25), 9434–9439 (2006)

13. Baˇzant, Z.P., Pang, S.-D.: Activation energy based extreme value statistics and size effect

in brittle and quasibrittle fracture. J. Mech. Phys. Solids 55, 91–134 (2007)

14. Baˇzant, Z.P., Planas, J.: Fracture and Size Effect in Concrete and Other Quasibrittle Ma-

terials. CRC Press, Boca Raton (1998)

15. Baˇzant, Z.P., Prat, P.C.: Effect of temperature and humidity on fracture energy of con-

crete. ACI Mater. J. 85-M32, 262–271 (1988)

16. Baˇzant, Z.P., Voˇrechovsk´y, M., Nov´ak, D.: Asymptotic prediction of energetic-statistical

size effect from deterministic ﬁnite element solutions. J. Engrg. Mech., ASCE 128, 153–

162 (2007)

58 Z.P. Baˇzant and J.-L. Le

17. Baˇzant, Z.P., Xi, Y.: Statistical size effect in quasi-brittle structures: II. Nonlocal theory.

J. Engrg. Mech., ASCE 117(7), 2623–2640 (1991)

18. Baˇzant, Z.P., Zhou, Y., Daniel, I.M., Caner, F.C., Yu, Q.: Size effect on strength of

laminate-foam sandwich plates. J. of Engrg. Materials and Technology ASME 128(3),

366–374 (2006)

19. Chiao, C.C., Sherry, R.J., Hetherington, N.W.: Experimental veriﬁcation of an accel-

erated test for predicting the lifetime of oragnic ﬁber composites. J. Comp. Mater. 11,

79–91 (1977)

20. Daniels, H.E.: The statistical theory of the strength of bundles and threads. Proc. R. Soc.

London A. 183, 405–435 (1945)

21. Duckett, K.: Risk analysis and the acceptable probability of failure. The Structural Engi-

neering 83(15), 25–26 (2005)

22. Duffy, S.F., Powers, L.M., Starlinger, A.: Reliability analysis of structural ceramic com-

ponents using a three-parameter Weibull distribution. Tran. ASME J. Eng. Gas Turbines

Power 115, 109–116 (1993)

23. Evans, A.G.: A method for evaluating the time-dependent failure characteristics of brit-

tle materials and its application to polycrystalline alumina. J. Mater. Sci. 7, 1146–1173

(1972)

24. Evans, A.G., Fu, Y.: The mechanical behavior of alumina. In: Fracture in Ceramic Ma-

terials, pp. 56–88. Noyes Publications, Park Ridge (1984)

25. Fett, T., Munz, D.: Static and cyclic fatigue of ceramic materials. In: Vincenzini, P. (ed.)

Ceramics Today – Tomorrow’s Ceramics, pp. 1827–1835. Elsevier Science Publisher B.

V., Amsterdam (1991)

26. Graham-Brady, L.L., Arwadea, S.R., Corrb, D.J., Guti´errezc, M.A., Breyssed, D., Grig-

oriue, M., Zabaras, N.: Probability and Materials: from Nano- to Macro-Scale: A sum-

mary. Prob. Engrg. Mech. 21(3), 193–199 (2006)

27. Gross, B.: Least squares best ﬁt method for the three parameter Weibull distribution:

analysis of tensile and bend specimens with volume or surface ﬂaw failure. NASA TM-

4721, 1–21 (1996)

28. Harlow, D.G., Smith, R.L., Taylor, H.M.: Lower tail analysis of the distribution of the

strength of load-sharing systems. J. Appl. Prob. 20, 358–367 (1983)

29. Kawakubo, T.: Fatigue crack growth mechanics in ceramics. In: Kishimoto, H., Hoshide,

T., Okabe, N. (eds.) Cyclic Fatigue in Ceramics, pp. 123–137. Elsevier Science B. V. and

The Society of Materials Science, Japan (1995)

30. Kaxiras, E.: Atomic and Electronic Structure of Solids. Cambridge University Press,

Cambridge (2003)

31. Krausz, A.S., Krausz, K.: Fracture Kinetics of Crack Growth. Kluwer Academic Pub-

lisher, Netherlands (1988)

32. Le, J.-L., Baˇzant, Z.P.: Finite weakest link model with zero threshold for strength distri-

bution of dental restorative ceramics. Dent. Mater. 25(5), 641–648 (2009)

33. Le, J.-L., Baˇzant, Z.P., Bazant, M.Z.: Crack growth law and its consequences on lifetime

distributions of quasibrittle structures. Journal of Physics D: Applied Physics 42, 214008,

8 (2009)

34. Lohbauer, U., Petchelt, A., Greil, P.: Lifetime prediction of CAD/CAM dental ceramics.

Journal of Biomedical Materials Research 63(6), 780–785 (2002)

35. Mahesh, S., Phoenix, S.L.: Lifetime distributions for unidirectional ﬁbrous composites

under creep-rupture loading. Int. J. Fract. 127, 303–360 (2004)

36. Melchers, R.E.: Structural Reliability, Analysis & Prediction. Wiley, New York (1987)

37. Munz, D., Fett, T.: Ceramics: Mechanical Properties, Failure Behavior, Materials Selec-

tion. Springer, Berlin (1999)