Kounadis A.N., Gdoutos E.E. (Eds.) Recent Advances in Mechanics
Подождите немного. Документ загружается.

Directional Distortional Hardening in Plasticity within Thermodynamics 69
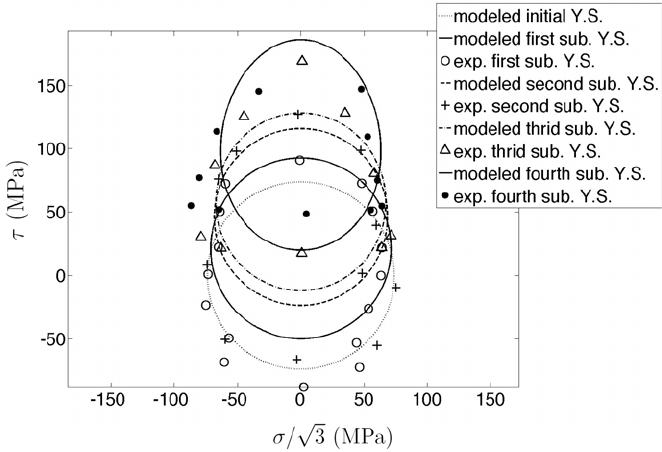
Fig. 3. α
α
α-model with evolving c compared to torsion experiments by Wu and Yeh (1991).
The first, second, third, and fourth subsequent yield surfaces resulted from strain controlled
loading with final values γ = 0.19%,0.38%,0.49% and 1.03%, respectively. Lines represent
the proposed constitutive model and discrete points represent experimental data.
models can simulate complex loading paths. The fitting of the data in Fig. 9 may be
considered satisfactory given the simplicity of the model used, however, as Fig. 8
shows the A
A
A-model simulates the data better.
3.1 Ratchetting
Since the A
A
A-model is the most complete and fits experimentally found yield surfaces
best, it will be used to simulate experimental cyclic plastic loading data. During
cyclic plastic loading, plastic strain accumulates and this is called ratchetting. When
the load is applied in two directions biaxial ratchetting occurs. Both stress and stress
controlled cyclic biaxial tests from Hassan et al. [9] will be simulated, and uniaxial
cyclic tests from Hassan and Kyriakides [8] will be used for calibration. In biaxial
stress controlled tests the material ratchets in two directions, and thus these test have
been especially difficult to simulate.
Experiments in the study by Hassan and Kyriakides [8] and Hassan et al. [9] were
performed on thin walled tubes of carbon steel 1026. For the uniaxial experiments,
axial stress was applied cyclically. Various levels of mean stress σ
xm
were used and
the stress amplitude was σ
xa
= 0.79σ
0
,whereσ
0
= 40 ksi. For the strain controlled
biaxial data, the tubes were first loaded with internal pressure σ
θ
and then cycled
axially using various levels of strain amplitude ε
xc
. While the cyclic loading was
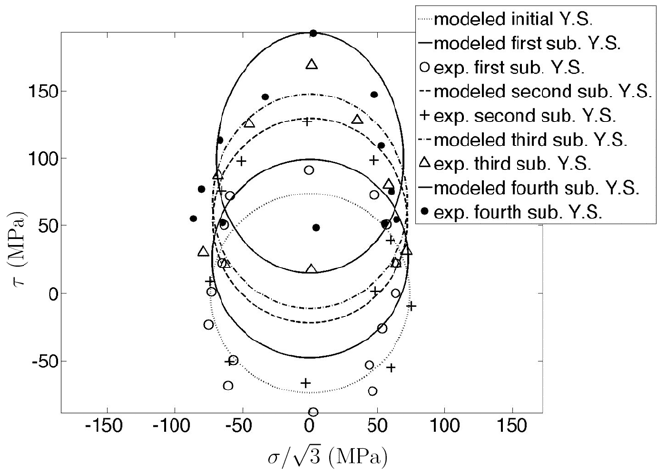
70 Y.F. Dafalias and H.P. Feigenbaum
Fig. 4. r-model compared to torsion experiments by Wu and Yeh (1991). The first, second,
third, and fourth subsequent yield surfaces resulted from strain controlled loading with fi-
nal values γ = 0.19%,0.38%,0.49% and 1.03%, respectively. Lines represent the proposed
constitutive model and discrete points represent experimental data.
occurring the internal pressure was kept constant. This results in strain accumula-
tion, i.e., ratchetting, in the circumferential direction ε
θ
. For the stress controlled
biaxial data, tubes were first stabilized by strain symmetric axial cycles in the range
of ±1% and in approximately twelve cycles the hysteresis loops were stable. Fol-
lowing the strain symmetric cycling the specimens were unloaded to approximately
zero axial stress and strain, although typically some small residual circumferential
strain remained non-zero, then preloaded using stress control with various internal
pressures. Following the internal pressure, the tubes were stress controlled cyclically
loaded in the axial direction about a nonzero mean stress σ
xm
with amplitude σ
xa
.
The stress controlled biaxial loading in conjunction with the cycling about nonzero
mean axial stress results in plastic strains accumulating in both the circumferential
and the axial directions.
With kinematic hardening alone, the Armstrong-Frederick kinematic hardening
rule generally does not fit experimental results from cyclic plastic loading paths
very well, therefore researchers have developed numerous other kinematic harden-
ing rules which show improvement in fitting cyclic loading. This paper will use the
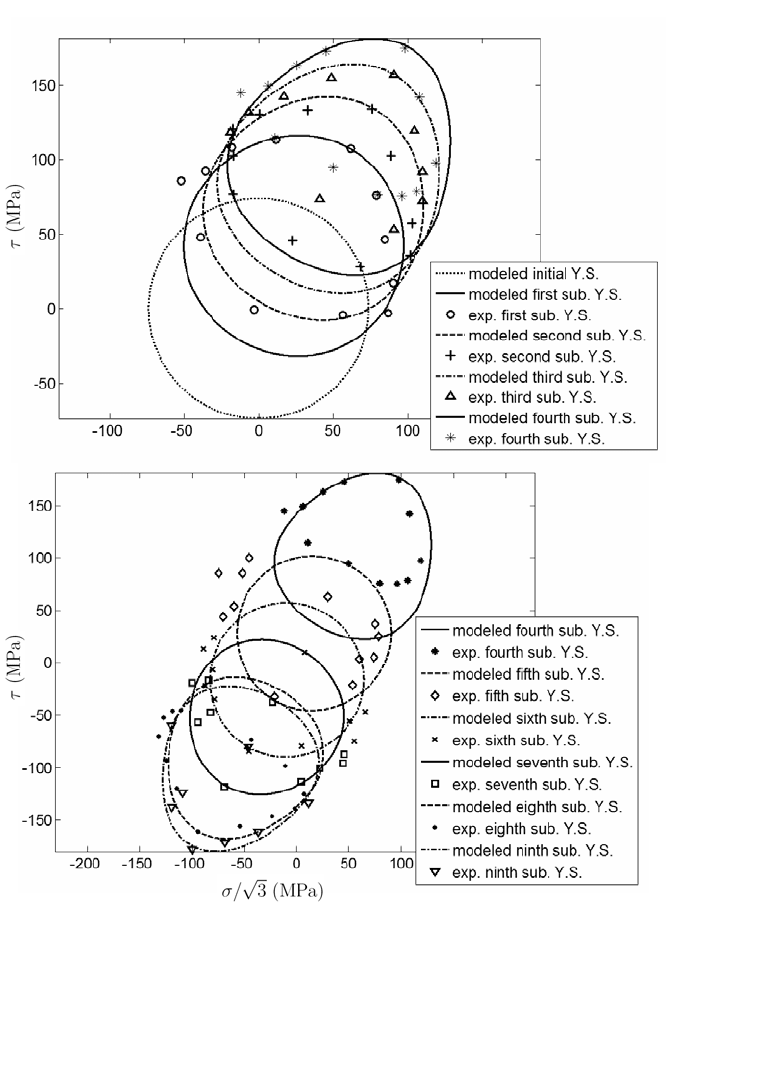
Directional Distortional Hardening in Plasticity within Thermodynamics 71
Fig. 5. A
A
A-model compared to stress controlled combined tension-torsion experiments by Wu
and Yeh [18]. Top figure shows the loading and bottom figure shows unloading and nega-
tive loading. For both figures the loading path was proportional with normal stress equal to
shear stress, i.e., σ = τ. Lines represent the proposed constitutive model and discrete points
represent experimental data.
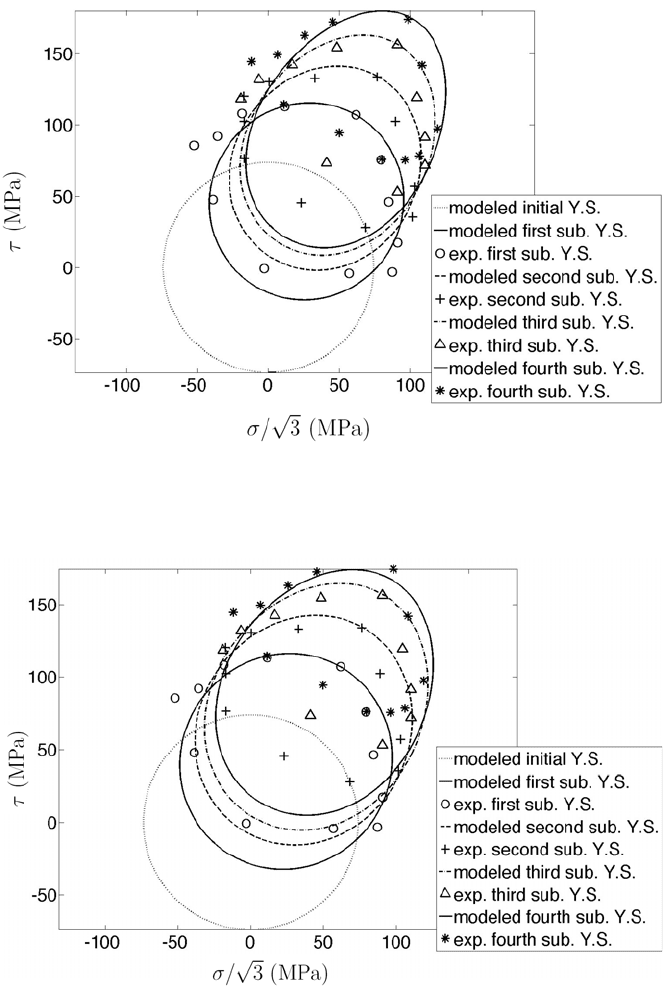
72 Y.F. Dafalias and H.P. Feigenbaum
Fig. 6. α
α
α-model with evolving c compared to stress controlled combined tension-torsion ex-
periments by Wu and Yeh (1991). The loading path was proportional with normal stress equal
to shear stress, i.e., σ = τ. Lines represent the proposed constitutive model and discrete points
represent experimental data.
Fig. 7. r-model compared to stress controlled combined tension-torsion experiments by Wu
and Yeh (1991). The loading path was proportional with normal stress equal to shear stress,
i.e., σ = τ. Lines represent the proposed constitutive model and discrete points represent
experimental data.
Directional Distortional Hardening in Plasticity within Thermodynamics 73
−300 −200 −100 0 100 200 300 400
−200
−150
−100
−50
0
50
100
150
200
250
σ
11
(MPa)
√
2σ
12
(MPa)
Comp. initial YS
Exp. initial YS
Comp. 1st sub. YS
Exp. 1st sub. YS
Comp. 2nd sub. YS
Exp. 2nd sub. YS
Load path O−A−B−C
C
B
A
O
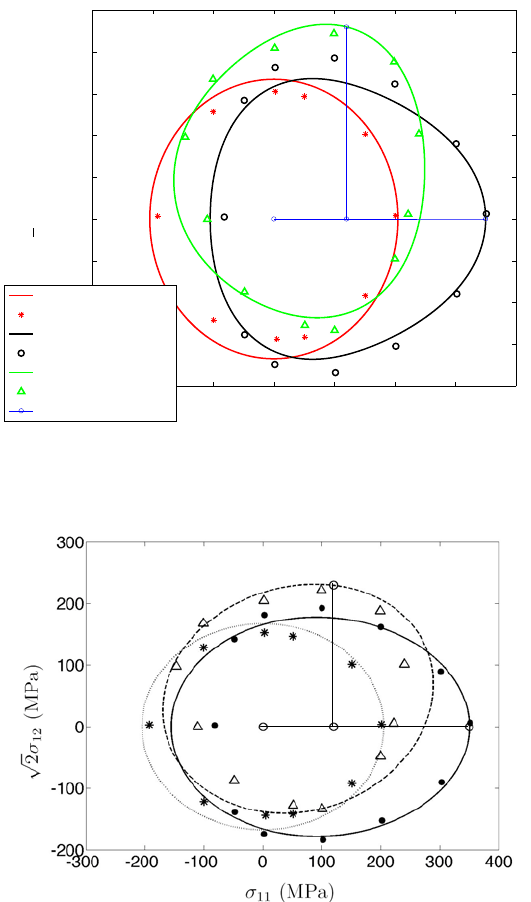
Fig. 8. A
A
A-model compared to stress controlled combined tension and shear experiments by
Boucher et al. [3]. Specimens were loaded in pure tension, unloaded some, then loaded in
pure torsion. The load path is shown on the figure as O-A-B-C.
OAB
C
Fig. 9. α
α
α-model with fixed c compared to stress controlled combined tension and shear ex-
periments by Boucher et al. [3]. Specimens were loaded in pure tension, unloaded some, then
loaded in pure torsion. The load path is shown on the figure as O-A-B-C. Computed initial
yield surface: ···, experiential initial yield points: ∗, computed first subsequent yield surface:
—–, experiential first subsequent yield points: •, computed second subsequent yield surface:
---, experiential second subsequent yield points: .
74 Y.F. Dafalias and H.P. Feigenbaum
Table 2. Material constants used to fit ratchetting data from Hassan et al. [9].
without distortion with distortion
k
0
18.8 18.8
c
1
20000 19000
a
s
1
32.85
c
2
400 380
a
s
2
8.07 7.67
c
3
10.45 11
a
s
3
39.3 41.36
c
4
5000 5000
a
s
4
33
¯
a 55
A
1
-2
A
2
- 1125
kinematic hardening rule proposed by Chaboche [4] for cyclic loading. This kine-
matic hardening rule can be expressed as:
α
α
α =
4
i=1
α
α
α
i
˙
α
α
α
i
= λ
∂f
∂σ
σ
σ
2
3
(
2
3
a
s
i
n− α
α
α
i
)(i = 1, 2, 3)
˙
α
α
α
4
= λ
∂f
∂σ
σ
σ
2
3
2
3
a
s
i
n − α
α
α
i
1 −
¯
a
f(α
α
α
4
)
(19)
where f(α
α
α)=
3
2
α
α
α : α
α
α.
To calibrate the model for ratchetting data, the model with kinematic harden-
ing alone was calibrated by Bari and Hassan [2] and then both material parameters
associated rate and saturation values for kinematic hardening are decreased and di-
rection distortional hardening parameters are added using Eqns. (17) and (18) as a
guide. This procedure exactly mimics what was done to fit experimentally found
yield surfaces, except in this case the strain symmetrically stabilized data as well as
the uniaxial cyclic data were used for calibration. For simplicity no isotropic harden-
ing was included and no adjustments were made to the parameters associated with
α
α
α
4
, where the threshold is contained, and approximately equal adjustments were
made to the parameters associated with α
α
α
i
where i =1, 2, or 3. The results of the
calibration can be seen in Fig. 10 and the parameters are given in Table 2 where all
units are consistent with ksi.
Figs. 11 and 12 show the model simulating ratchetting data from Hassan et al. [9].
For the strain controlled ratchetting in Fig. 11 the model with directional distortional
hardening performs about as well as the model with kinematic hardening alone. For
the stress controlled ratchatting in Fig. 12, the model with directional disortional
Directional Distortional Hardening in Plasticity within Thermodynamics 75
0 10 20 30 40 50
0
0.5
1
1.5
2
2.5
3
3.5
4
4.5
ε
θ p
(%)
N
σ
xa
= 0.79 σ
0
σ
xm
= 0.225 σ
0
σ
xm
= 0.160 σ
0
σ
xm
= 0.104 σ
0
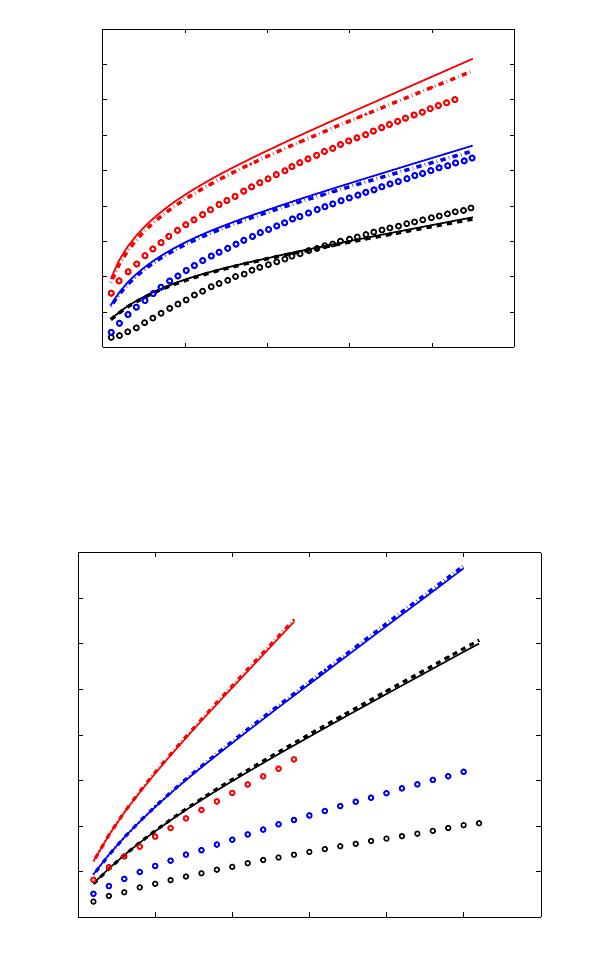
Fig. 10. A
A
A-model as calibrated to the uniaxial ratchetting experimental data. The simulations
with kinematic hardening alone are shown as solid lines, the simulation with kinematic hard-
ening and directional distortional hardening are shown as dashed lines, and the experimental
data are shown as discrete points. In all cases the stress amplitude is σ
xa
= 0.79σ
0
and three
different mean stress σ
xm
values are shown.
0 5 10 15 20 25 30
0
0.5
1
1.5
2
2.5
3
3.5
4
ε
θ p
(%)
N
σ
θ
= 0.24 σ
0
ε
xc
= 0.4%
ε
xc
= 0.4%
ε
xc
= 0.65%
ε
xc
= 0.5%
ε
xc
= 0.65%
ε
xc
= 0.5%
Fig. 11. Strain controlled biaxial ratchetting simulations and experimental data. The simula-
tions with kinematic hardening alone are shown as solid lines, the simulation with kinematic
hardening and directional distortional hardening are shown as dashed lines, and the experi-
mental data are shown as discrete points. In all cases the internal pressure is kept constant
(σ
θ
= 0.24σ
0
) while the strain is cycled symmetrically at different amplitude, ε
xc
.
76 Y.F. Dafalias and H.P. Feigenbaum
−1.5 −1 −0.5 0 0.5 1 1.5
−0.5
0
0.5
1
1.5
2
2.5
3
3.5
ε
θ m
(%)
ε
x m
(%)
σ
θ
= 0.353 σ
0
σ
θ
= 0.249 σ
0
σ
θ
= 0.120σ
0
σ
xa
= 0.8 σ
0
σ
xm
= 0.13 σ
0
N
max
= 45
(a)
−1 −0.5 0 0.5
−0.5
0
0.5
1
1.5
2
2.5
3
ε
θ m
(%)
ε
x m
(%)
σ
θ
= 0.318 σ
0
σ
θ
= 0.210 σ
0
(b)
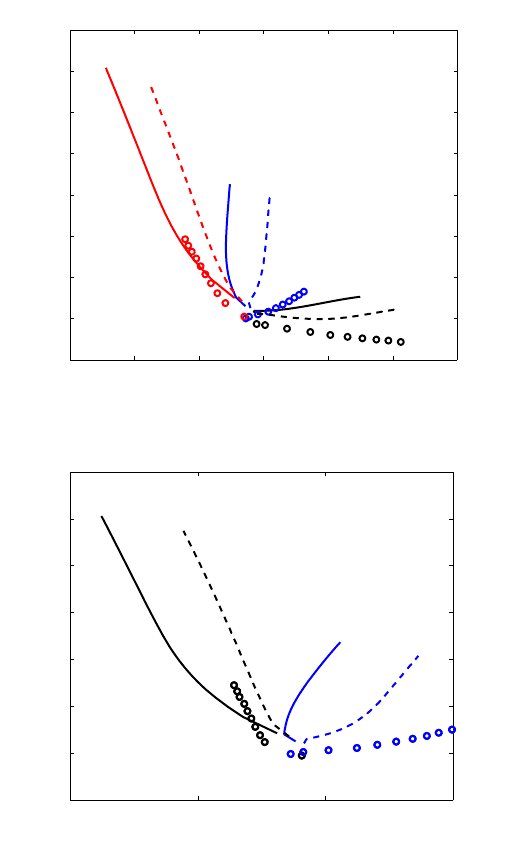
Fig. 12. Stress controlled biaxial ratchetting simulations and experimental data. The simu-
lations with kinematic hardening alone are shown as solid lines, the simulation with kine-
matic hardening and directional distortional hardening are shown as dashed lines, and the
experimental data are shown as discrete points. The mean axial strain ε
xm
and the mean
circumferential strain ε
θm
per cycle are both predicted values. In both 12(a) and 12(b) the
axial stress amplitude is σ
xa
= 0.8σ
0
, the mean axial stress is σ
xm
= 0.13σ
0
and 45 cycles
were performed. Each data set has a different the value for the internal pressure (which leads
to σ
θ
).
Directional Distortional Hardening in Plasticity within Thermodynamics 77
hardening performs consistently better. For some data sets the addition of directional
distortion hardening results in more dramatic improvement (e.g., Fig. 12(b) with
σ
θ
= 0.210σ
0
), while in others the improvement is minor (e.g., Fig. 12(a) with
σ
θ
= 0.353σ
0
). Nonetheless, from Fig. 12 it is clear that directional distortional
hardening improves the simulation of stress controlled biaxial ratchetting.
4Conclusion
It is very importance to accurately model the shape of the yield surface when using
the associative flow rule not only for capturing accurately the yield point, but mainly
because any deviation in shape may result in significant deviation of the direction
of the normal to the yield surface and, thus, of the coaxial plastic strain increment,
which during cyclic loading and resulting ratcheting accumulates the error to unac-
ceptable levels. Three different models were developed within thermodynamics by
Feigenbaum and Dafalias [5, 6] capturing this phenomenon of directional distortion
of the yield surface, and for the first time are presented in unison in this paper. The
Feigenbaum and Dafalias model [5] (i.e., the A
A
A-model) uses a fourth order tensor
and a scalar multiplier to capture directional distortion, and is the most successful
in data fitting, albeit the most complex as well, of the three models. In particular
this model is used to simulate experimental data from biaxial cyclic loading test
showing the improvement of simulations for ratchetting obtained by the introduced
directional distortional hardening scheme as compared with simulations which do
not involve directional distortion of the yield surface.
References
[1] Bardet, J.P., Choucair, W.: A linearized integration technique for incremental constitu-
tive equations. International Journal for Numerical and Analytical Methods in Geome-
chanics 15, 1–19 (1991)
[2] Bari, S., Hassan, T.: Anatomy of coupled consititutive models for ratcheting simulation.
International Journal of Plasticity 16, 381–409 (2000)
[3] Boucher, M., Cayla, P., Cordebois, J.P.: Experimental studies of yield surfaces of alu-
minum alloy and low carbon steel under complex biaxial loadings. European Journal of
Mechanics, A/Solids 14(1), 1–17 (1995)
[4] Chaboche, J.L.: On some modifications of kinematic hardening to improve the descrip-
tion of ratcheting effects. International Journal of Plasticity 7, 661–678 (1991)
[5] Feigenbaum, H.P., Dafalias, Y.F.: Directional distortional hardening in metal plasticity
within thermodynamics. International Journal of Solids and Structures 44, 7526–7542
(2007)
[6] Feigenbaum, H.P., Dafalias, Y.F.: Simple model for directional distortional hardening
in metal plasticity within thermodynamics. ASCE Journal of Engineering Mechan-
ics 134(9), 730–738 (2008)
[7] Franc¸ois, M.: A plasticity model with yield surface distortion for non proportional load-
ing. International Journal of Plasticity 17, 703–717 (2001)
[8] Hassan, T., Kyriakides, S.: Ratcheting in cyclic plasicity, part i: Uniaxial behavior. In-
ternational Journal of Plasticity 8, 91–116 (1992)
78 Y.F. Dafalias and H.P. Feigenbaum
[9] Hassan, T., Corona, E., Kyriakides, S.: Ratcheting in cyclic plasicity, part ii: Multiaxial
behavior. International Journal of Plasticity 8, 117–146 (1992)
[10] Kurtyka, T., Zyczkowski, M.: Evolution equations for distortional plastic hardening.
International Journal of Plasticity 12(2), 191–203 (1996)
[11] McComb, H.G.: Some experiments concerning subsequent yield surfaces in plasticity.
Tech. Rep. D-396, National Aeronautics and Space Adminstration (1960)
[12] Naghdi, P.M., Essenburg, F., Koff, W.: An experimental study of initial and subsequent
yield surfaces in plasticity. Journal of Applied Mechanics 25(2), 201–209 (1958)
[13] Ortiz, M., Popov, E.P.: Distortional hardening rules for metal plasticity. Journal of En-
gineering Mechanics 109(4), 1042–1057 (1983)
[14] Phillips, A., Tang, J.L., Ricciuti, M.: Some new observations on yield surfaces. Acta
Mechanica 20, 23–39 (1975)
[15] Plesek, J., Feigenbaum, H.P., Dafalias, Y.F.: Convexity of yield surfaces with direc-
tional distortional hardening. ASCE Journal of Engineering Mechanics 136(4), 477–
484 (2010)
[16] Voyiadjis, G.Z., Foroozesh, M.: Anisotropic distortional yield model. Journal of Ap-
plied Mechanics 57, 537–547 (1990)
[17] Wei-Ching Yeh, C.D.H., Pan, W.F.: An endochronic theory accounting for deforma-
tion induced anisotropy of metals under biaxial load. International Journal of Plastic-
ity 12(8), 987–1004 (1996)
[18] Wu, H.C., Yeh, W.C.: On the experimental determination of yield surfaces and some
results of annealed 304 stainless steel. International Journal of Plasticity 7, 803–826
(1991)
