Kamal A.A. 1000 Solved Problems in Classical Physics: An Exercise Book
Подождите немного. Документ загружается.

11.2 Problems 479
Fig. 11.13
11.89 Two square metal plates measuring ‘a’ on the side are used as a parallel
plate capacitor with the plates slightly inclined at an angle θ . If the smaller
gap between the plates is D, then show that the capacitance is given by
C =
ε
0
a
2
D
1 −
aθ
2D
11.90 Capacitors C
1
= 8 μF, C
2
= 4 μF and C
3
= 3 μF are arranged as in
Fig. 11.14. A voltage of V = 100 V is applied. Determine
(a) the potential difference across C
1
, C
2
and C
3
.
(b) the charge q
1
, q
2
and q
3
on C
1
, C
2
and C
3
.
(c) the energy U
1
, U
2
and U
3
stored in the capacitors.
Fig. 11.14
11.91 Capacitors C
1
= 8 μF, C
2
= 4 μF and C
3
= 3 μF are arranged as in
Fig. 11.15. A voltage of V = 100 V is applied. Determine
(a) the charges q
1
, q
2
and q
3
on C
1
, C
2
and C
3
, respectively.
(b) the potential difference across C
1
, C
2
and C
3
.
(c) the energy U
1
, U
2
and U
3
stored in the capacitors.
Fig. 11.15
11.92 Find the effective capacitance between points a and b in Fig. 11.16. Assume
that C
1
= C
2
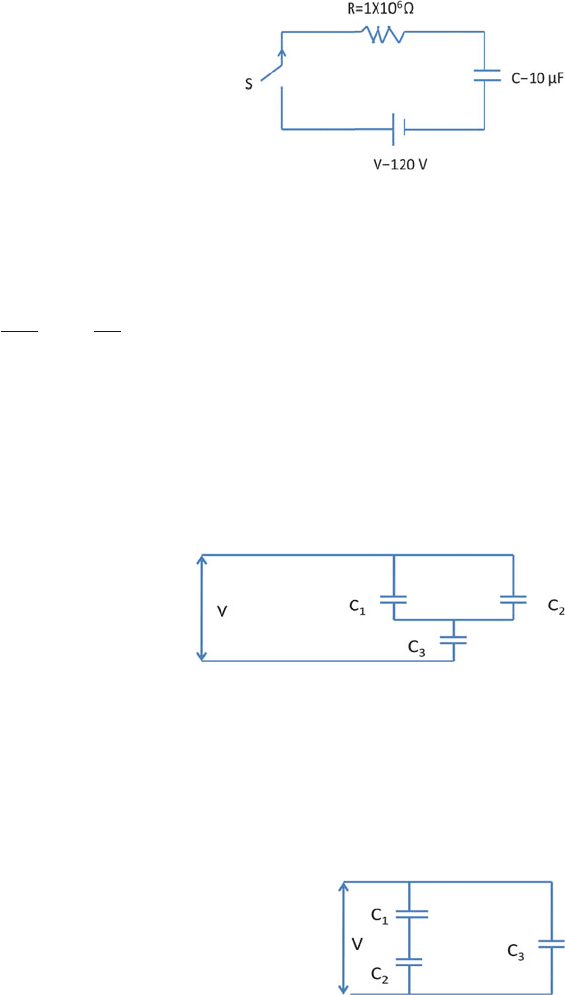
= C
3
= C
4
= 2 μF and C
5
= 1 μF.
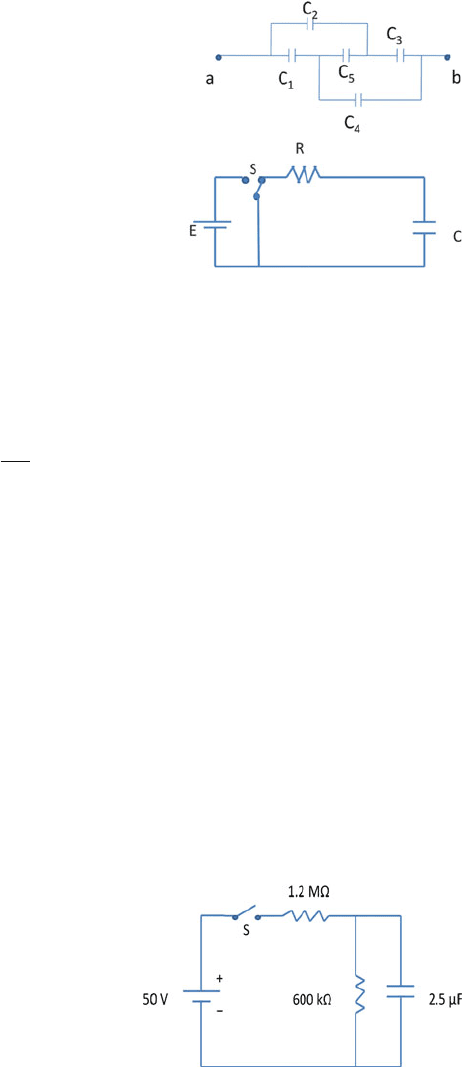
480 11 Electrostatics
Fig. 11.16
Fig. 11.17
11.93 Consider a circuit consisting of a resistor R and a capacitor C in series
with a battery of emf ξ and a switch (Fig. 11.17). The capacitor is initially
uncharged and the switch is closed at time t = 0. By considering the potential
drop across each of the components of the circuit, verify that the charge Q
on the capacitor has the form
Q = Cξ
⎛
⎝
1 − e
−
r
RC
⎞
⎠
(a) What is the current flowing in the circuit?
(b) What is the power supplied by the battery as a function of t?
(c) What is the power dissipated in the resistor as a function of t?
(d) What is the rate at which energy is stored in the capacitor as a function
of t?
[University of Durham 2000]
11.94 For the circuit shown in Fig. 11.18,
(i) What is the initial battery current immediately after switch S is closed?
(ii) What is the battery current a long time after switch S is closed?
(iii) If the switch has been closed for a long time and is then opened, find
the current through the 600 k resistor as a function of time.
Fig. 11.18

11.2 Problems 481
11.95 A capacitor of capacitance C = 500 μF is charged to a voltage of 900 V and
is then discharged through a resistance R = 200 k when a switch is closed.
(i) Find the initial charge stored in the capacitor.
(ii) Find the initial discharge current when the switch is closed.
(iii) Find the voltage across the capacitor in a time t = 25 s after the start of
discharge.
(iv) Find the time constant of this capacitor resistor network combination.
(v) Work out an equation to show the time it takes for the charge in the
capacitor to drop by one-half of its starting value and find this time.
[University of Aberystwyth, Wales 2008]
11.96 Charge q = 10
−9
C is uniformly distributed in a sphere of radius R = 1m.
(i) Find the divergence of the electric field inside the sphere.
(ii) A proton is moved from infinity to r = 0.8 m from the centre of the
sphere. Find the electric force experienced by the proton at r = 0.8m.
(iii) Find the work done by the electric field of the charged sphere when the
proton is moved from infinity to its current position (r = 0.8m).
11.97
(a) Write down the integral and differential forms of Gauss’ law in a dielec-
tric, defining all quantities used.
(b) A parallel plate capacitor is completely filled with a non-conducting
dielectric. Show that the electric displacement, D, is uniform between
the plates and calculate its value. (You may assume that the plates each
have area A and are separated by a small distance d. Each plate carries
a surface charge density σ C/m
2
.)
(c) The dielectric has a non-uniform relative permittivity
K (x) = ax + b
where a and b are constants and x is the perpendicular distance from one
plate. Using Gauss’ law, show that the electric field between the plates
satisfies
E(x) =
E
0
K (x)
where E
0
is a constant. Find the value of E
0
.
(d) Show that the voltage across the capacitor is given by
V =
E
0
ε
0
a
ln
1 +
Qd
b
and calculate the capacitance.
(e) Find the volume polarization charge density in the dielectric.

482 11 Electrostatics
11.98 Both gravitational field and electric field obey inverse square law. Using this
analogy show that the differential Gauss’ law for gravitation is given by ∇·
g =−ρ/G, where ρ is the mass density.
11.3 Solutions
11.3.1 Electric Field and Potential
11.1
(a)
(i)
F =
q
1
q
2
4πε
0
r
2
=
(8 × 1.6 × 10
−19
)(−4 × 1.6 × 10
−19
)
4π ×8.85 × 10
−12
× (0.2)
2
=−1.8432 × 10
−25
N
(ii) For the position of zero electric field the forces due to the two charges
must be equal in magnitude but oppositely directed. Clearly the neu-
tral point must be on the x-axis. On the left of Q
1
, the forces will
be oppositely directed but cannot be equal as |Q
1
| > |Q
2
|. Between
Q
1
and Q
2
, the forces are exerted in the same direction. On the right
of Q
2
conditions are favourable for a null point. Let the zero electric
field be situated at a distance x from Q
2
on the right.
8e
(x + 0.2)
2
−
4e
x
2
= 0
whence x = 0.4828 on the r ight of Q
2
.
(b) E =
e
4 πε
0
r
2
=
1.6 × 10
−19
4π ×8.85 × 10
−12
× (5.3 × 10
−11
)
2
= 5.12 ×10
18
N/C
Force F = Ee = 5.12 × 10
18
× 1.6 × 10
−19
= 81.92 N
11.2
(a) As the electric field is downwards, the force on the positive charge will
be downwards and the force on the negative charge will be upwards.
(i)
q =+8 μC
F
q
= qE =+8 ×10
−6
× 300 = 2.4 × 10
−3
N
F
g
= mg = 0.6 × 10
−3
× 9.8 = 5.88 × 10
−3
N
∴ Tension in the thread
T = F
g
+ F
q
= 5.88 ×10
−3
+ 2.4 × 10
−3
= 8.28 ×10
−3
N
11.3 Solutions 483
(ii)
q =−8 μC
F
q
= qE =−2.4 ×10
−3
N
Tension in the thread
T = 5.88 ×10
−3
− 2.4 × 10
−3
= 3.48 ×10
−3
N
(b) As the electric field is in t he negative x-direction, point b will be at a
higher potential than a.
(i) Therefore V
b
− V
a
will be positive
(ii)
E =
V
b
− V
a
d
=
10
5
(6 − 2)
= 2.5 ×10
4
N/C
11.3 The total charge on the circular loop Q = 2π Rλ; the distance of the point
P(0, 0, Z) from the loop is r = (Z
2
+R
2
)
1/2
. Therefore, the electric potential
P will be
V =
Q
4πε
0
r
=
λR
2ε
0
(Z
2
+ R
2
)
1/2
E =−
∂V
∂z
=
λRZ
2ε
0
(Z
2
+ R
2
)
3/2
11.4
(a)
F =
q
4πε
0
r
2
(Q = 1)
V =−
F dr =−
q
4πε
0
dr
r
2
=
q
4πε
0
r
+ C
When r =∞, V = 0. Therefore C = 0
(b) U =
q
2
4πε
0
1
d
−
1
d
+
1
d
−
1
d
−
√
2
d
−
√
2
d
!
For six pairs of charges
U =−
q
2
√
2πε
0
d
The potential energy does not depend on the order in which the charges
are assembled.
(c) Consider the forces on the top left-hand charge due to the three other
charges.
E
x
= E
y
=−
q
2
8 πε
0
d
2
∴ E =
E
2
x
+ E
2
y
=
√
2
q
2
8 πε
0
d
2
= 0

484 11 Electrostatics
Therefore the charge is not in equilibrium. Same thing is true for the other
three charges.
11.5
(i) V
1
=
q
4πε
0
r
=
2 × 10
−15
× 9 × 10
9
10
−3
= 0.018 V
(ii) If n droplets each of radius r coalesce to form a large drop of radius R,
then assuming that the droplets are incompressible, the volume does not
change. Therefore
4
3
π R
3
= n
4
3
πr
3
or R = n
1/3
r (1)
As charge is conserved
Q = nq (2)
where Q and q are the charges on the drop and droplet, respectively. Then
the potential of the droplet is
V
2
=
Q
4πε
0
R
=
nq
4πε
0
n
1/3
r
=
n
2/3
q
4πε
0
r
= n
2/3
V
1
= 2
2/3
V
1
= 0.0286 V
where n = 2, and V
1
= 0.018 V by (i).
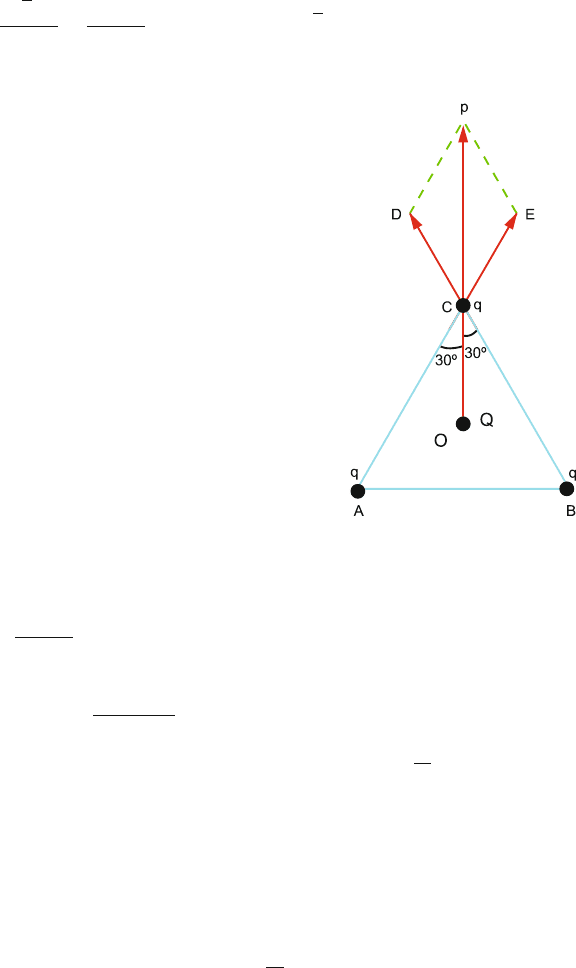
11.6 Electric force
F = qE = 2 × 10
−8
× 20, 000 = 4 × 10
−4
Gravitational force mg = 80 × 10
−6
× 9.8 = 7.84 × 10
−4
N
Balancing the horizontal and vertical components of forces, Fig. 11.19
T sin θ = F (1)
T cos θ = mg (2)
where T is the tension in the thread.
Fig. 11.19
11.3 Solutions 485
tan θ =
F
mg
=
4 × 10
−4
7.84 × 10
−4
= 0.51
θ = 27
◦
Squaring (1) and (2) and adding and extracting the square root
T =
F
2
+ (mg)
2
=
(4 × 10
−4
)
2
+ (7.84 × 10
−4
)
2
= 8.8 ×10
−4
N
11.7 Electric potential
V =
1
4πε
0
q
1
+
q
2
+
q
4
+
q
8
+···
=
q
4πε
0
1 +
1
2
+
1
2
2
+
1
2
3
+···
The terms in brackets form a geometric progression of infinite terms whose
sum is
S =
a
1 −r
=
1
1 −
1
2
= 2
∴ V =
q
2πε
0
Electric field
E =
1
4πε
0
q
1
+
q
2
2
+
q
4
2
+
q
8
2
+···
=
1
4πε
0
q
1 −
1
4
=
q
3πε
0
11.8
V =
1
4πε
0
q −
q
2
+
q
4
−
q
8
+
q
16
−
q
32
+···
=
q
4πε
0
1 +
1
4
+
1
16
+···
−
q
8πε
0
1 +
1
4
+
1
16
+···
=
1
4πε
0
q −
q
2
1 +
1
4
+
1
16
+···
=
q
8πε
0
1
1 −
1
4
=
q
6πε
0
486 11 Electrostatics
E =
1
4πε
0
q −
q
2
2
+
q
4
2
−
q
8
2
+
q
16
2
···
=
q
4πε
0
1 +
1
4
2
+
1
16
2
+···
−
q
16πε
0
1 +
1
4
2
+
1
16
2
+···
=
1
4πε
0
q −
q
4
1 +
1
4
2
+
1
16
2
+···
=
3q
16πε
0
1
1 −
1
16
=
q
5πε
0
11.9 By prob. (11.3), E =
Qx
4πε
0
x
2
+ R
2
3/2
, where we have replaced z by x and
substituted λ = Q/2π R.Asx << R and F = qE,
F =
Qq x
4πε
0
R
3
=−kx, where q is negative and k =
Qq
4πε
0
R
3
=
10
−5
× 10
−6
× 9 × 10
9
1
3
= 0.09
Thus, the motion of the negatively charged particle is approximately simple
harmonic with angular frequency
ω =
k
m
=
0.09
0.9 × 10
−3
= 10
T =
2π
ω
=
2π
10
= 0.628 s
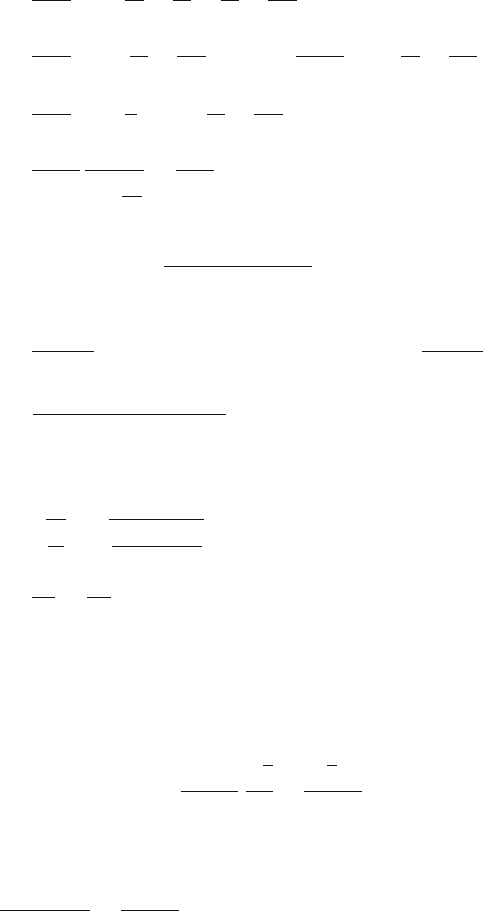
11.10 Let a be the side of the equilateral triangle. The forces on the charge q placed
at C due to the charges at A and B are repulsive and represented by CE and
CD, respectively, each given by q
2
/4πε
0
a
2
. The resultant of these two forces
is given by CP, the diagonal of the parallelogram CDPE, Fig. 11.20
CP = 2CD cos 30
◦
=
2q
2
4πε
0
a
2
√
3
2
=
√
3q
2
4πε
0
a
2
The force on q at C due to Q at the centre of the triangle is
Qq
4πε
0
(OC)
2
=
3Qq
4πε
0
a
2
i. If Q =−q, this force will be attractive and will be directed along CO. As
the attractive force due to −q is greater than the combined repulsive force
due to charges +q at A and B, the charge at C will be attracted towards
O. Same is true for the charges placed at A and B.
11.3 Solutions 487
ii. For equilibrium, the attractive force must balance the repulsive force:
√
3q
2
4πε
0
a
2
−
3Qq
4πε
0
a
2
= 0 → Q =−q/
√
3
Fig. 11.20
11.11 Referring to Fig. 11.21, the Coulomb force between the spheres in air is
F =
q
2
4πε
0
x
2
In liquid F
=
q
2
4πε
0
Kx
2
; weight of the sphere in air = mg
Apparent weight of the sphere in liquid = mg
1 −
ρ
ρ
, where ρ is the
density of the material of sphere and ρ
that of the liquid. For equilibrium
the vertical and horizontal components of the force must separately balance.
If T is the tension in the string when the sphere is in air and T
when it is in
the liquid,
T sin θ = F, T cos θ = mg
T
sin θ = F
, T
cos θ = mg
1 −
ρ
ρ
488 11 Electrostatics
tan θ =
F
mg
=
F
mg
1 −
ρ
ρ
F
F
= K =
1
1 −
ρ
ρ
=
ρ
ρ −ρ
=
1.6
1.6 − 0.8
= 2
Fig. 11.21
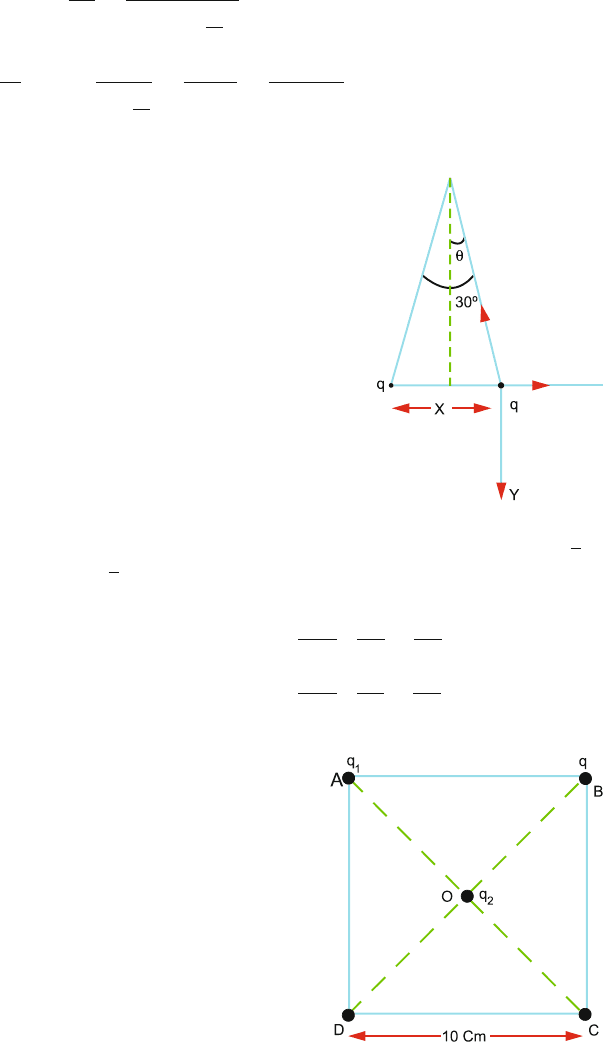
11.12 From the geometry of Fig. 11.22,AB= 0.1m, OB = OC = 0.05
√
2m,
AC = (0.1)
√
2m.
Potential energy of q at B is U(B) =
q
4πε
0
q
1
AB
+
q
2
OB
Potential energy of q at C is U(C) =
q
4πε
0
q
1
AC
+
q
2
OC
Fig. 11.22
