Kamal A.A. 1000 Solved Problems in Classical Physics: An Exercise Book
Подождите немного. Документ загружается.

438 10 Heat and Matter
10.28
−
dθ
dt
=
K
ms
(θ −θ
0
)(K = constant) (1)
Let x be the water equivalent of the calorimeter.
50 − 40
15
=
K
(x + 40 × 1)
50 + 40
2
− θ
0
(2)
50 − 40
33
=
K
(x + 100 × 1)
50 + 40
2
− θ
0
(3)
Dividing (2) by (3)
x + 100
x + 40
=
11
5
or x = 10 g
10.29 −
dθ
dt
=
K
ms
(θ −θ
0
)
Now K ∝ surface area or ∝ r
2
and m ∝ r
3
.
∴ dθ/dt ∝ 1/r
∴
(dθ/dt)
1
(dθ/dt)
2
=
r
2
r
1
=
2
1
10.30 Assuming a linear variation of resistance with temperature
R
T
= R
0
(1 + αT ) (1)
29.6 = 24.9(1 + 100α) (2)
whence α = 1.8875 × 10
−3
W/
◦
C(3)
26.3 = 24.9(1 + 1.8875 × 10
−3
T ) (4)
whence T = 29.79
◦
C
10.31 If R is the radius of the sun, r the mean distance of the earth from the sun,
E the energy emitted from 1 m
2
of the sun’s surface per second, T the abso-
lute temperature of the sun’s surface and σ the Boltzmann–Stefan constant,
then
S =
4π R
2
E
4πr
2
=
R
2
σ T
4
r
2
=
(6.95 × 10
8
)
2
(5.67 × 10
−8
)(5740)
4
(1.49 × 10
11
)
2
= 1339 W/m
2

10.3 Solutions 439
10.32 Radiant intensity at the sun’s surface is the power emitted by 1 m
2
of sun’s
surface.
σ T
4
= 63 ×10
6
T =
63 × 10
6
5.67 × 10
−8
1/4
= 5773 K
10.33
−
dE
dt
= σ A
T
4
1
− T
4
2
(Stefan–Boltzmann formula)
= σπr
2
T
4
1
− T
4
2
= π(5.67 ×10
−8
)(0.05)
2
(500
2
− 300
2
)
= 7.12 ×10
−5
W
10.34
λ
m
T = b = 3 × 10
−3
m K (Wien’s law) (1)
dE
dt
= σ T
4
(Boltzmann law) (2)
If dE/dt goes down to 1/16 of its original value then by (2) the temperature
T → T/2. Therefore λ
m
→ 2λ
m
by (1). Thus the wavelength under new
conditions λ
m
= 2λ
m
= 2 ×480 = 960 nm.
10.3.4 Specific Heat and Latent Heat
10.35
Q =
80
20
mC
p
dT + mL +
200
80
mC
p
dT
=
200
20
mC
p
dT + mL
(∵ C
p
relation for solid and
liquid phase is identical)
=
200
20
1 × (30.6 + 0.0103 T )dT + 1 × 6000
= 11710 J
10.36
C =
CdT
dT
=
T
0
(A + BT
2
)dT
T
=
1
T
AT +
BT
3
3
= A +
BT
2
3
C (midpoint) = A + B(T/2)
2
= A +
BT
2
4
∴
C − C (midpoint) = A +
BT
2
3
−
A +
BT
2
4
=
BT
2
12
440 10 Heat and Matter
10.37 Let the specific heats of liquids A, B and C be, respectively, C
A
, C
B
and C
C
.
When A and B are mixed, equilibrium of the mixture requires that
MC
A
(16 − 12) = MC
B
(18 − 16)
or C
B
= 2C
A
When B and C are mixed
MC
B
(23 − 18) = MC
C
(28 − 23)
or C
C
= C
B
= 2C
A
When A and C are mixed, let the equilibrium temperature be T .
MC
A
(T − 12) = MC
C
(28 − T ) = M2C
C
(28 − T )
∴ T = 22.67
◦
C
10.38
(a) The block is fixed. The kinetic energy of the bullet is entirely converted
into heat energy. Let m be the mass and v the velocity of the bullet.
Q =
1
2
mv
2
=
1
2
(3 × 10
−3
)(120)
2
= 21.6J= 5.167 cal
Rise in temperature
T =
Q
mc
=
5.167
3 × 0.031
= 55.56
◦
C
(b) The block is free to move. In this case, after the collision some kinetic
energy will go into the block + bullet system.
1
2
mv
2
=
1
2
(M + m)v
2
1
+ Q (energy conservation) (1)
mv = (M + m)v
1
(momentum conservation) (2)
where M is the mass of the block and v
1
the final velocity of the block
+ bullet system. Eliminating v
1
and simplifying
Q =
1
2
mv
2
M
M + m
=
1
2
× 3 × 10
−3
× (120)
2
50
50 + 3
= 20.38 J = 4.875 cal
T =
Q
mc
=
4.875
3 × 0.031
= 52.42
◦
C
10.39 Potential energy available from m kg of water through a fall of h metres is
mgh J. 15/100 mgh. Mechanical energy is converted into heat.

10.3 Solutions 441
∴
15
100
mgh = mcT × 4.18 = m × 1000T × 4.18
∴ T =
15 × 9.8 × 25
100 × 1000 × 4.18
= 0.0088
◦
C
10.40 Mechanical energy available, W = mgh
Heat absorbed, H = mcT J.
Loss of mechanical energy = gain of heat energy
∴ mgh = mcT J
∴ T =
gh
cJ
=
9.8 × 100
30.6 × 4.18
= 7.66
◦
C = 7.66 K
10.3.5 Thermodynamics
10.41
(a) (i) In an isobaric process pressure remains constant.
(ii) In an isochoric process volume remains constant.
(iii) In an adiabatic process heat is neither absorbed nor evolved by the
system.
(iv) In an isothermal process temperature remains constant.
(b) For adiabatic process use the relation
PV
γ
= P
1
V
γ
1
= const. (1)
or P =
P
1
V
γ
1
V
γ
(2)
Work done on the gas
W =−
v
2
v
1
PdV =−
v
2
v
1
P
1
V
γ
1
dV
V
γ
=−P
1
V
γ
1
v
2
v
1
dV
V
γ
P
1
V
γ
1
γ − 1
V
1−γ
2
− V
1−γ
1
=
1
γ − 1
P
1
V
γ
1
V
1−γ
2
− P
1
V
1
∴ W =
1
γ − 1
(
P
2
V
2
− P
1
V
1
)
(3)
where we have used the relation P
1
V
γ
1
= P
2
V
γ
2
.
W is positive if V
1
> V
2
(compression) and negative if V
1
< V
2
(expansion).
10.42 Applying the gas equation
T
A
=
P
A
V
A
nR
=
(1.013 × 10
5
)(44.8)
(2000)(8.31)
= 273 K

442 10 Heat and Matter
As the process AB is isometric (isochoric), V
B
= V
A
.
T
B
=
T
A
P
B
P
A
=
273 × 2
1
= 546 K
As the process CA is isobaric, Charles’ first law applies. Thus
V
C
T
C
=
V
A
T
A
→ V
C
=
V
A
T
C
T
A
As BC is an isothermal process, T
C
= T
B
= 546 K.
∴ V
C
=
V
A
T
B
T
A
=
(44.8)(546)
273
= 89.6m
3
10.43 For adiabatic process, dQ = 0sothatdU =−dW. The energy of 1 mol of
monatomic gas is given by
U =
3
2
RT
∴ dU =
3
2
R dT
dW = PdV =
RT
V
dV
∴
3
2
RdT =−
RT
V
dV
∴
dT
T
+
2
3
dV
V
= 0
Integrating
ln T +
2
3
ln V = constant
or TV
2/3
= constant
Eliminating T from the gas equation
PV
5/3
= constant
10.44
(a) U
f
−U
i
= U = Q − W (First law of thermodynamics)
Let a system change from an initial equilibrium state i to a final equi-
librium state f in a definite way, the heat absorbed by the system being
Q and the work done by the system being W. The quantity Q − W
represents the change in internal energy of the system.
Both Q and W are path dependent while U is path independent.

10.3 Solutions 443
(b)
W =
dW =−
P dV (1)
PV = nRT
1
= constant
or P =
nRT
1
V
(T = constant for isothermal process) (2)
Substituting (2) in (1)
W =−nRT
V
2
V
1
dV
V
=−nRT ln
V
2
V
1
(c) At the beginning of the cycle, P
1
= 1.01 × 10
5
Pa, V
1
= 1m
3
, T
1
=
273.15 K.
At the end of stage 1, P
2
= 5.05 ×10
4
Pa, V
2
= 2m
3
, T
2
= 273.15 K.
At the end of stage 2, P
3
= 1.01 ×10
5
Pa, V
3
= 2m
3
, T
3
= T
2
P
3
/P
2
=
546.3K.
(i) n =
P
1
V
1
RT
1
=
1.01 × 10
5
× 1.0
8.31 × 273.15
= 44.5mol
(ii)
Stage1, W
1
=−nRT ln
(
V
2
/V
1
)
=−44.5 × 8.31 × 273.15 ln(2/1) =−70046 J
Stage2, W
2
= P
2
V = P
3
(V
3
− V
2
) = P(V
2
− V
2
) = 0
Stage3, W
3
= P
3
(V
1
− V
3
) = 1.01 × 10
5
(1 − 2)
=−1.015 × 10
5
J
(iii) U = 0, overall.
10.45
(a) and (b)
P
1
V
1
T
1
=
P
2
V
2
T
2
∴ T
1
=
P
1
P
2
V
1
V
2
T
2
=
P
1
3P
1
V
1
V
1
1083 = 361 K
n =
P
1
V
1
RT
1
=
(10
5
)(0.06)
(8.31)(361)
= 2mol
P
3
V
3
T
3
=
P
2
V
2
T
2
∴ T
3
=
P
3
P
2
V
3
V
2
T
2
=
P
2
P
2
(V
2
/3)
V
2
1083 = 361 K
T
4
= T
3
= 361 K (∵ process 3 → 4 isothermal process)
P
4
V
4
T
4
=
P
3
V
3
T
3
444 10 Heat and Matter
∴ P
4
=
V
3
V
4
T
4
T
3
P
3
=
V
3
3V
3
T
3
T
3
P
2
=
P
2
3
=
3P
1
3
= P
1
= 10
5
N/m
2
V
4
=
nRT
4
P
4
=
2 × 8.31 × 361
10
5
= 0.06 m
3
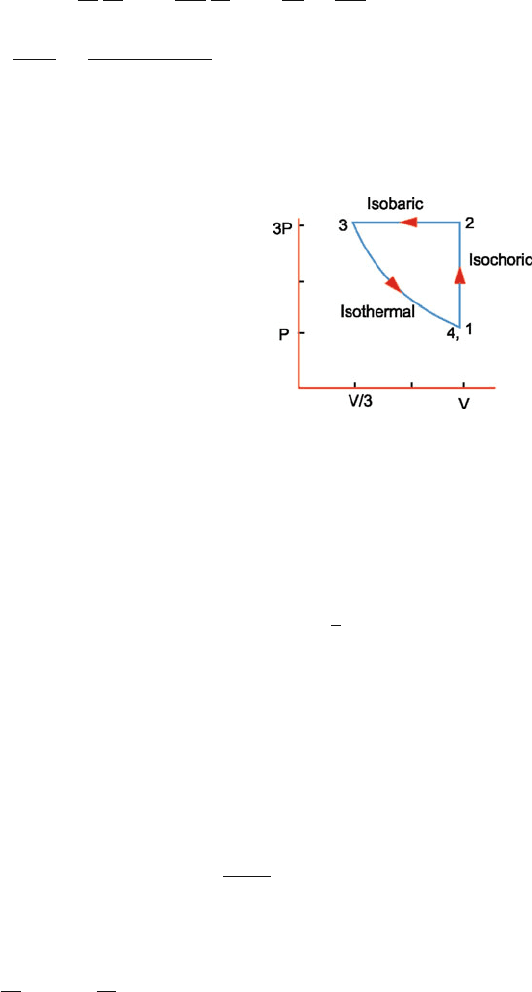
Thus the P, V , T coordinates of the initial and final points on the indi-
cator diagram are identical.
(c) Heat gained by N
2
in the first (isochoric) process (Fig. 10.10):
Fig. 10.10 The P–V diagram
C
V
= C
P
/γ = 29.12/1.4 = 20.8J/(mol K)
Q
1
= nC
v
T = 2 ×20.8 × (1083 − 311) = 32115 J
W
12
= 0(∵ process 1 → 2 is isochoric)
∴ U
1
= Q
1
= 32115 J
Q
2
= nC
p
T = 2 ×29.12 × (361 − 1083) =−42049 J
W
23
= PV = P
2
(V
3
− V
2
) =−3 × 10
5
×
2
3
× 0.06 =−12, 000 J
∴ U
2
= Q
3
− W
23
=−42049 + 12000 =−30049 J
U
3
= 0(∵ process 3 → 4 is isothermal)
(d) Net change in energy
U = U
1
+ U
2
+ U
3
= 32115 −30049 + 0 = 2066 J
The expected value is zero.
10.46 Number of degrees of freedom, f =
2
γ − 1
we can find γ from the relation
T
2
V
γ −1
2
= T
1
V
γ −1
1
∴
V
2
V
1
γ −1
=
T
1
T
2
= 1.32
∴ 2
γ −1
= 1.32

10.3 Solutions 445
whence γ = 1 +
log 1.32
log 2.0
= 1.4
∴ f =
2
1.4 − 1
= 5
10.47 The efficiency of the engine is
e = 1 −
T
2
T
1
= 1 −
300
400
= 0.25 or 25%
Work done by the engine
W = e × Q
1
= 0.25 ×10
8
cal
= 0.25 ×10
8
× 4.18 J
= 1.05 ×10
8
J
10.48
e = 1 −
T
2
T
1
=
1
6
(1)
∴ 5T
1
− 6T
2
= 0(2)
When the temperature of the sink is reduced by 62
◦
C, the efficiency becomes
e
= 2e (3)
e
= 2e = 1 −
(T
2
− 62)
T
1
=
1
3
(4)
∴ 2T
1
− 3T
2
− 186 = 0(5)
Solving (2) and (5), T
1
= 372 K = 99
◦
C, T
2
= 310 K = 37
◦
C.
10.49
(a)
PV = P
0
V
0
(isothermal conditions) (1)
Dividing by m, the mass of air
PV
m
=
P
0
V
0
m
or
P
ρ
=
P
0
ρ
0
∴ ρ =
Pρ
0
P
0
(2)
where the density of air on earth’s surface is ρ
0
and pressure is P
0
,the
corresponding quantities at height y being ρ and P.

446 10 Heat and Matter
Now, for a small increase in height dy, the pressure decreases by dp and
is given by
dp =−ρg dy =−
P
P
0
ρ
0
gdy (3)
where we have used (2). The negative sign shows that the pressure
decreases as the height increases.
Integrating
h
0
dy =−
P
0
ρ
0
g
P
P
0
dp
P
∴ h =−
p
0
ρ
0
g
ln
p
P
0
∴ p = p
0
exp
−
ρ
0
gh
P
0
(4)
Now, P
0
V
0
= μRT (5)
since the temperature is assumed to be constant and μ is in moles. Fur-
thermore
ρ
0
=
μM
V
0
(6)
where M is the molecular weight. Combining (5) and (6)
ρ
0
P
0
=
M
RT
(7)
Substituting (7) in (4)
p = p
0
exp
−
Mgh
RT
(8)
(b) If n is the number density, that is, the number of molecules per unit
volume and m
0
the mass of each molecule then
ρ = m
0
n
and ρ
0
= m
0
n
0
∴
ρ
ρ
0
=
n
n
0
(9)

10.3 Solutions 447
Combining (9) with (2)
p
P
0
=
n
n
0
(10)
Using (10) in (8)
n = n
0
exp
−
Mgh
RT
(11)
(c)
P
P
0
=
1
2
= exp
−
Mgh
RT
∴ h =
RT
Mg
ln 2 =
8.31 × 273 × 0.693
0.029 × 9.8
= 54224 m 54 km
10.50
(a) For a Carnot cycle
(i) e =
Q
H
− Q
C
Q
H
(ii) e =
T
H
− T
C
T
H
The symbols H and C are for hot and cold reservoirs.
(b) The Otto cycle, Fig. 10.11, consists of two reversible adiabatic processes
(paths AB and CD) and two reversible isochoric processes (path DA
and BC).
Suppose we start at the point C. The temperature T
C
at C i s low,
slightly above atmospheric temperature. The cylinder is filled with air
charged with the combustible gas or vapour. The air is compressed adia-
batically to the point D. At D a spark causes combustion, heating the air
at constant volume to the point A. The heated air expands adiabatically
along the path AB. At B, a valve is opened and the pressure drops to that
of the atmosphere. The point C is reached at constant volume. The cycle
is complete.
Fig. 10.11 The Otto cycle
