Jost J. Partial Differential Equations
Подождите немного. Документ загружается.


3.3 The Alternating Method of H.A. Schwarz 67
We exclude the trivial case ϕ = const. Let u
1
: Ω
1
→ R be harmonic with
boundary values
u
1
|
Γ
1
= ϕ
1
,u
1
|
γ
1
= M. (3.3.1)
Next, let u
2
: Ω
2
→ R be harmonic with boundary values
u
2
|
Γ
2
= ϕ
2
,u
2
|
γ
2
= u
1
|
γ
2
. (3.3.2)
Unless ϕ
1
≡ M, by the strong maximum principle,
u
1
<M in Ω
1
;
1
(3.3.3)
hence in particular,
u
2
|
γ
2
<M, (3.3.4)
and by the strong maximum principle, also
u
2
<M in Ω
2
, (3.3.5)
and thus in particular,
u
2
|
γ
1
<u
1
|
γ
1
. (3.3.6)
If ϕ
1
≡ M, then by our assumption that ϕ ≡ const is excluded, ϕ
2
≡ M,and
(3.3.6) likewise holds by the maximum principle. Since by (3.3.2), u
1
and u
2
coincide on the partition of the boundary of Ω
∗
, by the maximum principle
again
u
2
<u
1
in Ω
∗
.
Inductively, for n ∈ N,let
u
2n+1
: Ω
1
→ R,
u
2n+2
: Ω
2
→ R,
be harmonic with boundary values
u
2n+1
|
Γ
1
= ϕ
1
,u
2n+1
|
γ
1
= u
2n
|
γ
1
, (3.3.7)
u
2n+2
|
Γ
2
= ϕ
2
,u
2n+2
|
γ
2
= u
2n+1
|
γ
2
. (3.3.8)
From repeated application of the strong maximum principle, we obtain
1
The boundary values here are not continuous as in the maximum principle, but
they can easily be approximated by continuous ones satisfying the same bounds.
This easily implies that the maximum principle continues to hold in the present
situation.
68 3. Existence Techniques I: Methods Based on the Maximum Principle
u
2n+3
<u
2n+2
<u
2n+1
on Ω
∗
, (3.3.9)
u
2n+3
<u
2n+1
on Ω
1
, (3.3.10)
u
2n+4
<u
2n+2
on Ω
2
. (3.3.11)
Thus, our sequences of functions are monotonically decreasing. Since they
are also bounded from below by m, they converge to some limit
u : Ω → R.
The Harnack convergence theorem (1.2.10) ) then implies that u is harmonic
on Ω
1
and Ω
2
, hence also on Ω = Ω
1
∪Ω
2
. This can also be directly deduced
from the maximum principle: For simplicity, we extend u
n
to all of Ω by
putting
u
2n+1
:= u
2n
on Ω
2
\ Ω
∗
,
u
2n+2
:= u
2n+1
on Ω
1
\ Ω
∗
.
Then u
2n+1
is obtained from u
2n
by harmonic replacement on Ω
1
, and anal-
ogously, u
2n+2
is obtained from u
2n+1
by harmonic replacement on Ω
2
.We
write this symbolically as
u
2n+1
= P
1
u
2n
, (3.3.12)
u
2n+2
= P
2
u
2n+1
. (3.3.13)
For example, on Ω
1
we then have
u = lim
n→∞
u
2n
= lim
n→∞
P
1
u
2n
. (3.3.14)
By the maximum principle, the uniform convergence of the boundary values
(in order to get this uniform convergence, we may have to restrict ourselves
to an arbitrary subdomain Ω
1
⊂⊂ Ω
1
) implies the uniform convergence of
the harmonic extensions. Consequently, the harmonic extension of the limit
of the boundary values equals the limit of the harmonic extensions, i.e.,
P
1
lim
n→∞
u
2n
= lim
n→∞
P
1
u
2n
. (3.3.15)
Equation (3.3.14) thus yields
u = P
1
u, (3.3.16)
meaning that on Ω
1
, u coincides with the harmonic extension of its boundary
values, i.e., is harmonic. For the same reason, u is harmonic on Ω
2
.
We now assume that the boundary values ϕ are continuous, and that all
boundary points of Ω
1
and Ω
2
are regular. Then first of all it is easy to
see that u assumes its boundary values ϕ on ∂Ω \(Γ
1
∩Γ
2
) continuously. To
verify this, we carry out the same alternating process with harmonic functions
v
2n−1
: Ω
1
→ R, v
2n
: Ω
2
→ R starting with boundary values

3.3 The Alternating Method of H.A. Schwarz 69
v
1
|
Γ
1
= ϕ
1
,v
1
|
γ
1
= m (3.3.17)
in place of (3.3.1). The resulting sequence (v
n
)
n∈N
then is monotonically
increasing, and the maximum principle implies
v
n
<u
n
in Ω for all n. (3.3.18)
Since we assume that ∂Ω
1
and ∂Ω
2
are regular and ϕ is continuous, u
n
and
v
n
then are continuous at every x ∈ ∂Ω \(Γ
1
∩Γ
2
). The monotonicity of the
sequence (u
n
), the fact that u
n
(x)=v
n
(x)=ϕ(x)forx ∈ ∂Ω \(Γ
1
∩Γ
2
)for
all n, and (3.3.18) then imply that u = lim
n→∞
u
n
at x as well.
The question whether u is continuous at ∂Ω
1
∩∂Ω
2
is more difficult, as can
be expected already from the observation that the chosen boundary values
for u
1
typically are discontinuous there even for continuous ϕ.Inordertobe
able to treat that issue here in an elementary manner, we add the hypotheses
that the boundaries of Ω
1
and Ω
2
are of class C
1
in some neighborhood
of their intersection, and that they intersect at a nonzero angle. Under this
hypotheses, we have the following lemma:
Lemma 3.3.1: There exists some q<1, depending only on Ω
1
and Ω
2
,with
the following property: If w :
Ω
1
→ R is harmonic in Ω
1
, and continuous on
the closure
¯
Ω
1
,andif
w =0 on Γ
1
,
|w|≤1 on γ
1
,
then
|w|≤q on γ
2
, (3.3.19)
and a corresponding result holds if the roles of Ω
1
and Ω
2
are interchanged.
The proof will be given in Section 3.4 below.
With the help of this lemma we may now modify the alternating method in
such a manner that we also get continuity on ∂Ω
1
∩ ∂Ω
2
. For that purpose,
we choose an arbitrary continuous extension ¯ϕ of ϕ to γ
1
, and in place of
(3.3.1), for u
1
we require the boundary condition
u
1
|
Γ
1
= ϕ
1
,u
1
|
γ
1
=¯ϕ, (3.3.20)
and otherwise carry through the same procedure as above. Since the bound-
aries ∂Ω
1
, ∂Ω
2
are assumed regular, all u
n
then are continuous up to the
boundary. We put
M
2n+1
:= max
γ
2
|u
2n+1
− u
2n−1
|,
M
2n+2
:= max
γ
1
|u
2n+2
− u
2n
|.

70 3. Existence Techniques I: Methods Based on the Maximum Principle
On γ
2
,wethenhave
u
2n+2
= u
2n+1
,u
2n
= u
2n−1
,
hence
u
2n+2
− u
2n
= u
2n+1
− u
2n−1
,
and analogously on γ
1
,
u
2n+3
− u
2n+1
= u
2n+2
− u
2n
.
Thus applying the lemma with w =
(u
2n+3
−u
2n+1
)
M
2n+2
, we obtain
M
2n+3
≤ qM
2n+2
and analogously
M
2n+2
≤ qM
2n+1
.
Thus M
n
converges to 0 at least as fast as the geometric series with coefficient
q<1. This implies the uniform convergence of the series
u
1
+
∞
n=1
(u
2n+1
− u
2n−1
) = lim
n→∞
u
2n+1
on
¯
Ω
1
, and likewise the uniform convergence of the series
u
2
+
∞
n=1
(u
2n+2
− u
2n
) = lim
n→∞
u
2n
on
¯
Ω
2
. The corresponding limits again coincide in Ω
∗
, and they are harmonic
on Ω
1
, respectively Ω
2
, so that we again obtain a harmonic function u on Ω.
Since all the u
n
are continuous up to the boundary and assume the boundary
values given by ϕ on ∂Ω, u then likewise assumes these boundary values
continuously.
We have proved the following theorem:
Theorem 3.3.1: Let Ω
1
and Ω
2
be bounded domains all of whose boundary
points are regular for the Dirichlet problem. Suppose that Ω
1
∩ Ω
2
= ∅ and
that Ω
1
and Ω
2
are of class C
1
in some neighborhood of ∂Ω
1
∩∂Ω
2
, and that
they intersect there at a nonzero angle. Then the Dirichlet problem for the
Laplace equation on Ω := Ω
1
∪ Ω
2
is solvable for any continuous boundary
values.
3.4 Boundary Regularity 71
3.4 Boundary Regularity
Our first task is to present the proof of Lemma 3.3.1:
In the sequel, with r := |x − y| = 0, we put
Φ(r):=−dw
d
Γ (r)=
ln
1
r
for d =2,
1
d−2
1
r
d−2
for d ≥ 3.
(3.4.1)
We then have for all ν ∈ R
n
,
∂
∂ν
Φ(r)=∇Φ · ν = −
1
r
d
(x − y) · ν. (3.4.2)
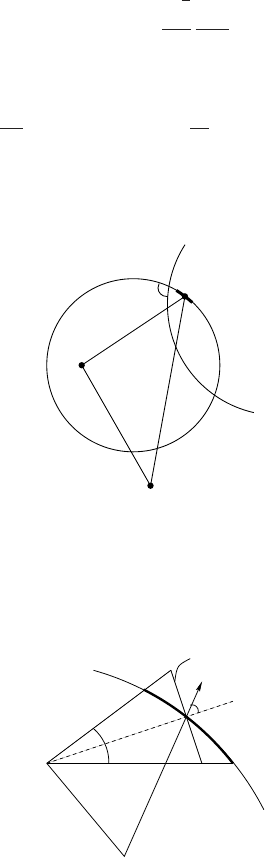
We consider the situation depicted in Figure 3.3.
Γ
1
O
x
y
γ
2
Ω
1
Γ
2
Ω
2
γ
1
dγ
1
(y)
α
Figure 3.3.
That is, x ∈ Ω
1
; y ∈ γ
1
, α =0,π,∂Ω
1
,∂Ω
2
∈ C
1
.Letdγ
1
(y) be an infinites-
imal boundary portion of γ
1
(see Figure 3.4).
O
x
y
dω
γ
1
dγ
1
(y)
ν
dγ
1
(y)cosβ
β
Figure 3.4.
72 3. Existence Techniques I: Methods Based on the Maximum Principle
Let dω be the infinitesimal spatial angle at which the boundary piece dγ
1
(y)
is seen from x.Wethenhave
dγ
1
(y)cosβ = |x − y|
d−1
dω (3.4.3)
and cos β =
y−x
|y−x|
. This and (3.4.2) imply
h(x):=
γ
1
∂
∂ν
Φ(r)dγ
1
(y)=
γ
1
dω. (3.4.4)
The geometric meaning of (3.4.4) is that
γ
1
∂Φ
∂ν
(r)dγ
1
(y) describes the spa-
tial angle at which the boundary piece γ
1
is seen at x. Since derivatives of
harmonic functions are harmonic as well, (3.4.4) yields a function h that is
harmonic on Ω
1
and continuous on ∂Ω
1
\ (Γ
1
∩ Γ
2
). In order to make the
proof of Lemma 3.3.1 geometrically as transparent as possible, from now on,
we only consider the case d = 2 and point out that the proof in the case
d ≥ 3 proceeds analogously.
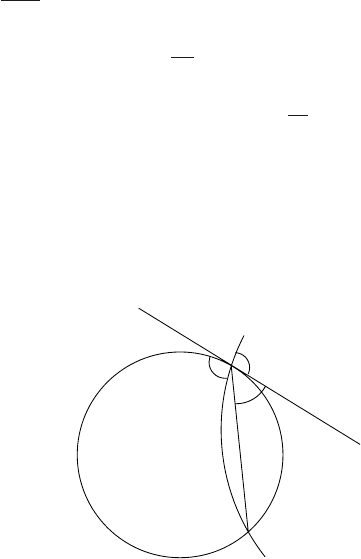
α
α
B
γ
2
s
γ
1
β
t
A
Γ
1
Ω
1
Γ
2
Figure 3.5.
Let A and B be the two points where Γ
1
and Γ
2
intersect (Figure 3.5). Then
h is not continuous at A and B, because
lim
x→A
x∈Γ
1
h(x)=β, (3.4.5)
lim
x→A
x∈γ
1
h(x)=β + π, (3.4.6)
lim
x→A
x∈γ
2
h(x)=α + β. (3.4.7)
Let
ρ(x):=π for x ∈ γ
1
and

3.4 Boundary Regularity 73
ρ(x):=0 forx ∈ Γ
1
.
Then h|
∂Ω
1
− ρ is continuous on all of ∂Ω
1
, because
lim
x→A
x∈Γ
1
(h(x) − ρ(x)) = lim
x→A
x∈Γ
1
h(x) − 0=β,
lim
x→A
x∈γ
1
(h(x) − ρ(x)) = lim
x→A
x∈γ
1
h(x) − π = β + π − π = β.
By assumption, there then exists a function u ∈ C
2
(Ω
1
) ∩ C
0
(
¯
Ω
1
)with
Δu =0 inΩ
1
,
u = h|
∂Ω
1
− ρ on ∂Ω
1
.
For
v(x):=
h(x) − u(x)
π
(3.4.8)
we have
Δv =0 forx ∈ Ω
1
,
v(x)=0 forx ∈ Γ
1
,
v(x)=1 forx ∈ γ
1
.
The strong maximum principle thus implies
v(x) < 1 for all x ∈ Ω
1
, (3.4.9)
and in particular,
v(x) < 1 for all x ∈ γ
2
. (3.4.10)
Now
lim
x→A
x∈γ
2
v(x)=
1
π
lim
x→A
x∈γ
2
h(x) − β
=
α
π
< 1, (3.4.11)
since α<πby assumption. Analogously, lim
x→B
x∈γ
2
v(x) < 1, and hence since
¯γ
2
is compact,
v(x) <q<1 for all x ∈ ¯γ
2
(3.4.12)
for some q>0. We put m := v − w and obtain
m(x)=0 forx ∈ Γ
1
,
m(x) ≥ 0forx ∈ γ
1
.
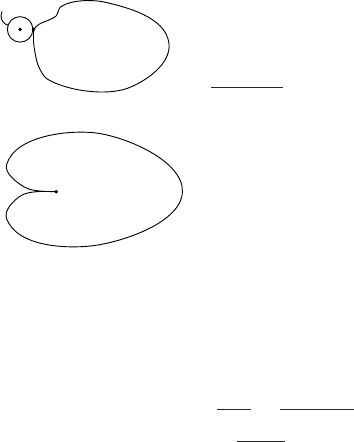
74 3. Existence Techniques I: Methods Based on the Maximum Principle
Since m is continuous in ∂Ω
1
\ (Γ
1
∩ Γ
2
), and ∂Ω
1
is regular, it follows that
lim
x→x
0
m(x)=m(x
0
) for all x
0
∈ ∂Ω
1
\ (Γ
1
∩ Γ
2
).
By the maximum principle, m(x) ≥ 0 for all x ∈ Ω
1
, and since also
lim
x→A
m(x) = lim
x→A
v(x) − w(A) = lim
x→A
v(x) ≥ 0(w is continuous),
we have for all x ∈ ¯γ
2
,
w(x) ≤ v(x) <q<1. (3.4.13)
The analogous considerations for M := v + w yield the inequality
−w(x) ≤ v(x) <q<1; (3.4.14)
hence, altogether,
|w(x)| <q<1 for all x ∈ ¯γ
2
.
(a)
(b)
Ω
y
y
x
B(s, x)
Ω
Figure 3.6.
We now wish to present a sufficient condition for
the regularity of a boundary point y ∈ ∂Ω:
Definition 3.4.1: Ω satisfies an exterior sphere
condition at y ∈ ∂Ω if there exists x
0
∈ R
n
with
B(ρ, x
0
) ∩
¯
Ω = {y}.
Examples: (a) All convex regions and all re-
gions of class C
2
satisfy an exterior sphere
condition at every boundary point. (See Fig-
ure 3.6(a).)
(b) At inward cusps, the exterior sphere condi-
tion does not hold. (See Figure 3.6(b).)
Lemma 3.4.1: If Ω satisfies an exterior sphere
condition at y, then ∂Ω is regular at y.
Proof:
β(x):=
1
ρ
d−2
−
1
|x−x
0
|
d−2
for d ≥ 3,
ln
|x−x
0
|
ρ
for d =2,
yields a barrier at y. Namely, β(y) = 0, and β is harmonic in R
n
\{x
0
}, hence
in particular in Ω.Sinceforx ∈
¯
Ω \{y}, |x − x
0
| >,alsoβ(x) > 0 for all
x ∈
¯
Ω \{y}.
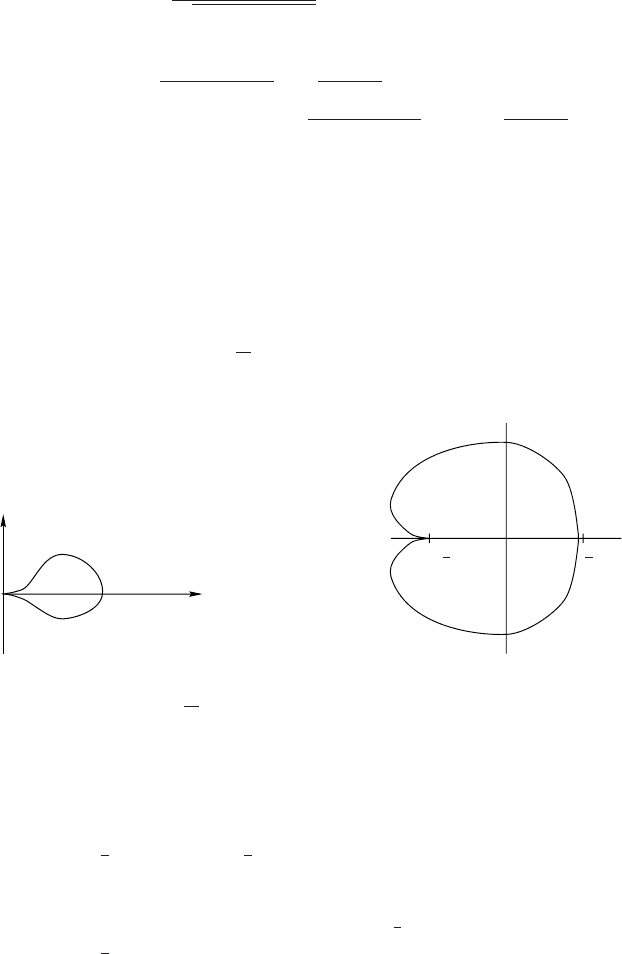
3.4 Boundary Regularity 75
We now wish to present Lebesgue’s example of a nonregular boundary point,
constructing a domain with a sufficiently pointed inward cusp.
Let R
3
= {(x, y, z)}, x ∈ [0, 1], ρ
2
:= y
2
+ z
2
,
u(x, y, z):=
1
0
x
0
(x
0
− x)
2
+ ρ
2
dx
0
= v(x, ρ) − 2x ln ρ
with
v(x, ρ)=
(1 − x)
2
+ ρ
2
−
x
2
+ ρ
2
+ x ln
1 − x +
(1 − x)
2
+ ρ
2
x +
x
2
+ ρ
2
.
We have
lim
(x,ρ)→0
x>0
v(x, ρ)=1.
The limiting value of −2x ln ρ, however, crucially depends on the sequence
(x, ρ) converging to 0. For example, if ρ = |x|
n
,wehave
−2x ln ρ = −2nx ln |x|
x→0
−−−→ 0.
On the other hand, if ρ = e
−
k
2x
, k, x > 0, we have
lim
(x,ρ)→0
(−2x ln ρ)=k>0.
x0
y, z
Figure 3.7.
Ω
−
1
2
0
1
2
Figure 3.8.
The surface ρ = e
−
k
2x
has an “infinitely pointed” cusp at 0. (See Figure
3.7.)
Considering u as a potential, this means that the equipotential surfaces of u
for the value 1 + k come together at 0, in such a manner that f
(0) = 0 if the
equipotential surface is given by ρ = f(x). With Ω as an equipotential surface
for 1 + k,thenu solves the exterior Dirichlet problem, and by reflection at
the ball (x −
1
2
)
2
+ y
2
+ z
2
=
1
4
, one obtains a region Ω
as in Figure 3.8).
Depending on the manner, in which one approaches the cusp, one obtains
different limiting values, and this shows that the solution of the potential
problem cannot be continuous at (x, y, z)=
−
1
2
, 0, 0
, and hence ∂Ω
is not
regular at
−
1
2
, 0, 0
.

76 3. Existence Techniques I: Methods Based on the Maximum Principle
Summary
The maximum principle is the decisive tool for showing the convergence of
various approximation schemes for harmonic functions. The difference meth-
ods replace the Laplace equation, a differential equation, by difference equa-
tions on a discrete grid, i.e., by finite-dimensional linear systems. The max-
imum principle implies uniqueness, and since we have a finite-dimensional
system, then it also implies the existence of a solution, as well as the control
of the solution by its boundary values.
The Perron method constructs a harmonic function with given boundary
values as the supremum of all subharmonic functions with those boundary
values. Whether this solution is continuous at the boundary depends on the
geometry of the boundary, however.
The alternating method of H.A. Schwarz obtains a solution on the union
of two overlapping domains by alternately solving the Dirichlet problem on
each of the two domains with boundary values in the overlapping part coming
from the solution of the previous step on the other domain.
Exercises
3.1 Employing the notation of Section 3.1, let x
0
∈ Ω
h
⊂ R
2
h
have neighbors
x
1
,...,x
4
.Letx
5
,...,x
8
be those points in R
3
that are neighbors of
exactly two of the points x
1
,...,x
4
. We put
˜
Ω
h
:= {x
0
∈ Ω
h
: x
1
,...,x
8
∈
¯
Ω
h
).
For u :
¯
Ω
h
→ R, x
0
∈
˜
Ω
h
, we put
˜
Δ
h
u(x
0
)=
1
6h
2
⎛
⎝
4
4
α=1
u(x
α
)+
8
β=5
u(x
β
) − 20u(x
0
)
⎞
⎠
.
Discuss the solvability of the Dirichlet problem for the corresponding
Laplace and Poisson equations.
3.2 Let x
0
∈ Ω
h
have neighbors x
1
,...,x
2d
. We consider a difference operator
Lu for u :
Ω
h
→ R,
Lu(x
0
)=
2d
α=0
b
α
u(x
α
),
satisfying the following assumptions:
b
α
≥ 0forα =1,...,2d,
2d
α=1
b
α
> 0,
2d
α=0
b
α
≤ 0.
Prove the weak maximum principle: Lu ≥ 0inΩ
h
implies
max
Ω
h
u ≤ max
Γ
h
u.
