Jost J. Partial Differential Equations
Подождите немного. Документ загружается.


4.2 The Fundamental Solution of the Heat Equation 97
Δw(x, t) −
∂
∂t
w(x, t)=φ(x, t) in Ω × (0, ∞),
w(x, t)=γ(x, t) in ∂Ω × (0, ∞). (4.2.27)
Then
lim
t→∞
w(x, t)=0 uniformly in x ∈ Ω. (4.2.28)
Proof: We choose R>0 such that
2x
1
<R for all x =(x
1
,...,x
d
) ∈ Ω, (4.2.29)
and consider
k(x):=e
R
− e
x
1
. (4.2.30)
Then
Δk = −e
x
1
.
With κ := inf
x∈Ω
e
x
1
,wethushave
Δk ≤−κ. (4.2.31)
We consider, with constants η,c
0
,τ to be determined, and with
κ
0
:= inf
x∈Ω
k(x),κ
1
:= sup
x∈Ω
k(x),
the expression
m(x, t):=η
k(x)
κ
+ η
k(x)
κ
0
+ c
0
k(x)
κ
0
e
−
κ
κ
1
(t−τ)
(4.2.32)
in Ω ×[τ,∞).
Then
Δm(x, t) −
∂
∂t
m(x, t)
< −η − η
κ
κ
0
− c
0
κ
κ
0
e
−
κ
κ
1
(t−τ)
+ c
0
κ
1
κ
0
κ
κ
1
e
−
κ
κ
1
(t−τ)
< −η. (4.2.33)
Furthermore,
m(x, τ ) >c
0
for x ∈ Ω, (4.2.34)
m(x, t) >η for (x, t) ∈ ∂Ω × [τ,∞). (4.2.35)
By our assumptions (4.2.25), (4.2.26), for every η, there exists some τ = τ(η)
with

98 4. Existence Techniques II: Parabolic Methods. The Heat Equation
|φ(x, t)| <η for x ∈ Ω, t ≥ τ, (4.2.36)
|γ(x, t)| <η for x ∈ ∂Ω, t ≥ τ. (4.2.37)
In (4.2.32) we now put
τ = τ(η),c
0
=sup
x∈Ω
|w(x, τ )|.
Then
m(x, τ ) ± w(x, τ ) ≥ 0forx ∈ Ω by (4.2.34),
m(x, t) ± w(x, t) ≥ 0forx ∈ ∂Ω, t ≥ τ,
by (4.2.35), (4.2.37), (4.2.27);
Δ −
∂
∂t
(m(x, t) ± w(x, t)) ≤ 0forx ∈ Ω, t ≥ τ,
by (4.2.33), (4.2.36), (4.2.27).
It follows from Theorem 4.1.1 (observe that it is irrelevant that our functions
are defined only on Ω × [τ,∞) instead of Ω × [0, ∞), and initial values are
given on Ω ×{τ})that
|w(x, t)|≤m(x, t)forx ∈ Ω, t > τ,
≤ η
κ
1
κ
+
κ
1
κ
0
+ c
0
κ
1
κ
0
e
−
κ
κ
1
(t−τ)
,
and this becomes smaller than any given ε>0ifη>0 from (4.2.36), (4.2.37)
is sufficiently small and t>τ(η) is sufficiently large.
4.3 The Initial Boundary Value Problem
for the Heat Equation
In this section, we wish to study the initial boundary value problem for the
inhomogeneous heat equation
u
t
(x, t) − Δu(x, t)=ϕ(x, t)forx ∈ Ω, t > 0,
u(x, t)=g(x, t)forx ∈ ∂Ω,t > 0,
u(x, 0) = f(x)forx ∈ Ω,
(4.3.1)
with given (continuous and smooth) functions ϕ, g, f. We shall need some
preparations.
Lemma 4.3.1: Let Ω be a bounded domain of class C
2
in R
d
. Then for every
α<
d
2
+1, T>0 there exists a constant c = c(α, T, d, Ω) such that for all
x
0
,x∈ ∂Ω, 0 <t≤ T , letting ν denote the exterior normal of ∂Ω, we have
∂K
∂ν
x
(x, x
0
,t)
≤ ct
−α
|x − x
0
|
−d+2α
.
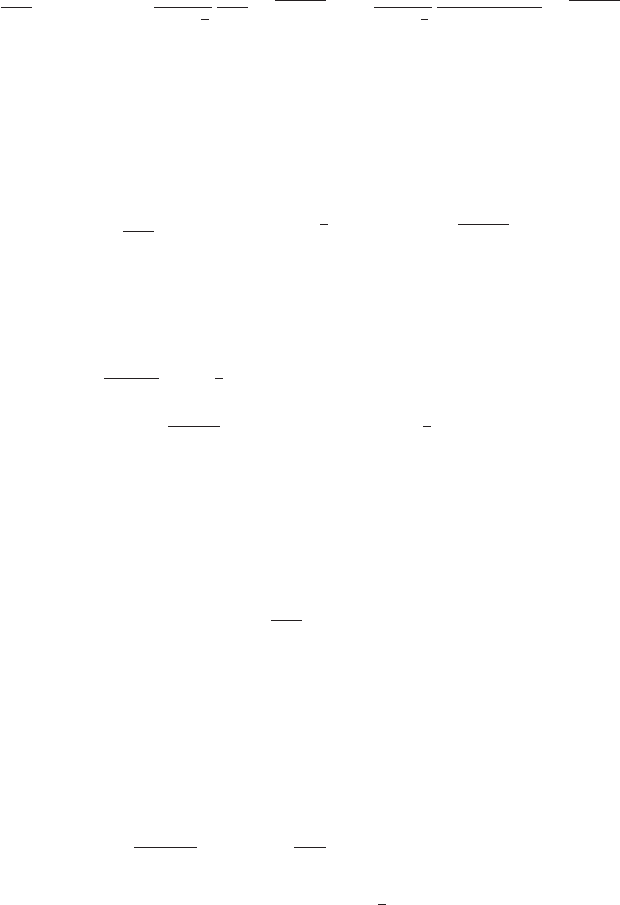
4.3 The Initial Boundary Value Problem for the Heat Equation 99
Proof:
∂
∂ν
x
K(x, x
0
,t)=
1
(4πt)
d
2
∂
∂ν
x
e
−
|x−x
0
|
2
4t
= −
1
(4πt)
d
2
(x − x
0
) · ν
x
2t
e
−
|x−x
0
|
2
4t
.
As we are assuming that the boundary of Ω is a manifold of class C
2
,and
since x, x
0
∈ ∂Ω,andν
x
is normal to ∂Ω,wehave
|(x − x
0
) · ν
x
|≤c
1
|x − x
0
|
2
with a constant c
1
depending on the geometry of ∂Ω.Thus
∂
∂ν
x
K(x, x
0
,t)
≤ c
2
t
−
d
2
−1
|x − x
0
|
2
e
−
|x−x
0
|
2
4t
(4.3.2)
with some constant c
2
. With a parameter β>0, we now consider the function
ψ(s):=s
β
e
−s
for s>0. (4.3.3)
Inserting s =
|x−x
0
|
2
4t
, β =
d
2
+1− α, we obtain from (4.3.3)
e
−
|x−x
0
|
2
4t
≤ c
3
|x − x
0
|
−d−2+2α
t
d
2
+1−α
, (4.3.4)
with c
3
depending on β, i.e., on d and α. Inserting (4.3.4) into (4.3.2) yields
the assertion.
Lemma 4.3.2: Let Ω ⊂ R
d
be a bounded domain of class C
2
with exterior
normal ν,andletγ ∈ C
0
(∂Ω × [0,T]) (T>0). We put
v(x, t):=−
t
0
∂Ω
∂K
∂ν
y
(x, y, τ)γ(y,t −τ)do(y)dτ. (4.3.5)
We then have
v ∈ C
∞
(Ω ×[0,T]),
v(x, 0) = 0 for all x ∈ Ω, (4.3.6)
and for all x
0
∈ ∂Ω, 0 <t≤ T ,
lim
x→x
0
v(x, t)=
γ(x
0
,t)
2
−
t
0
∂Ω
∂K
∂ν
y
(x
0
,y,τ)γ(y, t −τ)do(y)dτ. (4.3.7)
Proof: First of all, Lemma 4.3.1, with α =
3
4
, implies that the integral in
(4.3.5) indeed exists. The C
∞
-regularity of v with respect to x then follows
from the corresponding regularity of the kernel K by the change of variables
σ = t − τ . Equation (4.3.6) is obvious as well. It remains to verify the jump
relation (4.3.7). For that purpose, it obviously suffices to investigate

100 4. Existence Techniques II: Parabolic Methods. The Heat Equation
−
τ
0
0
∂Ω∩B(x
0
,δ)
∂K
∂ν
y
(x, y, τ)γ(y,t −τ)do(y)dτ (4.3.8)
for arbitrarily small τ
0
> 0, δ>0. In particular, we may assume that δ
0
and
τ are chosen such that for any given ε>0, we have for y ∈ ∂Ω, |y − x
0
| <δ,
and 0 ≤ τ<τ
0
,
|γ(x
0
,t) −γ(y, t − τ )| <ε.
Thus, we shall have an error of magnitude controlled by ε if in place of (4.3.8),
we evaluate the integral
−
τ
0
0
∂Ω∩B(x
0
,δ)
∂K
∂ν
y
(x, y, τ)γ(x
0
,t)do(y)dτ. (4.3.9)
Extracting the factor γ(x
0
,t) it remains to show that
− lim
x→x
0
τ
0
0
∂Ω∩B(x
0
,δ)
∂K
∂ν
y
(x, y, τ)do(y)dτ =
1
2
+ O(δ). (4.3.10)
Also, we observe that since γ is continuous, it suffices to show that (4.3.10)
holds uniformly in x
0
if x approaches ∂Ω in the direction normal to ∂Ω.In
other words, letting ν(x
0
) denote the exterior normal vector of ∂Ω at x
0
,we
may assume
x = x
0
− μν(x
0
).
In that case, μ
2
= |x −x
0
|
2
, and since ∂Ω is of class C
2
,fory ∈ ∂Ω,
|x − y|
2
= |y − x
0
|
2
+ μ
2
+ O
|y − x
0
|
2
|x − x
0
|
.
The term O
|y − x
0
|
2
|x − x
0
|
here is a higher-order term that does not
influence the validity of our subsequent limit processes, and so we shall omit
it in the sequel for the sake of simplicity. Likewise, for y ∈ ∂Ω,
(x − y) · ν
y
=(x − x
0
) · ν
y
+(x
0
− y) · ν
y
= −μ + O
|x
0
− y|
2
,
and the term O(|x
0
− y|
2
) may be neglected again.
Thus we approximate
∂K
∂ν
y
(x, y, τ)=
1
(4πτ)
d
2
(x − y) · ν
y
2τ
e
−
|x−y|
2
4τ
by
1
(4πτ)
d
2
(−μ)
2τ
e
−
|x
0
−y|
2
4τ
e
−
μ
2
4τ
.
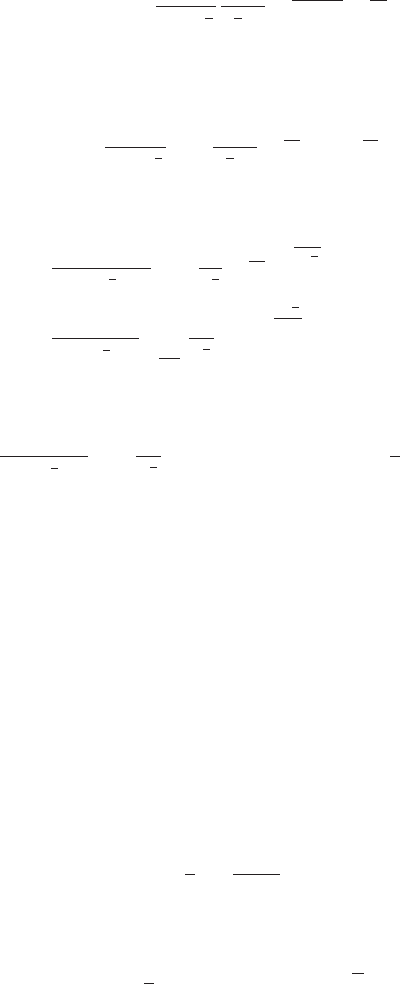
4.3 The Initial Boundary Value Problem for the Heat Equation 101
This means that we need to estimate the expression
τ
0
0
∂Ω∩B(x
0
,δ)
1
2(4π)
d
2
μ
τ
d
2
+1
e
−
|x
0
−y|
2
4τ
e
−
μ
2
4τ
do(y)dτ.
We introduce polar coordinates with center x
0
and put σ = |x
0
− y|.Wethen
obtain, again up to a higher-order error term,
μVol(S
d−2
)
1
2(4π)
d
2
τ
0
0
1
τ
d
2
+1
e
−
μ
2
4τ
δ
0
e
−
r
2
4τ
r
d−2
dr dτ,
where S
d−2
is the unit sphere in R
d−1
=
μVol(S
d−2
)
4π
d
2
τ
0
0
1
τ
3
2
e
−
μ
2
4τ
δ
2τ
1
2
0
e
−s
2
s
d−2
ds dτ
=
Vol(S
d−2
)
2π
d
2
∞
μ
2
4τ
0
1
σ
1
2
e
−σ
δσ
1
2
μ
0
e
−s
2
s
d−2
ds dσ.
In this integral we may let μ tend to 0 and obtain as limit
Vol(S
d−2
)
2π
d
2
∞
0
1
σ
1
2
e
−σ
∞
0
e
−s
2
s
d−2
ds dσ =
1
2
. (4.3.11)
By our preceding considerations, this implies (4.3.10).
Equation (4.3.11) is shown with the help of the gamma function
Γ (x)=
∞
0
e
−t
t
x−1
dt for x>0.
We have
Γ (x +1)=xΓ(x) for all x>0,
and because of Γ (1) = 1, then
Γ (n +1)=n!forn ∈ N.
Moreover,
∞
0
s
n
e
−s
2
ds =
1
2
Γ
n +1
2
for all n ∈ N.
In particular,
Γ
1
2
=2
∞
0
e
−s
2
ds =
√
π

102 4. Existence Techniques II: Parabolic Methods. The Heat Equation
and
π
d
2
=
R
d
e
−|x|
2
dx = Vol(S
d−1
)
∞
0
e
−r
2
r
d−1
dr =
1
2
Vol(S
d−1
)Γ
d
2
;
hence
Vol(S
d−1
)=
2π
d
2
Γ
d
2
.
With these formulae, the integral (4.3.11) becomes
2π
d−1
2
Γ
d−1
2
1
2π
d
2
Γ
1
2
·
1
2
Γ
d − 1
2
=
1
2
.
In an analogous manner, one proves the following lemma:
Lemma 4.3.3: Under the assumptions of Lemma 4.3.2, for
w(x, t):=
t
0
∂Ω
K(x, y, τ)γ(y,t − τ) do(y) dτ (4.3.12)
(x ∈ Ω, 0 ≤ t ≤ T ), we have
w ∈ C
∞
(Ω ×[0,T]),
w(x, 0) = 0 for x ∈ Ω. (4.3.13)
The function w extends continuously to
¯
Ω ×[0,T],andforx
0
∈ ∂Ω we have
lim
x→x
0
∇
x
w(x, t) · ν(x
0
)=
γ(x
0
,t)
2
+
t
0
∂Ω
∂K
∂ν
x
0
(x
0
,y,τ)γ(y, t −τ) do(y) dτ.
(4.3.14)
We now want to try first to find a solution of
Δu −
∂
∂t
u =0 inΩ × (0, ∞),
u(x, 0) = 0 for x ∈ Ω,
u(x, t)=g(x, t)forx ∈ ∂Ω, t > 0, (4.3.15)
by Lemma 4.3.2.
We try
u(x, t)=−
t
0
∂Ω
∂K
∂ν
y
(x, y, t − τ)γ(y,τ) do(y) dτ, (4.3.16)
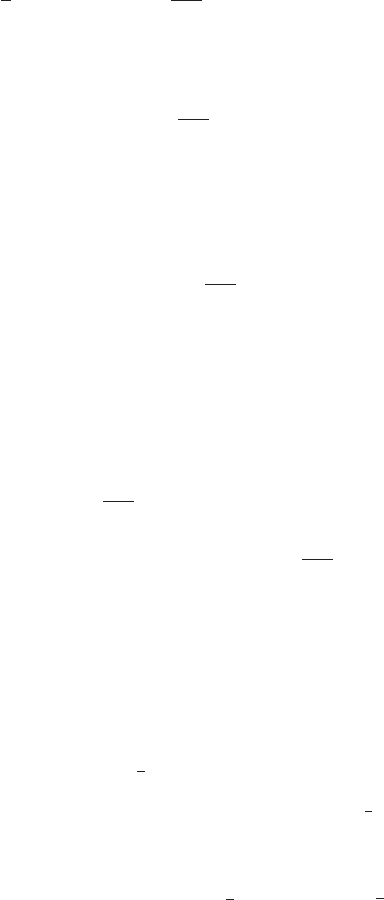
4.3 The Initial Boundary Value Problem for the Heat Equation 103
with a function γ(x, t) yet to be determined. As a consequence of (4.3.7),
(4.3.15), γ has to satisfy, for x
0
∈ ∂Ω,
g(x
0
,t)=
1
2
γ(x
0
,t) −
t
0
∂Ω
∂K
∂ν
y
(x
0
,y,t− τ)γ(y, τ) do(y) dτ,
i.e.,
γ(x
0
,t)=2g(x
0
,t)+2
t
0
∂Ω
∂K
∂ν
y
(x
0
,y,t− τ)γ(y, τ) do(y) dτ. (4.3.17)
This is a fixed-point equation for γ, and one may attempt to solve it by
iteration; i.e., for x
0
∈ ∂Ω,
γ
0
(x
0
,t)=2g(x
0
,t),
γ
n
(x
0
,t)=2g(x
0
,t)+2
t
0
∂Ω
∂K
∂ν
y
(x
0
,y,t− τ)γ
n−1
(y, τ) do(y)dτ
for n ∈ N. Recursively, we obtain
γ
n
(x
0
,t)=2g(x
0
,t)+2
t
0
∂Ω
n
ν=1
S
ν
(x
0
,y,t− τ)g(y, τ) do(y)dτ (4.3.18)
with
S
1
(x
0
,y,t)=2
∂K
∂ν
y
(x
0
,y,t),
S
ν+1
(x
0
,y,t)=2
t
0
∂Ω
S
ν
(x
0
,z,t−τ)
∂K
∂ν
y
(z,y, τ) do(z) dτ.
In order to show that this iteration indeed yields a solution, we have to
verify that the series
S(x
0
,y,t)=
∞
ν=1
S
ν
(x
0
,y,t)
converges.
Choosing once more α =
3
4
in Lemma 4.3.1, we obtain
|S
1
(x
0
,y,t)|≤ct
−3/4
|x
0
− y|
−(d−1)+
1
2
.
Iteratively, we get
|S
n
(x
0
,y,t)|≤c
n
t
−1+
n
4
|x
0
− y|
−(d−1)+
n
2
.
We now choose n = max(4, 2(d − 1)) so that both exponents are positive. If
now
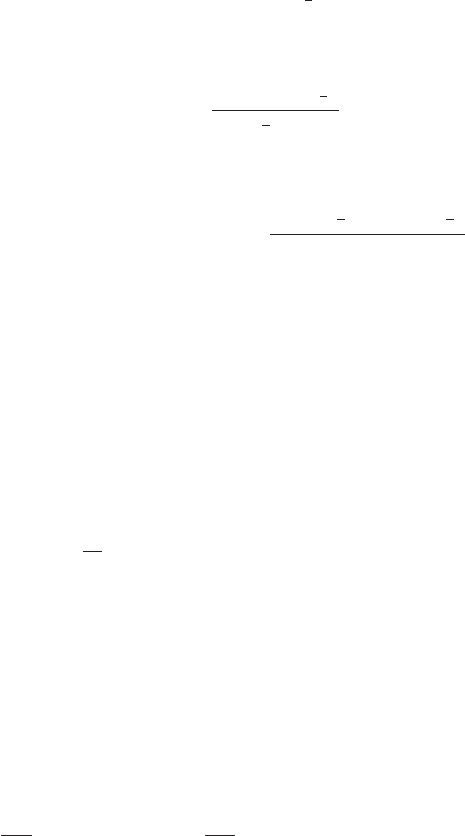
104 4. Existence Techniques II: Parabolic Methods. The Heat Equation
|S
m
(x
0
,y,t)|≤β
m
t
α
for some constant β
m
and some α ≥ 0,
then
|S
m+1
(x
0
,y,t)|≤cβ
0
β
m
t
0
(t − τ)
α
τ
−3/4
dτ,
where the constant c comes from Lemma 4.3.1 and
β
0
:= sup
y∈∂Ω
∂Ω
|z − y|
−(d−1)+
1
2
do(z).
Furthermore,
t
0
(t − τ)
α
τ
−3/4
dτ =
Γ (1 + α)Γ
1
4
Γ
5
4
+ α
t
α+1/4
,
where on the right-hand side we have the gamma function introduced above.
Thus
|S
n+ν
(x
0
,y,t)|≤β
n
(cβ
0
)
ν
t
α+ν/4
ν
!
μ=1
Γ
α +
3
4
+ μ/4
Γ
1
4
Γ (α +1+μ/4)
.
Since the gamma function grows factorially as a function of its arguments,
this implies that
∞
ν=1
S
ν
(x
0
,y,t)
converges absolutely and uniformly on ∂Ω ×∂Ω ×[0,T] for every T>0. We
thus have the following result:
Theorem 4.3.1: The initial boundary value problem for the heat equation
on a bounded domain Ω ⊂ R
d
of class C
2
, namely,
Δu(x, t) −
∂
∂t
u(x, t)=0 in Ω ×(0, ∞),
u(x, 0) = 0 in Ω,
u(x, t)=g(x, t) for x ∈ ∂Ω, t > 0,
with given continuous g, admits a unique solution. That solution can be rep-
resented as
u(x, t)=−
t
0
∂Ω
Σ(x, y, t − τ)g(y, τ) do(y) dτ, (4.3.19)
where
Σ(x, y, t)=2
∂K
∂ν
y
(x, y, t)+2
t
0
∂Ω
∂K
∂ν
z
(x, z, t − τ )
∞
ν=1
S
ν
(z,y, τ) do(z) dτ.
(4.3.20)

4.3 The Initial Boundary Value Problem for the Heat Equation 105
Proof: Since the series
∞
ν=1
S
ν
converges,
γ(x
0
,t)=2g(x
0
,t)+2
t
0
∂Ω
∞
ν=1
S
ν
(x
0
,y,t− τ)g(y, τ) do(y) dτ
is a solution of (4.3.17). Inserting this into (4.3.16), we obtain (4.3.20). Here,
one should note that
t
−3/4
|y − x|
−(d−1)+
1
2
∞
ν=1
S
ν
(x
0
,y,τ),
and hence also Σ(x, y, t) converges absolutely and uniformly on ∂Ω × ∂Ω ×
[0,T] for every T>0. Thus, we may differentiate term by term under the
integral and show that u solves the heat equation. The boundary values are
assumed by construction, and it is clear that u vanishes at t = 0. Uniqueness
follows from Theorem 4.1.1.
Definition 4.3.1: Let Ω ⊂ R
d
be a domain. A function q(x, y, t) that is
defined for x, y ∈
¯
Ω, t>0, is called the heat kernel of Ω if
(i)
Δ
x
−
∂
∂t
q(x, y, t)=0 for x, y ∈ Ω, t > 0, (4.3.21)
(ii)
q(x, y, t)=0 for x ∈ ∂Ω, (4.3.22)
(iii) and for all continuous f : Ω → R
lim
t→0
Ω
q(x, y, t)f(x)dx = f (y) for all y ∈ Ω. (4.3.23)
Corollary 4.3.1: Any bounded domain Ω ⊂ R
d
of class C
2
has a heat ker-
nel, and this heat kernel is of class C
1
on
¯
Ω with respect to the spatial vari-
ables y. The heat kernel is positive in Ω, for all t>0.
Proof: For each y ∈ Ω, by Theorem 4.3.1 we solve the boundary value prob-
lem for the heat equation with initial values 0 and
g(x, t)=−K(x, y, t).
The solution is called μ(x, y, t), and we put
q(x, y, t):=K(x, y, t)+μ(x, y, t). (4.3.24)
Obviously, q(x, y, t) satisfies (i) und (ii), and since
lim
t→0
μ(x, y, t)=0,

106 4. Existence Techniques II: Parabolic Methods. The Heat Equation
and K(x, y, t) satisfies (iii), then so does q(x, y, t).
Lemma 4.3.3 implies that q canbeextendedto
¯
Ω as a continuously dif-
ferentiable function of the spatial variables.
That q(x, y, t) > 0 for all x, y ∈ Ω, t > 0 follows from the strong maximum
principle (Theorem 4.1.3). Namely,
q(x, y, t)=0 forx ∈ ∂Ω,
q(x, y, t)=0 forx, y, ∈ Ω,x = y,
while (iii) implies
q(x, y, t) > 0if|x − y| and t>0 are sufficiently small.
Thus, q ≥ 0andq = 0, and so by Theorem 4.1.3,
q>0inΩ ×Ω × (0, ∞).
Lemma 4.3.4 (Duhamel principle): For all functions u, v on Ω × [0,T]
with the appropriate regularity conditions, we have
T
0
Ω
v(x, t)(Δu(x, T − t)+u
t
(x, T − t))
− u(x, T − t)(Δv(x, t) − v
t
(x, t))
dx dt
=
T
0
∂Ω
∂u
∂ν
(y, T −t)v(y, t) −
∂v
∂ν
(y, t)u(y,T − t)
do(y) dt
+
Ω
{u(x, 0)v(x, T ) − u(x, T )v(x, 0)} dx. (4.3.25)
Proof: Same as the proof of (4.1.12).
Corollary 4.3.2: If the heat kernel q(z, w,T) of Ω is of class C
1
on
¯
Ω with
respect to the spatial variables, then it is symmetric with respect to z and w,
i.e.,
q(z, w, T)=q(w,z, T) for all z,w ∈ Ω, T > 0. (4.3.26)
Proof: In (4.3.25), we put u(x, t)=q(x, z, t), v(x, t)=q(x, w, t). The double
integrals vanish by properties (i) and (ii) of Definition 4.3.1. Property (iii) of
Definition 4.3.1 then yields v(z, T)=u(w, T), which is the asserted symmetry.
Theorem 4.3.2: Let Ω ⊂ R
d
be a bounded domain of class C
2
with heat
kernel q(x, y, t) according to Corollary 4.3.1, and let
ϕ ∈ C
0
(
¯
Ω ×[0, ∞)),g∈ C
0
(∂Ω × (0, ∞)),f∈ C
0
(Ω).
