Jost J. Partial Differential Equations

Подождите немного. Документ загружается.

2.3 Maximum Principles for Nonlinear Diﬀerential Equations 47

Here, one should think of (2.3.6) as an analogue of the sign condition

c(x) ≤ 0 and the bound for the b

(x) as well as a bound of the right-hand

side f of the equation Lu = f .

Proof: We shall follow a similar strategy as in the proof of Theorem 2.3.1 and

shall reduce the result to the maximum principle from Section 2.1 for linear

equations. Here v is an auxiliary function to be determined, and w := u −v.

We consider the operator

Lw :=



i,j=1

(x)w



i=1

(x)w

with

(x):=



∂F

∂r



x, u(x),Du(x),tD

u(x)



dt, (2.3.8)

while the coeﬃcients b

(x) are deﬁned through the following equation:



i=1

(x)w



i,j=1





∂F

∂r



x, u(x),Du(x),tD

u(x)



−

∂F

∂r



x, u(x),Dv(x),tD

u(x)





dt · v

+ F (x, u(x),Du(x), 0) − F (x, u(x),Dv(x), 0) . (2.3.9)

(That this is indeed possible follows from the mean value theorem and the

assumption F ∈ C

. It actually suﬃces to assume that F is twice continu-

ously diﬀerentiable with respect to the variables r only.) Then L satisﬁes the

assumptions of Theorem 2.1.1. Now

Lw = L(u − v)



i,j=1





∂F

∂r



x, u(x),Du(x),tD

u(x)





+ F (x, u(x),Du(x), 0)

−



i,j=1





∂F

∂r



x, u(x),Dv(x),tD

u(x)





− F (x, u(x),Dv(x), 0)

= F



x, u(x),Du(x),D

u(x)



−

⎛

⎝



i,j=1

(x)v

+ F (x, u(x),Dv(x), 0)

⎞

⎠

(2.3.10)

with

(x)=



∂F

∂r



x, u(x),Dv(x),tD

u(x)



dt (2.3.11)

48 2. The Maximum Principle

(this again comes from the integral of a total derivative with respect to t).

Here by assumption

λ |ξ|

≤



i,j=1

(x)ξ

for all x ∈ Ω, ξ ∈ R

. (2.3.12)

We now look for an appropriate auxiliary function v with

Mv :=



(x)v

+ F (x, u(x),Dv(x), 0) ≤ 0. (2.3.13)

We now suppose that for δ := diam(Ω), Ω iscontainedinthestrip{0 <

<δ}.Wenowtry

v(x)=max

∂Ω



(μ

+1)δ

− e

(μ

+1)x



(2.3.14)

(x)=max(0,u(x))).

Then

Mv = −

(μ

+1)

(x)e

(μ

+1)x

+ F (x, u(x),Dv(x), 0)

≤−μ

(μ

+1)

(μ

+1)x

+ μ

(μ

+1)e

(μ

+1)x

+ μ

≤0

by (2.3.6), (2.3.12). This establishes (2.3.13). Equation (2.3.10) then implies,

even under the assumption F [u] ≥ 0inplaceofF [u]=0,

Lw ≥ 0.

By deﬁnition of v,wealsohave

w = u − v ≤ 0on∂Ω.

Theorem 2.1.1 thus implies

u ≤ v in Ω,

and (2.3.7) follows with c = e

(μ

+1) diam(Ω)

− 1. More precisely, under the

assumption F [u] ≥ 0, we have proved the inequality

sup

u ≤ max

∂Ω

+ c

, (2.3.15)

but the inequality in the other direction of course follows analogously, i.e.,

inf

u ≥ min

∂Ω

−

− c

(2.3.16)

−

(x):=min(0,u(x))). 

2.3 Maximum Principles for Nonlinear Diﬀerential Equations 49

Theorem 2.3.2 is of interest even in the linear case. Let us look once more

at the simple equation



(x)+κf(x)=0 forx ∈ (0,π),

f(0) = f(π)=0,

with constant κ. We may apply Theorem 2.3.2 with λ =1,μ

=0,



κ sup

(0,π)

|f| for κ>0,

0forκ ≤ 0.

It follows that

sup

(0,π)

|f|≤cκ sup

(0,π)

|f|;

i.e., if

κ<

we must have f ≡ 0. More generally, in place of κ, one may take any function

c(x)withc(x) ≤ κ on (0,π) and consider f



(x)+c(x)f(x) = 0, without

aﬀecting the preceding conclusion. In particular, this allows us to weaken

the sign condition c(x) ≤ 0. The sharpest possible result here is that f ≡ 0

if κ is smaller than the smallest eigenvalue λ

on (0,π), i.e., 1. This

analogously generalizes to other linear elliptic equations, e.g.,

Δf(x)+κf(x)=0 inΩ,

f(y)=0 on∂Ω.

Theorem 2.3.2 does imply such a result, but not with the optimal bound λ

A reference for the present chapter is Gilbarg–Trudinger [9].

Summary and Perspectives

The maximum principle yields examples of so-called a priori estimates, i.e.,

estimates that hold for any solution of a given diﬀerential equation or class

of equations, depending on the given data (boundary values, right-hand side,

etc.), without the need to know the solution in advance or without even

having to guarantee in advance that a solution exists. Conversely, such a

priori estimates often constitute an important tool in many existence proofs.

Maximum principles are characteristic for solutions of elliptic (and parabolic)

PDEs, and they are not restricted to linear equations. Often, they are even

the most important tool for studying certain nonlinear elliptic PDEs.

50 2. The Maximum Principle

Exercises

2.1 Let Ω

,Ω

⊂ R

be disjoint open sets such that

∩

contains a

smooth hypersurface T , e.g.,

:= {(x

,...,x

):|x| < 1,x

> 0},

:= {(x

,...,x

):|x| < 1,x

< 0},

T = {(x

,...,x

):|x| < 1,x

=0}.

Let u ∈ C

(

∪

) ∩C

(Ω

) ∩C

(Ω

)beharmoniconΩ

and on Ω

i.e.,

Δu(x)=0,x∈ Ω

∪ Ω

Does this imply that u is harmonic on Ω

∪ Ω

∪ T ?

2.2 Let Ω be open in R

= {(x, y)}. For a nonconstant solution u ∈ C

(Ω)

of the diﬀerential equation

=0 inΩ,

is it possible to assume an interior maximum in Ω?

2.3 Let Ω be open and bounded in R

.On

Ω ×[0, ∞) ⊂ R

d+1

= {(x

,...,x

,t)},

we consider the heat equation

= Δu, where Δ =



i=1

∂

(∂x

)

Show that for bounded solutions u ∈ C

(Ω ×(0, ∞)) ∩ C

(

Ω ×[0, ∞)),

sup

Ω×[0,∞)

u ≤ sup

(

Ω×{0})∪(∂Ω×[0,∞))

2.4 Let u : Ω → R be harmonic, Ω



⊂⊂ Ω ⊂ R

. We then have, for all i, j

between 1 and d,

sup



|≤



dist(Ω



,∂Ω)



sup

|u|.

Prove this inequality. Write down and demonstrate an analogous inequal-

ity for derivatives of arbitrary order!

2.5 Let Ω ⊂ R

be open and bounded. Let u ∈ C

(Ω) ∩ C

(

Ω) satisfy

Δu = u

,x∈ Ω,

u ≡ 0,x∈ ∂Ω.

Show that u ≡ 0inΩ.

Exercises 51

2.6 Prove a version of the maximum principle of Alexandrov and Bakelman

for operators

Lu =



i,j=1

(x)u

(x),

assuming in place of ellipticity only that det(a

(x)) is positive in Ω.

2.7 Control the maximum and minimum of the solution u of an elliptic

Monge–Amp`ere equation

det(u

(x)) = f(x)

in a bounded domain Ω.

2.8 Let u ∈ C

(Ω) be a solution of the Monge–Amp`ere equation

det(u

(x)) = f(x)

in the domain Ω with positive f. Suppose there exists x

∈ Ω where the

Hessian of u is positive deﬁnite. Show that the equation then is elliptic

at u in all of Ω.

2.9 Let R

:= {(x

)},Ω :=

B(0,R

) \ B(0,R

)withR

> 0. The

function φ(x

):=a + b log(|x|)isharmonicinΩ for all a, b.Let

u ∈ C

(Ω) ∩ C

(

Ω) be subharmonic, i.e.,

Δu ≥ 0,x∈ Ω.

Show that

M(r) ≤

M(R

) log(

)+M(R

) log(

)

log(

)

with

M(r):= max

∂B(0,r)

u(x)

and R

≤ r ≤ R

2.10 Let

+ y

−

+ y

Show that u

and u

solve the Monge–Amp`ere equation

− u

and

= u

=1 on∂B(0, 1).

Is this compatible with the uniqueness result for the Dirichlet problem

for nonlinear elliptic PDEs?

52 2. The Maximum Principle

2.11 Let Ω

:= Ω × (0,T), and suppose u ∈ C

(Ω

) ∩ C

(

) satisﬁes

= Δu + u

in Ω

u(x, t) >c>0 for (x, t) ∈ (Ω ×{0}) ∪ (∂Ω × [0,T)).

Show that

(a) u>cfor all (x, t) ∈

(b) If in addition u(x, t)=u(x, 0) for all x ∈ ∂Ω and all t,thenT<∞.

3. Existence Techniques I: Methods Based on

the Maximum Principle

3.1 Diﬀerence Methods: Discretization of

Diﬀerential Equations

The basic idea of the diﬀerence methods consists in replacing the given dif-

ferential equation by a diﬀerence equation with step size h and trying to

show that for h → 0, the solutions of the diﬀerence equations converge to a

solution of the diﬀerential equation. This is a constructive method that in

particular is often applied for the numerical (approximative) computation of

solutions of diﬀerential equations. In order to show the essential aspects of

this method in a setting that is as simple as possible, we consider only the

Laplace equation

Δu = 0 (3.1.1)

in a bounded domain in Ω in R

.WecoverR

with an orthogonal grid of

mesh size h>0; i.e., we consider the points or vertices



,...,x



=(n

h,...,n

h) (3.1.2)

with n

,...,n

∈ Z. The set of these vertices is called R

, and we put

:= Ω ∩ R

. (3.1.3)

We say that x =(n

h,...,n

h)andy =(m

h,...,m

h) (all n

∈ Z)are

neighbors if



i=1

− m

| =1, (3.1.4)

or equivalently,

|x − y| = h. (3.1.5)

The straight lines between neighboring vertices are called edges. A connected

union of edges for which every vertex is contained in at most two edges is

called an edge path (see Figure 3.1).

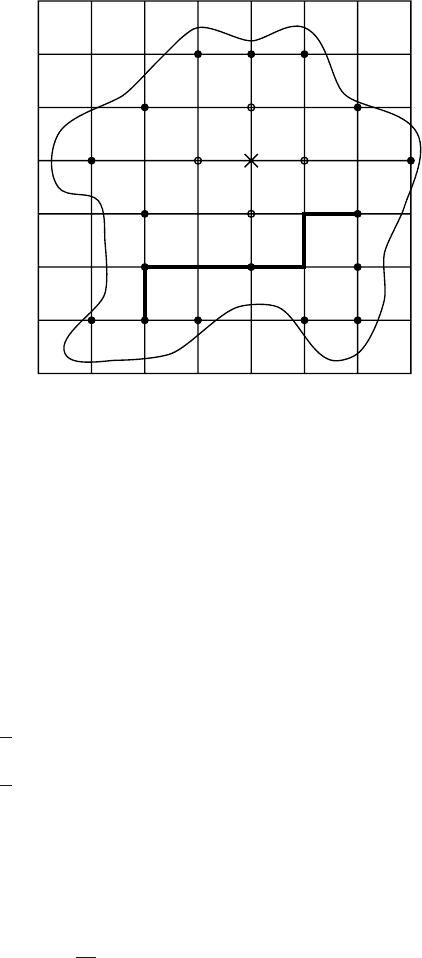
54 3. Existence Techniques I: Methods Based on the Maximum Principle

Figure 3.1. x (cross) and its neighbors (open dots) and an edge path in

(heavy

line) and vertices from Γ

(solid dots).

The boundary vertices of

are those vertices of

for which not all

their neighbors are contained in

.LetΓ

be the set of boundary vertices.

Vertices in

that are not boundary vertices are called interior vertices. The

set of interior vertices is called Ω

We suppose that Ω

is discretely connected, meaning that any two vertices

in Ω

can be connected by an edge path in Ω

. We consider a function

u :

→ R

and put, for i =1,...,d, x =(x

,...,x

) ∈ Ω

(x):=



u(x

,...,x

i−1

+ h, x

i+1

,...,x

) − u(x

,...,x

)



¯ı

(x):=



u(x

,...,x

) − u(x

,...,x

i−1

− h, x

i+1

,...,x

)



. (3.1.6)

Thus, u

and u

¯ı

are the forward and backward diﬀerence quotients in the ith

coordinate direction. Analogously, we deﬁne higher-order diﬀerence quotients,

e.g.,

i¯ı

(x)=u

¯ıi

(x)= (u

¯ı

)

(x)



u(x

,...,x

+ h,...,x

) − 2u(x

,...,x

)

+ u(x

,...,x

− h,...,x

)



. (3.1.7)

If we wish to emphasize the dependence on the mesh size h,wewrite

¯ıi

in place of u, u

i¯ı

, etc.

3.1 Diﬀerence Methods: Discretization of Diﬀerential Equations 55

The main reason for considering diﬀerence quotients, of course, is that for

functions that are diﬀerentiable up to the appropriate order, for h → 0, the

diﬀerence quotients converge to the corresponding derivatives. For example,

for u ∈ C

(Ω),

lim

h→0

i¯ı

∂

(∂x

)

u(x), (3.1.8)

if x

∈ Ω

tends to x ∈ Ω for h → 0. Consequently, we approximate the

Laplace equation

Δu =0 inΩ

by the diﬀerence equation



i=1

i¯ı

=0 inΩ

, (3.1.9)

and we call this equation the discrete Laplace equation. Our aim now is to

solve the Dirichlet problem for the discrete Laplace equation

=0 inΩ

= g

on Γ

, (3.1.10)

and to show that under appropriate assumptions, the solutions u

converge

for h → 0 to a solution of the Dirichlet problem

Δu =0 inΩ,

u = g on ∂Ω, (3.1.11)

where g

is a discrete approximation of g. Considering the values of u

at the

vertices of Ω

as unknowns, (3.1.10) leads to a linear system with the same

number of equations as unknowns. Those equations that come from vertices

all of whose neighbors are interior vertices themselves are homogeneous, while

the others are inhomogeneous.

It is a remarkable and useful fact that many properties of the Laplace

equation continue to hold for the discrete Laplace equation. We start with

the discrete maximum principle:

Theorem 3.1.1: Suppose

≥ 0 in Ω

where Ω

, as always, is supposed to be discretely connected. Then

max

=max

. (3.1.12)

If the maximum is assumed at an interior point, then u

has to be constant.

56 3. Existence Techniques I: Methods Based on the Maximum Principle

Proof: Let x

be an interior vertex, and let x

,...,x

be its neighbors. Then

(x)=





α=1

) − 2du

)



. (3.1.13)

If Δ

(x) ≥ 0, then

) ≤



α=1

), (3.1.14)

i.e., u

) is not bigger than the arithmetic mean of the values of u

at the

neighbors of x

. This implies

) ≤ max

α=1,...,2d

), (3.1.15)

with equality only if

)=u

) for all α ∈{1,...,2d}. (3.1.16)

Thus, if u assumes an interior maximum at a vertex x

,itdoessoatall

neighbors of x

as well, and repeating this reasoning, then also at all neighbors

of neighbors, etc. Since Ω

is discretely connected by assumption, u

has to

be constant in

. This is the strong maximum principle, which in turn

implies the weak maximum principle (3.1.12). 

Corollary 3.1.1: The discrete Dirichlet problem

=0 in Ω

= g

on Γ

for given g

has at most one solution.

Proof: This follows in the usual manner by applying the maximum principle

to the diﬀerence of two solutions. 

It is remarkable that in the discrete case this uniqueness result already

implies an existence result:

Corollary 3.1.2: The discrete Dirichlet problem

=0 in Ω

= g

on Γ

admits a unique solution for each g

: Γ

→ R.