James I.N. Introduction to Circulating Atmospheres

Подождите немного. Документ загружается.

2.6 Problems

The SGCM makes

attempt

simulate

the

stratosphere.

In the

GCM,

the temperature field,

and

hence,

thermal wind balance,

the

zonal wind

field in

the

lower stratosphere,

are

sensitive

to the

details

of the

radiation

scheme and the deep convection scheme. But

is clear from the diagram that

the SGCM captures many

the basic processes which determine

the

gross

features

of the

zonal wind field. Later,

shall

see

that

also simulates

various eddy fluxes

heat

and

momentum surprisingly realistically.

2.6 Problems

2.1 Show that

(

QR-QR.

2.2

some conserved quantity such that DQ/Dt

0, and Q

inde-

pendent

height, show that the root mean square meridional displacement

of air parcels

2.3 Show that

finite difference analogue

the second derivative can

written:

-2g

e:,

1+ 2

Using this formula and centred time differencing, show that the finite differ-

ence analogue

the linear diffusion equation:

is numerically unstable

for all At.

Suggest

modification

this finite

difference representation which would

stable.

Observing and modelling global circulations

LATITUDE

i r- i i ; i i

-60S

-30S 0 30N 60N 90N

LATITUDE

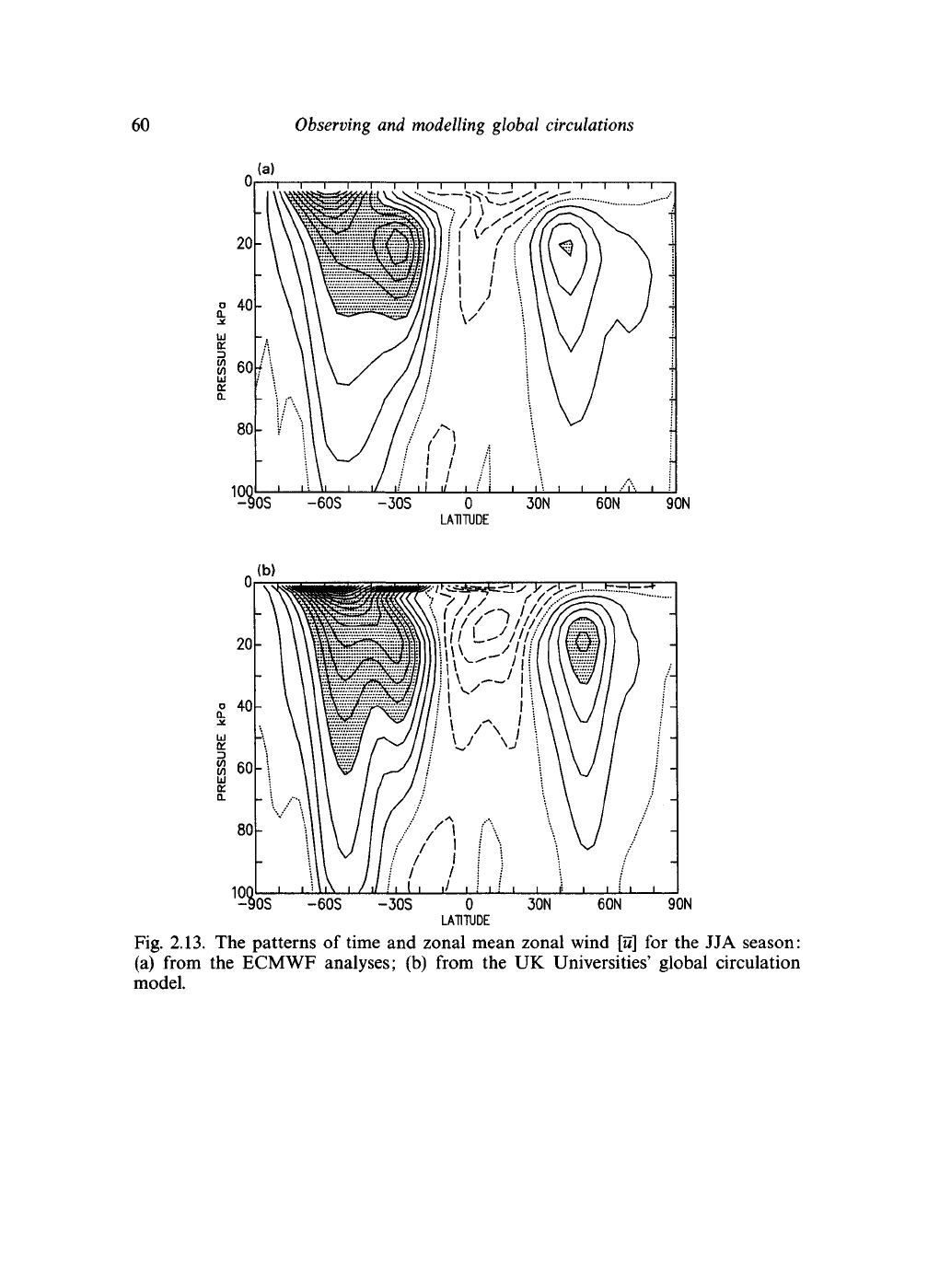
Fig. 2.13. The patterns of time and zonal mean zonal wind

[u]

for the JJA season:

(a) from the ECMWF analyses; (b) from the UK Universities' global circulation

model.

2.6 Problems

LATITUDE

Fig. 2.13

(cont.).

Contour interval

ms"

, with negative contours dashed. Shading indicates values

in excess of

ms"

The atmospheric heat engine

3.1 Global energy balance

In this section, we will discuss some basic principles which will help to

describe the thermal forcing of the global circulation. For the present, we

consider the global mean energy balance of the atmosphere; in the next

section we will consider the geographical variations of this balance. In these

sections, we wish to introduce the concept of radiative equilibrium, and

to indicate how a timescale for establishing such an equilibrium might be

estimated.

The basic physical principle to be used is Stefan's law. This states that the

radiant energy emitted per unit area of a perfectly black body is proportional

to the fourth power of its temperature:

S =

GT\ (3.1)

where a is the Stefan-Boltzman constant and has the value 5.67 x

10~

Wm~

. For our purposes, it will be adequate to regard a slab of

gas,

the surface of the Earth or the surface of the sun as black bodies which

obey Stefan's law.

A black body emits radiation with a range of frequencies, but with a

maximum at frequency v

max

. Wien's displacement law relates v

max

to the

temperature of the black body:

Vmax = WT, (3.2)

where W is a constant which has the value 1.035 x 10

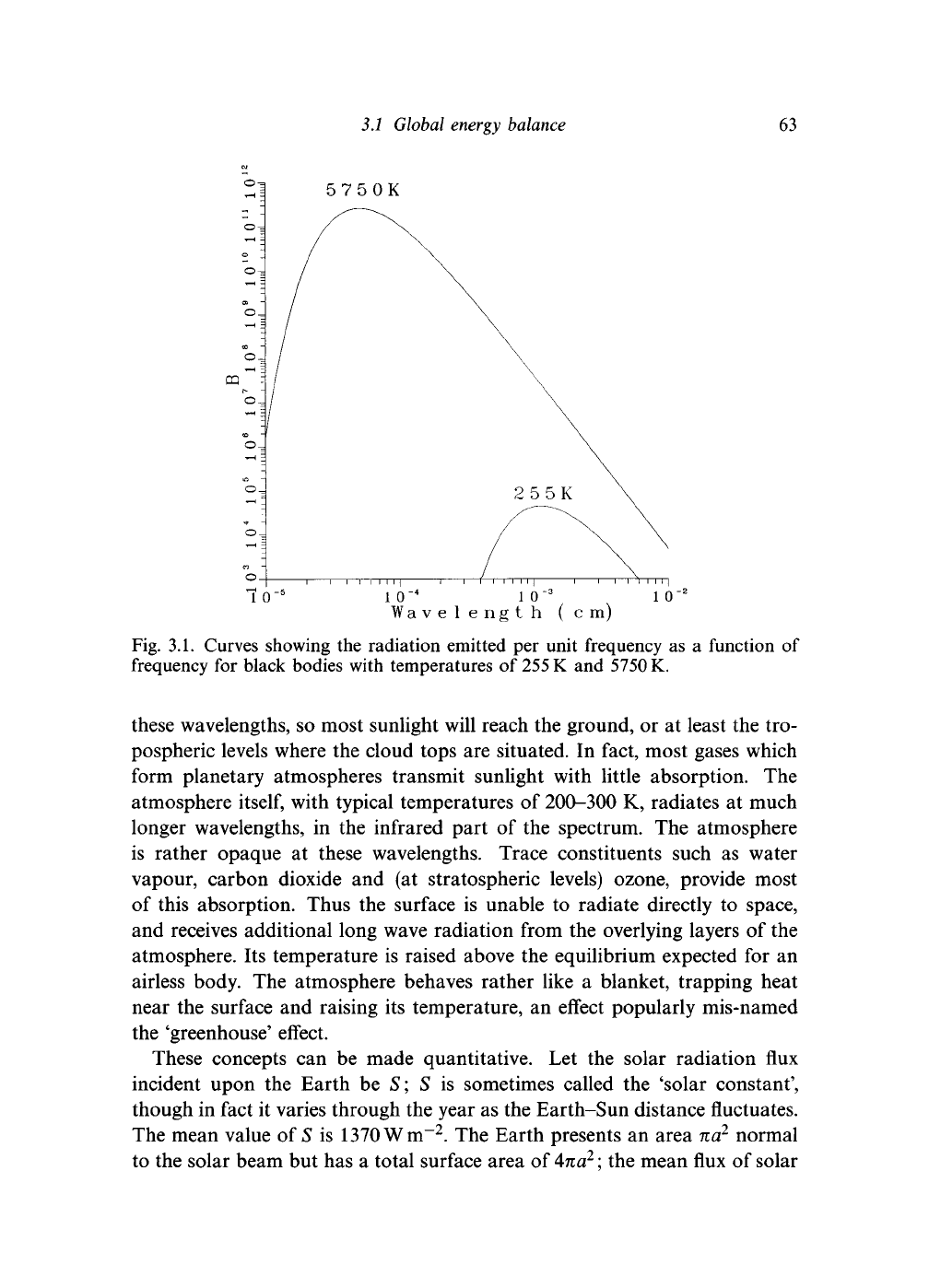
. Figure 3.1

shows graphs of energy versus frequency for black bodies at different tem-

peratures. The Sun, with a surface temperature of around 5750 K, radiates

mainly at visible and near infrared wavelengths, with a maximum in the

visible part of the spectrum. The clear atmosphere is nearly transparent to

3.1 Global energy balance

5750K

1111

1—i—f—i—i

i 111

1—i—i—i

i 1111

10"

Wavelength (cm)

Fig. 3.1. Curves showing the radiation emitted per unit frequency as a function of

frequency for black bodies with temperatures of 255 K and 5750 K.

these wavelengths, so most sunlight will reach the ground, or at least the tro-

pospheric levels where the cloud tops are situated. In fact, most gases which

form planetary atmospheres transmit sunlight with little absorption. The

atmosphere

itself,

with typical temperatures of 200-300 K, radiates at much

longer wavelengths, in the infrared part of the spectrum. The atmosphere

is rather opaque at these wavelengths. Trace constituents such as water

vapour, carbon dioxide and (at stratospheric levels) ozone, provide most

of this absorption. Thus the surface is unable to radiate directly to space,

and receives additional long wave radiation from the overlying layers of the

atmosphere. Its temperature is raised above the equilibrium expected for an

airless body. The atmosphere behaves rather like a blanket, trapping heat

near the surface and raising its temperature, an effect popularly mis-named

the 'greenhouse' effect.

These concepts can be made quantitative. Let the solar radiation flux

incident upon the Earth be S; S is sometimes called the 'solar constant',

though in fact it varies through the year as the Earth-Sun distance fluctuates.

The mean value of S is

1370

Wm~

The Earth presents an area na

normal

to the solar beam but has a total surface area of

Ana

;

the mean flux of solar

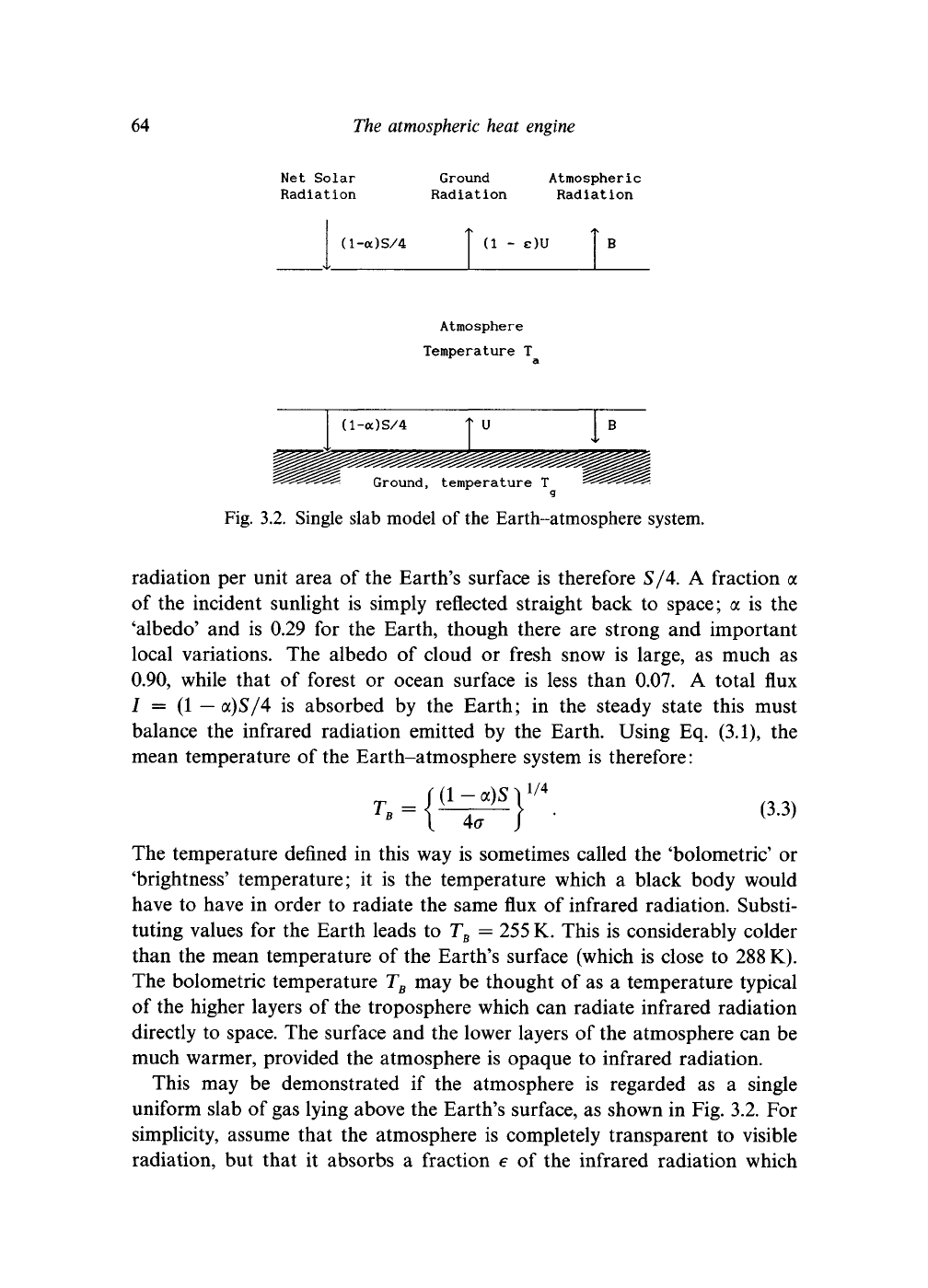
The atmospheric heat engine

Net Solar

Radiation

(l-a)S/4

Ground

Radiation

I"-

Atmosphere

Temperature

Atmospheric

Radiation

e)U

T B

Ground, temperature

Fig. 3.2. Single slab model

the Earth-atmosphere system.

radiation

per

unit area

the Earth's surface

therefore

S/4.

fraction

of the incident sunlight

simply reflected straight back

space;

a is

the

'albedo'

and

0.29

for

the

Earth, though there

are

strong

and

important

local variations.

The

albedo

cloud

fresh snow

large,

much

0.90, while that

forest

ocean surface

less than 0.07.

total flux

— cc)S/4

absorbed

by the

Earth;

in the

steady state this must

balance

the

infrared radiation emitted

the

Earth. Using

Eq.

(3.1),

the

mean temperature

the Earth-atmosphere system

therefore:

-{••

-«)sy

4(7

(3.3)

The temperature defined

this way

sometimes called

the

'bolometric'

'brightness' temperature;

it is

the

temperature which

black body would

have

order

radiate

the

same flux

infrared radiation. Substi-

tuting values

for the

Earth leads

to T

255

K. This

considerably colder

than

the

mean temperature

the Earth's surface (which

288

K).

The bolometric temperature

may

thought

temperature typical

of the higher layers

the troposphere which

can

radiate infrared radiation

directly

space. The surface

and

the lower layers

the atmosphere can

much warmer, provided

the

atmosphere

opaque

infrared radiation.

This

may

demonstrated

the

atmosphere

regarded

as a

single

uniform slab

gas lying above the Earth's surface,

shown

Fig. 3.2.

For

simplicity, assume that

the

atmosphere

completely transparent

visible

radiation,

but

that

absorbs

fraction

6 of

the

infrared radiation which

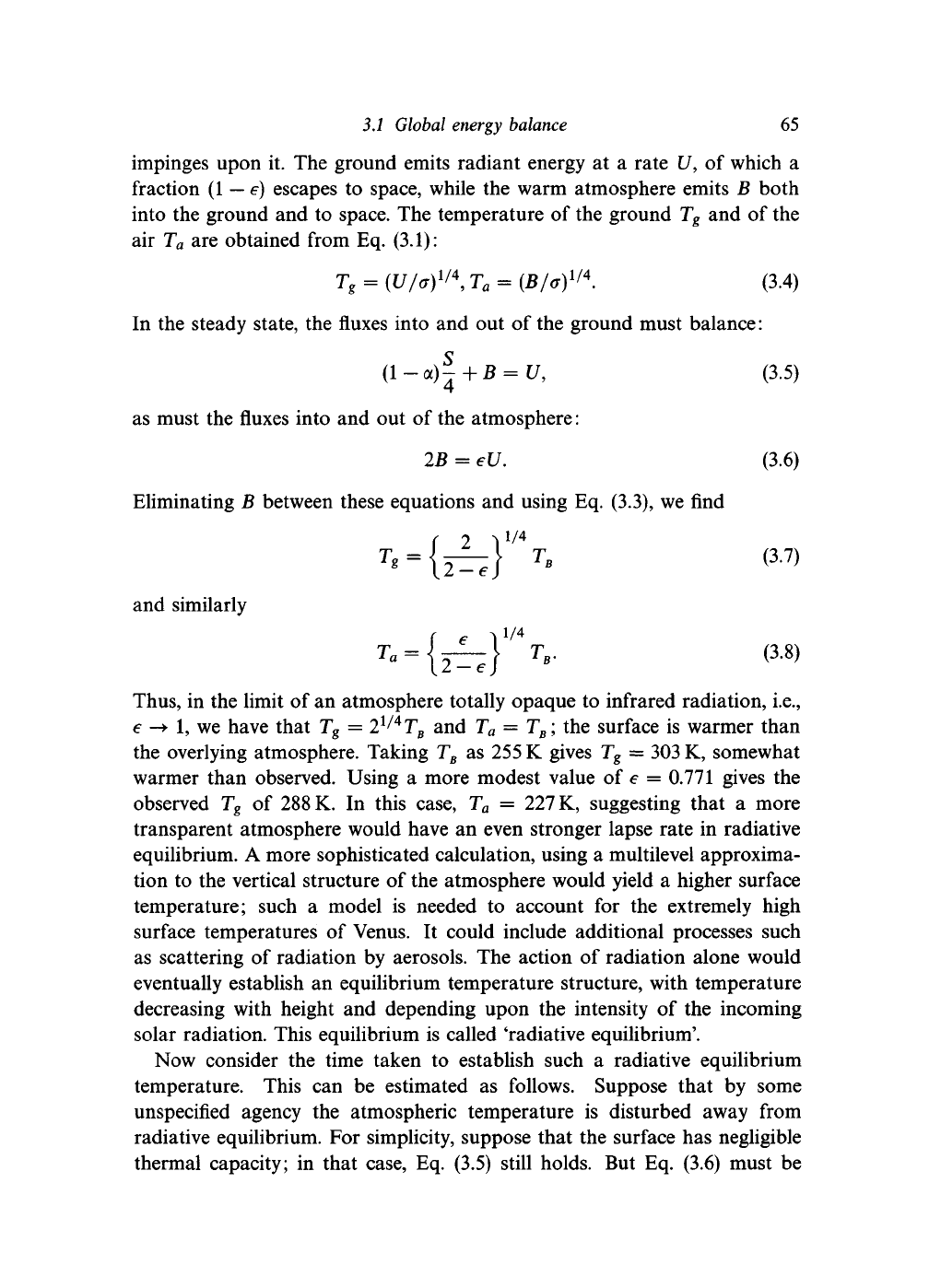
3.1

Global energy balance

impinges upon it. The ground emits radiant energy at a rate U, of which a

fraction (1

—

e) escapes to space, while the warm atmosphere emits B both

into the ground and to space. The temperature of the ground T

and of the

air T

are obtained from Eq. (3.1):

'\ (3.4)

In the steady state, the fluxes into and out of the ground must balance:

(l-a)|

+ B = l/, (3.5)

as must the fluxes into and out of the atmosphere:

IB = eU. (3.6)

Eliminating B between these equations and using Eq. (3.3), we find

* = \2=7}

and similarly

Thus,

in the limit of an atmosphere totally opaque to infrared radiation, i.e.,

—>

1, we have that T

= 2

and T

= T

; the surface is warmer than

the overlying atmosphere. Taking T

as 255 K gives T

= 303 K, somewhat

warmer than observed. Using a more modest value of e = 0.771 gives the

observed T

of 288 K. In this case, T

= 227 K, suggesting that a more

transparent atmosphere would have an even stronger lapse rate in radiative

equilibrium. A more sophisticated calculation, using a multilevel approxima-

tion to the vertical structure of the atmosphere would yield a higher surface

temperature; such a model is needed to account for the extremely high

surface temperatures of Venus. It could include additional processes such

as scattering of radiation by aerosols. The action of radiation alone would

eventually establish an equilibrium temperature structure, with temperature

decreasing with height and depending upon the intensity of the incoming

solar radiation. This equilibrium is called 'radiative equilibrium'.

Now consider the time taken to establish such a radiative equilibrium

temperature. This can be estimated as follows. Suppose that by some

unspecified agency the atmospheric temperature is disturbed away from

radiative equilibrium. For simplicity, suppose that the surface has negligible

thermal capacity; in that case, Eq. (3.5) still holds. But Eq. (3.6) must be

The

atmospheric

heat

engine

rewritten

J*i^=eU-2B,

(3.9)

where p

is the surface pressure; c

/g is the thermal capacity of an

atmospheric column of unit cross sectional area. Write T

o+AT,

where

o is the equilibrium value calculated from Eq. (3.7), and | AT |<C

Then

Eq. (3.9) can be linearized about T

o'.

_4(2

)aTi

)gAT

d£

This

equation has a simple and well-known solution: AT decays exponen-

tially

on a timescale T

where

which is called the 'radiative equilibrium timescale'. If the radiative timescale

is long compared to the typical timescale for motions in the atmosphere, then

fluid motions can maintain the atmospheric temperature far from radiative

equilibrium; on the other hand, if

is short, meteorology will do little to

disturb radiative equilibrium. For the Earth, we suppose that e is 1 so that

255

K; then

from Eq. (3.11) is around 30 days. This is much longer

than the typical timescales of 1-5 days associated with advection by large

scale tropospheric weather systems, and so we anticipate that the circulation

of the atmosphere will greatly modify its thermal structure.

The kind of model which has been described in this section is some-

times called an 'energy balance' climate model. Such models use the global

mean incoming and outgoing fluxes of energy to estimate the global mean

temperatures. More sophisticated versions of the genre include complicated

feedback effects, such as ice-albedo feedbacks. Of course, they give no

guidance as to global circulation, which is driven by variations of the tem-

perature fields from place to place in the atmosphere. Indeed, because of

the complicated nonlinearities within the climate system, such simple energy

balance models do not even give a very reliable guide to the global mean

climate, though they have been used to yield interesting possibilities about

the sensitivity of climate to various kinds of perturbation.

3.2 Local radiative balance

The arguments of the preceding section were global. But the large scale

circulation of the atmosphere is driven by variations of temperature from

3.2

Local radiative balance

place to place. So in this section, the geographical variations of the radiation

field will be described. These geographical variations will also change with

time,

thus inducing diurnal and seasonal changes into the atmospheric

circulation.

Both the incoming solar radiation and the albedo of the Earth vary

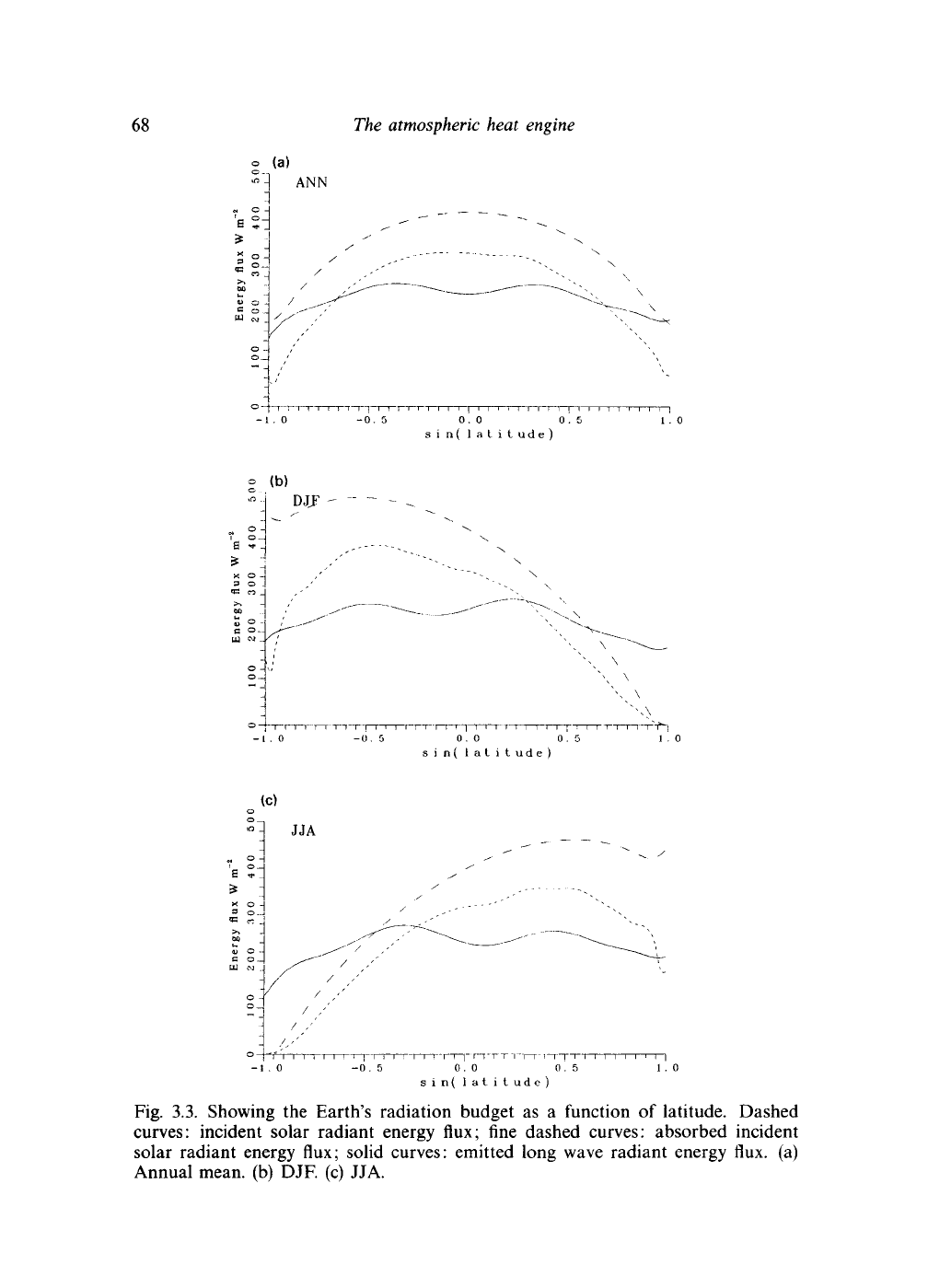
strongly with latitude. Figure 3.3(a) shows the annual mean incoming solar

radiant energy flux plotted against latitude. We denote this incoming flux

of radiation /. The global mean value of / is of course S/4, as shown

in the last section. Geometrical factors mean that / is large in the tropics

but considerably smaller at the poles. Were it not for the axial tilt of the

Earth, the polar value would of course be zero. As it is, there is substantial

insolation at the poles in the summer. In fact, the local value of / is a

maximum at the pole, for a time around the summer solstice. The equator

to pole decline of the annual mean / is accentuated by the variations of

albedo. The albedo of high latitudes, where the snow and ice cover is

large and permanent, is much higher than at lower latitudes. Again, the

midlatitudes are more cloudy than the subtropics. As a result, a nearly

constant short wave flux of about

100

Wm~

is reflected directly back to

space at all latitudes. Thus the radiation absorbed by the Earth and its

atmosphere varies from around

100

Wm~

at the poles to near

400

Wm~

at the equator.

The local radiative equilibrium temperature can be calculated in the same

way as can the global radiative equilibrium temperature, using Stefan's law.

The local bolometric temperature is given by:

(SJo)

(3.12)

where S

denotes the local absorbed solar radiation. At the pole, the annual

mean value of T

would be around 205 K from this formula, while at the

equator we find 290 K. Using Eq. (3.7) to estimate a surface temperature,

assuming e =

0.771,

the equator-pole temperature difference would be about

In fact, this temperature difference is much larger than observed. The

annual average temperature difference is around 35 K, with the poles con-

siderably warmer than would be predicted from local radiative balance, and

the tropics rather cooler. The third curve in Fig. 3.3(a) shows the outgoing

long wave radiation plotted against latitude. It is a considerably flatter curve

than that for S

, reflecting that the temperature contrast is smaller than it

would be in radiative equilibrium. Of course, the total outgoing radiation,

integrated over the globe balances the total incoming radiation. But locally,

the imbalances are substantial. These local imbalances are a manifestation

The atmospheric heat engine

(a)

ANN

—(-—

->—

—

-1.0

-0.5

0.0 0.

si n( latitude)

(b)

DJF -

1*1

JJA

;

-0.5 0.0

sin( latitude)

\ \

i—i—r~i—i—|~T~7"T'T"

-\—\—r""i~

0.0

sin(latitude)

Fig. 3.3. Showing the Earth's radiation budget as a function of latitude. Dashed

curves: incident solar radiant energy flux; fine dashed curves: absorbed incident

solar radiant energy flux; solid curves: emitted long wave radiant energy flux, (a)

Annual mean, (b) DJF. (c) JJA.