James I.N. Introduction to Circulating Atmospheres

Подождите немного. Документ загружается.

10.6

Laboratory systems

379

constant, 2Q. The thermal wind relationship for the zonal flow in the system

where the horizontal gradient of density is related to the horizontal temperat-

ure gradient by Eq. (10.29). Denoting the temperature difference across the

system by AT, the thermal wind relationship can be integrated to estimate

the zonal wind shear AC/ between the top and bottom of the annulus:

Thus,

the zonal flow will increase with height when the outer wall is warmer

than the inner. This zonal flow can be thought of as the result of Coriolis

forces deflecting meridional overturning motions driven by the temperature

gradients.

Such a vertically sheared flow will be baroclinically unstable. Assuming

that the curvature of the system is not too strong, the Eady model of

Section 5.4 can be applied directly to the system. The Eady model is

characterized by a minimum wavelength for which there is instability, that

is,

X> 1.31^. (10.32)

The Brunt-Vaisala frequency N is estimated by assuming that the temperat-

ure difference between the top and bottom of the system is comparable to

the imposed horizontal temperature difference. That is, warm fluid rises

against the warm wall and spreads across the top of the annulus, while cold

fluid sinks against the cold wall and spreads across the base. Hence

_g_^

gaAT

The longest wave which can be accommodated by the apparatus has wave-

length n(b + a), and so the condition for baroclinically unstable waves to be

present can be written

At slow rotation rates, for a given apparatus and AT, instability will not

be possible, and the flow will be purely axisymmetric. At rapid rotation

rates,

condition (10.34) can be satisfied and unstable baroclinic waves will be

expected to develop. The precise form of the flow will depend upon nonlinear

effects as the unstable waves reach finite amplitude. Condition (10.34) can

380 Planetary atmospheres and other fluid systems

be rewritten in terms of a Rossby number based on AU (Eq. (10.31)) and

the gap width, (b

—

a).

This is simply the thermal Rossby number Ro

(Eq. (10.20)) with the gap width

(b — a)

replacing the planetary radius:

The appearance of waves requires that the thermal Rossby number be less

than some critical value.

Figure 10.16 shows the flow for a given annulus with fixed AT but

increasing Q. At the lowest rotation rates, the flow is axisymmetric, in

agreement with Eq. (10.35). At larger rotation rates, the axisymmetric flow

is unstable and waves appear. As long as the wavelength of the most

unstable disturbance is long compared to (b

—

a), a 'regular regime' is

observed, in which the wave equilibrates at a finite amplitude. The zonal

flow is concentrated into a tight jet stream which meanders between the

inner and outer walls of the apparatus, and the entire pattern drifts around

the apparatus. The jet stream transports heat across the apparatus, even

though the motion is quasi-horizontal and geostrophic, so that

[v]

tends to

zero as Q increases. The narrow, meandering jet stream is rather similar to

the midlatitude jet observed in the Earth's troposphere. This regular flow,

characterized by a single dominant wavenumber with a constant drift speed,

is highly predictable. The Martian midlatitude flow is apparently somewhat

similar. At the largest rotation rates accessible to the apparatus, the most

unstable wavelength becomes smaller than

(b —

a).

Then the waves become

irregular both in space and time. The irregular flow resembles that of the

Earth's atmosphere in that it is inherently chaotic and unpredictable.

The transition from axisymmetric flow to regular waves depends upon

factors other than just Ro

. Viscosity and thermal diffusion can stabilize

baroclinic waves, and so affect the value of Ro

at which the transition

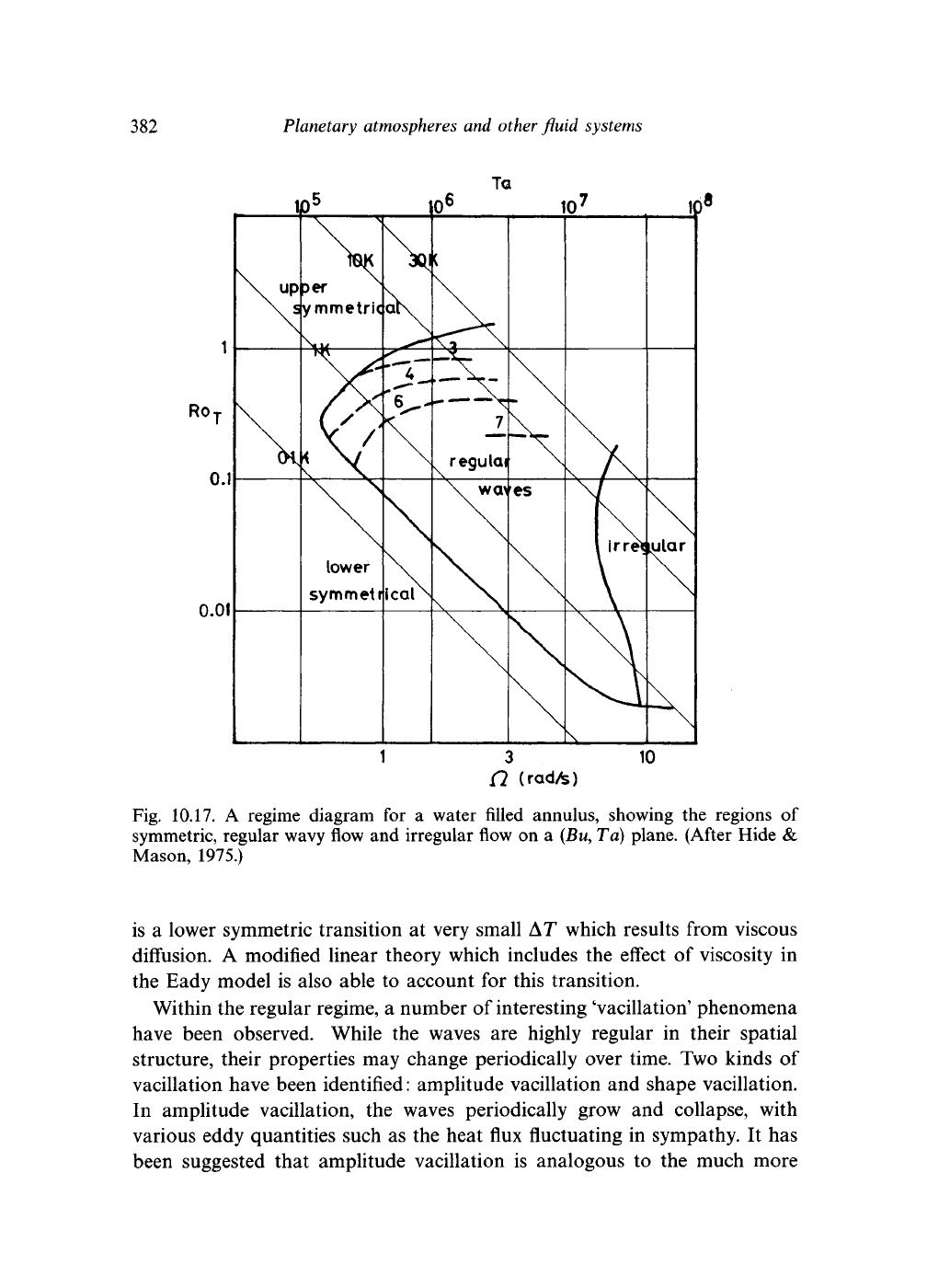
takes place. Figure 10.17 shows a regime diagram, in which the wavenumber

and character of the flow has been plotted against Ro

, and a dimensionless

measure of the viscosity, the Taylor number Ta. The Taylor number is

defined as

and is closely related to the Ekman number by Ta oc Ek~

. Inviscid theory,

as outlined above, suggests that the transition between axisymmetric and

wavy flow should simply be a horizontal line; this is indeed so at large Ta.

In contrast, at sufficiently large viscosity, waves are absent. At intermediate

viscosity, as well as the upper symmetric transition of inviscid theory, there

10.6 Laboratory systems

381

Fig. 10.16. Flow just beneath the upper surface in an annulus system at different

rotation rates. The flow was visualized by time exposure photographs of neutrally

buoyant beads. The working fluid was a water-glycerol solution with a viscosity of

1.56 x 10~

, the temperature difference was 9K, and the dimensions of the

annulus were a = 55mm, b = 101mm, d = 135mm. The rotation rates were: (a)

0.41 rads"

; (b) 1.07 rads"

; (c) 1.21 rads"

; (d) 3.22 rads"

; (e)

3.91

rads"

; and

(f)

6.4

rads"

(From Hide & Mason, 1975.)

382

Planetary atmospheres and other fluid systems

3 10

Cl (rad/s)

Fig. 10.17. A regime diagram for a water filled annulus, showing the regions of

symmetric, regular wavy flow and irregular flow on a (Bu, Ta) plane. (After Hide &

Mason, 1975.)

is a lower symmetric transition at very small AT which results from viscous

diffusion. A modified linear theory which includes the effect of viscosity in

the Eady model is also able to account for this transition.

Within the regular regime, a number of interesting 'vacillation' phenomena

have been observed. While the waves are highly regular in their spatial

structure, their properties may change periodically over time. Two kinds of

vacillation have been identified: amplitude vacillation and shape vacillation.

In amplitude vacillation, the waves periodically grow and collapse, with

various eddy quantities such as the heat flux fluctuating in sympathy. It has

been suggested that amplitude vacillation is analogous to the much more

10.6 Laboratory systems 383

irregular index cycles observed in the Earth's midlatitudes. Shape vacillation

is characterized by a more constant amplitude and hence heat flux; instead

the direction of the trough line fluctuates, leading to a large fluctuation in

the momentum flux carried by the eddies.

One of the most interesting aspects of the annulus system is that it

suggests several ways in which nonlinearity can be manifested in a baroclinic

system. All the wavy flows we have discussed have finite amplitude waves

whose mean intensity has equilibrated to the zonal flow; the linear processes

generating eddy kinetic energy balance the nonlinear cascade of kinetic

energy down to smaller scales where diffusive processes can remove it. This

nonlinear limiting may lead steady, regular waves, to vacillating waves with

periodic large amplitude, low frequency fluctuations of the flow, or to highly

irregular, 'chaotic' flows of the kind discussed in Section 8.7.

We have to be careful not to stretch the analogy between the annulus

system and planetary atmospheres too far. One difference is the absence of

a j8-effect in the laboratory systems. To some extent, this can be simulated

by using top or bottom boundaries such that the depth d increases with

radius. This is exactly equivalent to a /?-effect for a barotropic fluid, but

only approximately so for a baroclinic system. But perhaps the most import-

ant difference is the controlling effect of boundary layers on the cylindrical

inner and outer walls in the annulus. The inner cylinder can be reduced

to small radius (such a system is sometimes called a 'dishpan'), but the

outer boundary condition is really very different from the interface with a

tropical Hadley circulation in a terrestrial type atmosphere, or perhaps

with parallel jets in the case of a Jovian-type atmosphere. Weightless

environments in spacecraft have provided an opportunity to experiment

with a truly spherical laboratory system. Electrostatic forces have been

used to provide a radially symmetric body force to replace the gravitational

force in a planetary atmosphere. But such experiments are necessarily very

expensive, and perhaps numerical experimentation offers a more convenient

way of exploring regimes of planetary circulation now that large computers

are reasonably readily available to atmospheric scientists.

The scientific study of global circulations presents peculiar difficulties

since the number of natural systems which can be observed to check our

theoretical predictions is limited. Numerical models offer the chance to carry

out controlled and detailed experiments. But laboratory experiments provide

another independent way of testing hypotheses. This is always going to be a

stern and challenging task which is likely to lead to those unexpected results

which make any science a continuous adventure into the unknown.

384 Planetary atmospheres

and

other fluid systems

10.7 Problems

10.1 Show that the closure hypothesis, Eq. (10.9), for the simple para-

metrization of baroclinic eddies in a planetary atmosphere is equivalent

to specifying a 'mixing length' L, that is, a distance over which heat is

transported by individual eddies, where

L = 3.2L

10.2 Turbulent eddies are observed in the vicinity of Jupiter's Great Red

Spot, at 28

°S.

Their typical horizontal scale is

1200

km.

Assuming they

are baroclinic waves, estimate the Brunt-Vaisala frequency for Jupiter's

atmosphere. Estimate the difference between the implied and adiabatic lapse

rates.

10.3 A pole-equator temperature difference of around

K is observed

in the Titan atmosphere, between heights of

km and

km above the

surface. Assuming cyclostrophic balance, estimate the zonal wind speed at

these levels.

10.4 Images of Neptune's cloud features suggest the presence of four jets

between the equator and the pole. Assuming these are generated by the

Rhines mechanism, estimate a typical eddy velocity.

10.5 Use the simple analytical model of the global circulation driven by

baroclinic waves, with V

= 0 (Eqs. (10.17) and (10.18)), in the limit of large

a to show that the slope of the meridional heat flux vector is about 1/3 of

the slope of the isentropes.

10.6 Estimate Ro

and Ta for the Earth's midlatitudes. Ta can be estimated

if the thickness of the midlatitude boundary layer, which is around

km, is

used to estimate an effective eddy viscosity. Use your values of Ro

and Ta

to compare the regime of flow of the Earth's midlatitudes with that observed

in the annulus for similar parameters.

10.7 Assuming a 'Sverdrup balance' such that

Pv = k • V x

estimate a typical poleward current in the surface layers of the Southern

Ocean at

°S.

Assume that the surface wind stress is given by pC

| v |

where p is the density of the air and the drag coefficient Q> may be taken

as 10~

over the ocean.

10.8 In the midlatitude ocean, away from the continents, the sea surface

temperature is found to be 15 °C, decreasing to 4 °C at the base of the

10.7 Problems 385

thermocline at a depth of 1.5 km. Assuming a constant salinity and a

coefficient of thermal expansion of lO^K"

, estimate the Brunt-Vaisala

frequency for the upper ocean. Determine the Rossby radius and hence

deduce the typical scale of eddies.

Appendix

Solutions to Problems

A.I Solutions to Chapter 1

1.1 Use Dalton's law of partial pressures, and denote the gas constant of

dry air

and the gas constant of any constituent

R^.

The volume occupied

by unit mass of a consituent with partial pressure e

is simply the specific

volume

= RcT/e

= R

T/p. But if we compress the sample to pressure p

at the same temperature T, the volume it will occupy is

= RcT/p. The

volume mixing ratio r

= a

/a = e

/p. Now consider a unit volume of dry

air: its mass is p = p/RdT. The mass of the constituent is p

/RcT.

Therefore the mass mixing ratio r

is given by p

/p = Rde

/(R

p), i.e.,

= (Rd/RJrv. Since the gas constant R = 7?*/m, the final result is:

Using this formula and the data in Table 1.1, the mass mixing ratios in dry

air are 0.755

for N

, 0.231 38 for O

, 0.012

for Ar and 0.000

for CO

1.2 Since dQ = c

dT —

adp,

and using the hydrostatic relationship dp/dz =

—pg,

we have:

dT . [*

Q= / c

—dz+ / gdz.

Jo cz Jo

Again, using the hydrostatic relation, the Brunt-Vaisala frequency N

can

be written as

(g/T)(T

+ dT/dz), where the adiabatic lapse rate F

= g/c

Hence

dT_

_ N

386

A.I

Solutions

Chapter

387

constant this

can

integrated

give:

The integral

can now

evaluated,

and we

obtain:

Substituting values,

find

+8369

kg"

. This

positive,

i.e.

heat must

be supplied

to the

parcel. This

obvious,

for if it

were lifted adiabatically,

its temperature would follow

the

adiabatic lapse rate,

and

would arrive

at 3000

considerably colder than

its

surroundings.

1.3

Since

the

Coriolis force

always

right angles

the

velocity vector,

the Coriolis force cannot change

the

kinetic energy

the

parcel, only

its

direction

motion. Equating

the

magnitude

the

Coriolis force

to the

magnitude

of the

centripetal force required

maintain circular motion,

radius

have

2QU

sin

</>

= — or r =

2Qsin0*

The time taken to execute

complete circuit is simply 2nr/U or 2n/(2Q sin 0).

Since

the

length

of the day is

2n/Q

the

time taken

for

each orbit

conveniently written

sin 0)"

days. Such motion is termed 'inertial motion'.

1.4

The temperature

can be

written

T(z) = T

+ T(z

-z),

where

the surface temperature,

is the lapse rate and

the elevation

of the surface above mean

sea

level. The hydrostatic relation gives:

dz R(T

+ T(z

-z)Y

Integrating from

z = 0

where

p =

po>

to z = p

where

p = p

gives

the

required relationship

P0=Ps

were

set to

zero (isothermal case),

the

integration would yield:

exp

388 Solutions to Problems

In fact, the two formulae give nearly identical results. Using the given

numbers, po is

100.2693

kPa using the full formula and 100.2665 kPa using

the isothermal formula.

1.5 Let the length of the jet exit be L and its width W. Assuming that the

jet is orientated parallel to the x-axis, Eq. (1.65a) reduces to:

du d fu

u— = 7-hr =fv

dx dx

2 '

or, in finite difference terms:

1 u\-u\

L '

From continuity, dv

/dy =

—dco/dp,

and so the typical vertical velocity is

Choose Ap to be 37.5kPa; then a typical co works out to be 0.25Pas""

upward on the poleward flank of the jet and downward on the equatorward

flank.

1.6 Start with Eq (1.81) and break up the absolute vorticity, density and

potential temperature into a 'reference' part and an anomaly:

CA,

{z)

(z)

Substitute in and linearize by neglecting the products of anomaly quantities

to obtain:

Note from the equation of state and the ideal gas equation that p

from which it follows that

A.2 Solutions to Chapter 2

2.1 Let Q = Q +

Q',

R = R + R'. Then:

QR =

Q')(R

+ R') = QR + Q'R + R'Q + Q'R'.