Hungerford T.W., Shaw D.J. Contemporary Precalculus: A Graphing Approach
Подождите немного. Документ загружается.


There are no cut-and-dried rules for simplifying trigonometric expressions
or proving identities, but there are some common strategies that are often
helpful. Six of these strategies are illustrated in the following examples. There
are often a variety of ways to proceed, and it will take some practice before
you can easily decide which strategies are likely to be the most efficient in a
particular case.
EXAMPLE 2
Simplify (csc x cot x)(1 cos x).
SOLUTION Using Strategy 1, we have
(csc x cot x)(1 cos x)
sin
1
x
c
s
o
in
s
x
x
(1 cos x) [Reciprocal identities]
(1
sin
co
x
s x)
(1 cos x)
1
sin
co
x
s
2
x
s
s
i
i
n
n
2
x
x
[Pythagorean identity]
sin x. ■
(1 cos x)(1 cos x)
sin x
516 CHAPTER 7 Trigonometric Identities and Equations
Basic Trigonometric
Identities
Reciprocal Identities
sec x
co
1
s x
csc x
sin
1
x
tan x
c
s
o
in
s
x
x
cot x
c
s
o
in
s
x
x
tan x
co
1
t x
cot x
ta
1
n x
Periodicity Identities
sin(x 2p) sin x cos(x 2p) cos x
sec(x 2p) sec x csc(x 2p) csc x
tan(x p) tan x cot(x p) cot x
Pythagorean Identities
sin
2
x cos
2
x 11 tan
2
x sec
2
x 1 cot
2
x csc
2
x
Negative Angle Identities
sin(x) sin x cos(x) cos x tan(x) tan x
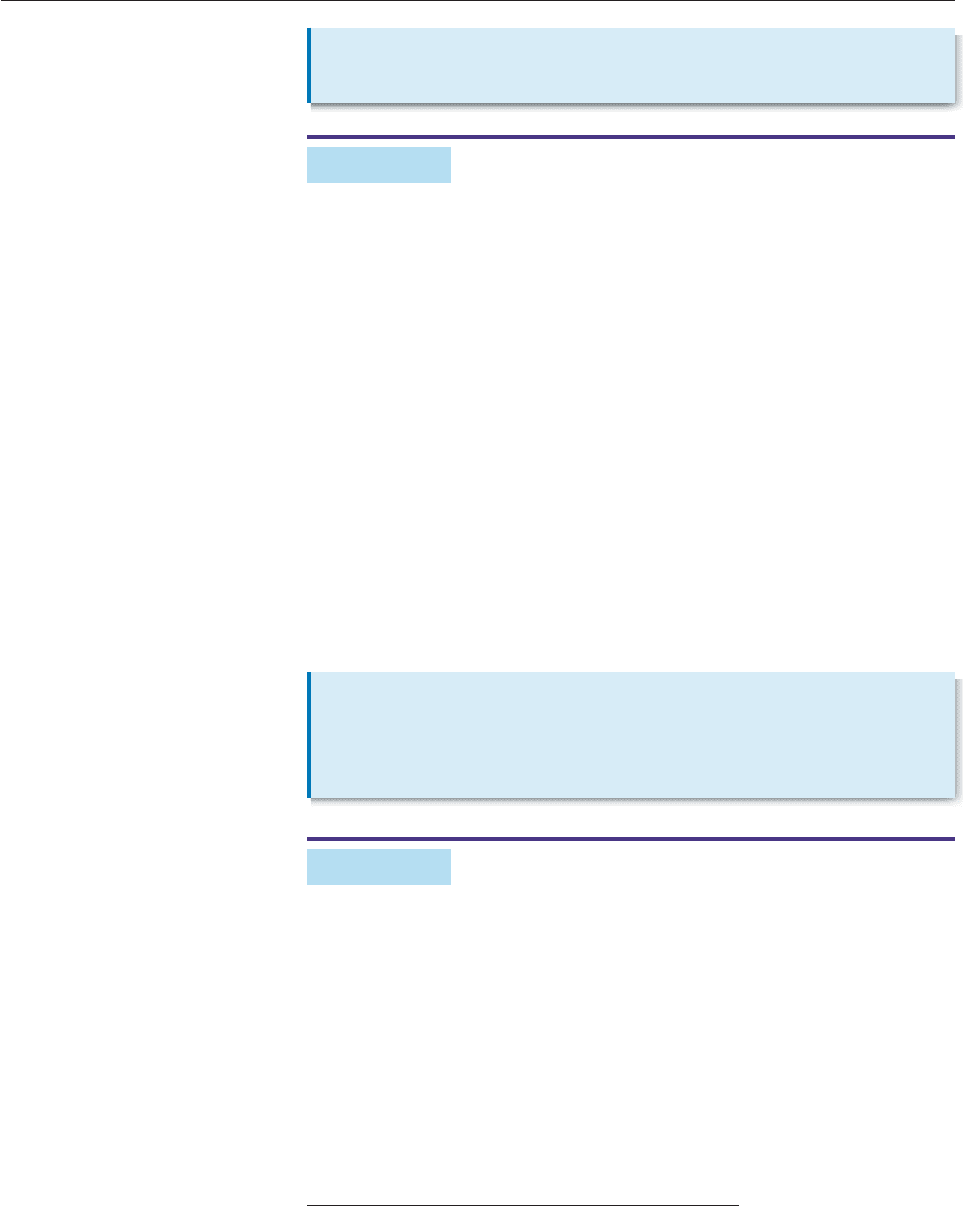
Strategy 1
Express everything in terms of sine and cosine.
EXAMPLE 3
In Example 1, we verified graphically that the equation
1 si
c
n
o
x
s
x
sin
2
x
cos x tan x
might be an identity. Prove that it is.
SOLUTION We use Strategy 2, beginning with the left side of the equation:
1 si
c
n
o
x
s
x
sin
2
x
(1 si
c
n
o
2
x
s
)
x
sin x
cos
2
c
x
o
s x
sin x
[Pythagorean identity]
c
c
o
o
s
s
2
x
x
c
s
o
in
s
x
x
cos x
c
s
o
in
s
x
x
cos x tan x. ■
EXAMPLE 4
Prove that
(1 cos x)(sec x 1) cos x tan
2
x.
SOLUTION We shall use Strategy 3. We begin by multiplying out the left side
and simplifying the result.
(1 cos x)(sec x 1) sec x 1 cos x sec x cos x
sec x 1 cos x
co
1
s x
cos x [Reciprocal identity]
sec x 1 1 cos x
(1 cos x)(sec x 1) sec x cos x (
*
)
SECTION 7.1 Basic Identities and Proofs 517
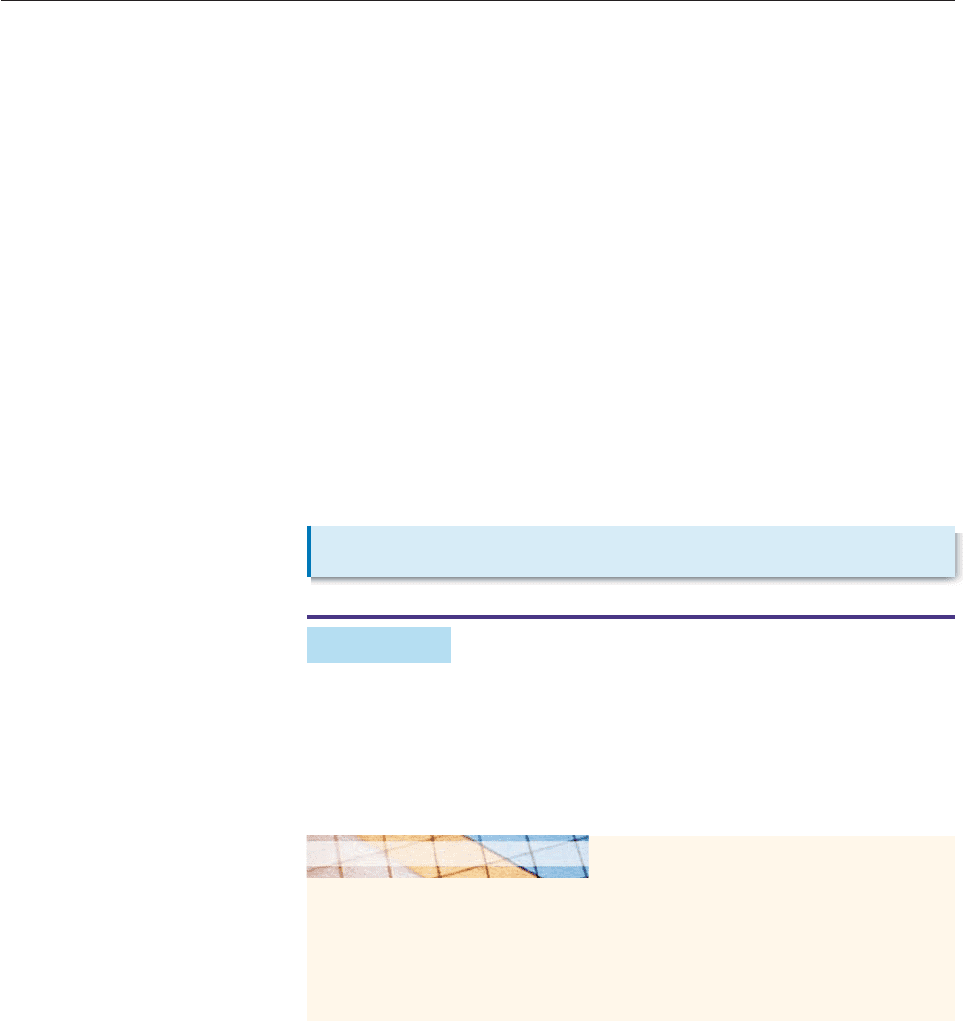
Strategy 2
Use algebra and identities to transform the expression on one side of the
equal sign into the expression on the other side.*
*That is, start with expression A on one side and use identities and algebra to produce a sequence of
equalities A B, B C, C D, D E, where E is the other side of the identity to be proved; conclude
that A E.
Strategy 3
Deal separately with each side of the equation A B. First use identities
and algebra to transform A into some expression C (so that A C). Then
use (possibly different) identities and algebra to transform B into the same
expression C (so that B C). Conclude that A B.
Since the right side of (
*
) is reasonably simple, we now work on the right side of
the alleged identity.
cos x tan
2
x cos x (sec
2
x 1) [Pythagorean identity]
cos x sec
2
x cos x
cos x
co
1
s x
2
cos x [Reciprocal identity]
cos x
co
1
s
2
x
cos x
co
1
s x
cos x
cos x tan
2
x sec x cos x [Reciprocal identity] (
**
)
Equations (
*
) and (
**
) show that (1 cos x)(sec x 1) and cos x tan
2
x are each
equal to sec x cos x. Therefore, they are equal to each other:
(1 cos x)(sec x 1) cos x tan
2
x. ■
There are several useful strategies for dealing with fractions.
EXAMPLE 5
Is this equation an identity?
1
cos
si
x
n x
1
cos
si
x
n x
2 sec x
SOLUTION We first check to see if the equation might be an identity.
518 CHAPTER 7 Trigonometric Identities and Equations
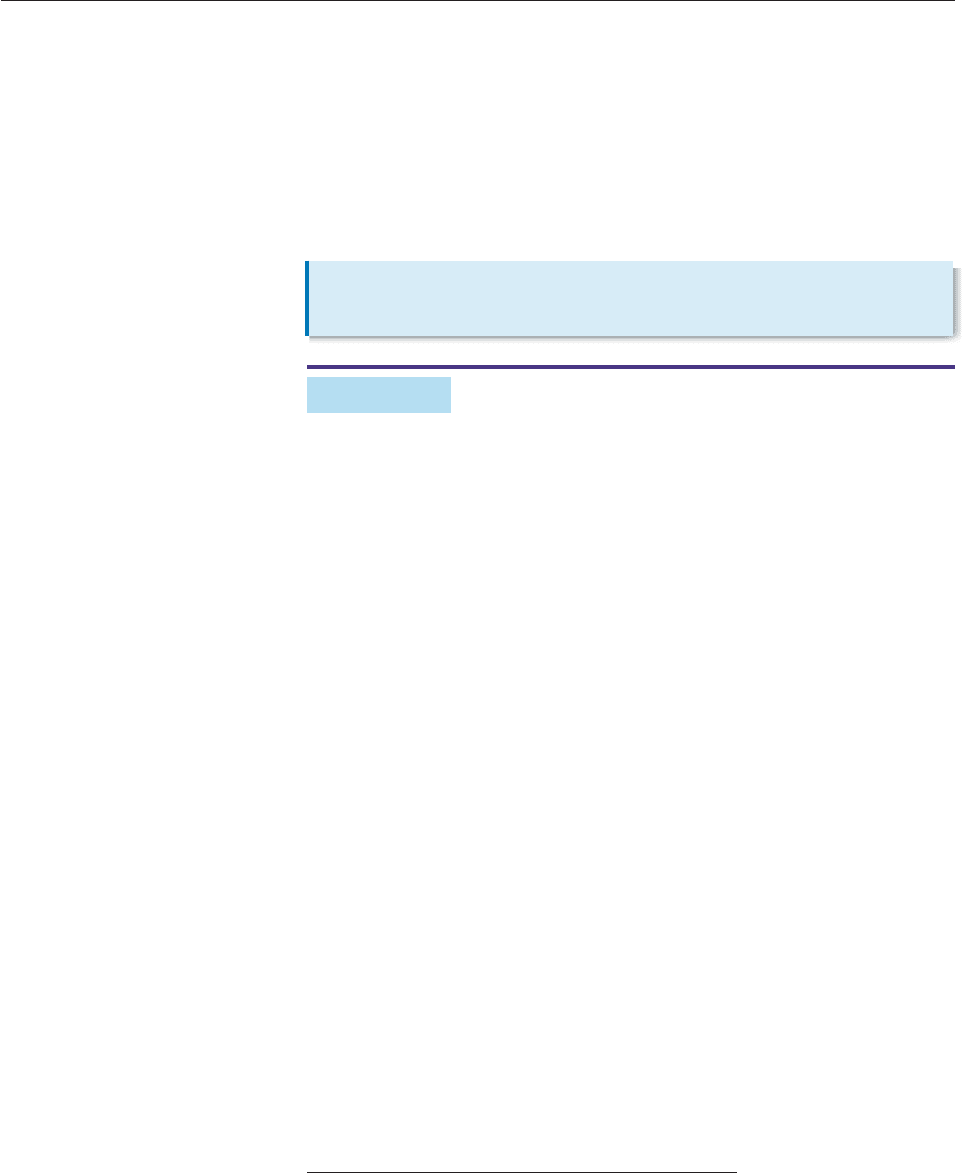
Strategy 4
Combine the sum or difference of two fractions into a single fraction.
On the same screen, graph
y
1
cos
si
x
n x
1
cos
si
x
n x
and y 2 sec x
co
2
s x
.
If the two graphs appear to be identical, the equation could possibly be an identity.
Do they?
GRAPHING EXPLORATION
The exploration suggests that this equation might be an identity. So we try to
prove it, beginning with Strategy 4. Express the fractions on the left side in terms
of a common denominator and add them. Then (using strategy 2) we attempt to
transform this result into 2 sec x.
1
cos
si
x
n x
1
cos
si
x
n x
co
(c
s
o
x
s
(1
x)
(co
s
s
in
x)
x)
[Common denominator]
[Add fractions]
(1 sin x)
2
cos
2
x
cos x(1 sin x)
(1 sin x)(1 sin x)
cos x(1 sin x)
[Expand numerator]
c
1
o
s x
2
(1
si
n x
si
n x
1
)
[Pythagorean identity]
cos
2
x
(1
2
si
s
n
in
x
x)
co
2
s
(
x
1
(
1
sin
si
x
n
)
x)
co
2
s x
2 sec x [Reciprocal identity] ■
1 2 sin x sin
2
x cos
2
x
cos x(1 sin x)
SECTION 7.1 Basic Identities and Proofs 519
Strategy 5
Rewrite a fraction in an equivalent form by multiplying its numerator and
denominator by the same quantity.
*This is analogous to the process used to rationalize the denominator of a fraction by multiplying its
numerator and denominator by the conjugate of the denominator, as in the example:
3
1
2
3
1
2
3
3
2
2
3
2
3
(
2
2
)
2
3
7
2
.
EXAMPLE 6
Prove that
1
sin
co
x
s x
1
sin
co
x
s x
.
SOLUTION We shall use Strategy 2 and transform the left side into the right
side. We apply strategy 5 by multiplying the numerator and denominator of the
left side by 1 cos x:*
1
sin
co
x
s x
1
sin
co
x
s x
1
1
c
c
o
o
s
s
x
x
sin
1
x(
1
cos
c
2
o
x
s x)
sin x(1
sin
2
x
cos x)
[Pythagorean identity]
1
sin
co
x
s x
.
ALTERNATE SOLUTION The numerators of the given equation look similar to
the Pythagorean identity—with the squares missing. So we begin with the left
side and introduce some squares by multiplying it by
s
s
i
i
n
n
x
x
1.
1
sin
co
x
s x
1
1
sin
co
x
s x
s
s
i
i
n
n
x
x
1
sin
co
x
s x
sin x(1
sin
2
x
cos x)
sin
1
x(
1
cos
c
2
o
x
s x)
[Pythagorean identity]
[Factor numerator]
1
sin
co
x
s x
. ■
(1 cos x)(1 cos x)
sin x(1 cos x)
sin x(1 cos x)
(1 cos x)(1 cos x)

Proving identities involving fractions can sometimes be quite complicated. It
often helps to approach a fractional identity indirectly, as in the following example.
EXAMPLE 7
Prove these identities.
(a) sec x(sec x cos x) tan
2
x (b)
s
ta
e
n
c
x
x
sec x
ta
n x
cos x
.
SOLUTION
(a) Beginning with the left side (Strategy 2), we have
sec x(sec x cos x) sec
2
x sec x cos x
sec
2
x
co
1
s x
cos x [Reciprocal identity]
sec
2
x 1
tan
2
x. [Pythagorean identity]
Therefore, sec x(sec x cos x) tan
2
x.
(b) By part (a), we know that
sec x(sec x cos x) tan x tan x.
Dividing both sides of this equation by tan x(sec x cos x) shows that
s
ta
e
n
c
x
x
sec x
ta
n x
cos x
. ■
Look carefully at how identity (b) was proved in Example 7. We first proved
identity (a):
sec x(sec x cos x) tan x
tan x.
AD BC
Then we divided both sides of AD BC by BD tan x(sec x cos x) and can-
celled factors to obtain identity (b):
A
B
D
D
B
B
D
C
s
ta
e
n
c
x
x
sec x
ta
n x
cos x
A
B
D
C
.
The same argument works in the general case and provides the following strategy
for dealing with identities involving fractions.
tan x tan x
tan x(sec x cos x)
sec x(sec x cos x)
tan x(sec x cos x)
tan x tan x
tan x(sec x cos x)
sec x(sec x cos x)
tan x(sec x cos x)
520 CHAPTER 7 Trigonometric Identities and Equations
——
—
——
14243

Many students misunderstand Strategy 6: It does not say that you begin with
a fractional equation A/B C/D and cross-multiply to eliminate the fractions. If
you did that, you would be assuming what has to be proved. What the strategy
says is that to prove an identity involving fractions, you need only prove a differ-
ent identity that does not involve fractions. In other words, if you prove that
AD BC whenever B 0 and D 0, then you can conclude that A/B C/D.
Note that you do not assume that AD BC; you use Strategy 2 or 3 or some other
means to prove this statement.
EXAMPLE 8
Prove that
c
c
o
o
t
t
x
x
1
1
1
1
t
t
a
a
n
n
x
x
.
SOLUTION We use Strategy 6, with A cot x 1, B cot x 1,
C 1 tan x, and D 1 tan x. We must prove that this equation is an identity:
AD BC
(
***
) (cot x 1)(1 tan x) (cot x 1)(1 tan x).
Strategy 3 will be used. Multiplying out the left side shows that
(cot x 1)(1 tan x) cot x 1 cot x tan x tan x
cot x 1
ta
1
n x
tan x tan x
cot x 1 1 tan x
cot x tan x.
Similarly, on the right side of (
***
),
(cot x 1)(1 tan x) cot x 1 cot x tan x tan x
cot x 1 1 tan x
cot x tan x.
Since the left and right sides are equal to the same expression, we have proved
that (
***
) is an identity. Therefore, by Strategy 4, we conclude that
c
c
o
o
t
t
x
x
1
1
1
1
t
t
a
a
n
n
x
x
is also an identity. ■
It takes a good deal of practice, as well as much trial and error, to become pro-
ficient in proving identities. The more practice you have, the easier it will get.
Since there are many correct methods, your proofs may be quite different from
those of your instructor or the text answers.
SECTION 7.1 Basic Identities and Proofs 521
Strategy 6
If you can prove that AD BC, with B 0 and D 0, then you can con-
clude that
A
B
D
C
.
TECHNOLOGY TIP
Using SOLVE in the TI-89 ALGEBRA
menu to solve an equation that might
be an identity produces one of three
responses. “True” means the equation
probably is an identity [algebraic proof
is required for certainty]. “False”
means the equation is not an identity.
A numerical answer is inconclusive
[the equation may or may not be an
identity].

EXERCISES 7.1
If you don’t see what to do immediately, try something and see where it leads:
Multiply out or factor or multiply numerator and denominator by the same
nonzero quantity. Even if this doesn’t lead anywhere, it might give you some ideas
on other things to try. When you do obtain a proof, check to see whether it can be
done more efficiently. In your final proof, don’t include the side trips that may
have given you some ideas but aren’t themselves part of the proof.
522 CHAPTER 7 Trigonometric Identities and Equations
In Exercises 25–64, state whether or not the equation is an
identity. If it is an identity, prove it.
25. sin x
1 co
s
2
x
26. cot x
c
se
sc
c
x
x
27.
c
s
o
in
s
(
(
x
x
)
)
tan x 28. tan x
sec
2
x
1
29. cot(x) cot x 30. sec(x) sec x
31. 1 sec
2
x tan
2
x
32. sec
4
x tan
4
x 1 2 tan
2
x
33. sec
2
x csc
2
x tan
2
x cot
2
x
34. sec
2
x csc
2
x sec
2
x csc
2
x
35. sin
2
x(cot x 1)
2
cos
2
x(tan x 1)
2
36. cos
2
x(sec x 1)
2
(1 cos x)
2
37. sin
2
x tan
2
x sin
2
x tan
2
x
38. cot
2
x 1 csc
2
x
39. (cos
2
x 1)(tan
2
x 1) tan
2
x
40. (1 cos
2
x)csc x sin x
41. tan x
s
c
e
s
c
c
x
x
42.
c
s
o
in
s
(
(
x
x
)
)
cot x
43. cos
4
x sin
4
x cos
2
x sin
2
x
44. cot
2
x cos
2
x cos
2
x cot
2
x
45. (sin x cos x)
2
sin
2
x cos
2
x
46. (1 tan x)
2
sec
2
x
47.
1
sin
si
x
n x
csc
co
x
t
2
x
1
48.
1
1
sin x
1
1
sin x
2 tan x sec x
49.
1
1
s
s
i
i
n
n
x
x
s
s
e
e
c
c
x
x
t
t
a
a
n
n
x
x
50.
c
s
o
in
s
x
x
1
cos
si
x
n x
sec x
51.
ta
1
n
x
co
s
s
in
x
x
tan x
52.
1
1
sin x
1
1
sin x
2 sec
2
x
53.
1
cos
si
x
n x
1
cos
si
x
n x
In Exercises 1–4, test the equation graphically to determine
whether it might be an identity. You need not prove those equa-
tions that seem to be identities.
1.
sec x
se
c x
cos x
sin
2
x
2. tan x cot x (sin x)(cos x)
3.
1 c
2
os(2x)
sin
2
x
4.
tan x
cs
c x
cot x
sec x
In Exercises 5–8, insert one of A–F on the right of the equal
sign so that the resulting equation appears to be an identity
when you test it graphically. You need not prove the identity.
A. cos x B. sec x C. sin
2
x
D. sec
2
x E. sin x cos x F.
sin x
1
cos x
5. csc x tan x 6.
t
s
a
in
n
x
x
7.
s
s
i
i
n
n
4
x
x
c
c
o
o
s
s
4
x
x
8. tan
2
(x)
si
s
n
i
(
n
x
x)
In Exercises 9–24, prove the identity.
9. tan x cos x sin x 10. cot x sin x cos x
11. cos x sec x 1 12. sin x csc x 1
13. tan x csc x sec x 14. sec x cot x csc x
15.
s
ta
e
n
c
x
x
sin x 16.
c
c
s
o
c
t x
x
cos x
17. (1 cos x)(1 cos x) sin
2
x
18. (csc x 1)(csc x 1) cot
2
x
19. cot x sec x sin x 1
20. sin x tan x cos x sec x
21. tan x cot x sec x csc x
22. tan x(cos x csc x) sin x sec x
23. cos x cos x sin
2
x cos
3
x
24. cos x sec x sin
2
x cos
2
x
54.
1
1
c
ta
o
n
t x
x
tan x
55.
sec
s
2
e
x
c
2
x
1
sin
2
x
56.
csc
c
2
s
x
c
2
x
1
cos
2
x
57.
s
c
e
s
c
c
x
x
c
s
o
in
s
x
x
2 tan x
58.
1
sin
co
x
s x
1
sin
co
x
s x
2 csc x
59.
se
1
c
x
ta
c
n
s
x
c x
csc x 60.
c
1
o
t x
ta
n
1
x
c
se
sc
c
x
x
61.
csc x
1
sin x
sec x tan x
62.
1
csc
cs
x
c x
1
cos
s
2
i
x
n x
63.
sin x
ta
n x
cos x
sin x
ta
n x
cos x
64.
csc
co
x
t
x
1
csc
co
x
t
x
1
In Exercises 65–68, half of an identity is given. Graph this half
in a viewing window with 2p x 2p and make a conjec-
ture as to what the right side of the identity is. Then prove your
conjecture.
65. 1
1
sin
c
2
o
x
s x
? [Hint: What familiar function has a
graph that looks like this?]
66.
1 co
s
s
in
x
x
cos
2
x
cot x ?
SECTION 7.2 Addition and Subtraction Identities 523
67. (sin x cos x)(sec x csc x) cot x 2 ?
68. cos
3
x(1 tan
4
x sec
4
x) ?
In Exercises 69–82, prove the identity.
69.
1
sec
si
x
n x
1
cos
s
3
i
x
n x
70.
1
sin
co
x
t x
1
cos
ta
x
n x
cos x sin x
71.
1
cos
si
x
n x
sec x tan x
72.
ta
1
n
x
se
s
c
in
x
x
csc x
73.
co
c
t
o
x
s x
c
c
o
o
t
s
x
x
co
c
t
o
x
s x
c
c
o
o
t
s
x
x
74.
c
c
o
o
s
s
3
x
x
s
s
i
i
n
n
3
x
x
1 sin x cos x
75. log
10
(cot x) log
10
(tan x)
76. log
10
(sec x) log
10
(cos x)
77. log
10
(csc x cot x) log
10
(csc x cot x)
78. log
10
(sec x tan x) log
10
(sec x tan x)
79. tan x tan y tan x tan y(cot x cot y)
80.
t
c
a
o
n
t
x
x
t
c
a
o
n
t
y
y
tan x tan y
81.
c
c
o
o
s
s
x
y
s
s
i
i
n
n
y
x
c
c
o
o
s
s
y
x
s
s
i
i
n
n
x
y
82.
t
c
a
o
n
t
x
x
t
c
a
o
n
t
y
y
t
1
an
x
c
t
o
a
t
n
x
y
c
ot
1
y
7.2 Addition and Subtraction Identities
■ Use the addition and subtraction identities to evaluate
trigonometric functions.
■ Use the addition and subtraction identities to prove other
identities.
■ Use the cofunction identities to prove other identities.
A common student ERROR is to write
sin
x
p
6
sin x sin
p
6
sin x
1
2
.
Section Objectives
Verify graphically that the equation above is NOT an identity by graphing
y sin(x p/6) and y sin x 1/2 on the same screen.
GRAPHING EXPLORATION

The exploration shows that “sin(x y) sin x sin y” is NOT an identity
(because it’s false when y p/6). There is an identity that enables us to express
sin(x y), but it is a bit more complicated, as we now see.
The addition and subtraction identities are probably the most important of all
the trigonometric identities. Before reading their proofs at the end of this section,
you should become familiar with the examples and special cases below.
EXAMPLE 1
Use the addition and subtraction identities to find the exact values of
(a) sin
1
p
2
(b) cos
7
1
p
2
.
SOLUTION The key here is to write p/12 and 7p/12 as a sum or difference
of two numbers whose sine and cosine are known.
(a) Note that
1
p
2
4
1
p
2
3
1
p
2
p
3
p
4
.
we apply the subtraction identity for sine with x p/3 and y p/4.
sin
1
p
2
sin
p
3
p
4
sin
p
3
cos
p
4
cos
p
3
sin
p
4
2
3
2
2
1
2
2
2
3
4
2
4
2
4
6
4
2
6
4
2
(b) In this case, we see that
7
1
p
2
4
1
p
2
3
1
p
2
p
3
p
4
. So we apply the addition
identity for cosine with x p/3 and y p/4.
cos
7
1
p
2
cos
p
3
p
4
cos
p
3
cos
p
4
sin
p
3
sin
p
4
1
2
2
2
2
3
2
2
4
2
3
4
2
4
2
4
6
2
4
6
. ■
524 CHAPTER 7 Trigonometric Identities and Equations
Addition and
Subtraction Identities
sin(x y) sin x cos y cos x sin y
sin(x y) sin x cos y cos x sin y
cos(x y) cos x cos y sin x sin y
cos(x y) cos x cos y sin x sin y
EXAMPLE 2
Find sin(p y).
SOLUTION Apply the subtraction identity for sine with x p.
sin(p y) sin p cos y cos p sin y
(0)(cos y) (1)(sin y)
sin y. ■
EXAMPLE 3
Show that the difference quotient of the function f (x) sin x is given by:
f (x h
h
) f (x)
sin x
cos h
h
1
cos x
sin
h
h
.
[This fact is needed in calculus.]
SOLUTION Use the addition identity for sin(x y) with y h.
f(x h
h
) f(x)
sin(x h
h
) sin x
sin x
cos h
h
1
cos x
sin
h
h
. ■
EXAMPLE 4
Prove the identity:
c
c
o
o
s
s(
x
x
c
os
y
y
)
1 tan x tan y.
SOLUTION Begin with the left side and apply the addition identity for cosine
to the numerator.
c
c
o
o
s
s(
x
x
c
os
y
y
)
c
c
o
o
s
s
x
x
c
c
o
o
s
s
y
y
c
s
o
in
s
x
x
s
c
i
o
n
s
y
y
1
c
s
o
in
s
x
x
c
s
o
in
s
y
y
1 tan x tan y. ■
cos x cos y sin x sin y
cos x cos y
sin x(cos h 1) cos x sin h
h
sin x cos h cos x sin h sin x
h
SECTION 7.2 Addition and Subtraction Identities 525
