Hungerford T.W., Shaw D.J. Contemporary Precalculus: A Graphing Approach
Подождите немного. Документ загружается.

SOLUTION
Product to a power (law 4):
(x
x
y
7/
7
2
/
y
4
3
)
2
x
2
x
(
7
y
/
7
2
/
y
4
3
)
2
7
4
2
7
2
:
x
x
7
2
/
y
2
7
y
/
3
2
Division with exponents (law 2): x
7/22
y
37/2
7
2
2
3
2
and 3
7
2
1
2
: x
3/2
y
1/2
Negative exponents (law 6):
x
y
3
1
/
/
2
2
■
RADICAL NOTATION
As we saw above, there are two notations for nth roots:
n
c
and c
1/n
. Similarly,
c
t/k
can be expressed in terms of radicals.
c
t/k
(c
t
)
1/k
k
c
t
and c
t/k
(c
1/k
)
t
(
k
c
)
t
.
When radical notation is used, the exponent laws have a different form. For instance,
(cd)
1/n
c
1/n
d
1/n
means the same thing as
n
cd
n
c
n
d
;
d
c
1/n
d
c
1
1
/
/
n
n
means the same thing as
n
d
c
n
n
d
c
.
When simplifying an expression involving radicals, either you can change to
rational exponents and proceed as in Examples 3–5, or you can use the exponent
laws in their radical notation, as in the next example.
EXAMPLE 6
Simplify each of the following.
(a) 63 (b) 12 75 (c)
3
40k
4
SOLUTION
(a) Look for perfect squares.
Factor a perfect square out of 63: 63 9
7
Write as a product of roots: 9
7
3 7
(b) Look for perfect squares.
Factor perfect squares out of 12 and 75: 12 75 4
3
25
3
Product of roots: 4
3
253
2 3
5 3
3 3
.
(c) Look for perfect cubes. We note that 8k
3
(2k)
3
is a perfect cube.
Factor out 8k
3
:
3
40k
4
3
8k
3
5
k
Product of roots:
3
8k
3
3
5k
3
(2k)
3
3
5k
2k
3
5k
. ■
346 CHAPTER 5 Exponential and Logarithmic Functions

EXAMPLE 7
Compute each of the following
(a) 27
5/3
(b) 2
3/2
(c) 256
3/4
(d) (1)
2/6
SOLUTION
(a) 27
5/3
27
1
5/3
3
1
27
5
3
1
5
2
1
43
(b) 2
3/2
2
3
22
(c) 256
3/4
4
256
3
4
3
64
(d) Recall that, in order to use our definition of rational exponents, the frac-
tion must be in lowest terms. So (1)
2/6
(1)
1/3
3
1
1. Notice that
we get an incorrect answer if we do not first reduce the fraction, because
6
(1)
2
6
1
1. ■
RATIONALIZING DENOMINATORS AND NUMERATORS
When dealing with fractions in the days before calculators, it was customary to
rationalize the denominators, that is, write equivalent fractions with no radicals
in the denominator, because this made many computations easier. With calcula-
tors, of course, there is no computational advantage to rationalizing denomina-
tors. Nevertheless, rationalizing denominators or numerators is sometimes
needed to simplify expressions and to derive useful formulas. For example,
several key calculus formulas can be derived by rationalizing the numerator of a
rational expression.
EXAMPLE 8
(a) To rationalize the denominator of
7
5
, multiply it by 1, with 1 written as a
suitable radical fraction.
7
5
7
5
1
7
5
5
5
7
5
5
.
(b) To rationalize the denominator of
3
2
6
, multiply by 1 written as a radi-
cal fraction and use the multiplication pattern (a b)(a b) a
2
b
2
.
3
2
6
3
2
6
1
3
2
6
3
3
6
6
3
6
2
2
(
6
6
)
2
6
9
2
6
6
6
3
2 6
. ■
2(3 6
)
(3 6
)(3 6
)
SECTION 5.1 Radicals and Rational Exponents 347
EXAMPLE 9
Assume that h 0, and rationalize the numerator of
x h
h
x
; that is, write
an equivalent fraction with no radicals in the numerator.
SOLUTION Again, the technique is to multiply the fraction by 1, with 1 writ-
ten as a suitable radical fraction:
x h
h
x
x h
h
x
1
x h
h
x
x
x
h
h
x
x
h(
x
x
h
h
x
x
)
h(x
h
h
x
)
x
1
h
x
.
Notice that in its original form, it is hard to determine what happens to this
fraction when h is close to zero. In the final form, we can see that when h gets
close to zero, the expression gets close to 1/2x
. ■
IRRATIONAL EXPONENTS
An example will illustrate how a
t
is defined when t is an irrational number.*
To compute 10
2
we use the infinite decimal expansion 2
1.414213562
...
(see Special Topics 1.1.A). Each of
1.4, 1.41, 1.414, 1.4142, 1.41421,
is a rational number approximation of 2
, and each is a more accurate approxi-
mation than the preceding one. We know how to raise 10 to each of these rational
numbers:
10
1.4
10
14/100
25.1189
10
1.41
10
141/1,000
25.7040
10
1.414
10
1,414/10,000
25.9418
10
1.4142
10
14,142/100,000
25.9537
10
1.41421
10
141,421/1,000,000
25.9543
10
1.414213
10
1,414,213/10,000,000
25.9545
It appears that as the exponent r gets closer and closer to 2
, 10
r
gets closer and
closer to a real number whose decimal expansion begins 25.954
. We define
10
2
to be this number.
Similarly, for any a 0,
a
t
is a well-defined positive number for each real exponent t.
We shall also assume this fact.
The exponent laws (page 345) are valid for all real exponents.
(x h
)
2
(x
)
2
h(x h
x
)
348 CHAPTER 5 Exponential and Logarithmic Functions
*This example is not a proof but should make the idea plausible. Calculus is required for a rigorous proof.

SECTION 5.1 Radicals and Rational Exponents 349
EXERCISES 5.1
Note: Unless directed otherwise, assume that all letters repre-
sent positive real numbers.
In Exercises 1–10, simplify the expression. Assume a, b, c, d 0.
1. (25k
2
)
3/2
(16k
1/3
)
3/4
2. (4x
5/6
)(2y
3/4
)(x
7/6
)(3y
1/4
)
3. (c
2/5
d
2/3
)(c
6
d
3
)
4/3
4.
3
3
x
2
y
3
9
x
1/3
y
3/5
2
5.
(x
2
)
3
1
x
/
2
3
/
(
3
y
y
2
2
)
2/3
6.
(a
1/
(a
2
b
b
2
2
)
)
3
2
(
(
a
b
1
c
/
5
2
)
b
0
0
c)
7.
(
(
7
5
a
a
)
)
2
3
(
/
5
2
(
b
7
)
b
3/
)
2
4
8.
9. (a
x
2
)
1/x
10.
(b
b
x
)
x
x
1
In Exercises 11–16, compute and simplify.
11. x
1/2
(x
2/3
x
4/3
)
12. x
1/2
(3x
3/2
2x
1/2
)
13. (x
1/2
y
1/2
)(x
1/2
y
1/2
)
14. (x
1/3
y
1/2
)(2x
1/3
y
3/2
)
15. (x y)
1/2
[(x y)
1/2
(x y)]
16. (x
1/3
y
1/3
)(x
2/3
x
1/3
y
1/3
y
2/3
)
In Exercises 17–22, factor the given expression. For example,
x x
1/2
2 (x
1/2
2)(x
1/2
1).
17. x
2/3
x
1/3
6 18. x
2/7
2x
1/7
15
19. x 4x
1/2
3 20. x
1/3
11x
1/6
24
21. x
4/5
81 22. x 3x
2/3
3x
1/3
1
In Exercises 23–28, write the given expression without using
radicals.
23.
1
x
24.
5
x
2
25. a a b
26.
3
a
3
b
4
27.
5
t
16t
5
28. x
(
3
x
2
)(
4
x
3
)
In Exercises 29–44, simplify the expression without using a
calculator.
29. 80 30. 120
31. 6
12 32.
3
12
3
10
33.
6
15
99
34.
18
3
126
35. 50 72 36. 150
24
37. 5 20 45 2 80
38.
3
40 2
3
135
5
3
320
ab
3
ab
4
a
3
b
4
39.
16a
8
b
2
40. 54m
6
n
3
41. 42.
43. 44.
In Exercises 45–52, rationalize the denominator and simplify
your answer.
45.
3
8
46.
2
6
47.
2
3
12
48.
5
1
1
3
0
49.
x
2
2
50.
x
x
c
51. 52.
In Exercises 53–56, use the fact that x
3
y
3
(x y)(x
2
xy y
2
) to rationalize the denominator.
53. 54.
55. 56.
In Exercises 57–60, find the difference quotient of the given
function. Then rationalize its numerator and simplify.
57. f(x) x 1
58. g(x) 2 x 3
59. f(x)
x
2
1
60. g(x)
x
2
x
In Exercises 61–64, use the equation y 92.8935
x
.6669
which gives the approximate distance y (in millions of miles)
from the sun to a planet that takes x earth years to complete
one orbit of the sun. Find the distance from the sun to the
planet whose orbit time is given.
61. Mercury (.24 years)
62. Mars (1.88 years)
63. Saturn (29.46 years)
64. Pluto (247.69 years)
Between 1790 and 1860, the population y of the United States
(in millions) in year x was given by y 3.9572(1.0299
x
), where
x 0 corresponds to 1790. In Exercises 65–68, find the U.S.
population in the given year.
65. 1800 66. 1817
67. 1845 68. 1859
3
3
2
3
3
1
3
4
3
2
1
5
6
3
5
1
3
3
1
6
3
4
10
3
2
5
16a
4
b
2
5
2
1
a
14
b
3
3
a
5
b
4
c
3
3
a
1
b
2
c
6
a
10
b
12
a
14
d
4
c
2
d
6
4c
3
d
4
350 CHAPTER 5 Exponential and Logarithmic Functions
69. Here are some of the reasons why restrictions are necessary
when defining fractional powers of a negative number.
(a) Explain why the equations x
2
4, x
4
4,
x
6
4, etc., have no real solutions. Hence, we cannot
define c
1/2
, c
1/4
, c
1/6
when c 4.
(b) Since 1/3 is the same as 2/6, it should be true that
c
1/3
c
2/6
, that is, that
3
c
6
c
2
. Show that this is
false when c 8.
70. Use a calculator to find (3141)
3141
. Explain why your
answer cannot possibly be the number (3141)
3141
. Why
does your calculator behave the way that it does?
71. (a) Graph f (x) x
5
and explain why this function has an
inverse function.
(b) Show algebraically that the inverse function is
g(x) x
1/5
.
(c) Does f(x) x
6
have an inverse function? Why or why
not?
72. If n is an odd positive integer, show that f(x) x
n
has an
inverse function and find the rule of the inverse function.
[Hint: Exercise 71 is the case when n 5.]
In Exercises 73–75, use the catalog of basic functions
(page 170) and Section 3.4 to describe the graph of the given
function.
73. g(x) x 3
74. h(x) x
2
75. k(x) x 4
4
76. (a) Suppose r is a solution of the equation x
n
c and s is a
solution of x
n
d. Verify that rs is a solution of
x
n
cd.
(b) Explain why part (a) shows that
n
cd
n
c
n
d
.
77. The output Q of an industry depends on labor L and capital
C according to the equation
Q L
1/4
C
3/4
.
(a) Use a calculator to determine the output for the follow-
ing resource combinations.
(b) When you double both labor and capital, what happens
to the output? When you triple both labor and capital,
what happens to the output?
78. Do Exercise 77 when the equation relating output to
resources is Q L
1/4
C
1/2
.
79. Do Exercise 77 when the equation relating output to
resources is Q L
1/2
C
3/4
.
80. In Exercises 77–79, how does the sum of the exponents on
L and C affect the increase in output?
LCQ L
1/4
C
3/4
10 7
20 14
30 21
40 28
60 42
5.1.A SPECIAL TOPICS Radical Equations
■ Use algebraic and graphical methods to solve radical equations.
■ Solve applied problems that involve radicals.
The algebraic solution of equations involving radicals depends on this fact: If two
quantities are equal, say,
x 2 3,
then their squares are also equal:
(x 2)
2
9.
Thus,
every solution of x 2 3 is also a solution of (x 2)
2
9.
But be careful: This works only in one direction. For instance, 1 is a solution of
(x 2)
2
9, but not of x 2 3. This is an example of the Power Principle.
Section Objectives
Power
Principle
If both sides of an equation are raised to the same positive integer power,
then every solution of the original equation is also a solution of the new
equation. But the new equation may have solutions that are not solutions of
the original one.
Consequently, if you raise both sides of an equation to a power, you must
check your solutions in the original equation. Graphing provides a quick way to
eliminate most extraneous solutions. But only an algebraic computation can con-
firm an exact solution.
EXAMPLE 1
Solve 5 3x 1
1
x.
SOLUTION We first rearrange terms to get the radical expression alone on
one side.
3x 1
1
x 5.
Then we square both sides and solve the resulting equation.
(3x 1
1
)
2
(x 5)
2
3x 11 x
2
10x 25
0 x
2
13x 36
0 (x 4)(x 9)
x 4 0orx 9 0
x 4 x 9.
These are possible solutions. We must check each one in the original equation. If
x 9 we have
Left side: 5 3x 1
1
Right side: x
5 3
9
11
9
5 16
9
Hence, x 9 is a solution of the original equation. When we try the same calcu-
lations with x 4, we obtain
Left side: 5 3x 1
1
Right side: x
5 3 4
11
4
5
1
6
The two sides are not the same, so x 4 is not a solution of the original equation.
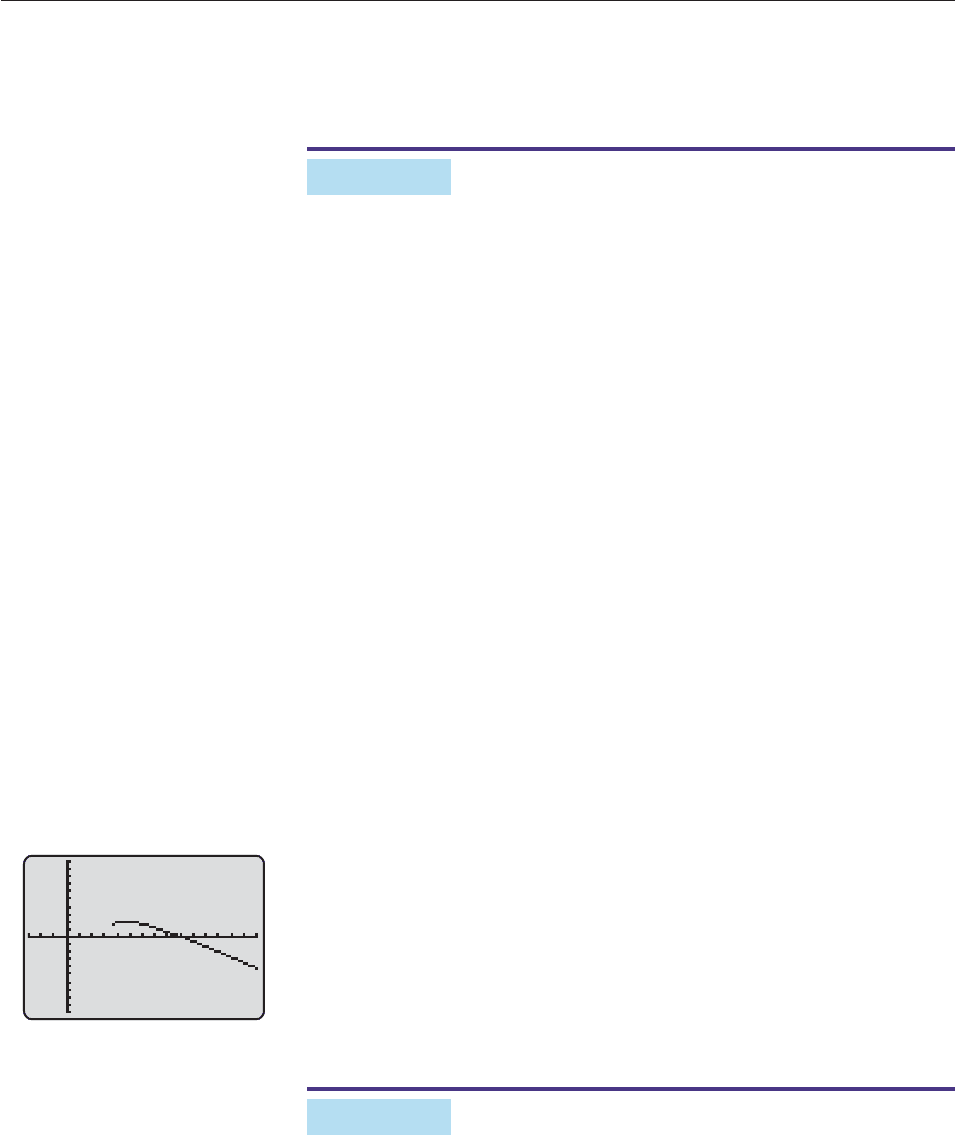
These results can be confirmed graphically by graphing y 5
3x 1
1
x,
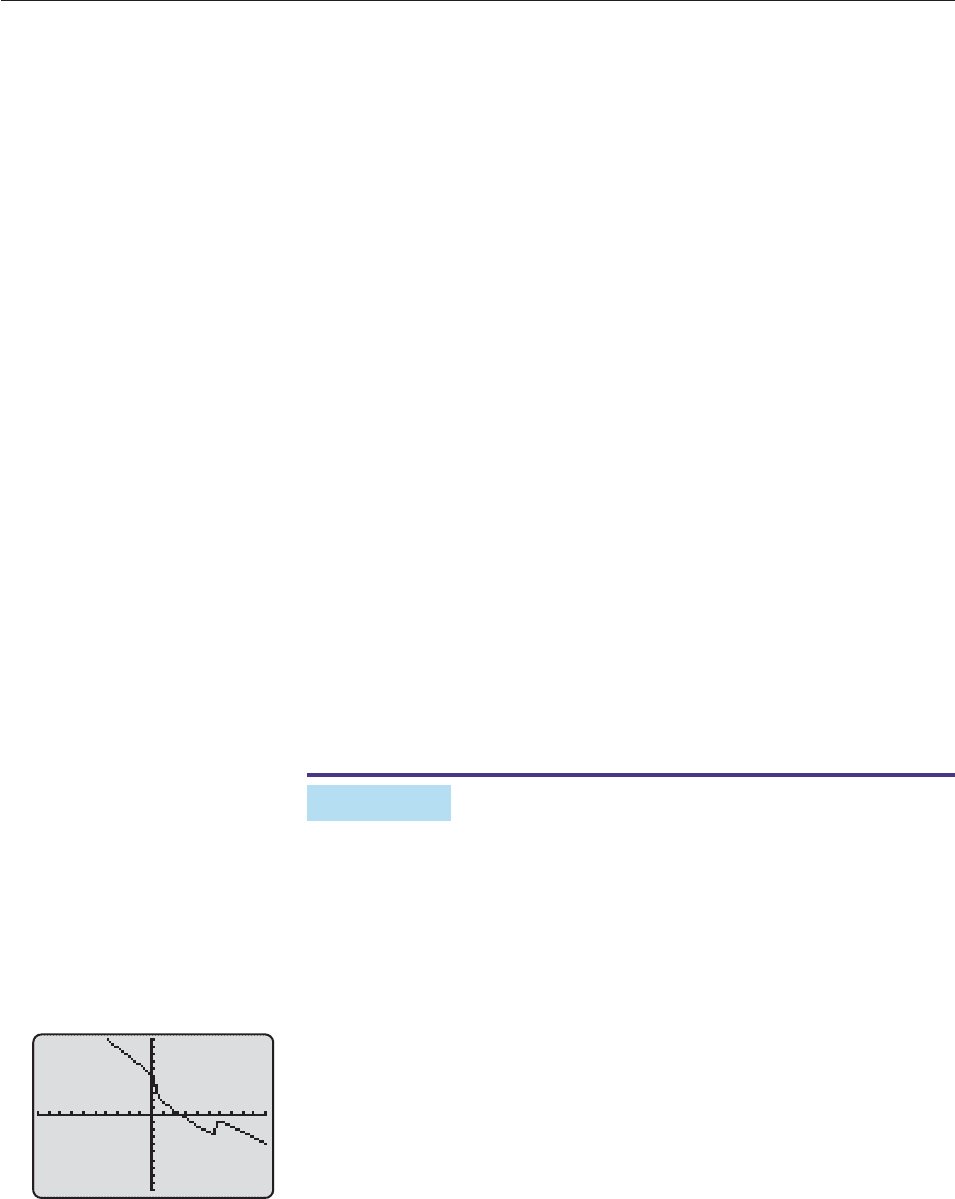
as in Figure 5–2. The x-intercepts of this graph are the solutions of the equation
(why?). There is an x-intercept at x 9, but none at x 4, indicating that x 9
is a solution and x 4 is not. ■
EXAMPLE 2
Solve 2x 3
x 7
2.
SOLUTION We first rearrange terms so that one side contains only a single
radical term.
2x 3
x 7
2.
SPECIAL TOPICS 5.1.A Radical Equations 351
10
15
−3
−10
Figure 5–2
Then we square both sides and simplify.
(2x 3
)
2
(x 7
2)
2
2x 3 (x 7
)
2
2
2
x 7
2
2
2x 3 x 7 4 x 7
4
x 14 4 x 7
.
Now we square both sides and solve the resulting equation.
(x 14)
2
(4 x 7
)
2
x
2
28x 196 4
2
(x 7
)
2
x
2
28x 196 16(x 7)
x
2
28x 196 16x 112
x
2
44x 84 0
(x 2)(x 42) 0
x 2 0orx 42 0
x 2 x 42.
Substituting 2 and 42 in the left side of the original equation shows that
2
2
3
2 7
1
9
1 3 2;
2
42
3
42 7
81 49 9 7 2.
Therefore, 42 is the only solution of the equation. ■
Many radical equations are not amenable to algebraic techniques. In such
cases, graphical or numerical means must be used to approximate the solutions.
EXAMPLE 3
Solve
5
x
2
6
x 2
x 4.
SOLUTION If we raise both sides of the equation to the fifth power, we obtain
x
2
6x 2 (x 4)
5
.
Unfortunately, this equation is not readily solvable, even if we multiply out the
right hand side. The best we can do is to approximate the solutions. We can do this
graphically as follows: We rewrite the equation as
5
x
2
6
x 2
x 4 0,
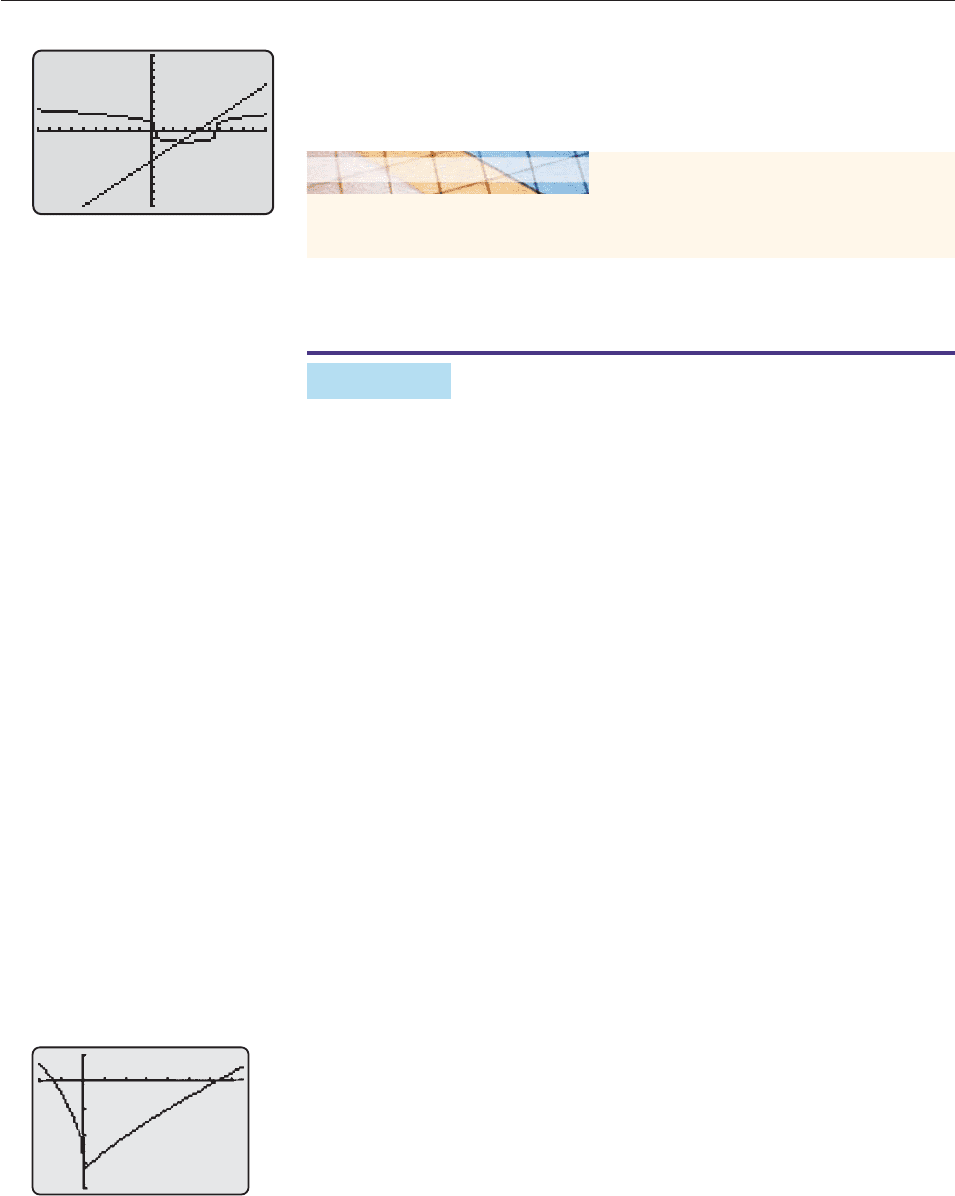
then the solutions are the x-intercepts of the graph of
h(x)
5
x
2
6
x 2
x 4
(see Figure 5–3). Alternatively, we can graph f (x)
5
x
2
6
x 2
and
g(x) x 4
on the same screen and find the x-coordinate of their intersection (Figure 5–4).
352 CHAPTER 5 Exponential and Logarithmic Functions
10
10
−10
−10
Figure 5–3
Equations involving rational exponents can be solved graphically, provided
that the function to be graphed is entered properly. Many such equations can also
be solved algebraically by making an appropriate substitution.
EXAMPLE 4
Solve x
2/3
2x
1/3
15 0 both algebraically and graphically.
SOLUTION
Algebraic:
Let u x
1/3
, rewrite the equation, and solve:
x
2/3
2x
1/3
15 0
(x
1/3
)
2
2x
1/3
15 0
u
2
2u 15 0
(u 3)(u 5) 0
u 3 0oru 5 0
u 3 u 5
x
1/3
3 x
1/3
5.
Cubing both sides of these last equations shows that
(x
1/3
)
3
(3)
3
or (x
1/3
)
3
5
3
x 27 x 125.
Since we cubed both sides, we must check these numbers in the original equation.
Verify that both are solutions.
Graphical: Graph the function f (x) x
2/3
2x
1/3
15 and find the
x-intercepts, namely, x 27 and x 125. The only difficulty is the one men-
tioned in the Note on page 344. Depending on your calculator, you might have to
enter the function f in one of these forms:
f (x) (x
2
)
1/3
2x
1/3
15 or f (x) (x
1/3
)
2
2x
1/3
15.
Otherwise, the calculator might not produce a graph when x is negative. Your
result should resemble Figure 5–5. ■
SPECIAL TOPICS 5.1.A Radical Equations 353
5
20
40 150
Figure 5–5
10
10
−10
−10
Figure 5–4
Use a root finder in Figure 5–3 or an intersection finder in Figure 5–4 to verify
that x 2.534 is the solution of the equation.
■
GRAPHING EXPLORATION
EXAMPLE 5
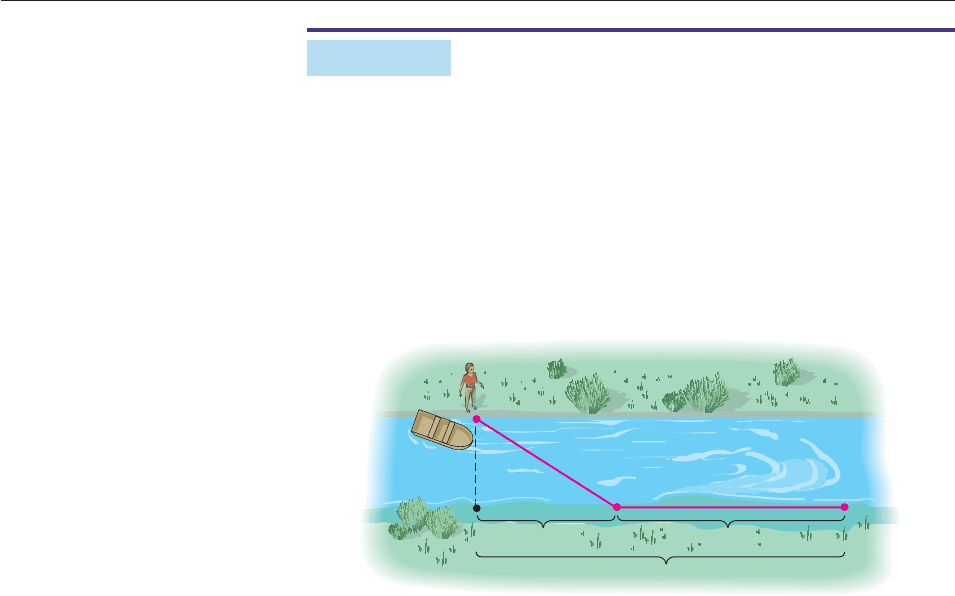
Hoa, who is standing at point A on the bank of a 2.5-kilometer-wide river
wants to reach point B, 15 kilometers downstream on the opposite bank. She
plans to row to a point C on the opposite shore and then run to B, as shown in
Figure 5–6. She can row at a rate of 4 kilometers per hour and can run at
8 kilometers per hour.
(a) If her trip took 3 hours, how far from B did she land?
(b) How far from B should she land to make the time for the trip as short as
possible?
Figure 5–6
SOLUTION Let x be the distance that Hoa ran from C to B. Using the basic for-
mula for distance, we have
Rate Time Distance
Time
Di
R
st
a
a
t
n
e
ce
8
x
.
Similarly, the time required to row distance d is
Time
Di
R
st
a
a
t
n
e
ce
d
4
.
Since 15 x is the distance from D to C, the Pythagorean Theorem applied to
right triangle ADC shows that
d
2
(15 x)
2
2.5
2
or, equivalently, d
(15
x)
2
6.25
.
Therefore, the total time for the trip is given by
T(x) Rowing time Running time
d
4
8
x
8
x
.
(a) If the trip took 3 hours, then T(x) 3, and we must solve the equation
8
x
3.
(x 1
5)
2
6.25
4
(x 1
5)
2
6.25
4
15 − x
x
15
2.5
C
d
B
D
A
354 CHAPTER 5 Exponential and Logarithmic Functions

This Graphing Exploration shows that Hoa should land approximately 6.74
kilometers from B to make the trip in 3 hours.
(b) To find the shortest possible time, we must find the value of x that makes
T(x)
8
x
as small as possible.
Therefore, the shortest time for the trip will be 2.42 hours and will occur if
Hoa rows to a point 13.56 kilometers from B. ■
(x 1
5)
2
6.25
4
SPECIAL TOPICS 5.1.A Radical Equations 355
Using the viewing window with 0 x 15 and 2 y 2, graph the function
f (x)
8
x
3
and use a root finder to find its x-intercept (the solution of the equation).
(x 1
5)
2
6.25
4
GRAPHING EXPLORATION
Using the viewing window with 0 x 15 and 0 y 4, graph T(x) and use a
minimum finder to verify that the lowest point on the graph (that is, the point with
the y-coordinate T(x) as small as possible) is approximately (13.56, 2.42).
GRAPHING EXPLORATION
EXERCISES 5.1.A
In Exercises 1–26, find all real solutions of each equation. Find
exact solutions when possible and approximate ones otherwise.
1. x 2
3 2. x 7
4
3. 4x 9
0 4. 4x 9
1
5.
3
5 11
x
3 6.
3
6x 1
0
2
7.
3
x
2
1
2 8. (x 2)
2/3
9
9.
x
2
x
1
1 10. x
2
5
x 4
2
11. x 7
x 5 12. x 5
x 1
13. (3x
2
7x 2)
1/2
x 1
14.
4x
2
10x
5
x 3
15. (x
3
x
2
4x 5)
1/3
x 1
16.
3
x
3
6
x
2
2
x 3
x 1
17.
5
9 x
2
x
2
1
18. (x
3
x 1)
1/4
x
2
1
19.
3
x
5
x
3
x
x 2
20.
x
3
2
x
2
1
x
3
2x 1
21. x
2
x
1
14 x
22.
3
x
3
3
x
3
3x
2
1
23. 5x 6
3 x 3
24. 3y 1
1 y 4
25. 2x 5
1 x 3
26. x 3
x 5
4
27. The surface area S of the right circular cone in the figure
is given by S pr
r
2
h
2
. What radius should be used
to produce a cone of height 5 inches and surface area
100 square inches?
h
r
