Houze Robert A., Jr. Cloud Dynamics
Подождите немного. Документ загружается.

(12.36)
12.2 Wave Clouds Produced by Long Ridges 519
fluid
"If containing a hydraulic jump, as idealized in Fig. 12.12. The volume "If is
bounded by
Xl
and X2 and y and y +
Lly
in a coordinate system that moves with the
discontinuity in fluid height. The flow is assumed to be homogeneous (i.e., of
constant density) and steady state in this moving coordinate system.
We first consider the mass continuity in the fluid containing thejump by averag-
ing (12.28) to obtain
Q ;:
iiH
= constant
(12.35)
Next,
we consider the conservation of momentum in the mass of fluid contained
in
"If. The equation of motion governing the mean fluid motion is obtained by
applying the averaging procedure described in Sec. 2.6 to (2.1), ignoring Coriolis
and molecular frictional forces and recalling that the density of an incompressible
fluid is constant. The result is
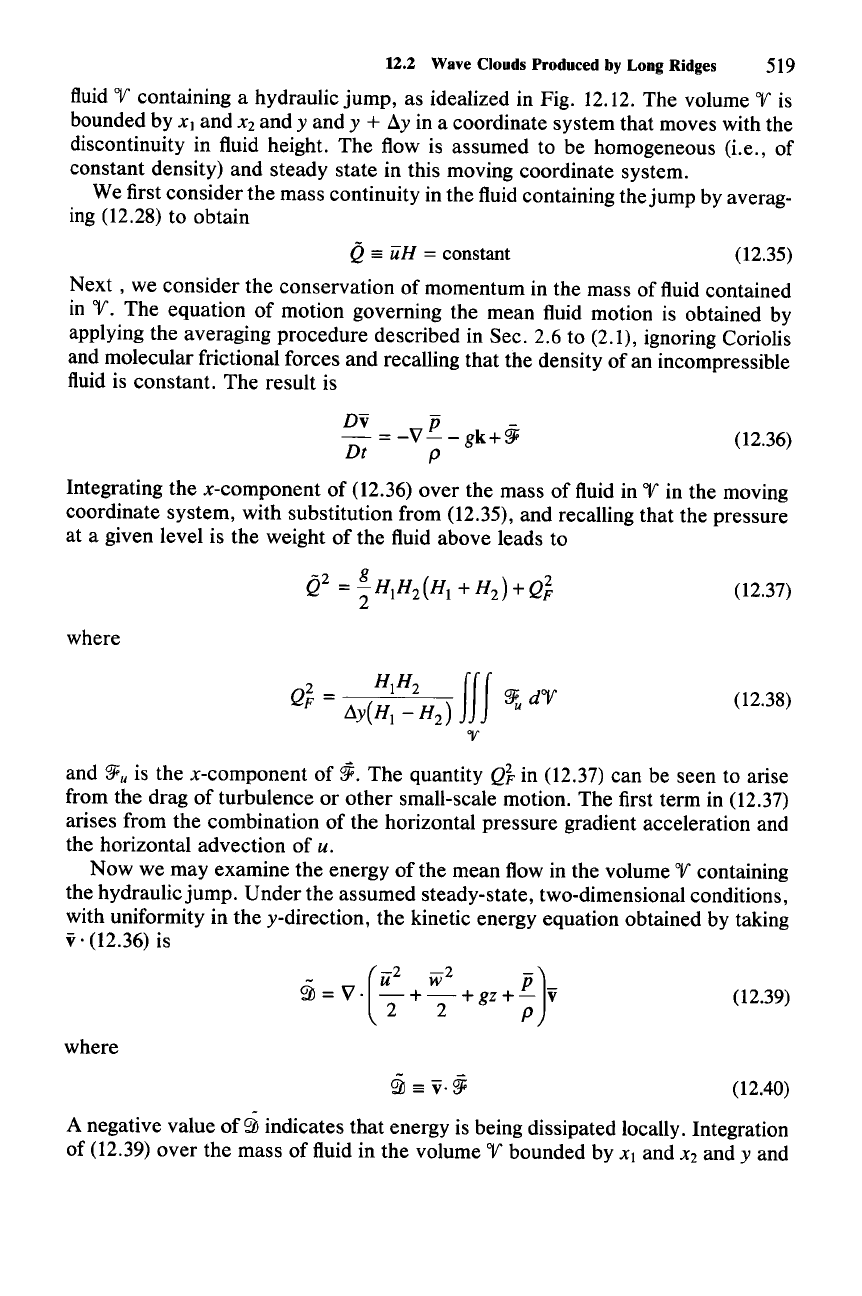
Dv P -
- =
-V--gk+~
Dr p
Integrating the x-component of (12.36) over the mass of fluid in "If in the moving
coordinate system, with substitution from (12.35), and recalling that the pressure
at a given level is the weight of the fluid above leads to
(12.37)
(12.38)
where
Q~
=
Lly(Z:~2H2)
III
?Jt
sv
"If
and
~u
is the x-component of
'#.
The quantity Q} in (12.37) can be seen to arise
from the drag of turbulence or other small-scale motion. The first term in (12.37)
arises from the combination of the horizontal pressure gradient acceleration and
the horizontal advection of u,
Now we may examine the energy of the mean flow in the volume
"If containing
the hydraulic jump. Under the assumed steady-state, two-dimensional conditions,
with uniformity in the y-direction, the kinetic energy equation obtained by taking
v·(12.36) is
(12.39)
where
(12.40)
-
A negative value of
q!J
indicates that energy is being dissipated locally. Integration
of (12.39) over the mass of fluid in the volume
"If bounded by
Xl
and X2 and y and

520 12 Orographic Clouds
y + Ay leads to
Iff
~p
dY
=
ff
PVn[~Z
+ :Z
+gz+
:)
dS
'V S
(12.41)
(12.42)
where
V
n
is the outward relative velocity component normal to the surface S
surrounding the volume Y.
If
the term on the right is negative, there is a net
convergence of energy into the fluid volume, and this convergence must be
matched by net dissipation in the volume in order to maintain the steady state.
Carrying out the integration on the right-hand side of (12.41), with substitution
from (12.35) and (12.37) and again letting the pressure be given by the weight of
the fluid above, leads to
Iff
- 3 pAyQQF
Z
(H
z
Z
-
H
1
Z
)
~p
d"lf = pAyQg(H
l
- Hz) _
4H
1H
z
2H1H1
'V
In the case represented in Fig. 12.12, Q> 0 and
HI
- Hz <
O.
The right-hand side
of (12.42) is therefore negative, indicating a net loss of energy in the volume
containing the jump. Moreover, (12.42) is still obtained even if the volume
"If is
made arbitrarily small by moving
XI
and Xz as close as we like to the jump in fluid
height. Thus, it is clear that all the energy loss occurs
at the jump. This result
implies that dissipative forces must be active in the vicinity of the jump in order to
conserve energy. Thus, (12.42) is a clear indication that some energy dissipation
must occur in the vicinity of the jump. The loss of energy at the jump is effected by
turbulence and/or downstream-propagating waves, which are allowed if the flow
to the right of the jump is subcritical.
It
is for this reason that rotor clouds located
at a hydraulic jump can be quite turbulent and are known by pilots to be a sign of
dangerous zones for light aircraft. Examples of spectacular rotor clouds were
shown in Figs. 1.23 and 1.24.
The primary dynamical characteristics of rotor clouds that can be explained by
the foregoing theory are the strong upward air motion that occurs at the hydraulic
jump and the strong turbulence or gravity wave motion required to accomplish the
dissipation of energy at the jump. There is some belief, especially from sail plane
pilots, that the air in the rotor clouds overturns around a horizontal axis normal to
the mean flow direction.
It
is from this aspect that the name rotor cloud is derived.
However, neither careful observation of rotor clouds nor model simulations have
yet confirmed whether or not such rotation is a predominant feature of the in-
cloud air motions.
12.3 Clouds Associated with Flow over Isolated Peaks
We have seen that small-amplitude wind perturbations produced by flow over a
long ridge take the form of vertically propagating and trapped lee waves. Some-
what similar responses occur in flow over an isolated mountain peak. However,

12.3 Clouds Associated with Flow over Isolated Peaks
521
parcel and wave motions are not restricted to only the x- and z-directions. Compo-
nents of motion in the y-direction can appear as the air flows around as well as
over the three-dimensional peak.
To illustrate this fact, we again consider the vorticity about the y-axis generated
as a basic unidirectional horizontal flow encounters and passes over the mountain.
We neglect Coriolis effects and use the steady-state form of the three-dimensional
Boussinesq y-vorticity equation (2.58)
(12.43)
which states that the advection of g is balanced by baroclinic generation
(-B
x
),
stretching gv
y,
and tilting
(~vz
+
7JVx)'
We will concern ourselves first with small-
amplitude motions by linearizing this equation about a purely horizontal, unidirec-
tional mean wind
u(z) and mean lapse rate e; To illustrate the essential physics
retained under linear conditions, we first linearize the right-hand side of (12.43).
The result is
(12.44)
This relation differs from the two-dimensional form (12.3) in that the stretching of
vorticity contained in the basic-state shear by perturbations lateral to the basic-
state current
(uzv
v
)
now appears on the right-hand side, along with the baroclinic
generation of
g associated with air moving up and down adiabatically and the
advection of
gby the y-component of motion (vg
y)
on the left-hand side. If we now
linearize the left-hand side of the equation and substitute from the perturbation
form of the three-dimensional Boussinesq continuity equation (2.55), we obtain,
after some rearrangement,
(12.45)
The only differences between this result and the two-dimensional case [cf. Eq.
(12.4)] are the first and last terms, which involve the perturbation of the flow
lateral to the basic current.
Since the variable
v is now involved, a further physical relationship is required
to close the system of equations.
For
this, we turn to the vertical vorticity equa-
tion (2.59), which under the present steady-state linearized conditions with no
Coriolis effects reduces to
(12.46)
which says that the horizontal advection of vertical vorticity by the basic-state
flow is
just
offset by the tilting of the base-state shear by y-variability of w. This
relation may be rewritten as
(12.47)
522 12 Orographic Clouds
Taking the horizontal Laplacian
V'iJ
=a
2
/
ax
2
+ a
2
/
a
y
2 of (12.45), making use
ofthe
mass continuity equation (2.55), and substituting from (12.47) leads to
(12.48)
where
V'
is the three-dimensional Laplacian. As in the two-dimensional case
(12.4), this vorticity equation has solutions of the forms of evanescent, vertically
propagating and trapped waves. However, the presence of the perturbation mo-
tions lateral to the basic current leads to the waves being distorted into interesting
configurations, which can affect the forms of clouds that develop in the waves.
The vertically propagating modes can be obtained by further simplifying the
problem such that
it does not vary with height. In this case, the Scorer parameter
given by (12.5) reduces to
(12.49)
and (12.48) becomes
which has solutions of the form
w =
w/(kx+
jy+mz)
with the following relation among k,
j,
and m:
2 k
2
+
j2
(2
2)
m = 2
£-k
k
(12.50)
(12.51)
(12.52)
which is similar to (12.7), except for the factor
(k
2
+ j2)/k
2
•
Thus, m
2
depends
onj
as well as k.
From (12.52) it is apparent that, as in the two-dimensional case, vertically
propagating or evanescent waves occur depending on whether
e
2
> k
2
or e
2
< k
2
,
respectively. As in the two-dimensional case, we can proceed by assuming some
specific form for the surface topography and disallowing downward energy propa-
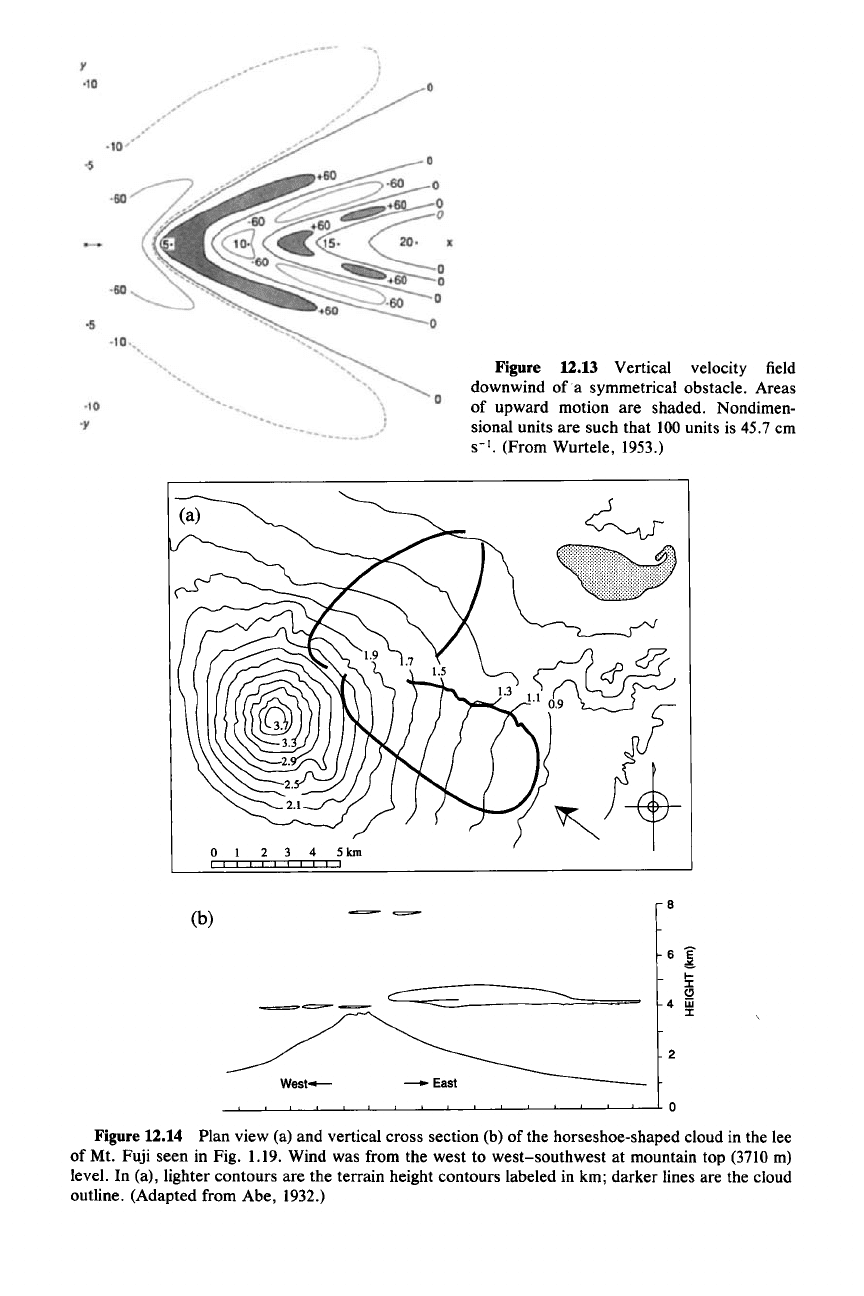
gation at the top boundary. Figure 12.13 shows an example of the vertical motion
pattern associated with steady, constant
B"
and constant it flow over a wide (e >
k) mountain. Alternating boomerang-shaped regions of upward and downward
motion occur downwind of the peak. This pattern of vertical motion occurs even if
the mountain is wide enough that the flow response is hydrostatic. That is, in the
three-dimensional hydrostatic case, the wave disturbance is not confined to the
region directly over the mountain. In this vertical motion pattern, a boomerang- or
horseshoe-shaped cloud can be observed in the lee of the mountain. The example
(a)
o
, I
(b)
2 3 4
5km
I I ! I I I I
Figure 12.13 Vertical velocity field
downwind
ofa
symmetrical obstacle. Areas
of upward motion are shaded. Nondimen-
sional units are such that 100units is 45.7 em
S-I.
(From Wurtele, 1953.)
8
2
West-
-East
Figure 12.14 Plan view (a) and vertical cross section (b) of the horseshoe-shaped cloud in the lee
of Mt. Fuji seen in Fig. 1.19. Wind was from the west to west-southwest at mountain top (3710 m)
level. In (a), lighter contours are the terrain height contours labeled in km; darker lines are the cloud
outline. (Adapted from Abe, 1932.)
524 12 Orographic Clouds
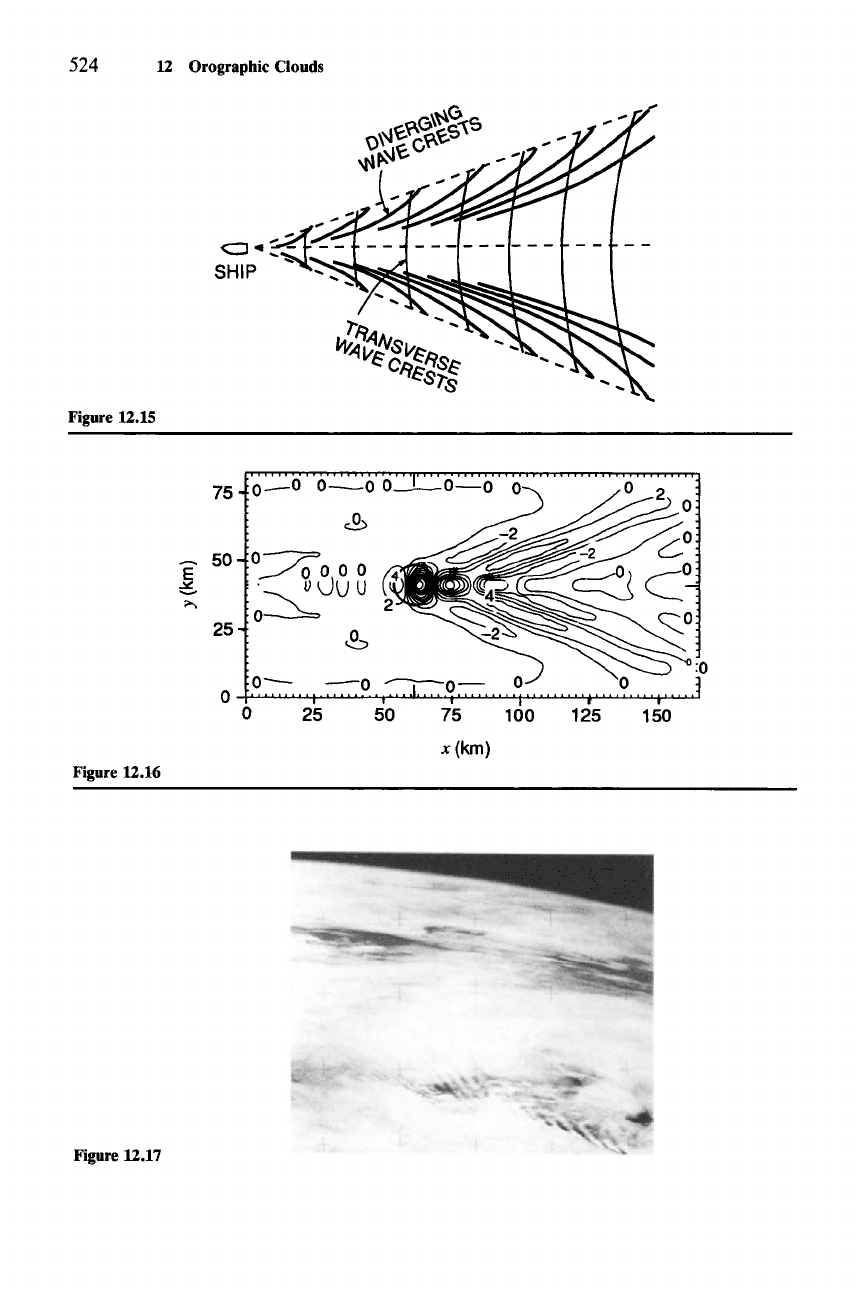
Figure 12.15
150
125100
0-
-0
..-----0-
o 25 50 75
x (km)
~
50
O~O
0 0 4
~
~~()UU
25
~
Figure 12.16
Figure 12.17

12.3 Clouds Associated with Flow over Isolated Peaks 525
shown photographically in Fig. 1.19 was studied
and
mapped photogrammetri-
cally as
shown
in Fig. 12.14.
327
The
vertical motion field in Fig. 12.13 is
not
the only form
that
mountain waves,
and
hence
wave
clouds,
can
take
downstream
of
an isolated peak. So far we have
only
considered
the
simplified
case
of
constant
ii
and
Hz
and hence
constant
£2.
The
wave
trapping
that
occurs
when
ii varies with height while
Hz
is
constant
has
been
studied-"
by examining
the
case
where
ii varies exponentially with height,
such
that
Ii =U e
z
/
L
o
(12.53)
where
U;
is
the
mean
wind at
the
ground
and
t: is the wind scale height.
It
is found
that
even
a slight wind
shear
is associated with a considerable amount of trap-
ping.F?
The
trapped
waves
that
develop in
the
flow
past
a mountain peak are
analogous to
waves
produced
by a ship.
These
ship waves
appear
as distinct
modes
ofthe
solution to (12.48),
when
the
full expression (12.5) is used for
£2,
and
iizz
is
determined
from
(12.53).
These
modes
are
of
two distinct types, as illus-
trated in Fig. 12.15.
Inside
a wedge-shaped
zone
downwind
of
the peak, there are
transverse
waves,
which
lie
more
or
less perpendicular to the basic-state flow, and
diverging
waves,
with
crests
that
meet
the
incoming flow at a small angle. Outside
the wedge, only diverging modes exist.
330
The
transverse
waves
are
analogous to the
trapped
lee waves that
occur
in two-
dimensional flow.
They
consist
of
superpositions
of
waves that have attempted to
327 The detailed photogrammetric study by Abe
(1932)
of a horseshoe-shaped cloud in the lee of Mt.
Fuji in Japan helped motivate Wurtele's (1957) study of the three-dimensional linear dynamics of the
flow over isolated three-dimensional mountain peaks. Wurtele's solution of (12.50), shown in Fig.
12.12, was meant to explain Abe's photographs.
328 By Sharman and Wurtele (1983).
329 This exponential representation of the wind actually has the property that £2
-+
- L
-2
as z
-+
00,
so that every wavelength is ultimately trapped.
330 For further discussion of these wave types, see Smith
(1979)
and Sharman and Wurtele
(1983).
Figure 12.15 Horizontal configuration of transverse and diverging phase lines for deep-water
"ship waves." (From Sharman and Wurtele, 1983. Research sponsored by NASA Dryden FRF,
L. J.
Ehernberger, monitor. Reprinted with permission from the American Meteorological Society.)
Figure 12.16 Numerical-model simulation of highly sheared flow over a 300-500-m-high obstacle.
Vertical velocity at the 5-km level is shown (in 2 cm
S-I
contour intervals) after the flow has reached a
steady state. Each tick mark on the frame enclosing the pattern indicates the location of a horizontal
grid point (2.5 km). The obstacle is centered at the long tick marks. The contour representing the
obstacle shape is shown as the heavy circle; the contour shown is the one for which the obstacle height
is 0.1 the maximum height. (From Sharman and Wurtele, 1983.Research sponsored by NASA Dryden
FRF,
L.
J. Ehernberger, monitor. Reprinted with permission from the American Meteorological
Society.)
Figure 12.17 Example of clouds associated with diverging wave modes in the lee of Bouvet
Island. The photo was taken by
Skylab astronauts (photo reference number: SL4-137-3632). The exact
scale of the picture is not known. Numerical simulations of this type of wave structure suggest that the
crests are
-10-40
km apart.

526 12 Orographic Clouds
propagate upstream but have been advected to the lee. The diverging waves have
not attempted to propagate upstream but instead have propagated laterally away
from the mountain while being advected to the lee by the basic current. Numerical
model experiments for flow over a 300-500-m-high obstacle have been performed
with the full set of time-dependent equations for a range of wind shear, indicated
by the ratio
R =
Nil
U«, where N is given by (2.98). Since N is constant, the range
of
Ris determined entirely by the shear, as measured by the wind scale height L.
We will examine results, after the flow reaches a steady state, for three values
of
R.
Results at low R (high shear) are shown in Fig. 12.16. Here only diverging
modes exist. Strong motions are organized in bands forming a pattern similar to
that of the idealized diverging wave crests in Fig. 12.15. The strong vertical
motions are concentrated on the edges of the wedge, with relatively little distur-
bance directly downstream of the mountain in the center of the wedge. Figure
12.17 shows an example of clouds associated with diverging wave modes.
Figure 12.18 shows results at moderate
R. In this case, both types of waves
occur in the wedge-shaped region downwind of the mountain. Only diverging
waves exist outside the wedge. Response at this particular
R includes a strong
transverse pattern across the wedge. These waves turn into diverging structure
outside the wedge. The effect of trapping in the case of the transverse waves is
evident by the series of waves downstream, which resemble lee waves downwind
of a two-dimensional ridge, and by the fact that the wave amplitude rapidly dies
out with increasing altitude (cf. Fig. 12.18a and b). Figure 12.19 shows an example
of clouds associated with predominantly transverse waves.
The case of infinite
R reduces to the no-shear
(if
= constant) case examined
previously. Figure 12.20illustrates this case, in which there is no trapping, and the
horseshoe-shaped vertical velocity pattern is again seen downwind. This pattern
is not readily distinguishable from that of moderate
R (cf. Fig. 12.18).
If
horse-
shoe-shaped clouds are observed to the lee of a mountain peak, they are probably
associated with trapped waves, since the calculations show that even small shear
produces significant trapping.
So far, we have considered only small-amplitude (linear) perturbations pro-
duced in flow over a three-dimensional mountain peak. In the two-dimensional
case, we saw that in the case of shallow-water flow over an obstacle of finite
height, the flow is blocked whenever
Fr
2
[defined by (12.29)] is sufficiently small.
A similar tendency is found in continuously stratified fluid if
~
A2 « 1 (12.54)
N h
m
where h
m
is the maximum height of the mountain. The condition (12.54) is met if
the fluid is either moving slowly, extremely buoyantly stable, or both. If the
obstacle is a three-dimensional peak, rather than a two-dimensional ridge, then
instead of being blocked, the flow turns laterally and finds a way around the
mountain.
It
can be shown''" that in the case of (12.54)the flow reduces to a purely
331 See Durran (1990) for further discussion and references.
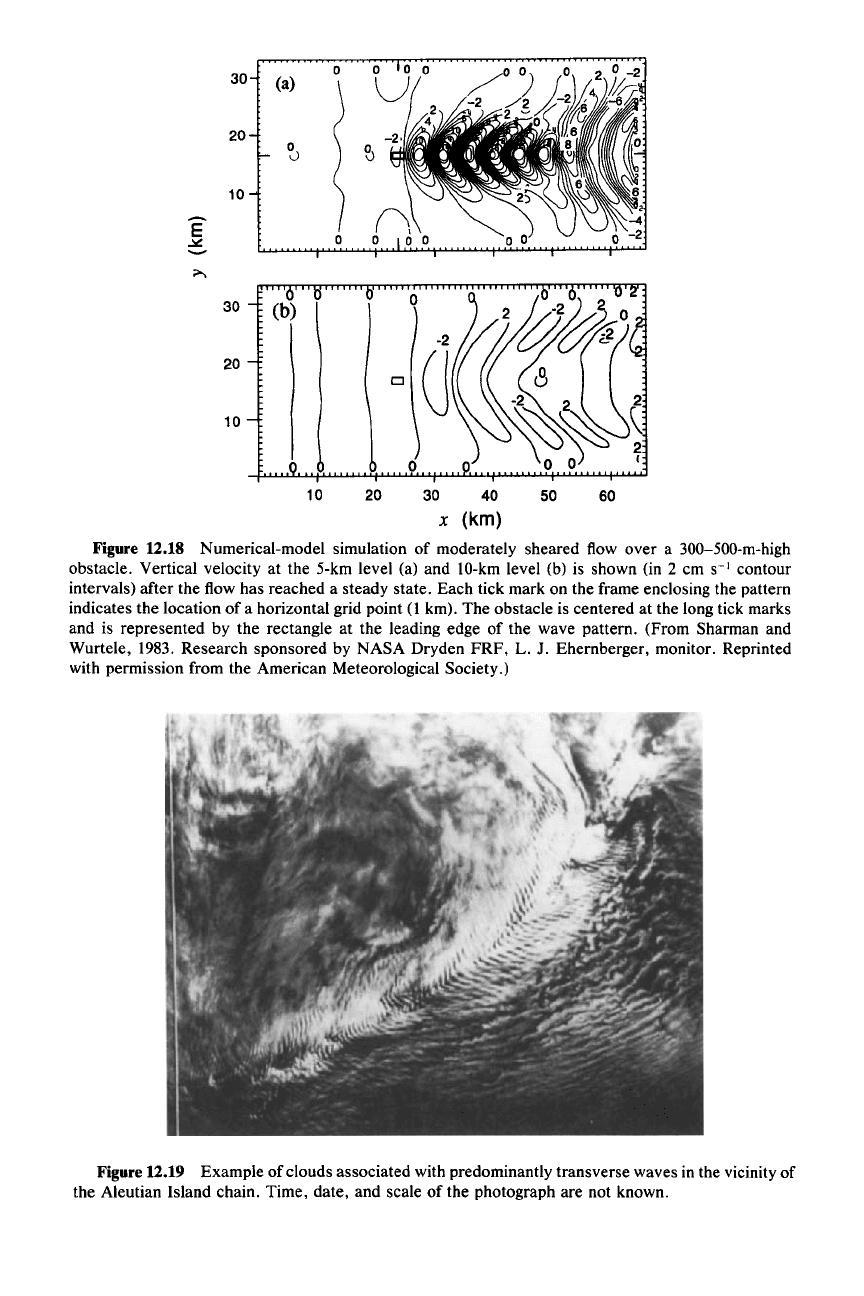
30
0
(a)
20
~
~
10
-
E
0
~
-
;>-,
30
20
10
6050
2010 30 40
x (km)
Figure
U.18
Numerical-model simulation of moderately sheared flow over a 300-500-m-high
obstacle. Vertical velocity at the 5-km level (a) and 10-km level (b) is shown (in 2 em
S-I
contour
intervals) after the flow has reached a steady state. Each tick mark on the frame enclosing the pattern
indicates the location of a horizontal grid point
(l
km). The obstacle is centered at the long tick marks
and is represented by the rectangle at the leading edge of the wave pattern. (From Sharman and
Wurtele, 1983. Research sponsored by NASA Dryden FRF,
L. J. Ehernberger, monitor. Reprinted
with permission from the American Meteorological Society.)
Figure
U.19
Example of clouds associated with predominantly transverse waves in the vicinity of
the Aleutian Island chain. Time, date, and scale of the photograph are not known.
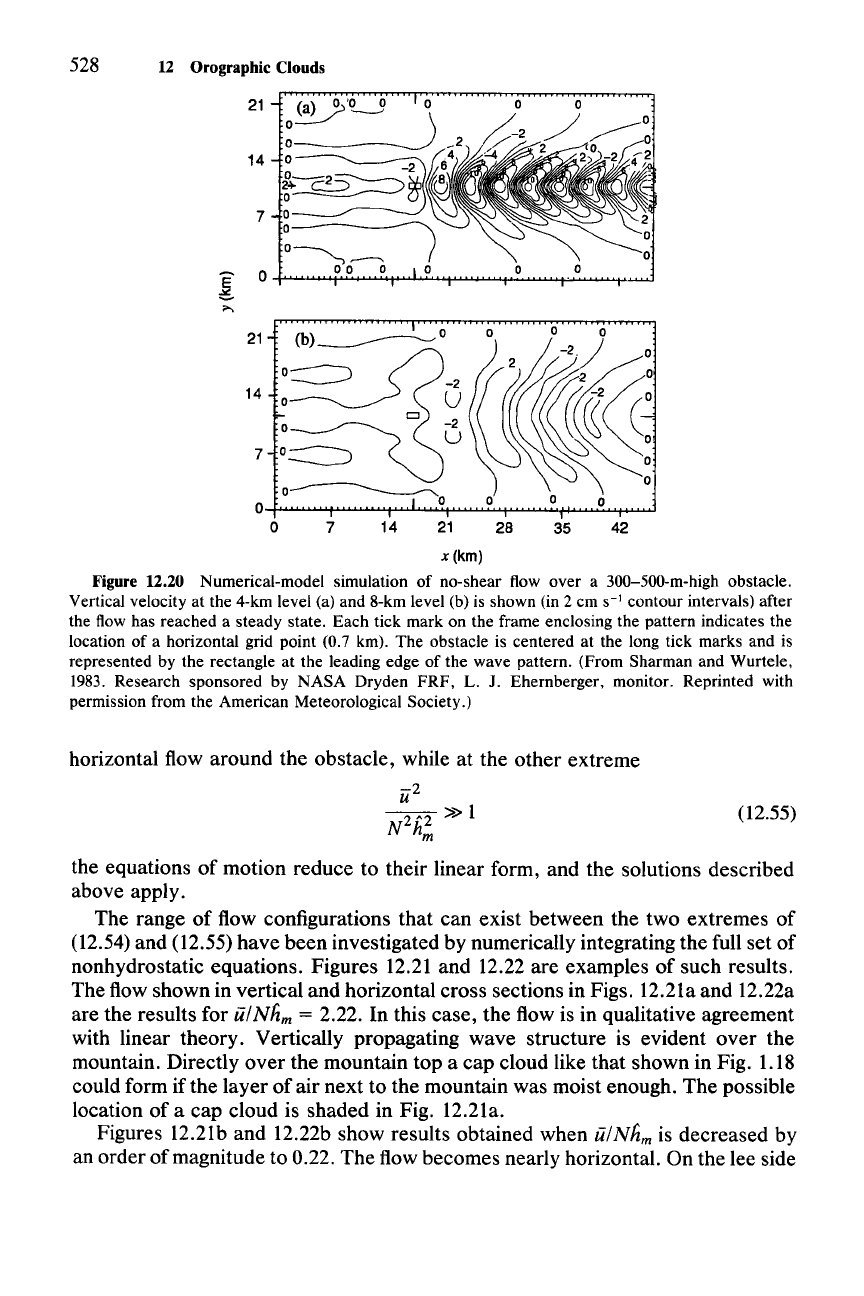
528
12
Orographic Clouds
21
14
7
0
!:
0
0
0
..,
21
O~
14
0
0
7
o~
O-f'-'~""""'t~~'t"'-'-'.L..u4""~.u:.....t'""'"'-~"T'-'''''''''';'4..............J
o 7 14 21 28 35 42
x (km)
Figure 12.20 Numerical-model simulation of no-shear flow over a 300-500-m-high obstacle.
Vertical velocity at the 4-km level (a) and 8-km level (b) is shown (in 2 em
S-l
contour intervals) after
the flow has reached a steady state. Each tick mark on the frame enclosing the pattern indicates the
location of a horizontal grid point (0.7 km). The obstacle is centered at the long tick marks and is
represented by the rectangle at the leading edge of the wave pattern. (From Sharman and Wurtele,
1983. Research sponsored by NASA Dryden
FRF,
L. J. Ehernberger, monitor. Reprinted with
permission from the American Meteorological Society.)
(12.55)
horizontal flow around the obstacle, while at the other extreme
u
2
2'T»
1
N h
m
the equations of motion reduce to their linear form, and the solutions described
above apply.
The range of flow configurations that can exist between the two extremes of
(12.54) and (12.55)have been investigated by numerically integrating the full set of
nonhydrostatic equations. Figures 12.21 and 12.22 are examples of such results.
The flow shown in vertical and horizontal cross sections in Figs. 12.21aand 12.22a
are the results for
iii
ss:
= 2.22. In this case, the flow is in qualitative agreement
with linear theory. Vertically propagating wave structure is evident over the
mountain. Directly over the mountain top a cap cloud like that shown in Fig. 1.18
could form if the layer of air next to the mountain was moist enough. The possible
location of a cap cloud is shaded in Fig. 12.21a.
Figures 12.21b and 12.22b show results obtained when
iilNh.
m
is decreased by
an order of magnitude to 0.22. The flow becomes nearly horizontal. On the lee side
