Heitjans P., Karger J. (Eds.). Diffusion in Condensed Matter: Methods, Materials, Models

Подождите немного. Документ загружается.

1 Diﬀusion: Introduction and Case Studies in Metals and Binary Alloys 39

are small relative to the vacancy formation enthalpies, which are in the order

of 1 eV [40]. Using ∆H = H

+ H

we get for the diﬀerence of the activation

enthalpies between solute- and self-diﬀusion

∆Q = ∆H

− ∆H = −H

+(H

− H

) − C. (1.73)

A useful theoretical approach associates ∆Q with the charge diﬀerence be-

tween solute and vacancy. Positive solutes, generally those of higher nominal

valence than the solvent, tend to have a net attraction with the vacancies.

Such solutes diﬀuse more rapidly and with lower activation energies than

self-diﬀusion. Calculations of ∆Q from a theory based on the free electron

model using the Thomas-Fermi approximation for the interaction potential

have been made, e. g., by LeClaire [52]. Good agreement was found with ex-

periments for solute diﬀusion in noble metals and zinc. ∆Q is negative and

its absolute value increases with the diﬀerence in valence between the solvent

and the solute. For transition-metal solutes in noble metals and for other sol-

vents such as the alkali metals, divalent magnesium, and trivalent aluminium

the calculated values of ∆Q do not agree with the experiment.

1.9.2 Slow Diﬀusion of Transition-Metal Solutes in Aluminium

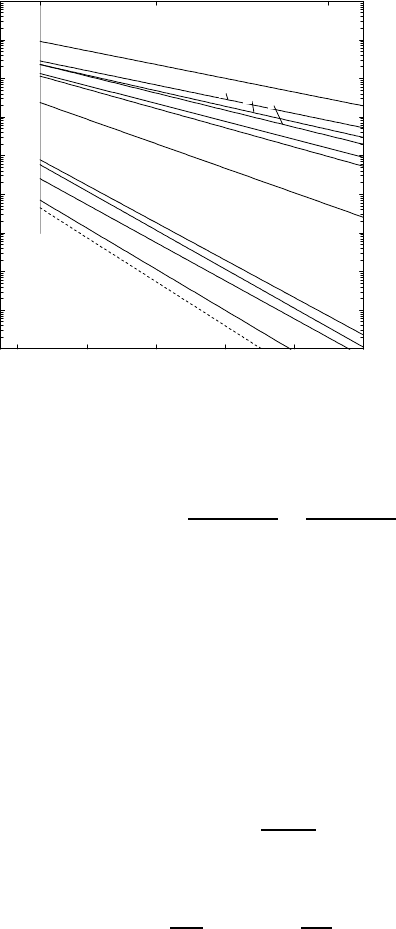
Fig. 1.20 shows an Arrhenius diagram of various solutes in aluminium to-

gether with aluminium self-diﬀusion according to [53]. The transition ele-

-18

-17

-16

-15

-14

-13

-12

-11

-10

10 11 12

13 14 15

16 17

900 800 700 600

=933

-1

/ 10

-4

-1

D / m

-1

T / K

Fig. 1.20. Diﬀusion of several solutes and self-diﬀusion (dashed line) in aluminium.

40 Helmut Mehrer

ments are diﬀusers with high activation energies and high pre-exponential fac-

tors. Most of them have extremely low diﬀusivities as compared to aluminium

self-diﬀusion. In contrast, non-transition elements have diﬀusion rates similar

or slightly higher than self-diﬀusion and show only small diﬀusivity disper-

sion.

The pressure dependence of the solute diﬀusion coeﬃcients in aluminium

has been studied in [35,54]. The activation volumes of non-transition element

solutediﬀusersareclosetooneatomicvolume(Ω) and not much diﬀerent

from the activation volume of self-diﬀusion. However, the transition elements

are diﬀusers with high activation volumes between 1.67 and 2.7 Ω.These

ﬁndings can be attributed to diﬀerences in vacancy-solute interaction in alu-

minium between transition and non-transition element solutes [54]. The large

∆H

values of transition element solutes according to (1.67) indicate a strong

repulsion between solute and vacancy (H

< 0) and/or a large activation en-

thalpy H

for the solute-vacancy exchange jump.

1.9.3 Fast Solute Diﬀusion in ‘Open’ Metals

Fast solute diﬀusion is observed in some polyvalent metals, which are some-

times also denoted as ‘open’ metals [55]. ’Open’ refers to the large ratio

between atomic and ionic radius of the solvent. This solvent property leads

for solutes with relatively small radii to the occurrence of fast solute diﬀusion.

As an example, Fig. 1.21 shows an Arrhenius diagram of solutes in lead to-

gether with lead self-diﬀusion (for references see Chap. 3 in [6]). Some solutes

(thallium, tin) in lead show ‘normal’ behaviour. However, noble metals, nickel

group solutes, and zinc have diﬀusivities which are three or more orders of

magnitude faster than self-diﬀusion.

Noble metal solutes are also fast diﬀusers in the group IVB metal tin

and in the group IIIB metals indium and thallium. The late transition el-

ements Fe, Co, and Ni in group IVA metals (α-titanium, α-zirconium, and

α-hafnium), and Co in niobium are very fast diﬀusers as well [55,56].

Fast solute diﬀusion in metals has been attributed to the dissociative

mechanism (see, e.g., [57]). This mechanism operates for solutes which are

incorporated not only on substitutional sites but also to some extend in

interstitial sites of the solvent metal (see Sect. 1.6). According to (1.47) the

dissociative reaction involves vacancies. Provided that local equilibrium is

established, the concentrations of the three involved species must fulﬁll the

law of mass action

= K(T )=

, (1.74)

where C

, C

,andC

denote molar fractions of interstitial solute, substitu-

tional solute, and vacancies. K(T ) is a constant which depends on tempera-

ture and the superscript eq denotes thermal equilibrium.

A metal crystal with a normal density of dislocations has a suﬃcient

abundance of vacancy sources or sinks to keep the vacancies everywhere in

1 Diﬀusion: Introduction and Case Studies in Metals and Binary Alloys 41

-17

-16

-15

-14

-13

-12

-11

-10

-9

-8

16 18 20

22 24

600 500

400

= 601

-1

/ 10

-4

-1

D / m

-1

T / K

Fig. 1.21. Diﬀusion

of several solutes and

self-diﬀusion (dashed

line) in lead.

equilibrium. Then the eﬀective diﬀusivity of solutes is given by [41, 57]

eﬀ

+ C

, (1.75)

where D

denotes the diﬀusivity of the solute in its interstitial state and D

its vacancy-mediated diﬀusivity on substitutional sites.

For solutes with dominating interstitial solubility and diﬀusivity (C



and D

 D

), (1.75) reduces to the trivial case of interstitial diﬀusion

eﬀ

≈ D

. (1.76)

For so-called hybrid solutes the substitutional solubility dominates (C



) but the interstitial diﬀusivity is much faster than the substitutional one

> D

). Then the eﬀective diﬀusivity (1.75) approaches

eﬀ

≈

. (1.77)

This relation contains the factor

=exp



−



, (1.78)

where G

denotes the Gibbs free energy diﬀerence between the interstitial and

substitutional positions of the solute. The rather wide ‘diﬀusivity dispersion’

of fast solute diﬀusers can be largely attributed to this factor.

42 Helmut Mehrer

Fast diﬀusion of solutes is well known for the semiconducting elements sil-

icon and germanium [41,58,59] (see also Chap. 4). It has been also attributed

to interstitial-substitutional exchange mechanisms. The kick-out mechanism

is dominating diﬀusion of Au, Pt, and Zn in silicon [41,42], whereas the disso-

ciative mechanism is operating, e. g., for Cu in germanium [60]. From a chem-

ical viewpoint these similarities are not surprising. Silicon and germanium are

group IV elements such as the ‘open’ metals lead and tin. Fast diﬀusion is

also observed for compound semiconductors (e. g. Zn in GaAs [61]). Actually,

the concepts growing out from studies of fast diﬀusion in semiconductors

(see Chap. 4) have strongly inﬂuenced the interpretation of fast diﬀusion in

metals.

1.10 Self-Diﬀusion in Binary Intermetallics

Some intermetallics (intermetallic compounds or ordered alloys) have at-

tracted much attention as technological materials for high-temperature ap-

plications. A knowledge of their diﬀusion behaviour is of interest for the

production of these materials and for their use in technological applications.

Whereas diﬀusion in many pure metals and dilute alloys is thoroughly in-

vestigated and reasonably well understood, systematic diﬀusion studies for

intermetallics are still relatively scarce although considerable progress has

been achieved in recent years [62–67].

An atomistic understanding of diﬀusion in intermetallics in terms of de-

fect structure and diﬀusion mechanisms is obviously more complex than for

metallic elements:

Intermetallics crystallize in a variety of structures with ordered atom dis-

tributions. Examples are the B2-, D0

-, L1

-, D0

-, B20-, and Laves-

phase structures. Some intermetallics are ordered up to the melting temper-

ature, others undergo order-disorder transitions because entropy favours a

less ordered or even a random arrangement of atoms at high temperatures.

We know intermetallic phases with wide phase ﬁelds and others which exist

as line compounds. Some intermetallics occur for certain stoichiometric com-

positions, others are observed for oﬀ-stoichiometric compositions only. Some

phases compensate oﬀ-stoichiometry by vacancies others by antisite atoms.

Let us concentrate on the more common cubic intermetallics. Their struc-

tures are the following (see Fig. 1.22):

B2 (or CsCl) Structure: The approximate composition is AB. The

B2 structure can be derived from the bcc lattice, if the two primitive cubic

sublattices are occupied by diﬀerent kinds of atoms. Examples are FeAl,

CoAl, NiAl, CoGa, PdIn, CuZn, AuZn, and AuCd.

(or Fe

Si) Structure: The approximate composition is A

B. The

-structure can also be considered as an ordered structure derived from

the bcc lattice. In Fig. 1.22 (middle) A-atoms occupy white and grey sites,

1 Diﬀusion: Introduction and Case Studies in Metals and Binary Alloys 43

Fig. 1.22. Some frequent structures of intermetallics: B2 (left), D0

(middle), L1

(right).

B-atoms occupy black sites. D0

order is observed for example in Fe

Si, in

Al below about 825 K, and for some high-temperature phases.

(or Cu

Au) Structure: The approximate composition is A

B. The

-structure is an ordered structure in the fcc lattice. In Fig. 1.22 (right)

A-atoms occupy white sites, B-atoms occupy black sites . Examples are the

Ni-based compounds Ni

Al, Ni

Ga, and Ni

Ge.

Self-diﬀusion is the most basic diﬀusion process also in alloys and com-

pounds. Like in the case of pure metals studies of self-diﬀusion utilize such

tiny amounts of tracer atoms (see Sect. 1.4) of the diﬀusing species that the

chemical composition of the sample does practically not change due to dif-

fusion. In a binary system two tracer self-diﬀusion coeﬃcients – one for A

atoms and another one for B atoms – can be determined.

Table 1.4 compiles those binary intermetallics with B2, D0

,andL1

structures for which self-diﬀusion data are available. In some cases self-

diﬀusion of both components has indeed been studied. For NiAl and FeAl

impurity diﬀusion of some solutes has been studied, since no appropriate Al

tracer is available. Also interdiﬀusion studies were used to deduce the Al

diﬀusivity via the Darken-Manning equation (see Sect. 1.11) from the in-

terdiﬀusion coeﬃcient, the tracer diﬀusivity of the other alloy constituent,

and the thermodynamic factor (see, e. g., [68]). In what follows it may suﬃce

to illustrate some characteristic features of self-diﬀusion in intermetallics. A

comprehensive discussion of all aspects of self-diﬀusion is beyond the scope

of this chapter. For further information the reader is referred to several

overviews [62–66,69].

1.10.1 Inﬂuence of Order-Disorder Transition

An order-disorder transition occurs, for example, between the β-andβ



-brass

phases of the Cu-Zn system. Below the order-disorder transition (at about

741 K) the compound shows B2 order (β



brass). At high temperatures the

44 Helmut Mehrer

Table 1.4. Self-diﬀusion in B2, D0

,andL1

structure intermetallics. For the

underlined components tracer self-diﬀusion data or data of suitable substitutional

substitutes (in brackets) are available

Structure Intermetallic

B2 CuZn,AuCd,AuZn,CoGa,PdIn,FeCo,NiAl(Ga),

Al(Zn,In,Cr,Mn,Ni,Co)AgMg,NiGa

Al, Ni

Ge,Ni

Ga,Co

Ti, Pt

Mn,Cu

Au (disordered)

Si(Ge), Cu

Sn,Cu

Sb, Ni

Sb, Fe

disordered A2 structure (β brass) is formed. The beautiful pioneering work

of Kuper et al. [70] on self-diﬀusion of

Cu and

Zn in CuZn is displayed

in Fig. 1.23. The inﬂuence of the order-disorder transition on the diﬀusion

behaviour of both components is visible as a change in slope of the Arrhenius

plot. The activation energies obey the inequality

. (1.79)

The occurrence of order impedes the diﬀusion of both components in a similar

way. Similar eﬀects of the B2-A2 transition have been observed for diﬀusion

in FeCo.

Recently Fe diﬀusion in Fe

Al has been studied over a wide temperature

range [21]. Fe

Al undergoes two order-disorder transitions from D0

order

at low temperatures to B2 order at elevated temperatures to the completely

disordered A2 structure at high temperatures. At the critical temperatures

the slope of the Arrhenius diagram changes and the activation energies obey

the following sequence

. (1.80)

The activation energy is highest for the structure with the highest degree

of order and lowest for the disordered structure. The eﬀect is, however, less

pronounced in Fe

Al than in CuZn.

1.10.2 Coupled Diﬀusion in B2 Intermetallics

Fig. 1.23 reveals another remarkable feature of self-diﬀusion in B2 inter-

metallics. We recognize that Zn in β-brass diﬀuses only slightly faster than

Cu and that the ratio D

never exceeds 2.3 [70]. For equiatomic FeCo

For references see [63–65].

1 Diﬀusion: Introduction and Case Studies in Metals and Binary Alloys 45

-17

-16

-15

-14

-13

-12

-11

-10

10 11 12 13 14

15 16

17 18

1000

900 800 700 600

468°

CsCl

bcc

CuZn

CuZ

-1

/ 10

-4

-1

D / m

-1

T / K

Fig. 1.23. Self-

diﬀusion of

Cu and

Zn in CuZn.

the ratio D

is always close to unity [71]. This type of ‘coupling’ be-

tween the diﬀusivities of the components seems to be typical of B2 phases.

It can be observed in Fig. 1.24 for practically all B2 compounds, for which

both constituents have been investigated. In some cases (e.g. NiGa, CoGa)

the bounds for D

∗

are somewhat wider than in the cases of CuZn and

FeCo. However, the diﬀerence between the diﬀusivities is less than one or-

der of magnitude. This ‘coupling’ between the diﬀusivities of the components

indicates that the diﬀusion of both atomic species is likely mediated by the

same defect.

As already mentioned the B2 structure consists of two primitive cubic

sublattices. In the completely ordered state of a stoichiometric B2 compound,

A atoms occupy one sublattice and B atoms the other. This implies that

each A atom is surrounded by 8 B atoms on nearest-neighbour sites and vice

versa. If the A and B atoms are distributed at random, a body-centered cubic

(bcc) structure (A2 structure) is obtained. When atomic diﬀusion in a highly

B2 ordered compound would take place by a random interchange between

vacancies and atoms via nearest-neighbour jumps, migrating vacancies would

leave traces of antisite defects (A

and B

) behind. In order to maintain order

such disordered regions must either be avoided or compensated during the

diﬀusion process.

If the order energy is high and the degree of order close to unity sublattice

diﬀusion of each component via second nearest-neighbour jumps is conceiv-

able. It is well known that diﬀusion of the components in ionic crystals and in

simple oxides occurs indeed by sublattice diﬀusion (see Chap. 5 and, e. g., [2]).

46 Helmut Mehrer

-21

-20

-19

-18

-17

-16

-15

-14

-13

-12

-11

-10

7 8

9 10

11 12 13 14 15 16

17 18 19

1300 1100 900

800 700 600

FeC

FeCo

NiAl

AgMg

eAl

NiGa

AgMg

PdIn C

uZn

CoG

CoGa

PdIn

uZn

-1

/ 10

-4

-1

D / m

-1

T / K

Fig. 1.24. Self-

diﬀusion in B2 struc-

ture intermetallics.

However, sublattice diﬀusion cannot be the dominating mechanism in B2 in-

termetallics since sublattice diﬀusion does not lead to a coupled diﬀusion of

the components.

Ingenious order-retaining mechanisms, for which diﬀusion of both com-

ponents is coupled, have been proposed for B2 intermetallics:

– Six-Jump-Cycle Mechanism: A vacancy trajectory of 6 consecutive

nearest-neighbour jumps displaces atoms in such a way that after the

cycle is completed the order is re-established [72]. The ratio of the diﬀu-

sivities for this mechanism for a highly ordered stochiometric compound

lies within the following narrow limits [69]: 0.5 <D

∗

< 2.

– Triple-Defect Mechanism: In a stoichiometric B2 compound vacancies

and antisite defects can associate to form triple defects A

+2V

,withV

denoting a vacancy on the A-sublattice and A

an A-antite atom on the B-

sublattice. Then the ratio of the diﬀusivities for this mechanism lies within

the following limits: 1/13.3 <D

∗

< 13.3 [73]. As the composition

deviates from stochiometry antisite atoms widen these limits [74]. Thus,

in a less ordered state, values of D

∗

beyond these limits can no

longer be considered as an indication that the six-jump-cycle mechanism

does not operate.

– Antistructure-Bridge Mechanism: For an ordered B2 phase with

some substitutional disorder antisite defects can act as ‘bridges’ to es-

tablish low-energy sequences for vacancy jumps [75]. Long-range diﬀusion

1 Diﬀusion: Introduction and Case Studies in Metals and Binary Alloys 47

via this mechanism requires a suﬃcient concentration of antisite defects

to reach the percolation threshold [76].

– Vacancy-Pair Mechanism: A bound pair of vacancies (on both sublat-

tices) can mediate diﬀusion of both components by successive correlated

next-nearest-neighbour jumps. Whereas this mechanism has some rele-

vance for CsCl-type ionic crystals it is unlikely for B2 intermetallics.

A detailed description of these mechanisms can be found in [63,67]. It seems

that in those B2 compounds, which are composed of a group VIIIB metal

(Co, Fe, Ni, Pd, etc.) and a group IIIA metal (Al, Ga, In, etc.), the triple

defect mechanism is important. By contrast, B2 phases composed of a noble

metal (Cu, Ag, Au) and a divalent metal (Mg, Zn, Cd) are considered as

candidates for the 6-jump cycle mechanism. Clearly, the antistructure bridge

mechanism becomes more important at deviations from stoichiometry.

Perhaps the most thoroughly studied B2 compound is NiAl, where Ni

diﬀusion has been measured for various compositions on both Al- and Ni-

rich sides and over wide temperature intervals [77, 78]. While the Ni tracer

diﬀusivity increases notably on the Ni-rich side of the stoichiometric compo-

sition it is practically not changed with the composition on the Al-rich side in

spite of the large amount of structural Ni-vacancies (several %). Calculations

of the atomic mechanism using embedded atom potentials showed that the

triple-defect mechanism dominates self-diﬀusion on the Al-rich side and for

stoichiometric NiAl. With increasing Ni-content after reaching the percola-

tion threshold the antistructure-bridge mechanism dominates on the Ni-rich

side [77]. These ﬁndings for NiAl agree with the pioneering work on the B2

phase CoGa by Stolwijk et al. [79] .

1.10.3 The Cu

Au Rule

The Cu

Au rule (see, e.g., [2]) provides a logical tool to fathom the self-

diﬀusion behaviour in non-equiatomic intermetallics. It states that in some

compounds of type A

,wheretheratiom/n is equal to or greater than

2, the majority element diﬀuses faster than the minority element:

∗

. (1.81)

Here D

∗

and D

∗

denote the tracer diﬀusion coeﬃcients of the components

A and B. For geometric reasons discussed below, A

B intermetallics with L1

or D0

structure are good candidates to test the validity of the ‘Cu

Au’ rule

According to [2] it should only be applied to intermetallics in which diﬀusion

occurs via vacancies. Phases such as, e.g., Fe

C, where one of the two elements

is suﬃciently small to occupy interstitial sites in a matrix composed of the other

element, must be excluded. A nice essay about the Cu

Au rule can be found

in [80].

48 Helmut Mehrer

The A sublattice in D0

structure compounds is interconnected by nearest-

neighbour bonds, whereas this is not the case for the B sublattice (see

Fig. 1.22). Thus the A atoms can diﬀuse within their own sublattice via

nearest-neighbour (NN) jumps. If B atoms migrate within their own sublat-

tice, their jump vector corresponds to a third-nearest neighbour jump with

respect to the bcc unit cell. An alternative for the diﬀusion of B atoms are

nearest-neighbour jumps which, however, create B antisite defects. Both op-

tions are very likely associated with higher activation enthalpies for diﬀusion

of B atoms compared to A atoms. Those D0

compounds (Fe

Si, Cu

Sn, see

Table 1.4), for which reliable diﬀusion data for both constituents are available,

indeed fulﬁll this rule [63]. In L1

compounds each A atom is surrounded by

8 A atoms and 4 B atoms on nearest-neighbour sites (see Fig. 1.22). In con-

trast to this situation, a B atom faces only A atoms on surrounding nearest-

neighbour sites. This implies that similar to the D0

structure the sublattice

of the majority component A is interconnected by nearest-neighbour bonds,

whereas this is not the case for the sublattice of the minority component B.

Vacancy motion restricted to the majority sublattice can promote diﬀusion

of A atoms. However, diﬀusion of B atoms either requires jump lengths larger

than the nearest-neighbour distance or the formation of antisite defects, if it

is promoted by NN jumps of vacancies.

As can be seen from Fig. 1.25, diﬀusion of the majority component Ni

in Ni

Ge is indeed signiﬁcantly faster than that of the minority component

Ge. Experiments on Ni

Ga revealed that the trend is similar to the case of

Ge, but the diﬀerence of the diﬀusivities is not so large [81].

For the technologically important compound Ni

Al no unquestioned data

of Al diﬀusion are available [62,63]. There are, however, indications that the

ratio of the two diﬀusion coeﬃcients is not far from unity [64]. It is quite

natural that Ni diﬀusion in L1

compounds occurs by a sublattice vacancy

mechanism. On the other hand, it is not clear how the diﬀusion of the minor-

ity elements occurs in Ni based L1

compounds. Possible mechanisms have

been discussed in [64]. Most likely minority elements diﬀuse as antisite atoms

in the majority sublattice. Thus the Cu

Au rule should not be considered to

be universal. A compound which is a beautiful example for the validity of the

rule is MoSi

. Tracer diﬀusion studies of Si and Mo diﬀusion revealed a huge

asymmetry between the diﬀusion of the majority and the minority compo-

nent. Si diﬀusion is 6 to 7 orders of magnitude faster than Mo diﬀusion [82].

It is interesting to note that MoSi

is one of the compounds on which the

formulation of the rule was based to interpret silicide formation from thin

Mo layers on Si wafers [80].