Hawkes P.W., Spence J.C.H. (Eds.) Science of Microscopy. V.1 and 2
Подождите немного. Документ загружается.

1202 J.C.H. Spence
become important in future work, and should be preserved. The terms
“real object” and “complex object” have also been widely adopted in
optics, but should strictly refer to the nature of the exit-face wavefunc-
tion for two-dimensional imaging. [For a strong phase object described
by Eq. (1), the object ∆n may be real, but the exit-face wavefunction
complex. Most authors would refer to this as a “complex object,”
however, the object property we wish to recover is ∆n(r).] It is also
useful to reserve the word image for real-space functions, and diffraction
for reciprocal space, so that the term “diffraction image,” when refer-
ring to a diffraction pattern, should be avoided. The measured diffrac-
tion pattern intensity is I(u) = |Φ(u)|
2
, with Φ(u) the Fourier transform
of Ψ(r) and scattering vector |u| = Θ/λ for small scattering angles Θ.
(Here λ is the X-ray, or relativistically corrected de Broglie wavelength
for electrons.) I(u) = I(−u) if Ψ(r) is real, or, for complex objects, if Ψ(r)
= Ψ(−r). Φ(u) = Φ*(−u) for real Ψ(r), while Φ(u) is a signed real quantity
if Ψ(r) = Ψ(−r) and Ψ(r) is real. The aim of diffractive imaging is to
reconstruct the object from the scattered intensity I(u); however, as a
fi rst step the exit-face wavefunction Ψ(r), which is simply related to
Φ(u) by a Fourier transform, is obtained. [This reduces to determina-
tion of the sign of Φ(u) if Ψ(r) = Ψ(−r) and Ψ(r) is real.] The further
recovery of object properties ρ(R) or V(R) from Ψ(r) may be possible
only in the absence of multiple scattering or inelastic scattering. For
electron diffraction, the weak-phase approximation generates a “real
object” in the language of optics and diffractive imaging, as described
below. For X-ray diffraction, where single-scattering conditions are
common, the absence of spatially dependent absorption (due to the
photoelectric effect) provides such a “real object,” so that imaging
should be performed at energies that avoid absorption edges for any
elements present if phase contrast is expected. A single, spatially
uniform absorptive process, however, may allow the phase contrast
formulation to be used. The introduction of the transmission function
allows a simple extension to the case of coherent convergent-beam
illumination and related methods for phasing (Spence and Zuo,
1992).
We now relate Ψ(r) to the wanted object properties ρ(R) or V(R) for
the case of visible light, electron beams, and X-rays in the projection,
iconal, or “fl at Ewald sphere” approximation. Both refractive and dis-
sipative (inelastic) processes may occur. In each case it is necessary to
consider whether an iconal or projection approximation may be made,
and the question of whether three-dimensional (tomographic) infor-
mation (discussed later) may be extracted.
The simplest case for each radiation is that in which the projection
approximation holds, in which case the image Ψ(r) may be treated as
a simple projection of some property of the sample, taken in the beam
direction. Then, if a transmission sample in the form of a thin plate of
thickness t is illuminated by a plane-wave,
ψψ πλπrrr r ur
(
)
=
(
)
(
)
=−
(
)
[]
−⋅Tini
p00
22exp / exp∆
((
)
(1)
For normal-incidence plane-wave illumination u
o
= 0, and we may
set Ψ
0
(r) = 1, and Ψ(r) = exp[−iθ(r)]. Here ∆n
p
is proportional to the

Chapter 19 Diffractive (Lensless) Imaging 1203
complex refractive index of the sample for the radiation concerned. We
see that a real (“mask-like”) object can be obtained only if the real part
of ∆n
p
is independent of r, and all structural information is contained
in the imaginary part. These experimental conditions (pure “absorp-
tion” contrast) can be obtained only at relatively low spatial resolution
(under incoherent conditions) for both electrons and X-rays.
For X-rays,
∆nidz
p
t
rRR
(
)
=
(
)
−
(
)
[]
∫
δβ
0
(2)
where d is a positive quantity (Kirz et al., 1995). In terms of mean
values, the complex index of refraction for X-rays is n = 1 − ∆n, =(1 − δ)
+ iβ, where δ describes refraction and β absorption (mainly the photo-
electric effect, arising from absorption edges). The linear absorption
coeffi cient is µ = 4πβ/λ. The dependence of ∆n(r) on the real and imagi-
nary parts of the atomic scattering factors f and f′ is given by δ = (r
e
λ
2
/
2π)n
a
f, and β = (r
e
λ
2
/2π)n
a
f′, with n
a
atoms per unit volume and r
e
the
classical electron radius. Away from absorption edges, the electronic
charge density (excluding the nuclear contribution) is
ρ
π
λ
δRR
(
)
=
(
)
2
2
r
e
(3)
If small bonding effects are ignored, ρ(R) is obtainable from tabulated
X-ray scattering factors for neutral atoms.
Since δ is about 10
−3
at 6 kV for light materials, a thickness of about
0.3 µm of sapphire is needed to obtain a phase shift of π/2, allowing a
fi rst-order expansion of Eq.(1). Then the diffracted amplitudes are
simply proportional to the Fourier transform of the projected charge
density of the object.
For an electron beam of kinetic energy eV
o
,
∆n
V
V
dz
t
p
c
r
R
(
)
=
(
)
∫
2
0
0
(4)
where V
c
(R) is the complex “optical” potential for high energy electrons
(Radi, 1970; Howie and Stern, 1972), and the mean refractive index for
electrons is n = 1 + ∆n. The real part of this, for high energies, is the
positive electrostatic or Coloumb potential, also obtainable from X-ray
scattering factors using Poisson’s equation (Spence and Zuo, 1992). The
imaginary part (typically about a tenth of the real part) accounts for
depletion of the elastic wavefi eld by inelastic scattering events such as
plasmon, inner-shell, and phonon scattering. The average value V
0
of
Re(Vc) is about 12 eV for light materials, and is positive, so this may be
used as a constraint. Both real and imaginary parts of the potential
V
c
(R) are positive if the mean inner potential is included, since electron
beams are attracted predominantly to the positive nuclei. Electron dif-
fraction in the transmission geometry is not, however, sensitive to the
mean potential V
0
, which produces only a constant phase shift. The
mean inner potential can be meaningfully defi ned only for a fi nite
1204 J.C.H. Spence
object with zero total charge (O’Keeffe and Spence, 1993). Although the
potential due to an (unphysical) isolated negative ion may have small
negative excursions, the mean value depends on the volume assumed,
and, in a real crystal, any long range ionic potential is also screened.
In summary, in the absence of its mean value, the real part of the optical
potential used to describe electron diffraction (when synthesized from
diffraction data, with resolution limited by a temperature factor) is
positive, except for possible very small negative excursions around
negative ions. The positivity of the imaginary part is guaranteed by
the requirement that energy gain is forbidden in inelastic processes if
very small virtual processes are ignored. Fourier coeffi cients of the
total optical potential may have either sign, and those of the real
(elastic) and imaginary (inelastic) potential become mixed in objects
without inversion symmetry. Hence a sign condition on the optical
potential may be used as a convex constraint unless atomic resolution
reconstructions are attempted of suffi ciently high accuracy to detect
the very weak bonding effects.
By estimating the maximum value of [Re(Vc) − V
o
], the maximum
thickness can be estimated for which a fi rst-order expansion of Eq.(1)
may be made, the weak-phase approximation, in which θ(r) << π/2.
This is also a limited case of the single scattering approximation. For
X-rays, the maximum thickness allowable in the weak-phase object
approximation can be estimated using the CXRO web calculator page
supported by the Lawrence Berkeley Laboratory at http://www-cxro.
lbl.gov/. For electrons the Fourier coeffi cients of electrostatic potential
published for many materials by Radi may be useful (Radi, 1970), since
these also provide an estimate of the imaginary part of the optical
potential for electron diffraction due to inelastic scattering.
For visible light, ∆n
p
(r) has a similar interpretation as for X-rays (with
n given by the square root of the complex dielectric constant), however,
n > 1. Thus visible light and electrons (n > 1) are bent toward the normal
on entering a denser medium, while X-rays undergo total external
refl ection, with n < 1.
In summary, if inelastic processes are neglected (away from absorp-
tion edges), at high energies, transmission samples of thickness t are
phase objects for electrons and X-rays, for which the refractive index
is proportional to the electron density for X-rays and to the total elec-
trostatic potential, including the nuclear contribution, for electrons.
Poisson’s equation relates these. The magnitudes of these quantities are
such that a fi rst-order expansion of Eq.(1) (weak phase object approxi-
mation) is justifi ed [2πin
p
(r)/λ < π/2) if t < 20 nm for electrons (light
elements, V
0
= 200 kV) but t < 0.3 µm for X-rays (light inorganic material,
6 kV). Higher order terms in the expansion of Eq.(1) correspond to the
multiple-scattering terms of the Born series in a “fl at Ewald sphere”
approximation.
This “fl at sphere” or projection approximation, on which Eq.(1) is
based, depends on the ratio of wavelength to smallest detail d of inter-
est (with spatial frequency u = 1/d), and on the thickness of the sample.
Scattering kinematics restrict elastic scattering to regions of reciprocal
space near the energy and momentum-conserving Ewald sphere of

Chapter 19 Diffractive (Lensless) Imaging 1205
radius 1/λ. The projection approximation holds if the “excitation error”
distance S
u
≈ λu
2
/2 from this sphere onto a plane in reciprocal space
normal to the beam (passing through the origin) is less than either 1/t
or 1/ξ
u
, whichever is the smallest. (ξ
u
is a multiple-scattering extinction
distance for spatial frequency u. Thus samples never look thicker than
ξ
u
, to diffracting radiation.) Hence we require
λ
ξ
u
t
or
u
2
2
11
<
(5)
or
λt
d
2
12
<
(6)
for the validity of Eq.(1). Since the width of the fi rst Fresnel fringe due
to propagation over distance t is approximately w = (λt/2)
1/2
, this condi-
tion requires that the spreading of the wavefi eld due to free-space
propagation over a distance equal to the thickness of the sample be
small compared to the resolution required.
The failure of Eq.(1) may require either single or multiple scattering
treatments, depending on the strength of the interaction and sample
thickness. We note that Eq.(1) is an exact solution that sums the Born
series, including all multiple scattering effects, in the limit of vanishing
wavelength. For X-ray tomography, use of Eq.(1) greatly simplifi es the
collection of data for three-dimensional reconstruction.
The preceding discussion has concerned two-dimensional imaging.
For tomography there is a different analysis. Again, a single-scattering
approximation must be used. But the introduction of a curved Ewald
sphere does not prevent three-dimensional reconstruction (Miao et al.,
2002), since data may be collected for various object orientations, and
in each case assigned to points on the sphere until all of the reciprocal
space volume is fi lled. (Experimentally this is done by rotating the
sample about an axis normal to the beam and recording a diffraction
pattern at each orientation.) Use of the Fienup algorithm with three-
dimensional Fourier transform iterations can then take advantage of
the improved convergence in three dimensions. A sign constraint may
or may not be applied to both real and imaginary parts of the scattering
potential, and a general spherical support, enclosing the object, has
been found useful.
4 The HIO Algorithm and Its Variants
We now assume the weak-phase approximation, in which the exit-face
wavefunction is computed along a single optical path along the beam
direction, and no multiple scattering or inelastic processes are permit-
ted other than an overall, spatially independent exponential attenua-
tion with thickness. The recorded intensity of the diffraction pattern,
excluding the central portion, is then given as
I(u) = Φ(u)Φ(u)* = |FT(Ψ(r)|
2
(7)
1206 J.C.H. Spence
If the phase of the Fourier transform FT[Ψ(r)] could be recovered,
than the aberration-free complex exit wave Ψ(r) could be reconstructed.
Aberrations of any diffraction lenses in the electron case that magnify
the diffraction pattern (astigmatism, distortion, etc.) could alter the
intensity distribution I(u) and so complicate recovery.
For nonperiodic objects the phase problem can now be solved if the
object has a fi nite support, i.e., the image is nonzero only within a fi nite
region of image space and if the image is real and nonnegative. For a
weak phase object the exit-face wavefunction is Ψ(r) ∼ 1 − iθ(r) where
θ(r) may be treated as real and positive. The diffracted amplitude is
Ψ(u) = δ(u) − iΦ(u), for which the scattered part differs in phase by 90°
from the direct beam given by the fi rst term. This direct “unscattered”
beam is absorbed by the beam-stop; diffi culties arising from the fi nite
size of this are discussed later, and we note that the average value of
θ(r) is also lost within the beam stop. Including a larger area around
the object known a priori to have zero or unit transmission function is
equivalent to “oversampling” in Fourier space, and the spatial coher-
ence of the illumination must span this larger area, at least equal to
that of the autocorrelation function of the object. Hence diffractive
imaging requires spatial coherence twice that of coherent imaging with
a lens (Spence et al., 2004), increasing exposure time by a factor of four
for a given source and resolution limit.
We assume that |FT[Ψ(x, y)]| has been measured, and that the
support S(x, y) of θ(r) (which is the same as that of the object) is known.
S(x, y) is the region outside which the object is known to be zero. The
iterations start with an initial estimate
˜
G
1
(u, v) = |FT[Ψ(x, y)]|exp[iθ
1
(u,
v)] of the spectrum. θ
1
(u, v) is chosen to be an array of independent
pseudorandom real numbers distributed between 0 and 2π. The itera-
tive Fourier transform algorithm consists of the following steps (with
subscript k labeling quantities at the kth iteration):
1. Inverse Fourier transform
˜
G
k
(u, v) to obtain the image ˜g
k
(x, y)
2. Defi ne g
k+1
(x, y) as
gxy
gxy xy Sxy
gxy g
k
k
k
+
(
)
=
(
)
(
)
∈
(
)
(
)
−
1
,
,,,
,
if
β
kk
xy xy Sxy,,,
(
)
(
)
∉
(
)
{
if
(8)
This constitutes the HIO version of the algorithm. β is a constant
chosen between 0.5 and 1. In the ER version of the algorithm this step
is replaced by
gxy
gxy xy Sxy
xy Sx
k
k
+
(
)
=
(
)
(
)
∈
(
)
(
)
∉
1
0
,
,,,
,
if
if ,, y
(
)
{
(9)
3. Fourier transform g
k+1
(x, y) to obtain G
k+1
(u, v)
4. Defi ne new Fourier domain function
˜
G
k+1
(u, v) using the known
Fourier modulus |FT[Ψ(x, y)]| with the computed phase:
˜
G
k+1
(u, v) = |FT[Ψ(x, y)]|exp[iθ
k+1
(u, v)]
5. Go to step 1 with k replaced by (k + 1).
To monitor the progress of the algorithm, the object space error metric
ε
k
is calculated during each iteration:

Chapter 19 Diffractive (Lensless) Imaging 1207
ε
k
k
xy S
k
xy
gxy
gxy
=
(
)
(
)
(
)
∉
(
)
∑
∑
,
,
,
,
2
2
(10)
ε
k
is the amount by which the reconstructed image violates the image-
space constraints. Physically, in the X-ray case, it is the normalized
amount of charge that remains outside the boundary of the object,
which should be zero. In all our calculations we have used β = 0.7 and
a combination of the ER and HIO algorithms, with 20 ER iterations
followed by 50 HIO cycles, all repeated until the error ε
k
drops below
a certain level. Simulations based on the above procedure with small
noise levels invariably converge to the correct solution (Weierstall
et al., 2001; Spence et al., 2002). Since g(x, y), g*(−x − a
1
, −y − a
2
)exp(iθ)
and g(x − a
1
, y − a
2
)exp(iθ) all have the same Fourier modulus they
cannot be distinguished by the algorithm. Each run of the algorithm
started with different random phases may thus produce images cen-
tered on different origins or related by inversion symmetry. We call
these equivalent images. (Similarly, in three dimensions, enantio-
morphs cannot be distinguished.)
Some understanding of the inversion can be obtained by considering
the Fourier equations relating the object to its diffraction pattern. We
reverse the domains originally considered by Shannon, and treat the
object space as if it applies a “bandlimit” to the diffraction pattern (the
object is assumed compact). Shannon’s theorem then specifi es either
the sampling interval on the diffraction pattern intensity needed to
fully reconstruct the autocorrelation function of the object, or the sam-
pling of the complex scattered amplitude needed to reconstruct the
object. Consider a simple one-dimensional complex exit-face wavefunc-
tion f(x), which is nonzero only for 0 < x < W, so the support has width
W. We fi rst treat this function as the “bandlimit” on the diffraction
pattern F(u), which must therefore be sampled at intervals u
n
= n/W by
the detector to satisfy Shannon’s theorem. We then have the N equa-
tions (one for each pixel in the detector)
Fu f x inx W n N
ni i
i
N
(
)
=
(
)
(
)
=
=
∑
exp ...21
1
π
relating measured intensities |F| to the 2N unknown complex values
of f(x
i
) we seek. Since there are more unknowns than equations, the
complex values of f(x) cannot be found from these measurements.
However, now consider the same function placed within a domain
of width 2W, so that the values of f(x) in W < x < 2W are known a priori
to be zero. The sampling interval on F(u) is now 1/(2W) and we thus
have 2N equations, but the number of unknown values of f(x) remains
at 2N, so that the system of equations now becomes solvable in princi-
ple. Loosely speaking, we compensate for the missing half of the data
in the diffraction domain (the phases) by requiring that half of the
object values be known (they are zero outside the support of width W).
Since the HIO algorithm assigns a random set of phases initially, the
1208 J.C.H. Spence
equations are also linear within the algorithm unless a sign constraint
is applied. Experimentally, real data from a CCD may not provide
independent equations (symmetrical support shapes also lead to
dependent equations) and the effects of noise must be considered.
Large systems of nonlinear equations are normally extremely diffi cult
or impossible to solve, so it is remarkable that the HIO algorithm does
this so effectively, for reasons that are not fully understood, since
few methods exist for analyzing nonconvex optimization. However,
although the Fourier modulus constraint is nonconvex, it is known that
the error surface in hyperspace is not a rugged landscape, but consists
of a single rather smooth minimum with a few small bumps. Use of a
boundary estimate (support) without symmetry also drives the algo-
rithm toward one particular enantiomorph ρ(r) rather than a spurious
mixture involving ρ(−r).
This example may be extended to other cases: for complex, two-
dimensional objects there are 2N
2
unknowns but N
2
equations (if the
CCD has linear pixel dimension N). But by placing empty space around
our compact object, which increases the diffracting volume by 2
1/2
in
both dimensions, we sample the diffraction pattern more fi nely and
recover the 2N
2
equations needed to solve the phase problem. It is the
knowledge of the support (the object boundary) that ensures that
known object pixel values may be inserted in the algorithm correctly
outside the support. Experimentally, this creates the greatest diffi culty
of the diffractive imaging method; it is necessary to know a priori that
the diffraction pattern comes from an isolated object whose size is approxi-
mately known. The existence of any material outside the assumed
support that unwittingly contributes to the diffraction pattern will
result in inconsistent constraints being applied by the algorithm, which
will then not converge.
When experimental data are analyzed, the physical support in the
object may not be known accurately. To avoid confusion we call the
support actually present in the experiment the “physical support” and
the support estimate used in the data analysis the “computational
support.” The term “loose support” describes the situation in which
the computational support is larger than the physical support. “Tight
support” means the physical support is the same as the experimental
support. For two-dimensional simulations with real objects, it is found
that a “default” triangular-shaped support may invariably be used, if
it is chosen to lack any symmetry and enclose the object. With experi-
mental data, convergence may be slower (or nonexistent) due to high
noise levels, the absence of data around the origin at the beam-stop,
and excessively loose support. Then the simplest initial choice of
support is the boundary of the autocorrelation function (obtained by
Fourier transform of the diffracted intensity). This estimate is rapidly
improved upon by the shrinkwrap algorithm, which uses a specifi ed
intensity threshold to fi nd an improved smaller boundary at every
iteration of the HIO algorithm (Marchesini et al., 2003b). This appears
to be the most useful practical algorithm at present. Depending on
noise levels, the support estimate has been found to be suffi ciently
accurate to deal with complex objects in many cases.
Chapter 19 Diffractive (Lensless) Imaging 1209
We conclude this section with some comments on additional con-
straints, the beam stop problem, support determination, and recent
algorithm developments. In the general case of strong multiple scatter-
ing no simple closed-form expression relates the object to the exit-face
wavefunction, and the two are related only by symmetry constraints.
For a general (“strong”) phase object [Eq. (1)], a unit modulus con-
straint may be applied, so that reconstructed pixels are forced to lie on
a unit circle on an Argand diagram; however, this constraint is non-
convex and so has not been found very useful in practice. For such an
object, to which a spatially independent absorption term is added [so
that ρ(R) or V
c
(R) has a constant known imaginary part], the recon-
structed image pixels may be constrained to lie on a given spiral on an
Argand diagram. A summary of the many constraints that have been
tried and experimental results is given in Weierstall et al. (2001). Other
constraints include atomicity, symmetry (most useful for reducing
computing time), imposition of a known histogram of gray-levels for
the object density, and low-resolution imaging by a different technique
to provide a support estimate. In the following section the use of pre-
pared objects is demonstrated to allow the method of Fourier trans-
form holography to be used to provide a support estimate. The reference
object need not be a simple point scatterer (He et al., 2004), and may
have an extended complicated known shape (Szoke, 1997). The shrink-
wrap algorithm described below rapidly improves iteratively on any
initial support estimate and is found to converge for both real and
complex objects under a wide range of conditions. For arrays of semi-
conductor devices the support will often be known, so that tomo-
graphic imaging of defects within an array element might be based on
a support provided by the lithography pattern used to make the
array.
A remarkable new “fl ipping” algorithm has recently appeared
(Oszlanyi and Suto, 2004) for the crystallographic phase problem,
which may also be adapted for nonperiodic objects, in which case it
reduces to Fienup’s output–output algorithm with feedback parameter
β = 2 and a dynamic support defi ned by an adjustable threshold (Wu
et al., 2004b). This algorithm operates as follows: First, random phases
are assigned to the structure factors, which must extend to atomic reso-
lution. These are transformed to yield a real charge density. All density
values below a certain threshold have their sign reversed. The result is
transformed, and Fourier magnitudes are replaced with measured
values. The process is continued to convergence. This algorithm (far
simpler than the direct methods normally used in crystallography) has
been used to solve new crystal structures from X-ray data (Wu et al.,
2004a), and is found to perform very well. It does not require a support
estimate or knowledge of atomic scattering factors, but does require
atomic-resolution data. The “atomicity” constraint in crystallography
assumes that the solution density consists of a set of smooth peaks, and
requires that diffraction data extend to atomic resolution. The support
then consists of spheres around each atom; most of the density within
a crystal consists of empty space between atoms, akin to the zero-
density band generated by oversampling around an object in the HIO
1210 J.C.H. Spence
algorithm. Since all matter in the cold universe consists of atoms (whose
scattering factors are known), this constraint provides an extremely
powerful ab initio assumption if one has atomic-resolution data, and is
the basis of the direct methods algorithms of X-ray crystallography.
Other known “building blocks” (such as gold balls or lithographed
dots) have been used to reduce the number of unknown parameters in
image defi nition (Spence et al., 2003b). (For proteins, for example, both
the sequence and the atomic structure of the 20 amino acids of which
they consist are usually known a priori, together with a typical gray-
level histogram for the density maps.) An algorithm that has used the
atomicity idea for nonperiodic data is Speden (Hau-Riege et al., 2004),
which has been applied to the data of Figure 19–3. Finally, we note the
use of the HIO algorithm for two-dimensional protein crystals in elec-
tron microscopy. This technique uses Fourier transforms of conven-
tional weak-phase-object images (formed with a magnetic lens) to
provide the phases of structure factors, and diffraction patterns for
their magnitudes. The subnanometer resolution images must be
obtained over as large a range of tilts as possible, creating serious
experimental diffi culty. These two-dimensional monolayer crystals are
nonperiodic in the direction normal to the plane of the crystal, so that
lines of diffraction are generated in reciprocal space. By oversampling
along these lines it is possible to phase these reciprocal lattice rods
individually. The phase relation linking them can then be obtained
form a few high-resolution images recorded at small tilts. In this way
the number of electron microscope images needed for three-
dimensional imaging of two-dimensional organic crystals can be
greatly reduced, with most of the reconstruction based on easy-to-
obtain diffraction data (Spence et al., 2003a).
We note in passing that the nonuniqueness of the crystallographic
phase problem has been well studied; the so called “homometric”
crystal structures studied by Pauling, Burger, and others have the same
diffraction patterns, but different structures. (They are not enantio-
morphs.) Fortunately these are very rare.
Several approaches have been made to the problem of data lost
behind a synchrotron beam-stop, which is essential to protect a sensi-
tive area detector. Any additional blooming can mean much loss of
low-frequency data. In several HIO applications these missing values
have simply been treated as free adjustable parameters, and the algo-
rithm was found to converge. Calibrated absorption fi lters have been
placed in front of the inner portion of the detector. Another solution is
to use a sample consisting of an unknown object fi lling a small hole
in an otherwise opaque mask (Eisebett et al., 2004; Weierstall et al.,
2001). Stray scattering of X-rays from the edges of the holes in the mask
can be a diffi culty; however, surface roughness, which is smaller than
the wavelength of the X-rays, produces little scattering. The use of very
small silicon nitride windows (e.g., 2 µm width) greatly reduces the
intensity of the direct beam and blooming effects; however, the detailed
shape of the partially transparent silicon wedge around the window
must then be modeled and used as a support for inversion. (When
making samples of small particles deposited from solution, it is most
Chapter 19 Diffractive (Lensless) Imaging 1211
effi cient to use a focused ion beam to remove all but a favorably located
and isolated particle in the center of the window.) Finally, the “diffuse”
X-ray scattering around Bragg peaks (which forms the “shape trans-
form” of the object) from a crystallite has been inverted to an image,
thus avoiding the direct-beam scattering (Robinson et al., 2001;
Williams et al., 2003).
5 Experimental Results
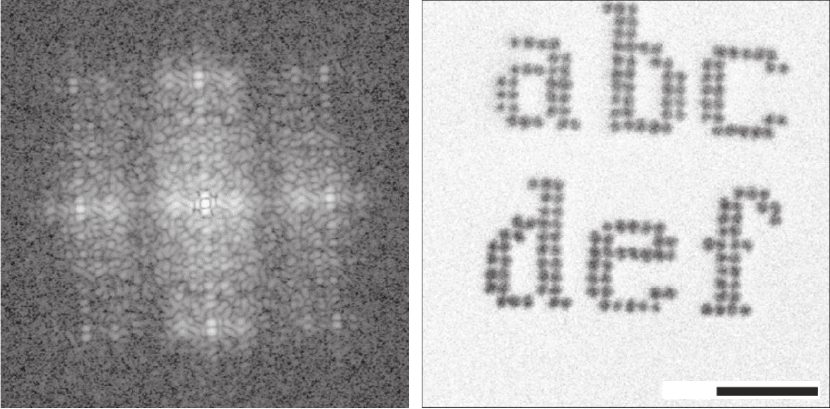
Figure 19–1 shows the fi rst X-ray images reconstructed by this lensless
method in 1999 (Miao et al., 1999). The test object consists of letters
formed from gold dots, 100 nm in diameter and 80 nm thick, on a trans-
parent silicon nitride membrane. The transmission X-ray diffraction
pattern formed with 1.7-nm monochromatic soft X-rays is shown in
Figure 19–1A and the reconstructed image in Figure 19–1B. The object
was illuminated through a coherently fi lled 10-µm-diameter pinhole,
and a 25-cm camera length was used. Missing data from the central
region within the beamstop were obtained from a lower-resolution
optical image. The exposure time was 15 mins at the Brookhaven syn-
chrotron. The Fienup algorithm was used for reconstruction, with a
sign constraint applied to both real and imaginary parts of the scatter-
ing potential. A square support was used (irregular shapes usually
work better) and 1000 iterations were needed for convergence. The
resolution is about 75 nm. A detailed description of an improved version
of the apparatus used to obtain this and other recent results at the
Advanced Light Source is given in Beetz et al. (2005).
A
B
1 mm
Figure 19–1. (A) Soft X-ray transmission diffraction pattern formed with 1.7-nm X-rays from the set
of lithographed letters shown in (B). (B) Image recovered from (A) using a modifi ed form of the HIO
algorithm. (From Miao et al., 1999.)
