Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations
Подождите немного. Документ загружается.


transform
the
graph
ofa
functlon
to
find
the
function's
transform!
transform
ofa
function
bya
group
element
29.2
SYMMETRY
GROUPS
OF
DIFFERENTIAL
EQUATIONS
943
Wigner
showed
that
application
ofLie
algebras
tothe
Lorentz
transformation
required
that
all
particles
havethe
intrinsic
properties
of massandspin.
To
make
precise the above statement, we have to clarify the meaning
nf
the
action
of
G on a function u = f (x). We start
with
identifying the function f (i.e.,
a map) with its graph (see Chapter
0),
rl
ea
{(x,
f(x»[x
E Q}
eX
x U,
where Q e X is the domain
of
definition
of
f.
If the action
of
g E G on
I'
I is
defined, then the transform
of
rI by g is
g.
r
1=
{(i,
it) =
g.
(x, u)
[(x,
u) E r
I}'
In general, g . rI may
not
represent the graph
of
a
function-in
fact, it may
not
be even a function at all. However, by choosing g close to the identity
of
G
and
shrinking the size
of
n,we
can
ensure that g .rI = rJ' i.e., that g . rI is indeed
the graph
of
a function it = I
(i).
We write I = g . f and
calli
the transform
of
fby
g.
29.2.1. Example. LetX =
IR
=U, sothatwearedealingwithanODE.Let G =
SO(2)
bethe
rotation
group
actingonX x U =
JR2.
Theactionis givenby
(i,
il) = O· (x, u) = (x coss - u
sinO,x
sinf
+ucosO). (29.6)
Ifu
=
f(x)
is afunction, thegronp
SO(2)
actsonits graphrI byrotatingit.Thisprocess
canleadtoa
rotated
graph
()
.
I'
i» whichmaynotbethe
graph
ofa
single-valued
function.
However,
if
we
restrict
the
interval
of
definition
of f. and
make
(}
small
enough,
then
()
.
I'
f
will be the graphof a well-defined functionil =J(x) with rJ =0 . r
I'
If we substitnte
f(x)
for u, weobtain
(x,il) = 0 . (x,
f(x))
= (x coes -
f(x)
sms, x sinO+
f(x)
ccs
s),
or
x
=xcosO
-
f(x)
sinO,
il=xsinO+f(x)cosO.
(29.7)
Eliminating
x
from
thesetwo
equations
yieldsil in
terms
of
i,
from
whichthe
function
j
canbe
deduced.
As a specific example,consider
f(x)
=
kx
2.
Then,the firstequationof (29.7)gives
(
k ' 0)
2 -
cosO-Jcos
20-4kisinO
sm x
-cosf}x+x=O
=>
x=
2ksin8 '
where we kept the root of the
quadratic
equation that gives a finite answer in the limit
o
-->
D.
Insertingthis in the secondequationof (29.7)and simplifyingyields
_
f-(-)
coss -
Jcos
20
-
4kx sinO _
u = x = 2
-xcotO.
2ksin 0
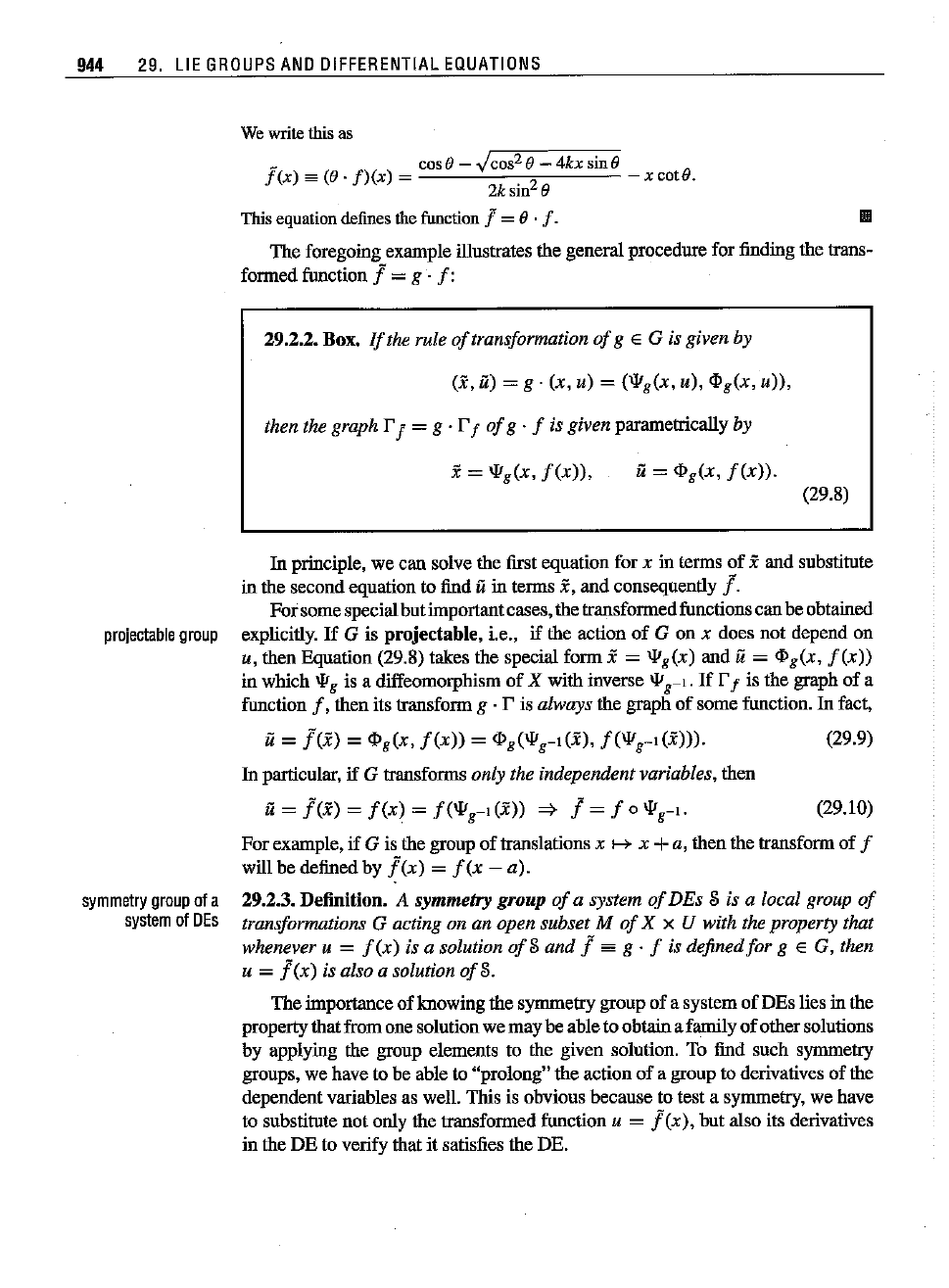
944 29. LIE
GROUPS
AND
DIFFERENTIAL
EQUATlDNS
We
write
thisas
_ coss -
Jcos
20
-
4kx
sinO
!(x)
==
(0 .
f)(x)
= 2 - x
cor
s.
2ksin 0
This eqoation defines the function 1= 0 .
I:
III
The foregoing example illustrates the general procedure for finding the trans-
formed function
I = g .
f:
29.2.2. Box.
If
the rule
of
transformation
of
g
EGis
given by
(i,
it)
=
g.
(x, u) = (Wg(x, u),
<l>g(X,
u)),
then the graph
r j = g . rf
of
g . f is given parametrically by
i
= Wg(x,
f(x)),
it =
<l>g(x,
f(x)).
(29.8)
In
principle, we can solve the first equation for x in
terms
of
i and substitute
in the secondequation to find
it in terms
i,
and consequently
1-
For
some specialbutimportantcases, the transformedfunctionscan be obtained
projectable
group
explicitly.
If
G is
projectable,
i.e.,
if
the action
of
G on x does not depend on
u, then Equation (29.8) takes the special form i = Wg(x) and it =
<l>g(x,
f(x))
in which 'I'g is a diffeomorphism
of
X with inverse
'1'.-"
If
rf is the graph of a
function
f,
then its transform g . r is always the graph
of
some function.
In
fact,
it =
I(i)
=
<l>g(x,
f(x))
=
<l>g(W.-,
(i),
f(W.-,(i))).
In
particular,
if
G transforms only the independent variables, then
it =
I(i)
=
f(x)
=
f(W.-,(i))
=}
1= f 0
'1'.-"
(29.9)
(29.10)
symmetry
group
ofa
system
of
DEs
For
example, if G is the group of translations x
f->
x +a, then the transform of f
will be defined by
I(x)
=
f(x
- a).
29.2.3. Definition. A symmetry group
of
a system
of
DEs S is a local group
of
transformations G acting on an open subset M
of
X x U with the property that
whenever u
=
f(x)
is a solution
ofS
and
I'"
g.
f is definedfor g E G, then
u =
I(x)
is also a solution
ofS.
The importance of knowing the symmetry group of a system
of
DEs lies in the
propertythat from one solutionwe may be able to obtainafamily
of
othersolutions
by applying the group elements to the given solution. To find such symmetry
groups, we have to be able to "prolong" the action of a group to derivatives of the
dependent variables as well. This is obvious because to test a symmetry, we have
to substitute not only the transformed function
u = I
(x),
but also its derivatives
in the DE to verify that it satisfies the DE.

29.2
SYMMETRY
GROUPS
OF
OIFFERENTIAL
EQUATIONS
945
29.2.1 Prolongation
of
Functions
Givena function f :X
--+
JEt,
there are
differentderivatives of order
k of
f.
Weuse the multi-indexnotation
aJi(x)
"'"
. a
k
f(x)
.
aXil8xJ2
...
OXik
for these derivatives, where J =
(h,
...
,
jk)
is an unordered k-tuple of nonnega-
tiveintegerswith I
:s:
A
:s:
p (seealsoSection21.1). Theorder of the multi-index
J, denoted by IJ
I,
is the sum of its components and indicates the order of differ-
entiation.So, in the derivative above,
IJI= h +...+A =k. For a smoothmap
f:
X
--+
U,wehavef(x)
=
(fl(x),
...
,
fq(x)),
so tharwenecd
q-ji,
numbers
a]
f"(x)
to representall k-th order derivatives of all components of
f.
To geometrize the treatment of DEs (and thus facilitate the study of their
invariance), we needto constmct a spacein whichderivativesof all ordersup to a
certain
number
n
participate.
Since
derivatives
need
functions
toacton,we
arrive
at the space of functionswhose derivatives share certain common properties. To
be specific,me makethe followingdefiultion.
29.2.4. Definition. Let f and h be functions defined on a neighborhood
of
a
n-equlvalenoe
of
point a E X with values in U. We say that f and
hare
n-equivalent at a
if
functions
a]
f"
(a) = a]h"(a) for all '" and J corresponding to all derivatives up to nth
order.The collection
of
all U-valued functions defined on a neighborhood
of
a
will be denoted by r
.(X
x U), and allfunctions n-equivalent to f at a by
j~
f.
A convenientrepresentativeof such equivalentfunctions is the Taylorpoly-
nomial of order
n (the terms in the Taylor series up to nth order) about a. Now
collect all
j~
f for alla and
f,
and denotetheresult by In
(X
x
U),
so that
r(XxU)"",[j~fIVaEX
and
VfEr.(XXU)).
(29.11)
nth
jet
space
of
U
reX
x U) is calledthenth prolongation of U, orthenth
jet
space of U.
It
tums
out that
r(X
x
U)
is a manifold(see [Saun89, pp. 98 and 199]).
29.2.5. Theorem.
In(X
x U) is a manifold with natural coordinate functions
(xi,
u",
uj)
definedby
Xi(j~f)
=ai,
u"(j~f)
=
rea),
uj(j~f)
=
aJi"(a).
Note
that
the
"points"
of
J"(X x
U)
are
U-valued
funotions!
Thenaturalcoordinatefunctionsallowus toidentifythespaceofthederivatives
withvariouspowersofR, Let
Uk
"'"
JEtqpk
denote thesetofcoordinates
uj,
andlet

946 29. LIE
GROUPS
ANO
DIFFERENTIAL
EQUATIONS
U(,,)
'"
U X UI X
...
x U" be the Cartesian product space! whose coordinates
represent all the derivatives
u
J
of
all orders from 0 to n,
The
dimension
of
U(,,)
is
A typical point in
U(,,)
is denoted by
u(,,),
whichhas qp(") different components
{uJ}~=I'
where J runs over all unordered multi-indices J =
(h,.··,
ik) with
1
:0:
ik
:0:
p and 0
:0:
k
:0:
n. By convention, k = 0 refers to no derivatives at
all, in which case we
set
J = 0
and
ug
sa u",
The
nth
jet
space
J"(X
x U) can
now be identifiedwith X x
U(,,).
From
now on, we shall use X x
U(,,)
in place
of
J"(X
xU).
29.2.6. Example. Let p = 3 and u = 1,i.e., X =
JR.3
and U = R. Thecoordinatesof X
are(x, y, z) andthat
of
U isu. The
coordinates
of Ul are
(UXI
"y. u
z),
where
the
subscript
denotes
the
variable
of
differentiation.
Similarly,
the
coordinates
of U2
are
(uxx,
Uxy.
"xz.
U
yy•
Uyz.
u
z
z)
andthose of
U(2)
==
U X
UI
x U2 are
(u;
"x.
"y.
U
z
; Uxx. Uxy.
"xz.
Uyy.
uyz. u
z
z),
whichshowsthat
U(2)
is lO-dimensional.
III
29.2.7. Definition. Given a smooth map f : X
:::J
g -+ U, we define a map
prolongation
ofa pr(") f : g
-+
urn) whose components (pr(")
f)~
are given by
function
This map is called the nth prolongation
of
f.
Thus, for each x E X, pr(n)
f(x)
is a vector in jRqp(" whose components are
the values
of
f and allits derivatives up to order n at the point x.
For
example, in
the
case
of
p = 3, q = 1 discussed above, pr(2)
f(x,
y, z) has components
When
the underlying space is an
open
subset M
of
X
xU,
its corresponding
jet
space is
M(n) saM x UI X
•.•
X Un,
which is a subspace
of
X x urn)
~
J" (X x U).
If
the graph
of
f :X -+ U lies
in
M,
the graph
of
pr(")f lies in M(").
INotethatU,
identified
with thespaceof
zeroth
derivative,
is a
factor
in o».

29.2
SYMMETRY
GROUPS
OF
OIFFERENTIAL
EQUATIONS
947
Prolongation allows us to turn a system
of
DEs into a system
of
algebraic
equations: Given a system of I DEs
Ll.
v
({xi},
{U
U},
{ai
UU},
{aiajU
U},
...
,
(ai""
ai,Uuj) = 0, v = 1,
...
, I,
one can define a map
Ll.
:
M(n)
-+
~l
and identify the system
of
DEs with
S"
'"
{(x,
u(n)
E
M(n)
I
Ll.
(x,
u(n)
= O}.
By identifying the system of DEs with the snbset
S"
of the
jet
space, we have
translated the abstract relations among the derivatives of
u into a geometrical
object
S",
which is more amenable to symmetry operations.
29.2.8. Definition.
Let Q be a subset
of
X and f : Q -+ U a smooth map. Then
f is called a solution
of
the system
of
DEs S" if
Ll.
(x,
pln)
f(x))
= 0
vx E
Q.
Just as we identified a function with its graph, we can identify the solution
of
a system
of
DEs with the graph of its prolongation pr(n)
f.
This graph, which is
denoted by
rr),
will clearly be a subset
of
S,,:
rjn)
'"
{(x, pr
tn)
f(x))}
c
s",
29.2.9. Box. An nth order system
of
differential equations is taken to be
a subset S"
of
the
jet
space
J"(X
x U), and a solution to be a smooth
map f : Q
-+
J"(X
x U) the graph
of
whose nth prolongation pr(n)f is
contained in
S",
29.2.10. Example.
Consider
Laplace'sequation
v
2
u
=u
xx
+Uyy +Uzz = 0
withp = 3,q = I, andn = 2. Thetotaljet spaceis the
13-dimensional
Euclidean space
X x
U(2),
whose
coordinates
are
taken
tobe
(x, y, z; u;ux, u
y.
u
z;
u:cx,
Uxy.uxz. Uyy. uYz. uzz)·
In
this13-dimensional
Euclidean
space,Laplace's
equation
defines
a 12-dimensional sub-
space g
li. consisting of all pointsin thejet space whose
eighth,
eleventh,
and
thirteenth
coordinates
add
upto
zero.
A
solution
f :
JR.3
:>
Q
-+
U c
IR
must
satisfy
V(X,y,Z)EQ.

948
29. LIE
GROUPS
AND
DIFFERENTIAL
EQUATIONS
This is the same as requiring the graph
I'
j)to lie in S6..
For
example,
if
fer)
==
f(x,
y, z) = x
3yZ
- y3
xZ
+y3 - 3
YZ2,
then, collecting
each
section
of
pr(2)f withfixed a (see Definition 29.2.7) separately, we
have
(pr(2)
f)
1
(r)
= x
3yZ
_ y3XZ+y3 _ 3YZ2,
(pr(2)
f)2(r)
= (3x
2yz
_ y3
Z,x
3Z
-
3lxz
+3
y2
_ 3z
2,x
3y
_ y3
x
- 6yz) ,
(pr(2)
f)\r)
= (6xyz, 3x
2z
- 3y2
Z,
3x
2y
- y3,
-6xyz
+6y, x
3
-
3xl-
fiz,
-6y)
whichliesin
S.6.
becausethesumof theeighth,theeleventh, andthethirteenth coordinates
of (x, y, z; pr(2)
f(x,
y, z)) is.tixyz -
6xyz
+6y - 6y = O. III
nth
prolongation
ofa
group
action
29.2.2 Prolongation of Groups
Suppose G is a group
of
transformations acting on M C X
xU.
It
is possible to
prolong this action
to the n-jet space
M(n).
The
resulting gronp that acts on
M(n)
is called the nth
prolongation
of
G and denoted by pr(n)G with group elements
pr(n)
g, for g E G. This prolongation is defined naturally: The derivatives
of
a
function
f with respect to x are transformed into derivatives
of
j = g . f with
respect to
x.
More precisely,
pr(n)
g .
U;
/) '"(x,
pr(n)
j(x))
'"
(x,
pr(n)
(g .
/)(1'))
.
(29.12)
For n = 0, Equation(29.12) reduces to the action
of
G on M as given by Equation
(29.8). That the outcome
of
the action
of
the prolongation
of
G in the defining
equation
(29.12) is independent
of
the representative function f follows from the
chain rule and the fact that only derivatives up to the
nth order are involved.
The
following example illustrates this.
29.2.11.
Example.
Let x, U, andG beasin Example29.2.1.
In
thiscase,wehave
pr(llO.
til
f)
espr(l)o . (x, u, Ut) es
(i,
ii,
iit).
We calculated xand uin thatexample. They are
x=xcos6-usin8,
u=xsin6+ucosB.
(29.13)
To find u1. we need to differentiate the second equation
with
respect to xand express the
result
in terms
of
the original variables. Thus
_ dii dii
dx
( . du )
dx.
dx
u1
ea
di
=
dx
di
= smO+
dx
cosO
di
= (smO+ ul cosO)
di:
dx
/
di
is obtainedby differeotiating the firsteqoationof (29.13):
~
~
~
~~
~
1=
d-
cosB -
d-
sinB =
---=-
cos s - ----;;sinO = (cosO- Ul sinO)---;;.
x x dx
dx dx
dx

29.2
SYMMETRY
GROUPS
OF
OIFFERENTIAL
EQUATIONS
949
or
dx
di
=
COS(}-Ulsin(}'
It
therefore
follows
that
_
sin(}+UICOS(}
u1 =
--;;--'---:----;:
CDSe - Ul sine
and
(1)
( .
OO8+U
1CO'8)
pr
f),(x,u,ul)=
xcos(}-usin01xsm(}+ucos(},
8 . 8 .
cos
-"1
sm
Wenote
that
the
RHS
involves
derivatives
upto
order
one.
Therefore,
thetransformation
is
independent
of the
representative
function.
So, if we hadchosen ilk
where
h is 1-
equivalent
to
I,
we
would
have
obtained
thesame
result.
Thisholdsfor
derivatives
of all
orders.
Therefore,
the
prolongation
of the
action
of the
group
G is
well-defined.
11II
In
many case" it is convenient to choose the nth-order Taylor polynomial as
the representative of the class of n-equivalent functions, and, if possible, write the
transformed function
f explicitly in terms of
i,
and differentiate it to obtain the
transformed derivatives (see Problem 29.3).
Example 29.2.11 illustrates an important property
of
the prolongation
of
G.
Wenote that the first prolongation
pr(1)Gacts on the original coordinates (x, u) in
exactly the same way that
G does. This holds in general:
29.2.12. Box. The effect
of
the
nth
prolongation
prCnlG
to derivatives up to
order m
::::
n is exactly the same as the effect ofpr(m)G.
Ifwe
already know
the action
of
the mth-orderprolongedgroup pr(mlG,then to compute
prCnlG
we needonly find how the derivatives
u~
of
order higher than m transform,
because the lower-order action is already determined.
29.2.3 Prolongationof VectorFields
The geometrization of a system of DEs makes it possible to use the machinery
of
differentiable manifolds, Lie groups, and Lie algebras to unravel the synunetries
of the system. At the heartof this machinery are the infinitesimal transformations,
which are directly connected to vector fields. Therefore, it is necessary to find out
how a vector field defined in
M C X x U is prolonged. The most natoral way to
prolong a vector field is to prolong its integral
curve-which
is a one-parameter
group
of
transformations
of
M-to
a curve in
MCnl
and then calculate the tangent
to the latter curve.

950
29. LIE
GROUPS
AND
DIFFERENTIAL
EQUATIONS
nth
prolongation
ofa
vector
field
29.2.13.
Definition.
Let
M be an open subset
of
X x U and X E
X(M).
The nth
prolongation
of
X, denoted by pr(u)X, is a vectorfield on the nth
jet
space M(n)
defined by
pr(n)XI(x
u("»
=
~pr(n)[exp(tX)l'
(x, u(n»1
'dt
,~O
for
any (x,
u(n»
E M(n).
Since
(x,
u(n»
E M(n) form a coordinate
system
on M(n),
any
vector field in
M(n)
can
be written as a linearcombination
of
a/ax
i
and
a/au]
with
coefficients
being, in general, functions
of
all coordinates xi
and
u].
For
a prolonged vector,
however, we have
P a q a
pr(n)x=
LX
i
-
i
+LLX]-., (29.14)
i=l
ax
a=l
J
au]
wbere
Xi
and
X
o
are functions only
of
x
k
and
u.
This
is due to the
remark
made
in
Box
29.2.12.
For
the same reason,
the
coefficients
X]
correspondingto derivatives
of
order
mwill be independent
of
coordinates u]
that
involve derivatives
of
order
higher
than
m. Thus, it is possible to construct various prolongations
of
a given
vector field recursively.
29.2.14.
Example.
Let us consider our recurrent example of
X;:
U
;:
JR,
G = SO(2).
Given the infinitesimal generator v =
-ua
x
+
xa
u
, one can solve the DE
of
its integral
curve to obtain
2
exp(tv)(x,
u) = (x
cost
- u
sint,
x
sint
+u
cost).
Example 29.2.11 calculated the firstproloogation of SO (2). So
(1) (
Sint+UICOSl)
pr
exp(tv),(x,U,Ut)=
xcost-usint,xsint+ucost,
.'
cost
-"1
smr
Differentiating the components withrespect to t at t = 0 gives
i(XCost-usint)1
=-u,
at
1=0
~(XSint+ucost)1
=x,
at
1=0
a (Sint+UICOSl)1 2
- .
=I+u.
at
cost
-"1
sm r t=O 1
Therefore,
a a a
pr(l)v
=
-u-
+x-
+(1
+uI)-.
ax
au
aU!
Note that the first two terms in pr(l)v are the
same
as those in v itself, in agreement with
Box 29.2.12.
..
20
ne can, of course, also write the finite group element directly.

29.3
THE
CENTRAL
THEOREMS
951
29.3 The CentralTheorems
We are now in a position to stale the first central theorem
of
the application
of
Lie
gronps to the solution
of
DEs. This theorem is the exactreplica
of
Theorem29.1.6
in the language
of
prolongations:
29.3.1. Theorem. Let
v
= 1,
...
,1,
be a system
of
DEs defined on M C X x U whose Jacobian matrix
Cn)
_
(aA
v
8A
v
)
Ja(x,u
)=
--.
'-a-
ax'
au]
has rank Ifor all (x,
u(n»)
E
SD..
JfG
is a local Lie group
of
transformations acting
onM,and
v = I,
...
, I, whenever A (x, u
C
n»)
=0
for every infinitesimal generator
e
of
G, then G is the symmetry group
of
the
system.
29.3.2. Example. Considerthefirstorder(so,n = I) ordinaryDE
A(x,u,
Uj)
= (u
_x
3
- u
2x)Uj
+x+x
2u
+u
3
=0,
so that X
~
JR
and U
~
JR.
Wefirstnote that
(
aA aA
aA)
J.6,
=
B;'
a;;'
aUl
=
«-3x
2
_ u
2)Uj
+I +
2xu,
(I - 2ux)Uj +x
2
+3u
2,
U_ x
3
_ u
2x),
which
is of
rank
1
everywhere.
Nowletus
apply
the
first
prolongation
of the
generator
of
SO
(2)---<:a1culated
in Example
29.2.14-to
A. Wehave
aA aA aA
pr(l)v(A)
=
-u-
+x-
+
(l
+UT)-
ax au aUj
=
-U[(
-3x
2
- u
2)Uj
+I +2xu] +x[(1 - 2ux)Uj +x
2
+3u
2]
+(I +uT)(u - x
3
- u
2x)
= Uj[(u - x
3
- u
2x)Uj
+x
+x
2u
+u
3]
=
ujA.
It followsthat pr(l)v(D.) = 0 wheneverA = 0, andthat SO(2) is a symmetrygroupof the
DE.
Thus,
rotations
will
change
solutions
oftheDEinto
other
solutions.
Infact,the
reader
mayverify
that
in
polar
coordinates,
theDEcanbe
written
inthe
incredibly
simple
form
dr
3
dB
=r
.
andthe
symmetry
of theDE
conveys
thefact
that
adding
a
constant
to (} doesnot
change
the polar form of theDE. II

952
29. LIE
GROUPS
AND DIFFERENTIAL
EQUATIONS
Theorem 29.3.1 reduces the invariance
of
a system of DEs to a criterion in-
volving the prolongation
of
the infinitesimal generators of the symmetry group.
The urgent task in front
of
us is therefore to construct an explicit formula for the
prolongation
of
a vector field.
In
order to gain insight into this construction, we
first look at the simpler case of U
~
IR
and a group G that transforms only the
independent variables. Furthermore, we restrictourselves to the first prolongation.
An infinitesimal generator of such a group will be of the form
p . a
v=
LX'(x)-.,
i=l
ax
l
which is assumed to act on the space M C X xu. The integral curve
of
this vector
field is
exp(tv)
which acts on points of M as follows:
(E,
it)
=
exp(tv)·
(x, u) sa
(W,(x),
u) es
(W;(x),
u).
By the construction of the integral curves in general, we have
dW;(x)
I =
Xi
(x).
dt
,~O
(29.15)
(29.16)
Denote the coordinates of the first
jet
space
M(ll
by
(xi,
u, Uk), where
Uk(j;
f)
sa
aflax
k
.
By the definition of the action of the prolonged group,
pr(l)
exp(tv)
.
(j;
f)
= (W,
(x),
u, it
[),
whereu
=
!(x)anditj
= a!/ai
j
. Once we find
itj,
wecan differentiate Equation
(29.16) with respect to
t at t = 0 to obtain the prolonged vector field. Using
Equation (29.10) and commas to indicate differentiation,3 we obtain
Since v does not have any component along
a/au,
its prolongation will not have
such components either. The components
Uj
along
a/aUj
are obtained by differ-
3Wehavefound it exceedingly
convenient
to use
commas
to
indicate
differentiation
withrespecttothex's. The
alternative,
i.e.,theuseof
partials,
makesitalmostimpossible to findone's wayin themazeof
derivatives
involving
xi
•
ii,
andt, with:xi
depending
ont. The
reader
will recall
that
theindex
after
thecommais tobe
thought
of asa
"position
holder,"
andthe
argument
asa
SUbstitution.
Thus,for
example,
alesl,''''
sp) I
~
alcol,,,.,
Op)
I .
OSj S=X aOj O='x
