Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations
Подождите немного. Документ загружается.

29.4
APPLICATION
TO
SOME
KNOWN
PDES
963
The
Uij
in this equation are all independent; so, we can set their coefficients equal
to zero:
X
(!) - X(2) - X(3)
t - 2 - 3
(29.34)
(29.35)
The reader may verify that these relations imply that
xj'11
= 0 for
any
i,
j,
k, and
I. For example,
X~~2
=
X~~2
=
Xj~2
= -
Xj~3
= -
X~~3
= -
X~?3
'-v--'
'-.-'
'-.-'
'-v--'
::=Xg'3
=xg~
=xj~~
=xji>r
= -
x~;),
= -
Xm
=
-X~~2'
- -
=X~~l
=xWz
So, the first link
of
this chainis equalto its negative. Therefore, all the third deriva-
tives in the chain
of
Equation (29.35) vanish.
It
follows that all Xli) 's are mixed
polynomials of at mostdegreetwo. Writing the mostgeneral such polynomials for
the three functions
X(l),
X(2), and X(3) and having them satisfy Equation (29.34)
yields
X(l)
=
a,
+a4X +a7Y +
ast
+
as(x
2
+i +t
2)
+
2a9xt
+
2alQyt,
X(2) = a2 +
asx
- a6Y +a4t +
a9(x
2
-
i +t
2)
+
2alQxy
+
2asxt,
X(3) = a3 +a6X +
asy
+a7t +alQ(
_x
2
+i +t
2)
+2U9xy +2aSyt.
(29.36)
Settingthe coefficientof
Uk and
~ij
Uij equalto zero and usingEquation(29.33)
gives
2a = X(2) +X(2) _ X(2)
x
xx
yy
ttl
2a
=
X(!)
-
X(l)
-
X(!)
t tt xx
yy'
2a
=
x(3)
+X(3) _ X(2)
y xx yy
ttl
fJtt
-
fJxx
-
fJyy
=
O.
It
follows that
fJ
is any solution of the wave equation, and
a(x,
y,
t)
=
au
-
ast
- a9x - alQY.
By inserting the expressions found for Xli) and U in (29.30) and writing the
result in the form
Li
aiVi,
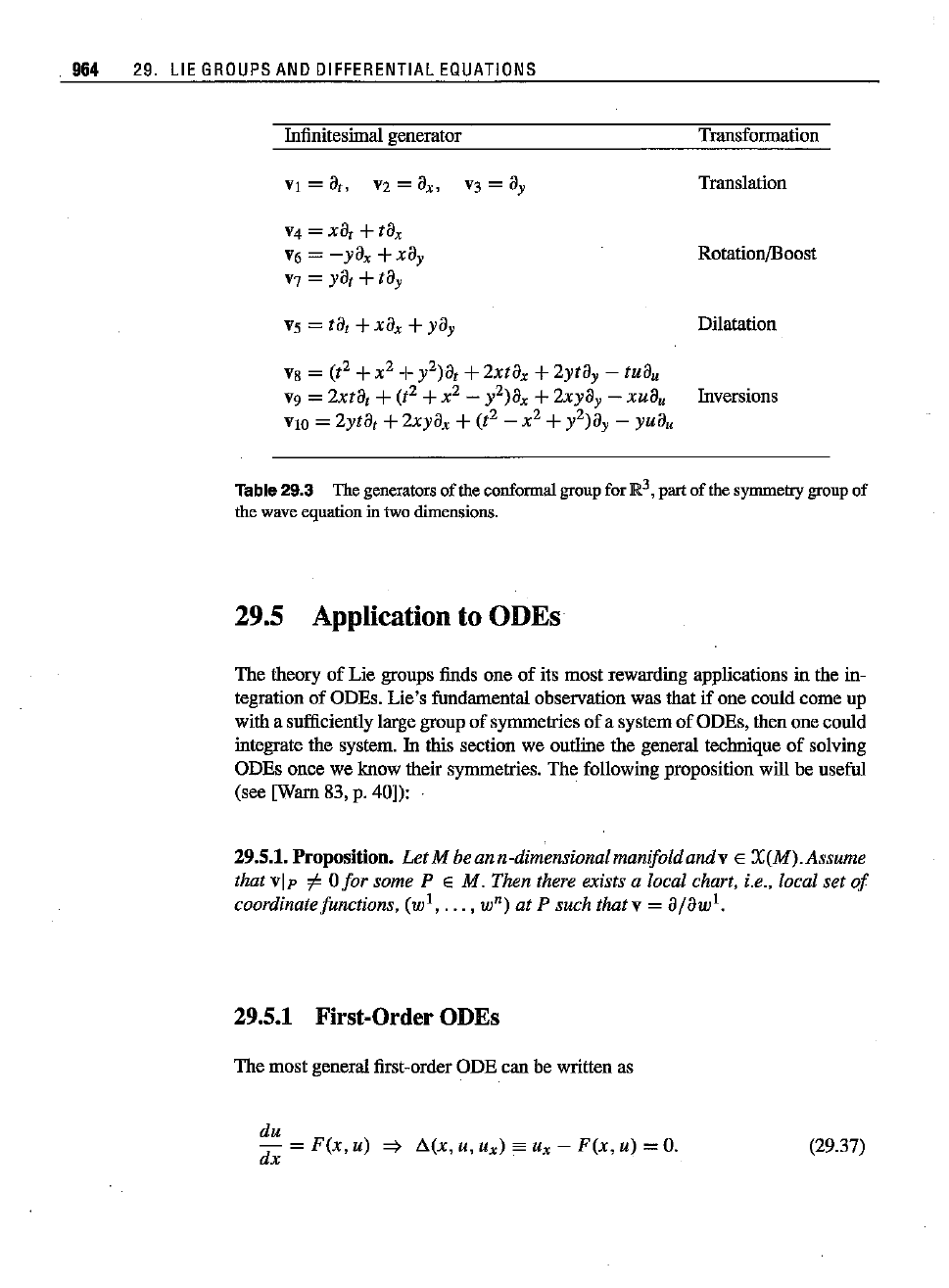
we discover that the generators of the symmetry group
consist
of
the ten vector fields given in Table 29.3 as well as the vector fields
Ua
u
, vp = fJ(x, y,
t)a
u
for
fJ
an arbitrary solution
of
the wave equation. The ten vector fields of Table
29.3 comprise the generators of the conformal group in three dimensions whose
generalization to
m dimensions was studied in Section 28.4.
964 29. LIE
GROUPS
AND DIFFERENTIAL
EQUATIONS
Infinitesimal generator
VI =
a"
V2 = ax, V3 = ay
Transformation
Translation
V4
=xa,
+tax
V6 =
-ya
x
+
xa
y
Rotation/Boost
V7 = ya, +tay
Vs
= ta, +
xa
x
+yay Dilatation
VB = (t
2
+x
2
+y2)a, +
2xta
x
+
2yta
y
- tuau
V9 =
2xta,
+(t
2
+x
2
-
y2)a
x
+
2xya
y
-
xua
u
Inversions
VlO =
2yta,
+
2xya
x
+(t
2
-
x
2
+y2)a
y
-
yua
u
Table29.3 Thegenerators oftheconformal group
for]R3
,partofthesymmetry groupof
thewaveequationintwodimensions.
29.5 Application to ODEs
The theory
of
Lie gronps finds one
of
its most rewarding applications in the in-
tegration of ODEs. Lie's fundamental observation was that if one could come up
with a sufficiently large group
of
symmetries
of
a system
of
ODEs, then one could
integrate the system. In this section we outline the general technique
of
solving
ODEs once we know their symmetries. The following proposition will be useful
(see [Wam 83, p. 40]):
29.5.1.Proposition.
LetM
be an n-dimensionalmanifold
andv
E
X(M).Assume
that vip
t=
0
for
some P E M. Then there exists a local chart, i.e., local
set
of
coordinatefunctions,
(wI,
...
, w")
at
P such that v =
a/awl.
29.5.1 First-Order ODEs
The most general first-order ODE can be written as
du
dx
=
F(x,
u)
=}
Ll.(x, u,
ux)
es U
x
-
F(x,
u) =
O.
(29.37)

29.5 APPLICATION
TO
ODES
965
u(x)
es U» +(Uu -
Xx)u
x
-
Xuu~,
(29.38)
where
A typical infinitesimal generator
of
the symmetry group
of
this equation is" v =
X
ax
+u
au,
whose prolongation is
a
pr(l) =
v+
u
ex
)_ ,
au
x
asthe reader
may
verify.
The
infinitesimalcriterionfor the one-parametergroup
of
transformations G to be a symmetry group
of
Equation (29.37) is pr(1)v(t» = 0,
or
au
+(au _ ax)F_ ax
F2
= x
aF
+u
a
F.
(29.39)
ax
au
ax
au
ax
au
Any solution (X, U)
of
this equation generates a I-parameter group
of
transfor-
mations. The problem is that a
systematic procedure for solving (29.39) is more
difficult
than solving the original equation. However, in
most
cases,
one
can guess
a symmetry transformation (based on physical,
or
other, grounds), and thatmakes
Lie's method worthwhile.
Suppose we have found a symmetry group G with infinitesimal generator v
that does not vanish at
P E M C X x U. Based on Proposition 29.5.1, we can
introduce
new coordinates
w =
~(x,
u),
y =
~(x,u)
(29.40)
in a neighborhood
of
Psuch that v = a
jaw,
whose prolongation is also a
jaw
[see (29.38)]. This transforms the DE
of
(29.37) int0
5
2i.(y, w, w
y
) = 0, and the
infinitesimal
criterion
into
pr(1)v(ii) = a
ii
=
o.
aw
It
follows that
ii
is independent
of
w.
The
transformedDE is therefore
ii
(y, w
y
)
=
0, whose normal form, obtained by implicitly solving for
dwjdy,
is
dw
l
Y
- =
H(y)
"*
w
==
H(t)dt
-
w(a)
dy
a
for some function H
of
y alone and some convenientpoint y = a. Substituting this
expression
of
was
a function
of
y in Equation (29.40) and eliminating y between
the two equations yields
u as a function
of
x.
Thusour taskisto find the change
of
variables (29.40). For this, we use
v(w)
=
I and v(y) = 0, and express
them
in terms
of
x and u:
.
a~ a~
v(w)
=v(~)
=
X-
+
u-
= I,
ax
au
a~
a~
v(y) =
v(~)
= X
ax
+u
au
=
O.
(29.41)
4The
reader
is
warned
against
the
unfortunate
coincidence of
notation:
X and U
represent
boththe
components
of the
infinitesimal
generator
andthespacesof
independent
and
dependent
variables!
5Herewe arechoosingw to bethe
dependent
variable.
Thischoiceis a
freedom
thatis always
available
tous.

966 29. LIE
GROUPS
AND
DIFFERENTIAL
EQUATIONS
The second equation says that
ry
is an invariant
of
the group generated by v. We
therefore
nse
the associated characteristic
ODE
[see (29.3) and (29.5)] to find y
(or
ry):
dx
du
=
X(x,
u)
U(x,
u)
(29.42)
To find
W (or
s),
weintrodnce
X(x,
u, v) = v
-Hx,
u)
and
note thatan eqnivalent
relation containing the same information as
the
first eqnation in (29.41) is
x
ax
+U
ax
+ aX
=0,
ax au av
which
has
the
characteristic
ODE
dx
du
dv
X(x,
u) =
U(x,
u) =
T'
(29.43)
for which we
seek
a solntion
of
the form v - !;(x, u) = c to
read
off
Hx,
u).
The reader
may
wonder whether it is sane to go through so
much
trouble
only
to replace the original single
ODE
with two
ODEs
such as (29.42)
and
(29.43)1
The
answer
is
that
inpractice, the
latter
twoDEsaremucheasierto solve
than
the
original ODE.
29.5.2.
Example.
The homogeneous FODE
dufdx
= F(u/x) is invariant under the
scaling
transformation (x, u) t-+ (sx, su) whose
infinitesimal
generator
isv = xa
x
+ua
u.
The
first
prolongation
ofthis
vector
is thesameasthe
vector
itself
(reader,
verify!).
Tofiodthe new coordinatesw and y, firstuse Equation (29.42) with
X(x,
u) =x and
U(x,
u) = u:
. dx
x
du u u
=>
- = C1
=>
Y = - (see Box 29.1.8).
u x x
Next,we note that (29.43)yields
dx du .
- = - = dv
=>
Inu = v
+luC2
=>
v = lu(u/C2)'
x u
Substituting
from
theprevious
equation,
we
obtain
v = lu(CjX/C2) = lux + lu(CJ/C2)
=>
v
-lux
= C
=>
w =
lux.
The chain rule gives
duldx
= (1 +
yWy)/Wy.
so that theDE becomes
1+
YWy =
F(y)
=>
dw
= 1 ,
Wy
dy
F(y)-y
whichcan be integratedto give w =
H(y)
or
lux
=
H(y)
=
H(u/x),
whichdefinesu as
animplicit
function
ofx. II

29.5 APPLICATION
TO
ODES
967
29.5.2 Higher-OrderODEs
The
same argument used in the first order
ODEs
can be used for higher-order
ODEs to reduce their orders.
29.5.3.
Proposition.
Let
,;(x,
u(n)) =
,;(x,
u,
UI,
...
, un) =0,
be an nth order ODE.
If
this ODE has a one-parametersymmetry group, then there
exist variables w
=
~(x,
u)
and
y =
~(x,
u) such that
A(
(n)
_ -
(dw
dnw)
_ 0
L3. X, U ) _ /),. y,
-,
...
,
--
- ,
dy dy"
i.e., in terms
ofw
and y, the ODE becomes
of
(n - I)st order in W
y
•
Proof
The
proof
is exactly the same as in the first-order case.
The
only difference
is that one has to consider pr(n)y, where y
=
8/8w.
But
Problem 29.7 shows that
pr(n)v
= v, asin the
first-order
case. 0
29.5.4.
Example.
Considera second-orderDE
Ll.(u,
ux, uxx) = 0, which does not de-
pendonx
explicitly.The fact that
aa/ax
= osuggests w = x. So, we switchthe dependent
and
independent
variables
andwritew = x, andy = u.
Then,
usingthechainrule,we get
du 1
dx = Wy
Snbstitutingin the originalDE,we obtain
_ ( 1
wYY )
~(y,
Wy,
W
y
y)
==
A
y,
-,
--3
= 0,
Wy w
y
whichis of
first
order
in Wy.
..
29.5.5.
Example.
Theorderof the SOLDE
Uxx
+ p(x)ux +q(x)u = 0 can be reduced
by
noting
that
theDEis
invariant
under
thescaling
transformation
(x, u)
I->
(x, su),whose
infinitesimal generatoris v =
ua
u
•
Withthis vector field,Equations(29.42) and (29.43)
give
dx du
0=-;;-
dx = du = dv.
o u
Forthe
first
equation
tomakesense,we havetohave
dx = 0
~
x = CI
~
Y= x (byBox 29.1.8).
Thesecond
equation
inu gives
v=lnu+c::::}
v-lnu=c
=>
w=lnu
=>
u=e
w
.

968 29. LIE
GROUPS
AND
DIFFERENTIAL
EQUATIONS
Usingthe
chain
rule,we
obtain
dw w dw w
ux w
rr-e
=-e
dx dy
and
Riccati
equation
By
inserting
thisin the
original
DEand
writing
z = wy, we
obtain
dz 2
dy =
-Z
- p(y)z - q(y),
whichis the
well-known
first-order
Riccatiequation.
29.5.3 DEs with Multiparameter Symmetries
III
We have seen that I-parameter symmetries reduce the order of an ODE by 1.
It
is natnral to suspect that an r-parameter symmetry will reduce the order by r.
Althoughthis suspicioniscorrect,it turns
out
that in general,one cannotreconstrnct
the solution
of
the original equation from those of the reduced (n - r )th-order
equation. (See [Olve 86, pp. 148-158] for a thorough discussion of this problem.)
However, the special, but important,case
of
second-orderDEs is an exception. The
deep reason behind this is the exceptional strnctnre
of
2-dimensionalLie algebras
given in Box 27.2.5. Wecannotaffordto go into details
of
thereasoning, but simply
quote the following important theorem.
29.5.6.Theorem. Let
~(x,
urn»)
= 0 be an nth-order ODE invariant under a
2-parametergroup. Then there isan (n - 2)nd-order
ODE
li(y,
w(n-2») = 0 with
theproperty that the general solution to
~
can be
found
by integrating the general
solution to
li.
In particular, a second-order ODEhaving a 2-parameter group
of
symmetries can be solved by integration.
Let us analyze the case
of
a second-order
ODE
in some detail. By Box 27.2.5,
the infinitesimal generators
VI and V2 satisfy the Lie bracket relation
c=Oor1.
We shall treat the abelian case (c = 0) and leave the nonabelian case for the
reader.To begin with, we use
sand
t for the transformed variables, and at the end
replace them with
y and w.
By Proposition 29.5.1, we
can
let VI =
a/as.
ThenV2 can be expressed as the
linearcombination
a a
V2 = ",(s,
t)-
+P(s,
t)-.
as at
The commutation relation
[VI,
V2] = 0 gives
a", ap
0=
[a"
",a, +
pa,j
=
-at
+
-a"
as as

29.5
APPLICATION
TO
ODES
969
showing that a and
fJ
are independent
of
s, We want to simplify
V2
as mnch as
possible without changing Vt.
A transformation
that
accomplishes this is S =
s +
h(t)
and T = T'tt), Then, by Equation (26.8) we obtain
a a
as
a ar a a
vi = Vj(S)
as
+vr(T) aT = as
as
+
a:;;-
aT =
as'
V2
=
V2(S)~
+
v2(T)~
= (a
as
+
fJ
as)
~
+
(aaT
+
fJ
aT)
~
as
aT as at
as
as at er
(
, a , a
= a +
fJh
)
as
+
fJT
ar
If
fJ
'" 0, we choose T' =1/
fJ
and h' =
-a/
fJ
to obtain
a
V2=-,
at
(29.44)
where we have substituted
s for S and t for T.
If
fJ
=0, we choose a =T, and
change the notation from S to
s and T to t to obtain
a
V2
=t-.
as
(29.45)
The next step isto decidewhichcoordinateis the independentvariable, prolong
the vectorfields, and apply
ittothe
DE to find the infinitesimalcriterion.
For
fJ
'" 0,
the choice is immaterial. So, let
w = s and y = t. Then theprolongation
of
Vj
and
V2
will be the same as the vectors themselves, and with !;.(y, w, w
y,
w
yy)
es
W
yy
- F(y, w, w
y),
the infinitesimal criteriafor invariance will be
2
a!;.
aF
0=
pre
)Vj(!;.) = vi (!;.) = - =
--,
aw aw
2
a!;.
aF
0=
pre
)V2(!;.) =
V2(!;.)
= - =
--.
ay ay
It
follows that in the (y, w) system, F will be a function
of
w
y
alone and the DE
will be
of
the form
dw
y
W
yy
=F(w
y)
=}
dy =F(w
y)
f
WY
~
=}
F(z)
.
~
=H(wy)
The
last equation can be solved for w
y
in terms
of
y and the result integrated.
For
fJ
= 0, choose w = t and y = s, Then Vj will not prolongate, and as the
reader
may
verify,
(2) 2
a
a a
2a
a
pr
V2
=
V2
- W - - 3w
ywy
y--
=
w-
- w
y-
-
3wywYY-aw
'
Yaw
y
aW
yy
ay aw
y
yy

970 29. LIE
GROUPS
AND
DIFFERENTIAL
EQUATIONS
and the infinitesimal criteria for invariance will be
. 2
aL'.
aF
0=
pr(
)VI
(L'.)
= VI (L'.) = - =
--,
ay ay
er
aF
0=
pr(2)v2(L'.)
=
-w
-
+w;-
+3wy W
yy
•
ay aw
y
_
'-..-'
=F
~O
It
follows that in the (y, w) system, F will be a function of w and
wyand
satisfy
the DE
aF
w
y
- = 3F,
aw
y
whosesolutionisoftheforrnF(w,
w
y)
=
w~F(w).
The original DE now becomes
3 -
W
yy
=
wyF(w),
for which we use the chain rule W
yy
= WyaWy/aw to obtain
dw
y
2 - 1 I
W
- dw 1
dw =
wyF(w)
=}
- w
y
=
F(z)dz
=}
dy = -
H(w)'
'-.----'
wH(w)
which can be integrated. Had we chosen w =s and y =t, F wonld have been
a function of
y and the DE wonld have reduced to W
yy
=
F(y),
which conld be
solved by two consecutive integrations. The nonabelian 2-dimensional Lie algebra
can be analyzed similarly. The reader may verify that
if
fJ
= 0, the vector fields
can be chosen to be
a
VI = as'
a
V2
=S-,
as
(29.46)
leading to the ODE
W
yy
= wyF<Y), and if
fJ
i'
0, the vector fields can be chosen
to be
a
VI
=-,
as
a a
V2=S-+t-,
as
at
(29.47)
leading to the ODE W
yy
=
F(wy)/y.
Both of these ODEs are integrable as in the
abelian case.
29.6 Problems
29.1. Suppose that {Fi
li'=1
are invariants
ofthePDE
(29.3). Show that any function
f
(FI,
F2,
...
, F
n
)
is also an invariant of the PDE.

and
Korteweg-de
Vries
equation
29.6
PROBLEMS
971
29.2. Find the function 1 =
IJ
. I when
I(x)
= ax +b and
IJ
is the angle
of
rotation of
80(2).
29.3. Use the resnlt
of
Problem 29.2 to find UI. Hint: Note that a =
UI.
29.4. Transform the DE
of
Example 29.3.2 from Cartesian to polarcoordinates to
obtaindrjdlJ
= r
3
.
29.5. Using the definition
of
total derivative, verify Eqnation (29.21).
29.6. Show that
80(2)
is a symmetry group
of
the first-order DE
L\.(x, u,
UI)
= (u -
X)UI
+X +U =0
and write the same DE in polar coordinates.
29.7. Show that the
nth
prolongation
of
the generator
of
the
ith
translation.
ai,
is
the same as the original vector.
29.8. Find the first prolongation of the generator
of
scaling: xa
x
+ua
u
•
29.9. Show that when the group acts only on the single dependent variable u, the
prolongation of
v = U
au
is given by
P a
au au
pr(l)v=v+
LUj-,
where U· =
-.
+U·-.
j=l
aUj ) ax} } au
29.10. Show that the nth prolongation
of
v =
X(x,
u)a
x
+
U(x,
u)a
u
for an
ordinary DE of
nth
orderis
n a
pr(n)V
= v +
"U[kl
__
c:
aU(k)'
k=l
where
(k) _
aku
u
--
- ax
k
29.11. Compute the second prolongation
of
the infinitesimal generators of the
symmetry group
of
the heat equation.
29.12. Derive Equations (29.31) and (29.32).
29.13. Using Equation (29.34) show that
XjZl
= 0 for any i,
j,
k, and I.
29.14. The
Korteweg-de
Vries equation is u, +U
xxx
+uU
x
=
O.
Using the
technique employedin computing the symmetries of the heat and wave equations,
show that the infinitesimal generators
of
symmetries of the Korteweg-de Vries
equation
are
VI = a
X I
V2 =
8"
V3 = tax +au,
V4 = xa
x
+
31a,
- 2ua
u
·
translation
Galilean boost
scaling

972
29. LIE
GROUPS
AND
DIFFERENTIAL
EQUATIONS
29.15. Suppose
M(x,
u)
dx-s-Nix,
u) du =
Ohasa
I-parametersymmetry group
with generatorv = xa
x
+
ua
u
.
Show that the function
q(x,
u) =
I/(XM
+
UN)
is an integrating factor.
29.16. Show that the second prolongation of v = Way (with y treated as indepen-
dent variable) is
pr(2)v = v _ w
2_
a
_ _
3w
yw
y
y_
a_.
Yaw
y
aW
yy
29.17. Go through the case
of
f3
= 0 in the solution of the second order ODE
and, choosing
w = s and y = t, show that F will be a function
of
y alone and the
original DE will reduce to W
yy
=
F(y).
29.18. Show that in the case of the nonabelian 2-dimensional Lie algebra,
(a) the vector fields can be chosen to be
a
VI
=-,
as
a
Vz
=8-
as
if
f3
=
O.
(b) Show that these vector fields lead to the
ODE
W
yy
=
wyF(y).
(c)
If
f3
'i-0, show that the vector fields can be chosen to be
a
VI
=-,
as
a a
V2 =
s-
+t-.
as
at
(d) Finally, show that the latter vector fields lead to the
ODE
Wyy =
F(wy)/y.
Additional Reading
I. Blurnan, G. and Kumei, S. Symmetries
and
DifferentialEquations, Springer-
Verlag, 1989. A readable book on the subject
of
this chapter with many
worked-out examples.
2. Olver, P.
Application
of
Lie Groups to Differential Equations, Springer-
Verlag, 1986. Our treatment of the subject follows closely that
of
Olver.
This self-contained book, although formal, is very lucid in style with many
historical notes. All the concepts introduced are clearly stated and many
examples introduced to clarify them.
3. Stephani,H.DifferentialEquations: Their solutionsusing symmetries, Cam-
bridge University Press, 1989. Written by a physicist, this little book treats
solutions of ordinary differential equations in great detail with very little
formalism.
