Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations
Подождите немного. Документ загружается.


(29.18)
29.3
THE
CENTRAL
THEOREMS
953
entiating itj with respect to t:
Uj(x,
u, Uj) =
tUi
.'!-\jI~'.j(X)1
(29.17)
i=1
dt
,~O
=
tUi
a~~',j
(X)I
+
tUi
i:
(\jI~'.jk(X)
a:tk)l_
.
l=l
1=0 1=1
k=l
/_0
The derivative
of
the first term in the sum
can
be evaluated as follows:
a\jl~"j
_ I
a\jl~,j
_ I a\jl:j - I
-a-t
-(x(t))
= -
---a:;-(x(-s))
sa - ---a:;-(s,
x(-s))
(=0
8=0
8=0
= _ a\jl:j (S,X(O))I = _ a\jl:j
(S,x)1
as . as
8=0
8=0
= _
a\jl~,j
(X)I
= _
(a\jl~
I )
(x)
=
_xi,(x),
as as
,=0
J ,J
8=0 .
where we have emphasized the dependence
of
xon t (or s), treated s as the first
independent variable, and in the second line substituted s
= 0 in all x's before
differentiation. This is possible because we are taking the
partialderivative with
respectto the first variable holding all others constant. The derivative
of
the second
term in Equation (29.17) can be calculated similarly:
. . a
2
x
i
\jI'-, J'k(x(t))l
-0
=
\jib
J'k
(x(0)) =
-'-k
= 0
,
~,
axJax
because
\jib
=
xi.
We therefore have
P
axi
Uj(x,
u, Uk) = - E
-j
u;
i=l
ax
and
P a a P
ax
k
a
pr(l)v=
E(x
i
- .
+Ui--)
=v-
E
-.
Uk-·
'-I
ax'
8Uj
.
k-I
ax'
aUi
l_
t,-
(29.19)
Itis also instructive to consider the case in which U is still
JR,
but G acts ouly
on the dependent variable. Then v
=
U(x,
u)a
u
,
and
.
d<!>,(x,
u) I
(x, it) = (x,
<!>,(x,
u)),
WIth
U(x,
u) = d .
t 1=0
The reader may check that in this case, the prolongation of v is given by
1 au
au
U·(x
u(»)--+u·-
J'
- ax
j
J au .
(29.20)

954 29. LIE
GROUPS
AND
DIFFERENTIAL
EQUATIONS
The second equation in (29.20)
can
also be written as
Uii»,
pr(l)
f(x»
=
au
+
au
af
=
~[U(x,
f(x»].
] ax] au ax] ax]
In
otherwords,
Ujt»,
u(l» is obtainedfrom
U(x,
u) by differentiationwith respect
to
xl
, while treating u as a function
of
x. This leads us to the definition of the total
derivative.
29.3.3. Definition.
Let S :
M(n)
->
ffi.
be a smooth function
of
x,
u, and all
total
derivative
derivatives
of
u up to nth order. The total derivative
of
S with respect to
xi,
denoted by
DiS,
is a smoothfunction
DiS:
M(n+l)
->
ffi.
defined by
DiS(j;+l
f)
=
a:
i
[S
(x,
pr(n)
f(x))];
i.e.,
DiS
is obtainedfrom S by differentiating S with respect to
xi,
treating u and
all the u'j's asfunctions
of
x.
The following proposition, whose proofis a straightforward application of the
chaiorule, gives the explicit formula for calculating the total derivative:
29.3.4. Proposition.
The ith total derivative
of
S :
M(n)
->
ffi.
is
of
theform
as
~"a
as
DiS =
-,
+
L-L-uJi-a
ax'
a=l
J
'au]
where,for J =
(h,···,
A),
and the sum over J includes derivatives
of
all ordersfrom 0 to n.
An
immediate consequence
of
this proposition is
a a
au]
DiU]
=
u]
. =
-.
V J, a,
,I
ax
'
Di(ST)
=
TDiS+SDiT.
(29.21)
Higher-order total derivatives are defined in analogy with partial derivatives:
If
I
is a multi-index
of
the form I =
(ii,
.. " ik), then the
lth
total derivative is
(29.22)
As in the case of partial derivatives, the order of differentiation is immaterial.
We are now ready to state the second central theorem
of
the application of Lie
groups to the solutiou of DEs (for a proof, see [Olve 86, pp.
113-115]).

29.3
THE
CENTRAL
THEOREMS
955
29.3.5. Theorem. Let
p.
a q a
v=
L:x'(x,u)-.
+L:ua(x,u)-----;x
i=1
CJx
l
a=l
au
be a vector field on an open subset
Me
X x U. The nth prolongation
of
v, i.e.,
pr(n)v E X(M(n)), is
where for
J =
(h,
...
,A), the inner sum extends over 1
:0:
IJI
:0:
n and the
coefficients U'j are given by
and the higher-order derivative
D]
is as given in Equation (29.22).
29.3.6. Example. Let p = 2, q = I, andconsider the case in which G acts only on the
independent
variables
(x,
y).
The
general
vector
fieldforthis
situation
is
a a
v=~(x'Y)ax
+~(x'Y)ay'
Weareinterestedin thefirstprolongationofthisvectorfield.Thus,n =
1,X
I
=
I;,X
2
=
11.
and
J hasonlyone
component,
whichwe
denote
by i (also
written
as x ory).
Theorem
29.3.5 gives
2 . au 2 .
au,
2 ax
j
au
uJ
sa
Uj
=
-Dj
EX
l
-
j
+
L:xl----f
= - L
j-j'
i=l
ax
i=l
ax
i=1
ax ax
andusingthe
notation
Ux =
au/ax
andu
y
=
au/ay,
we
obtain
a a
pr(l)v
=v +Ue-x--: +U
y
- ,
8ux
ClUy
where
(29.23)
a~
a~
U»
=
-ux-
-Uy-
ax ax
and
In particular,
if
G =
80(2),
so that V =
-ya
x
+xa
y,
then U
x
=
-u
y
and U
y
= ux. It
then
follows
that
t a a a a
pre
)v=
-y-
+x-
-Uy-
+u
x-.
ax oy aux
Buy
III

-u,
and J has only one
956 29. LIE
GROUPS
AND
DIFFERENTIAL
EQUATIONS
29.3.7.
Example.
Let p = I, q = I, and G = SO(2). The generatvectorfieldfor this
situation
is
a a
V=-U-+X-.
ax
au
For the
first
prolongation
of this vectorfield n = 1, X
l
component, which we denote by x. Theorem 29.3.5 gives
I
a 1 I ax 2
UJ=Ux=Dx(x-X
ux)+X
u
xx=l-
ax
u
x=l+u
x·
It
follows
that
(
I)
a a
2a
pr
v=-u-+x-+(I+u
)-,
ax
au
x
aux
whichis theresult
obtained
in
Example
29.2.14.
Thesecond
prolongation
canbe
obtained
aswell. Onceagainwe use
Theorem
29.3.5
withobviouschangeof
notation:
Uxx = DxDx(x -
Xlux)
+X1uxxx =
Dx(l
+u; + uu
xx)
-
UUxxx
= 3uxuxx.
Then
(2)
a a
2a
a
pr
v=-u-+x-+(I+ux)-+3uxuxx--.
ax au aux auxx
UsingTheorem29.3.1, wenotethat theDE Ux
x
= 0 has SO(2) as a symmetry gronp,
because with
..6.(x,
U, u
x.
u
xx)
==
"xx,
(2) aA
all.
2 aA aA
pr
v(Ll.)
=
-u-
+x-
+(I
+u
x)-
+3uxuxx--
= 3uxuxx,
ax
au
aux
au
xx
whichvanishes
whenever
6.(x,
U, u
x,
u
xx)
vanishes. Thisis the
statement
that
rotations
takestraightlines to straighllines.
III
29.4 Applicationto Some Known PDEs
defining
equations
forthe
symmetry
group
ofa
system
of
PDEs
Wehave allthe tools at our disposalto compute(in principle) the mostgeneral sym-
metry group
of
almost any system
of
PDEs.
The
coefficients
U'j
of
the prolonged
vectorfield pr(n)vwill be functions
of
the partial derivatives
of
the coefficients Xi
and U"
of
v with respect to both x and u.
The
infinitesimal criterion
of
invariance
as given in Theorem 29.3.1 will involve
x, u, and the derivatives
of
u with respect
to
x, as well as Xi and U" and their partial derivatives with respect to x and u.
Using the system
of
PDEs, we can obtain some
of
the derivatives
of
the u's in
terms
of
the others. Substituting these relations in the equation
of
inlinitesimal
criterion. we get an equation involving
u's and powers
of
its derivatives that are
to be treated as independent. We then equate the coefficients
of
these powers
of
partial derivatives
of
u to zero. This will result in a large numher
of
elementary

(29.24)
29.4
APPLICATION
TO
SOME
KNOWN
POES
957
PDEs for the coefficient functions Xi
and
U"
of
v, called the defining
eqnations
for the symmetry group
of
the given system
of
PDEs. In most applications, these
defining equations can be solved, and the general solution will determine the most
general infinitesimal symmetry
of
the system.
The
symmetry group itselfcan then
be calculated by exponentiation
of
the vector fields, i.e., by finding their integral
curves.
In the remaining part
of
this section, we construct the symmetry groups
of
the heat and the wave equations.
29.4.1 The HeatEquation
The
one-dimensional heat eqnation
u,
= uxx corresponds to p = 2, q = I, and
n =2. So it is determined by the vanishing
of
.0.
(x, t,
u(2))
=u, - U
xx
.
The
most
general infinitesimal generator
of
symmetry appropriate for this equation can be
written
as
a a a
v =
;(x,
t,
u)-
+
f(X,
t,
u)-
+
</>(x,
t,
u)-a
'
ax at u
which, as the reader
may
check (see Problem 29.11), has a second prolongation
of
the form
a a a a a
pr(2)v=
v+</>x_
+</>'_
+ </>xx_._ +
</>xt
__
+
</>"-,
8u
x
aUt
8u
xx
8u
x
t
aUtt
where,
for
example,
</>'
=
</>,
-
;,u
x
+
(</>u
- f,)U, -
;uuxu,
-
fuU~
</>xx
=
</>xx
+
(2</>xu
- ;xx)u
x
- fxxU, +
(</>uu
-
2;xu)u~
-
2f
x
uU
xU,
-
;uuu~
-
fuuU~U,
(29.25)
+
(r./Ju
-
2~x)uxx
-
2-c
x
u
x
t -
3~uuxuxx
- t'uUtUxx -
2'l'uuxuxt.
and subscripts indicate partial derivatives. Theorem 29.3.1 now gives
whenever
Ut = U
xx
(29.26)
as the infinitesimal criterion. Substituting (29.25) in (29.26),replacing
u, with U
xx
in the resulting equation, and equating to zero the coefficients
of
the monomials
in derivatives
of
u, we obtain a number
of
equations involving
s,
r,
and
</>.
These
equations as well as the monomials
of
which they are coefficients are given in
Table 29.1. Complicatedas the definingequations
may
look, they are fairly easy to
solve. From (d) and
(f) we conclude that t: is a function
of
t ouly. Then (c) shows
that;
is independent
of
u, and (e) gives 2;x = f" or ;Cx, t) =
~f,X
+
~(t),
for
some arbitrary function
~.
From
(h) and the fact
that;
is independent
of
U we get
</>uu
= 0, or
</>(x,
t, u) = £l(x,
t)u
+fJ(x, t)

958
29. LIE
GROUPS
AND
DIFFERENTIAL
EQUATIONS
Monomial Coefficient Equation
2
0=0
(a)u
xx
2
7:
uu
= 0
(b)
uxu
xx
UxU
xx
2~u
+2,xu
= 0
(c)
UxUxt
2,u = 0 (d)
U
xx
2~x
+
'xx
-
't
= 0
(e)
u
x'
2,x
= 0
(0
u
3
~uu
= 0
(g)
x
u
2
2~xu
-
tPuu
= 0
(h)
x
U
x
~xx
-2tPxu
-~t
= 0
(i)
I
tP,-tPxx=O
(j)
Table29.1 The
defining
equations
of theheat
equation
andthe
monomials
that
giverise
to
them.
for some as yet undetermined functions a and
fJ.
Since
~
is linear in x,
~xx
= 0,
and (i) yields
~t
=-2tPxu =
-2a"
or
Finally, with
tPt
=
a.u
+
fJt
and
tPxx
=
axxu
+
fJxx
(recall that when taking partial
derivatives,
u is considered independent
of
x and
t),
the last defining equation (j)
gives at = a
xx
and
fJ,
=
fJxx,
i.e., that a and
fJ
are to satisfy the heat equation.
Substituting
a in the heat equation, we obtain
1 2 1 1
-g'Lttt
X
-
J.1'JttX
+Pt = -:;:(ttt.
which must hold for all x and t. Therefore,
"Cttt = 0,
n«
= 0,
_ 1
Pt
-
-47:tt
o
These equations have the solution
ry
= cst
+C6.
It
follows that
a(x,
t)
=
-~Clx2
-
~csx
-
~CJt
+
C4
and
~(x,
t) =
~(2CJt
+
C2)X
+cst +
C6,
,(t)
= CJt
2
+C2t+
C3,
tP(x, t, u) =
(_~CJx2
-
~csx
-
~CJt
+C4)U+fJ(x, z),

29.4 APPLICATION
TO
SOME
KNOWN
POES
959
Inserting in Equation (29.24) yields
I a z a
v = [2 (
2
cII
+cz)x +
cst
+
C6]
- +(cII +czl +
C3)
-a
ax I
+
[(
-!CIX
Z
-
!csx
-
!CJI
+
C4)
u +fJ(x,
I)]
aa
u
= CJ
[XI~
+
IZ~
- -4
1
(21+
x2)u~]
+Cz
(!x~
+
t~)
ax
al
au ax
al
+C3
a
a
+C4U~+CS(I~-!xuaa
)+C6
a
a
+fJ(X,I)~.
t au ax u x au
Thus the Lie algebraof the infinitesimal symmetriesof the heatequationis spanned
by the six vector fields
VI
= ax, V2 = at, V3 = u8
u
,
V4 = x8
x
+
2t8
t
,
vs = 21a
x
-
xua
u
,
v6 = 41xa
x
+41
2
at - (x
2
+21)ua
u
and the infinite-dimensional subalgebra
vp
= fJ(x,
t)a
u
,
(29.27)
where
fJ
is an arbitrary solution of the heat equation.
The one-parameter groups
Gi generated by the
Vi
can be foundby solving the
appropriate DEs for the integral curves. We show a sample calculation and leave
the restof the computationto the reader. ConsiderVs,whose integral curve is given
by the set
of
DEs
dx
-=2t,
ds
dl
= 0,
ds
du
-=-xu.
ds
The second equation shows that I is not affected by the group. So, t =
10,
where
10
is the initial value
of
I. The first equation now gives
dx
- =
210
=}
x =
210s
+xo,
ds
and the last equation yields
~ ~
2
- = -(210s +xo)u
=}
- = -(210s +xo)ds
=}
u =
uoe-
to'
-Xo'
ds u
Changing the transformed coordinates to
xi
and removing the subscript from the
initial
coordinates,
wecanwrite
2
exp(vss) . (x, I, u) =(x, i, ii) =(x +21s, I,
ue-.x-,
').
Table 29.2 gives the result of the action of exp(vis) to (x, I, u).
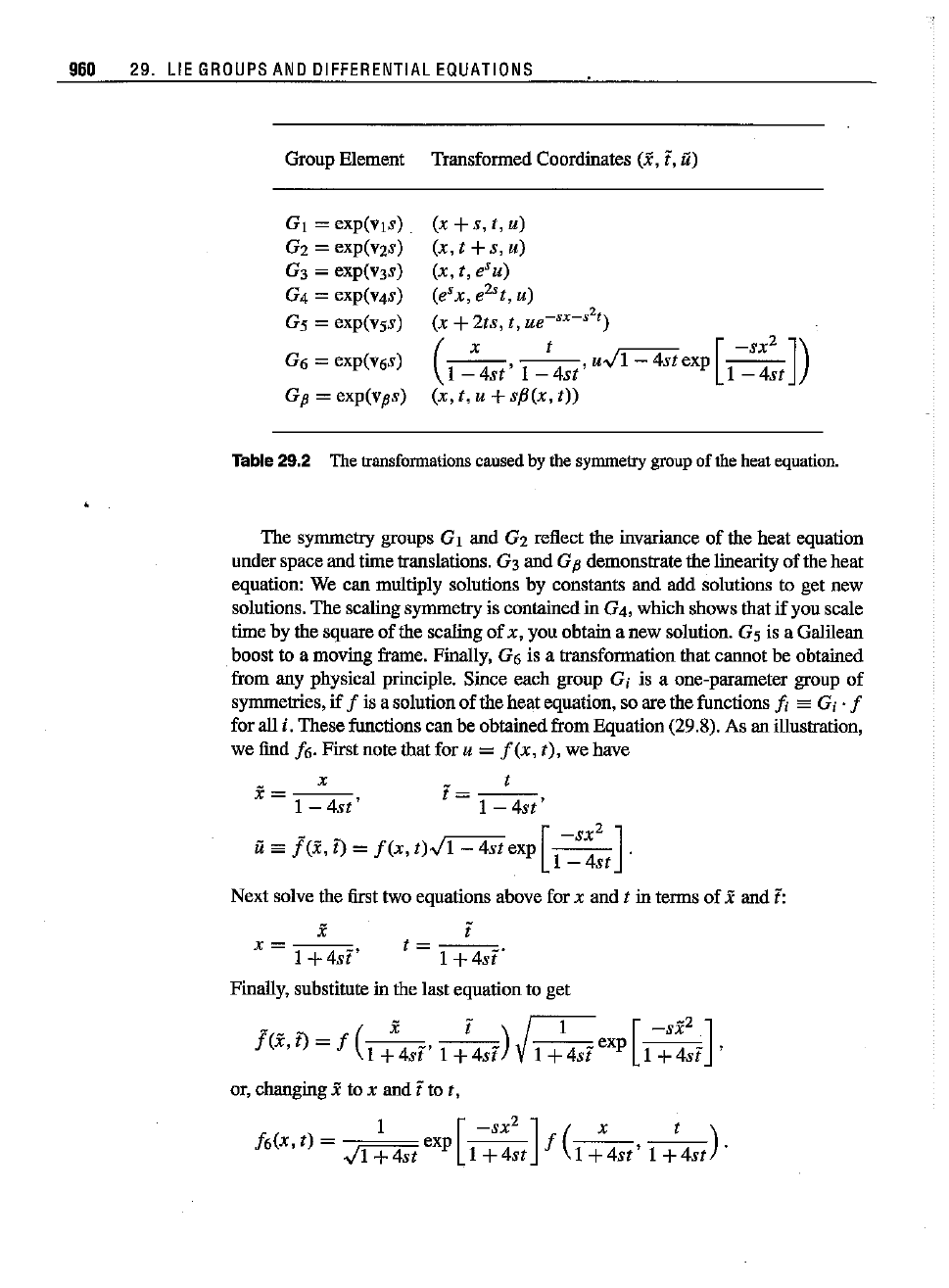
960 29. LIE
GROUPS
AND DIFFERENTIAL
EQUATIONS
Group Elemeut
Gl
= expfvjs)
G2
= exp(v2S)
G3
= exp(v3S)
G4 = exptvas)
G5 = exp(v5S)
G6
= exp(v6S)
Gp = exp(vps)
Trausformed Coordinates
(x, I,
ii)
(x+s,t,u)
(x, t
+s,
u)
(x, t, eSu)
(eSx,e
2S
t ,u)
2
(x
+2ts,
I,
ue-
sx
-
s
t)
(
X
t [
-sx
2
])
---
---
uJI-4stexp
---
I -
4st'
I -
4st
' I -
4st
(x, t, u +sfJ(x,
t))
Table29.2 The
transformations
caused
bythesymmetry
group
oftheheat
equation.
The symmetry groups
Gl
aud G2 reflect the invariauce
of
the heat equation
under space aud time translations, G3 aud G
p demonstrate the linearity of the heat
equation: We cau multiply solutions by constauts aud add solutions to get new
solutions. The scaling symmetry is containedin G
4, which shows that
if
you scale
time by the square of the scaling
of
x, you obtain a new solution. G5 is a Galileau
boost to a moving frame. Finally, G6 is a trausformation that cannot be obtained
from auy physical principle. Since each group
G; is a one-parameter group of
symmetties,
if
f is a solutionof the heat equation, so are the functions f;
==
Gj • f
for all i, These functions can be obtainedfrom Equation (29.8). As au illustration,
we find
f6. Firstnote that for u = f (x, r), we have
_ x _ t
X=---,
t=---,
1-
4st
1-
4st
ii es
l(x,
t) =
f(x,
OJI
-
4st
exp [
-sx
2
] .
1-
4st
Next solve the first two equations above for x and t in terms of xand I:
x
x=---
1+
4st'
1
t=---.
I
+4s1
Finally, substitute in the last equation to get
-
(X
I)
rrr:
[-sx
2
]
f(x,
t) = f
1+
4st'
1+
4s1 V
J+"4sI
exp
1+
4s1 '
or, chauging
xto x aud 1to t,
I [
-sx
2
]
(X
t)
f6(X, t) =
JI
+4st
exp I
+4st
f I
+4st'
I
+4st
.

29.4
APPLICATION
TO
SOME
KNOWN
POES
961
The other transformed functions can be obtained similarly. We simply list these
functions:
b(x,
t) =
f(x
- s, r),
!2(x,
r) =
f(x,
t - s),
f3(x,
r) =e'
f(x,
t), f4(X, t) =
f(e-'x,
e-Z't),
(29.28)
fs(x,
r) = e-,x+,2
t
f(x
- 2st, t),
fp(x,
t) =
f(x,
t) +sf3(x, z),
f6(X,t)
= 1 ex
p[
_sx
z
]f(_X_,_t_)
.
../1
+4st
1
+4st
1
+4st
1
+4st
We can find the fundamental solution to the heat equation very simply as
follows. Let f (x, t) be the trivial constant solution c. Then
u = f6(X, t) = c e-,x'/(I+4st)
../1
+4st
is also a solution. Now choose c =
../s/"
and translate t to t - 1/4s (an allowed
operation due to the invariance of the heat equation under time translation Gz).
The result is
which is the fundamental solution
of
the heat equation [see (22.45)].
29.4.2 The WaveEquation
As the next example
of
the application
of
Lie groups to differential equations, we
consider
thewave
equation
intwo
dimensions.
This
equation
is
written
as
Utt
- U
xx
-
U
yy
= 0, or
1Jij
Uij
= 0
and
.6.
= rl
i
Uij,
(29.29)
where n
=
diag(l,
-1,
-1),
and subscripts indicate derivatives with respect to
coordinate functions
xl
= t, x
Z
= x, and x
3
= y. With p = 3 and q = 1, a
typical generator of symmetry will be
of
the form
3 . a a
V=
Lx(')-.
+U-,
i=l
ax'
au
(29.30)
where {X(i)l;=l and U are functions
oft,
x, y, and u to be determined. The second
prolongation of such a vector field is

962 29. LIE
GROUPS
AND
DIFFERENTIAL
EQUATIONS
where by Theorem 29.3.5,
U(i) =o,
(U
-
tXCk)Uk)
+
tX(k)Uik
=
DiU
- i:(DiX(k»Uko
k~l
k~l
k=1
UCijl
=
DiDj
(U
-
tXCklUk)
+
tXCklUijk
k=l
k~l
3
=
DiDjU
- L [(DiDjXCk» Uk+Uik (DjXCk» +Ujk (Di
X Ck
l)],
k~l
and we have used Equation (29.21). Using (29.21) further, the reader may show
that
U(ijl = Uij +Uk(8jkUiU +8ikUju -
xt»)
+
UlUk
(8il8jkUuu -
Xi:
l8j,
-
Xj~8il)
+
Ukl
(8il8jkUu -
xi
k)8jl
-
xjk)8il)
(29.31)
-
UkUlm
(X~kl8il8jm
+
X~ml8il8
jk +
X~ml8ik8jl)
-
UiU
jUkX~~,
where a sum over repeated indices is understood.
Applying pr(2)vto
/1, we obtain the infinitesimal criterion
U(tt) =
UCxx)
+U(yyl or
ryi
j
U(ij)
=
O.
Multiplying Equation (29.31) by
ryij
and setting the result equal to zero yields
o=
ryi
j
U(ij)
=
ryij
Uij +
Uk
(2ryikUiu -
ryi
j
xt
l
)
+
UlUk(rykl
U
uu
-
2xi:
lryil)
- 2UklXik)ryil -
2UkUlmX~m)rykl
-
UiU
jUkX~~ryij,
(29.32)
where we have used the wave equation,
rykl
ukl
=
O.
Equation (29.32) must hold
for all derivatives of
U and powers thereof (treated as independent) modulo the
wave
equation.
Therefore,
thecoefficients of such
"monomials"
must
vanish.
For
example, since all the terms involving
UkUl
m
are independent (even after substi-
tuting
uxx +U
yy
for Utt), we have to conclude that
X~m)
= 0 for all m, i.e., that
X(i) are independent of u. Setting the coefficient of
UkUl
equal to zero and noting
that
Xi:
l
=
ax~k)
lax
i
= 0 yields
U
uu
= 0
=}
U(x,
y, t, u) =
a(x,
y,
t)u
+fJ(x, y, r). (29.33)
Let us concentrate on the functions
XCi).
These are related
via
the term linear
in
Ukl.After inserting the wave equation in this term, we get
UklXik)ryil =
U12
(xf) -
X~l))
+
U13
(X~3l
-
X~l))
-
U23
(X~3)
+
X~2»)
+
U22
(X?)
-
X~2»)
+
U33
(X?)
-
X~3l).
