Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations
Подождите немного. Документ загружается.

22.4
THE
FOURIER
TRANSFORM
TECHNIQUE
633
there are no poles in the upper
half
plane (UHF). We
must
therefore introduce a
step function0(r) in the Green's function. Evaluatingthe residue, the
'"
integration
yields - 2rri
e-
k
" . (The minus signarises because
of
clockwisecontourintegration
in the LHP.) Substitnting this in Equation (22.43), using spherical coordinates in
whichthe last k-axis is along
r,
and integrating overall angles except01,we obtain
Q
roo
a
t"
G,(r,
r)
=8(t)
(2:)~
1
0
km-ldke-
k'
1
0
(sinOl)m-2eikrcosOldOl
Q
(2)m/2-l
(m
I)
1n
00
a
=O(t)
m-l..;rr
- r
-2-
k
m/
2e-
k
'J
m
/2_l (kr)dk.
(2n)ffl r 0
For the
01
integration, we used the result
quoted
in Section 22.4.1.
Using the integral formula (see [Grad 65, pp.
716
and 1058])
(22.44)
v
(!L+
V+1)
roo
I'
_ax'
fJ
r 2
(!L+V+1·.
fJ2)
10
x e
Jv(fJx)dx
= 2
v+
lct(I'+v+l)/2r(v
+I)
<I>
2 ' V +I, - 4ct '
where
<I>
is the confluenthypergeometric function, we obtain
_ 21l'(m-l)/2
(2)m/2-l
r
m/
2-
l
(m
m r
2
)
G,(r,
t) - 8(t)
(21l')m
..;rr
;:
2m/2tm/2
<I>
2'
2' - 4t .
The power-series expansion for the confluent hypergeometric function
<I>
shows
that
<I>
(o, a; z) = e': Substituting thisresultin (22.44)
and
simplifying, we finally
obtain
e-
r2/
4t
o,«.
t)
= (
/20(t).
4rrt)m
(22.45)
22.4.4 GF for the m-Dimensional WaveEquation
The difference between this example
and
the preceding
one
is that here the time
derivative is
of
second order. Thus, instead
of
Equation (22.43), we start with
(22.46)
G,(r,
r) =
I
fd
m
ke
ik
.
r
1
00
dt»
e-
iwt
(2rr)m+l
-00
",2
- k
2'
The
ci
integrationcan be done usingthe
method
of
residues. Sincethe singularities
of
the integrand,
'"
= ±k, are on the real axis, it seems reasonable to use the
principal value as the value
of
the integral. This, in turn, depends on the sign
of
t.
If
t > 0 (t < 0), we have to close the contour in the
LHP
(UHF): to avoid the
explosion
of
the exponential.
If
one
also insists on
not
including the poles inside
the conrour.' then one can show that
1
00
e-iwt
sinkt
P dto =
-rr--«t),
-00
",2
_k
2
k
3This
will
determine
howto (semi)circle
around
thepoles.
634 22. MULTIDIMENSIONAL
GREEN'S
FUNCTIONS;
APPLICATIONS
where
E(t) es
e(t)
_
e(-t)
=
{I
-1
if t > 0,
if
t <
O.
Substituting this in (22.46) and integrating over all angles asdone in the previous
examples yields
E(t)
10
00
k",/2-ll
(k ) . k
dk
G,(r,
r) = /2 /2 1
",/2-1
r sm t .
2(2".)"' r"' - 0
(22.47)
Physics
determines
the
contour
of
integration
As Problem 22.25 shows, the Green's function given by Equation (22.47)
satisfies only the homogeneous wave equation with no delta function on the RHS.
The reason for this is that the principal valne of an integral chooses a specific
contour that may not reflect the physical situation.
In
fact, the Green's function
in
(22.47) contains two pieces corresponding to the two different contours
of
integration, and it turos out that the physically interesting Green's functions are
obtained, not from the principal value, but from giving small imaginary parts to
the poles. Thus, replacing the
w integral with a contour iutegral for which the two
poles are pushed in the LHP and using the method of residues, we obtain
1
00
e-iwt
1
e-
izt
2Jr
I
up
==
dto 2 2 =
-2--2
dz
=
-e(t)sinkt.
-00
w - k c, z - k k
The integral is zero for t < 0 becausefor negative values of t, the contourmustbe
closedin the UHP, where there are no poles inside C
I.
Substitutingthis in (22.46)
and working through as before, we obtain what is called the
retarded
Green's
retarded
Green's
function:
function
If
the poles are pushed in the UHP we obtain the
advanced
Green's
function:
advanced
Green's
funclion
(ret)
e(t)
(00un/2-1 .
G,
(r,t)
= (2".)m/2
r",/2-1
fo K J
m/2_I(kr)smktdk.
e(
-t)
10
00
",/2-1
.
/2 /2
1
k J",/2_I(kr)
smkt
dk.
(2".)"' r'" 0
(22.48)
(22.49)
Unlike the elliptic and parabolicequations discussed earlier, the integralover k
is not a function but a distribution, as will becomeclearbelow. To find the retarded
and advanced Green's functions, we write the sine term in the integral in terms
of
exponentials and use the following (see [Grad 65, p. 712]):
(00
v
-ax
(2fJ)Vr(v
+
1/2)
fo X e Jv(fJx) dx =
,fii(a2
+
fJ2)v+1/2
for
Re(a)
> 1Im(fJ)
I·

(22.50)
(22.51)
(22.52)
22.4
THE
FOURIER
TRANSFORM
TECHNIQUE
635
Toensure convergence at infinity, we add a smallnegative number to the exponen-
tial and define the integral
r:
sa
10
00
kVe-(Tit+,)k
Jv(kr)
dk
=
(2r)Vr~+
1/2)
[('fit
+
.)2
+r
2r(v+l/2).
For
the GFs, we need to evaluate the (common) integral iu (22.48) and (22.49).
With v =
m/2
- 1, we have
I(V)
==
roo
k
V
Jv(kr) sinkt dk =
2,
lim
(1:
-
I;)
io
2z
E-?O
(2r)Vr(v +
1/2).
I 1
1)
=
2i,jii
,~o
[r
2
+
(-it
+<)2]v+I/2 - [r
2
+(it +
<)2]v+1/2
.
At this point, it is couvenient to discuss separately the two cases
of
m odd and
m even. Let us derive the expressiou for
odd
m (the even case is left for Problem
22.26). Defiue the integer n =(m -
1)/2
=v +!and write I(v) as
(2r)n-I/2r(n)
[1
1)
I(n) = lim - .
2i,jii
,....0 [r
2+(_it+.)2]n
[r
2
+
(it
+
<)2]n
Define u = r
2
+
(-i
t +
.)2.
Then
using the identity
1
(_1)n-1
d
n
-
I
1
un = (n -
1)1
dun 1
(;;-)
and the chain rule,
dt/du
=
(l/2r)af/ar,
we obtain
d/du
=
(l/2r)a/ar
and
1 1
(1
a
)n-I
[ 1 ]
[r
2
+
(±it
+
.)2]n
= (n -
1)1
- 2r ar r
2
+
(±it
+
.)2
.
Therefore, Equatiou (22.50) Canbe written as
I(n) =
loo
k"-1/2
Jn_
I/
2(kr)sinktdk
(2r)n-I/2r(n)
1
2i,jii
(n
1)1
(
1
a
)n-I
[[
1
1])
.
-2rar
,~o
[r
2+(-it+<)2]
- [r
2+(it+<)2]
.
The limit in (22.51) is found iu Problem 22.27. Using the result of that problem
and
I'(n)
= (n -
1)1,
we get
I(n) =
10
00
k
n-
I/2J
n_l/2(kr)sinktdk
=
,jii(2r)n-I/2
(_~!-)n-I
[~[8(t
+ r) _ 8(t _
-n].
2 2r ar r
636 22. MULTIDIMENSIONAL
GREEN'S
FUNCTIONS:
APPLICATIONS
Employing this result in (22.48)
and
(22.49) yields
Glret)(r, r) =
2.-
(__
I_~)"-1
[~(t
-
r)]
4Jl'
21fT
ar
r
(adv)
1 ( 1 a
),,-1
[~(t
+
r)]
G,
(r,t)
= -
---
---
4rr 2Jl'r ar r
m
-I
forn=
-2-'
m
-I
forn=
-2-'
(22.53)
The theta functions are not needed in (22.53) because the arguments
of
the delta
functions already
meet
the
restrictions
imposed
by the thetafunctions.
The two functions in (22.53) have an interesting physical interpretation.
Green'sfunctions are propagators
(of
signals
of
somesort),
and
Glret)
(r,
t) is capa-
ble
of
propagating signals only for positive times.
On
the otherhand,
G;arlv)
(r,
t)
can
propagate only in the negative time direction. Thus,
if
initially (t = 0) a sig-
nal
is produced (by appropriate BCs), both G;,et)(r, t)
and
G;arlV)(r, t) work to
propagate it in their respective time directions.
It
may
seem
that G;adv)(r, t) is
useless because every signal propagates forward in time. This is true, however,
Feynman
propagator
only
for classicalevents. In relativistic
quantum
field theory antiparticles are inter-
preted
mathematically as
moviug
in the negative time direction! Thus, we cannot
simply ignore G;adV)(r,
t). In fact, the correct propagator to choose in this the-
ory is a linear combination
of
G;arlv)
(r, t)
and
G;ret)(r,
t),
called
the
Feynman
propagator
(see [Wein 95, pp.
274-280]).
The foregoing example shows a subtle
difference between
Green's
functions for second-order differential operators in
one dimensionand in higherdimensions. We
saw
in
Chapter
20 thatthe former are
continuous functions in
the
interval on
which
they
are defined. Here, we see that
higher
dimensionalGreen's functions are
not
only
discontinuous, but that they are
not
even functions in the ordinary sense; they contain a delta function. Thus, in
general, Green'sfunctions in
higher
dimensions
ought
to be treated as distributions
(generalizedfunctions).
22.5 The Eigenfunction Expansion Technique
Supposethat
the
differentialoperatorLx,definedin a domainD withboundary
aD,
has discrete eigenvalues
P.n}~l
with corresponding orthonormal eigenfunctions
{um(x)}~~l'
These two sets
may
not
be in one-to-one correspondence. Assume
that
the
U
m
(xl's
satisfy
the
same
BCs
as the
Green's
function to be defined below.
Now
consider the operator Lx - A1, where A is different
from
all An'S. Then,
as in theone-dimensional case, this
operator
is
invertible,
and
we can
define
its
Green's function by (Lx- A)G,(X, y) =
~(x
- y)
where
the weight function is set
equal
to one. The completeness
of
{un(x)}~=l
implies that
00
~
(x - y) = L
Un
(x)U~
(y)
n=l
and
00
G,(x,
y) =
Lan(y)Un(x).
n=l

22.5
THE
EIGENFUNCTION
EXPANSION
TECHNIQUE
637
Substituting lbese two expansions in the differential equation for GF yields
00 00
LO.n
- A)an(y)un(x) =
LUn(X)U:(y).
n=l
n=l
The ortbononnality
oflbe
Un's gives an(y) =
u:(Y)!(A
n
-
A).Therefore,
G (
)
_
~
un(x)u:;(y)
).x'Y-L.-J
.
n=l
An
- A
(22.54)
In
particular, if zero is not an eigenvalue
of
Lx,its Green's function can be written
as
G(
) _
~
un(x)u:;(y)
X,Y-t=1
An
.
(22.55)
This is an expansion of lbe Green's function in terms of lbe eigenfunctions of Lx.
I!
is instructive to consider a formal interpretation of Equation (22.55). Recall
lbat lbe spectral decomposition lbeorem perntits us to write
f(A)
=
Li
f(Ai)Pi
for an operatorAwilb (distinct) eigenvalues Ai and projectionoperators Pi. Allow-
ingrepetitionofeigenvaluesinlbesum, we may write
f(A)
=
Ln
f(A
n)
Iu
n)
(unl,
where n counts all lbe eigenfunctions corresponding to eigenvalues. Now, let
f(A)
=
A-
t
. Then
or,
in
"matrix
element"
form,
This last expression coincides wilb lbe RHS
of
Equation (22.55).
Equations (22.54) and (22.55) demand lbat lbe
un(x) form a complete dis-
crete ortbonormal set. We encountered many examples
of
such eigenfunctions
in discussing
Sturm-Liouville systems in Chapter 19. All lbe S-L systems lbere
were,of
course,
one-dimensional.
Here
we are
generalizing
theS-L systemtom
dimensions. This is not alintitation, however,
because-for
lbe PDEs of
interest-
lbe separation of variables reduces an m-dimensional POE to m one-dimensional
ODEs.
If
lbe BCs are appropriate, lbe m ODEs will all be S-L systems. A review
of Chapter 19 will reveal lbat homogeneous BCs always lead to S-L systems.
In
fact, Theorem 19.1.1 guaranteeslbis claim. Since Green's functions mustalso sat-
isfy homogeneous BCs, expansions such as lbose
of
(22.54) and (22.55) become
possible.

for
m,n
= 1,2,
...
,
638 22. MULTIOIMENSIONAL
GREEN'S
FUNCTIONS:
APPLICATIONS
22.5.1. Example. As a
concrete
example,
let us
obtain
an
eigenfunction
expansion
of
the GFof thetwo-dimensional Laplacian, V
2
= a
2
jax
2
+a
2
ja
y
2, insidethe rectangular
region 0
:::
x::::
a,a::::
vs bwithDirichletBCs.SincetheGFvanishesattheboundary,the
eigenvalue problembecomesV
2
u =
AU
withu(O, y) =
uta,
y) =
u(x,
0) =
u(x,
b) =
O.
The
method
of
separation
of
variables
givestheorthonormal eigenfunctions"
Umn(X,
y) =
~
sin
(n;
x)
sin
(m
b
7r
y)
whose
corresponding
eigenvalues areA
mn
= _
[(n;)2
+
(m
b
1r
)2].
Inserting
theeigenfunctions andtheeigenvalues in
Equation
(22.55), we
obtain
G(
') _
G(
.'
') _
~
umn(x, y)umn(x',
y')
r, r -
x,
y, x
,y
-
m~l
A
mn
(
n1
r )
(mrr).
(nrr
,)
(mrr
,)
4
00
sin
-;;x
sin
bY
sm
-;;x
sin
bY
=- ab L
(nrr)2
(mrr)2
m,n=l
-
+-
a b
wherewe changedx tor and
y tor', Notethattheeigenvaluesareneverzero;thus,G(r, r")
is well-defined. III
In the preceding example, zero was not an eigenvalue
of
Lx. This condition
must hold when a Green's function is expanded in terms
of
eigenfunctions. In
physical applications, certain conditions (whichhave nothing to do with the
Bes)
exclude the zero eigenvalue automatically when they are applied to the Green's
function. For instance, the condition that the Green's function remain finite at the
origin is severe enough to exclude the zero eigenvalue.
22.5.2.
Example.
Let us considerthe two-dimensional Dirichlet BVP V
2
u = t, with
u = 0 on a circleof
radius
a.
If
we
consider
only the BCs andask
whether
zerois an
eigenvalue
of V
2,
the
answer
willbeyes, asthefollowing
argument
shows.
Themost
general
solution to thezero-eigenvalue
equation,
V
2u
=
O.
in polar
coordi-
natescanbe
obtained
bythe
method
of
separation
of variables:
00 00
u(p,
rp)
= A + B
lup
+ L (bnP" +
b~p-n)
cosnrp+
L(cnP"
+
c~p-n)
sinnrp.
n~l n~l
(22.56)
Invoking the
Be
gives
00 00
0=
u(a,
cp)
= A +
BIna
+
L(bna
n
+b~a-n)
cosncp+
L(cna
n
+
c~a-n)sinncp,
n=l n=l
whichholdsfor arbitrary
rp
if andonly if
A =
-Blua,
b
' b
2n
n = - n
Q
,
4The
inner
product
is
defined
asadouble
integral
overthe
rectangle.

(22.57)
22.5
THE
EIGENFUNCTION
EXPANSION
TECHNIQUE
639
Substitutingin (22.56) gives
u(p,
q;) =
Bin
m+f
(pn
- a
2
: ) (h
n
cosnq;
+ensinnq;).
n=l
p
Thus,
if
we
demand
nothing
beyond
the Bes, V
2
will havea
nontrivial
eigen-solution
corresponding
tothezero
eigenvalue,
givenby
Equation
(22.57).
Physical reality, however, demands that
u(p,
q;) be well-behaved at the origin. This
condition sets B,
b~.
and
c~
of
Equation (22.56) equal to zero. The
Bes
then make the
remainingcoefficientsin (22.56) vanish. Thus, the demand that
u(p,
q;) be well-behaved
at
p = 0
turns
the
situation
completely
around
and
ensures
thenonexistence of a zero
eigenvalue
forthe
Laplacian,
whichin tum
guarantees
theexistenceof a
GF.
III
In
many
cases the operator
Lx
as a whole is
not
amenable to a full Stnrm-
Liouville treatment,
and
as such will
not
yield orthonormal eigenvectors in terms
of
which
the
GF
can
be expanded. However, it
may
happen
that
Lx
can
be broken
up into two pieces one
of
which is an SoL operator. In such a case, the GF
can
be found as follows: Suppose that Ll
and
L2 are two
commuting
operators with
L2 an SoL operator whose eigenvalues
and
eigenfunctions are known. Since L2
commutes with LI, it
can
be regarded as a constant as far as operations with
(and
on) Ll are concerned. In particular, (Ll +L2)G = 1
can
be regarded as an operatnr
equation in LI
alone
with
L2treated as a constant.
Let
XI denote
the
subset
of
the
variables on
which
L1 acts, and
let
X2
denote
the
remainder
of
the
coordinates.
Then
we
can
write8(x-y)
= 8(Xl
-n)8(X2
-Y2).
Now
let
Gl(Xl,
n;
k) denote
the
Green's function for LI +k, where k is a constant.
Then
it is easily verified
that
(22.58)
In fact,
(LI
+L2)G(X, y) = .[(Ll +L2)Gl(Xl, YI; L2)]8(X2 - Y2).
=O(Xt-YI) by
definition
of Gl
Once GI is found as a function
of
L2, it
can
operate on 8(X2 - Y2) to yield the
desired
Green's
function.
The
following example illustrates the technique.
22.5.3. Example. Let us evaluatethe Dirichlet GF for the two-dimensionalHelmholtz
operator
V
2
- k
1
in the
infinite
strip0
:::::
x
:::::
a,
-(Xl
< Y <
00.
LetLl = a
2/a
y
2 - k
2
and
L2
= a
2
lax
2
•
Then,
G(r, r
')
'"
G(x,
x',
y, y') = Gl (Y, y';
L2)~(X
- x'},
where (d
2
Idyl
~
/-,,2jGl
=
~(y
-
y'),
1'2
'"
k
2
- L2,and Gl (y =
-00)
= Gl (y =
00) =
O.
The GF Gl can be readilyfound (see Problem 20.12):
e-~IY-Y'I
2/k
2
~
L2
.
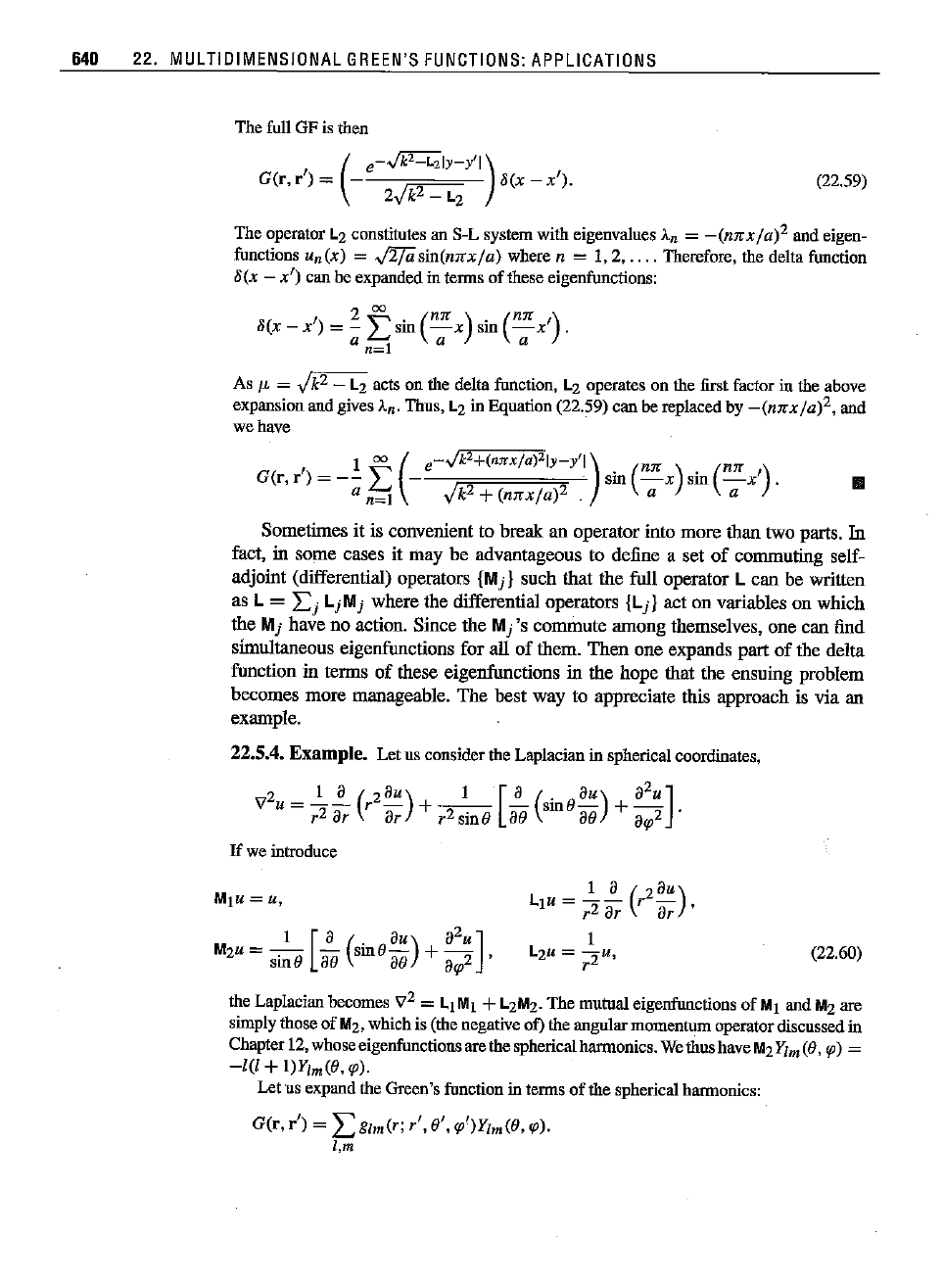
(22.59)
II
640 22. MULTIDIMENSIONAL
GREEN'S
FUNCTIONS:
APPLICATIONS
Thefull GFis then
(
-Jk
2-
L2IY-Y'I)
,e
r
G(r,r)=
~
~(x-x).
2yk
2
- L2
The
operator
Lzconstitutes an S-L systemwitheigenvalues
An
=
-(nrrx/a)2
andeigen-
functions
Un
(x) = ,fITa
sin(m,.
x/a)
where
n = I, 2,
....
Therefore, thedelta
function
8(x - x') canbe
expanded
in
terms
of
these
eigenfunctions:
'(
, 2
~
.
(mr
) .
(mr
')
ax-x)=-L-sm-xsm-x.
a
n=l
a a
As
JL
=
Jk
2
-
Lz
actson the
delta
function,
Lz
operates
onthe
first
factor
in the
above
expaosion
aodgivesAn.Thns,L2in
Equation
(22.59) caohereplaced by
-(nnx/a)2,
aod
we have
, I
~
( e-Jk2+(m,x/aj2IY-Y'I) .
(nn
) .
(nn
')
G(r,r)=--
L-
- sm
-x
sm
-x
.
a
n=1
Jk
2
+
(nnx/a)2
. a a
Sometimes it is convenient to break an operator into more than two parts. In
fact, in some cases it may be advantageous to define a set
of
commuting self-
adjoint (differential) operators
{Mj} such that the full operator L can be written
as
L =
Lj
LjMj where the differential operators {Lj} act on variables on which
the
Mj have no action. Since the Mj 's commute among themselves, one can find
simultaneous eigenfunctions for all of them. Then one expands part
of
the delta
function in
terms of these eigenfunctions in the hope that the ensuing problem
becomes more manageable. The best way to appreciate this approach is via an
example.
22.5.4. Example. Letus
consider
the
Laplacian
in
spherical
coordinates,
2
la(2aU)
I
[a(.
au) a
2u]
'V
u=--
r -
+---
-
sm8-
+-
.
r
2
ar ar
r
2
sine
ae ae
a<p2
If
we
introduce
MtU
=
U,
LtU =
~~
(r
2aU
) ,
r
2
aT
ar
I
Lzu = "2u,
r
(22.60)
the
Laplacian
becomes
V
2
= L1
Ml
+
LzMz-
The
mutual
eigenfunctions of Ml
and
Mz
are
simply
thoseof
Ml'
whichis (the
negative
of)the
angular
momentum
operator
discussed in
Chapter
12,whoseeigenfunctions arethe
spherical
harmonics,We
thus
have
M2
YZ
m
(8,
cp)
=
-1(1 +
l)Ylm(e,
<p).
Letus
expand
the
Green's
function
in
terms
of the
spherical
harmonics:
G(r, r') = L
8Im(r;
r',e',
<p')Ylm(e,
<p).
I,m

(22.61)
22.6 PROBLEMS
641
Wealsowritethedelta
function
as
8( _ ') = 8(r -
r')8(0
- O')8(rp- rp') = 8(r -
r')
"y:
(0
)Y'
(0' ')
r r . t2 . ()' t2
L-
1m
,cp
1m
,cp,
r sm r I,m
where
we haveusedthe
completeness
of the
spherical
harmonics.
Substituting
all of the
abovein '1
2
0 (r,
r')
=
8(r
-
r'),
we obtain '
'1
2
0 (r,
r')
= (L)M) +
L2M2)
L gIm(r;
r',
0',
rp')Ylm(O,
rp)
I,m
=
L{[(LI
-1(1
+ I)L2]gIm(r;
r',
0',
rp'»)Ylm(O,
rp)
t.m
8(r -
r'»"
• , ,
= 12
L.
Ylm(O ,rp )Ylm(O,
rp).
r
t.m
The orthogonality of the Ylm(0,
rp)
yields
, " 8(r - r') * , ,
[(LI
-1(1
+I)L2]glm(r; r
,0,
rp) = 2 Ylm(O, rp).
r'
This shows thai the angular part of glm is simply Y
I';,,(8',
rp'). Separaling this from the
dependence
onr andr' and
substituting
forLl and
L2,
we
obtain
~~
(r2dglm)
_ 1(1+ I) glm = 8(r -
r'),
,2 dr
dr,2
,2
where
thislastgim is a
function
of r andr' only..The
techniques
of
Chapter
20 canbe
employed10solve Equation (22.61) (see Problem 22.29).
III
The separationofthe full operatorinto two "smaller"operators can also be used
for cases in which both operators have eigenvalues and eigenvectors. The result
of such an approach will, of course, be equivalent to the eigeufunction-expansion
approach. However, there will be an arbitrariness in the operator approach; Which
operator are we to choose as our LI? While in Example 22.5.3 the choice was
clear(the operatorthat had no eigenfunctions), here eitheroperator
can
be chosen
as
LI. The eusuing GFs will be 'equivalent,
and
the series representing them will
be convergent,
of
course. However, the rate
of
couvergence may be different for
the two.
It
turns out, for example, that if we are interested in G(x, y;
x',
y') for
the two-dimensioual Laplacian at points
(x, y) whose y-coordiuates are far from
s'.
then the appropriate expansion is obtained by letting LI = a
2
jay2, that is, an
expansionin terms
of
x eigenfunctions. Onthe otherhand,
if
the Green'sfrmctiou is
to
be calculated for apoiut (x, y) whose x-coordinateis far away from the singular
point
(x', y'), then the appropriate expansionis obtained by letting
L)
= a
2
jax
2
•
22.6 Problems
22.1. Find the GF for the Ditichlet BVP iu two dimensious if D is the UHP and
aD
is the x-axis.

642 22. MULTIDIMENSIONAL
GREEN'S
FUNCTIONS:
APPLICATIONS
22.2. Add
f(r")
to
H(r,
r")
in Example 22.1.2 and retrace the argument given
there to show that
f(r")
=
O.
22.3. Use the methnd
of
images to find the GF for the Laplacian in the exterior
region
of a
"sphere"
of
radius
a in twoand
three
dimensions.
22.4. Derive Equation (22.7) from Equation (22.6).
22.5. Using Equation (22.7) with p = 0, show that
if
g(e',
rp') =
Vo,
the potential
at any point inside the sphere is
Vo.
22.6. Find the BC that the GF must satisfy in order for the solution u to be rep-
resentable in terms of the GF when the BC on u is mixed, as in Equation (22.10).
Assume a self-adjoint SOLPDO
of
the elliptic type, and consider the two cases
a(x)
01
0 and f3(x)
01
0 for x E aD. Hint:
In
each case, divide the mixed BC
equation by the nonzero coefficient, substitute the result in the Green's identity,
and set the coefficient of the
u term in the aD integral equal to zero.
22.7. Show that the diffusion operator satisfies
Lx"G(x,
y; t -
'J
=
8(x
-
y)8(t
-
c).
Hint: Use
ae
-(t
-
r)
=
8(t
-
c).
at
22.8. Show that for m = 3 the expression for
G,(r)
given by Equation (22.36)
reduces to
G,(r)
=
-e-p.r
1(4rr:r).
22.9. The time-independent Schrodinger equation can be rewritten as
where
k
2
= 2/1o£/fj,2and
/10
is the mass of the particle.
(a) Use techniques of Section 21.4 to write an integral equation for
1jI.
(b) Show that the Neumann series solution
of
the integral equation converges ouly
if
(c) Assume that the potential is of Yukawa type:
V(r)
= g2
e
- "
[r
. Find a con-
dition between the (bound state) energy and the potential strength g that ensures
convergence
of the
Neumann
series.
22.10. Derive Equation (22.29).
