Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations
Подождите немного. Документ загружается.


22 _
Multidimensional Green's Functions:
Applications
Theprevious chaptergathered togethersome generalproperties
of
the GFs andtheir
companion, the Dirac delta function. This chapter considers the
Green's
functions
for elliptic, parabolic, and hyperbolic equations that satisfy the BCs appropriate
for each type of POE.
22.1 Elliptic Equations
The most general linear
POE
in m variables of the elliptic type was discussed in
Section 21.1.2. We will not discuss this general case, because all elliptic PDQs
encountered
in mathematical physics are
of
a much simpler nature. In fact, the
self-adjoint elliptic
PD~
of the form
Lx
=
'1,72
+
q(x)
is sufficiently general for
purposes of this discussion. Recall from Section 21.1.2 that the BCs associated
with an elliptic POE are
of
two types, Dirichlet and Neumann.
Let
us consider
these separately.
22.1.1 The Dirichlet Boundary Value Problem
A Dirichlet BVP consists
of
an elliptic POE together with a Dirichlet BC, such as
Lx[u] =
'I,72
u
+
q(x)u
=
f(x)
for
xED,
U(Xb) = g(Xb) for Xb E aD,
(22.1)
where g(Xb) is a given function defined on theclosed hypersurface aD.
The Green's function for the DirichletBVP mustsatisfy the homogeneous BC,
for the same reason as in the one-dimensional Green'sfunction. Thus, the Dirichlet

614 22. MULTIDIMENSIONAL
GREEN'S
FUNCTIONS:
APPLICATIONS
Green's function, denoted by
Gn(x,
y), must satisfy
Lx[Gn(x,
y)]
= 8(x - y),
for Xb E S.
(22.2)
As discussed in Section 21.3.2, we can separate
Gn
into a singular part Gg)and
a regular part
H where G
g)
satisfies the same DE as Gn and H satisfies the
corresponding homogeneous DE and the
Be
H(Xb,
y) =
-Gg)
(Xb, y).
Using Equation(22.1) and the properties
of
G
n(x,
y) in Equation (21.27), we
obtain
u(X) = (
d"'yGn(x,
y)f(y)
+ ( g(Yb)
aa
Gn
(x, Yb)da,
JD
laD
n
y
where a
jan
y
indicatesnormaldifferentiation with respectto the secondargument.
GustavPeter LejeuneDirichlet
(1805-1859), thesonofapost-
master,
firstattendedpublicschool,thenaprivateschoolthatem-
phasized Latin. He was precociously interested in mathematics;
it is said that before the age
of
twelve he used his pocket money
to buy mathematical books.
In
1817 he entered the gymnasium
in Bonn. He is reported to have been an unusually attentive and
well-behaved pupil who was particularly interested
in
modem
historyas wellas in mathematics.
After two years in Bonn, Dirichlet was sent to a Jesuit col-
lege
in Cologne that his parents preferred. Among his teachers
was the physicist Georg Simon Ohm, who gave
him
a thorough
grounding in theoretical physics. Dirichlet completed his
Abitur examination at the very
early age
of
sixteen. His parents wanted
him
to study law, but mathematics was already
his chosen field.
At
the time the level
of
pure mathematics in the German universities was
at a low ebb: Except for the formidable Carl Gauss, in G6ttingen, there were no outstand-
ing mathematicians, while in Paris the firmament was studded by such luminaries asP.-S.
Laplace, AdrienLegendre, Joseph Fourier,and Simeon Poisson.
Dirichletarrived in Paris in May 1822.
In
the
summer
of
1823 he was fortunate in being
appointed to a well-paid and pleasantposition as tutorto the children
of
General
Maximilien
Fay,a national hero
of
the Napoleonic wars and then the liberal leader
of
the opposition in
the Chamber
of
Deputies. Dirichletwas treated as a
member
of
the family and met many
of
the
most
prominent figures in French intellectual life. Among the mathematicians, he was
particularly attracted
to Fourier, whose ideas
had
a strong influence upon his laterworks on
trigonometric series and mathematical physics.
General Fay died in November 1825, and the next
year
Dirichlet decided to return to
Germany, a plan strongly supported by Alexandervon Humboldt,who was working for the
strengthening of the natural sciences in Germany. Dirichlet was permitted to qualify for
habilitation as Privatdozent at the University
of
Breslau; since he did not have the required
doctorate, this was awarded honoris
causa
by the University of Cologne. His habilitation
thesis dealt with polynomials whose prime divisors belong to special arithmetic series. A
second paper from this period was inspired by
Gauss's
announcements on the biquadratic
law
of
reciprocity.

22.1 ELLIPTIC EQUATIONS 615
Dirichletwas appointedextraordinaryprofessor in Breslau,but the conditionsforsci-
entific work were
not
inspiring.
In
1828 he moved to Berlin, again with the assistance
of
Humboldt.tobecomeateacherofmathematicsat themilitary
academy.
Shortlyafterward,
at the age
of
twenty-three, he was appointed extraordinary (later ordinary) professor at the
Universityof Berlin.In 1831he became a member of the Berlin Academy of Sciences,and
in the same year he married Rebecca Mendelssohn-Bartholdy, sister
of
Felix
Mendelssohn,
the composer.
Dirichletspenttwenty-sevenyearsasaprofessorin Berlinandexertedastronginfluence
on the developmentof German mathematics through his lectures, through hismany pupils,
and through a series of scientific papers
of
the highest quality that he published during
this period. He was an excellent teacher, always expressing himselfwith great clarity. His
manner was modest; in his later years he was shy and at times reserved. He seldom spoke
at meetings and was reluctant to make public appearances.
In
many
ways he was a direct
contrast to his lifelong friend, the mathematician
Karl
Gustav Jacobi.
One of Dirichlet's most importantpapers,publishedin 1850,deals with the boundary
value problem, now known as Dirichlet's boundary value problem, in which one wishes to
determine a potential function satisfying Laplace's equation and having prescribed values
on a given surface, in Dirichlet's case a sphere.
In 1855, when Gauss died, the University
of
GOttingen was anxious to
seek
a successor
of
great distinction, and the choice
fell
upon Dirichlet. Dirichlet moved to Gottingen in
the fall
of
1855, bought a house with a garden,
and
seemed to enjoy the quieter life
of
a
prominent university in a small city. He had a
number
of
excellent pupils and relished the
increased leisure for research. His
work
in this periodwas centered on general problems
of
mechanics. This new life, however, was not to
last
long. In the summer
of
1858 Dirichlet
traveled to a meeting in Montreux, Switzerland, to deliver a memorial speech
in honor
of
Gauss. While there, he suffered a heart attack and was barely able to return to his family
in Giittingen. Duringhis illness his wife died of a stroke, and Dirichlet himself died the
followingspring.
Some
specialcases
of
(22.2) are worthy
of
mention.
The
first is U(Xb) = 0, the
solntion to an inhomogeneous
DE
satisfying the homogeneous BC. We obtain this
by substituting zerofor
g(Xb) in (22.2) so thatonly the integrationoverD remains.
The second special case is when the DE is homogeneous, that is,
when
f(x)
= °
but the BC is inhomogeneous. This yields an integration over the bonndary
aD
alone. Finally, the solution to the homogeneous
DE
with the homogeneous
BC
is
simply
U = 0, referred to as the zero solution. This is consistent with physical
intuition:
If
the function is zero on the boundary
and
there is no source f (x) to
produce any "disturbance," we expectno nontrivial solution.
22.1.1.
Example.
Let us lind the Green's functionfor the three-dimeosional Laplacian
Lx
= V
2
satisfyingthe Dirichlet
Be
GD(P, y) = 0 for P, on thexy-plane. Here D is the
upperhalf-space(z '" 0) and
aD is the xy-plane.
It is more convenient to use r = (x, y, z) and
r'
=
(x',
s',
z') instead
of
x and y,
respectively. Using(21.21)as Gg),we canwrite
, I ,
GD(r,r)=-4
I 'I
+H(r,r)
rrr-r
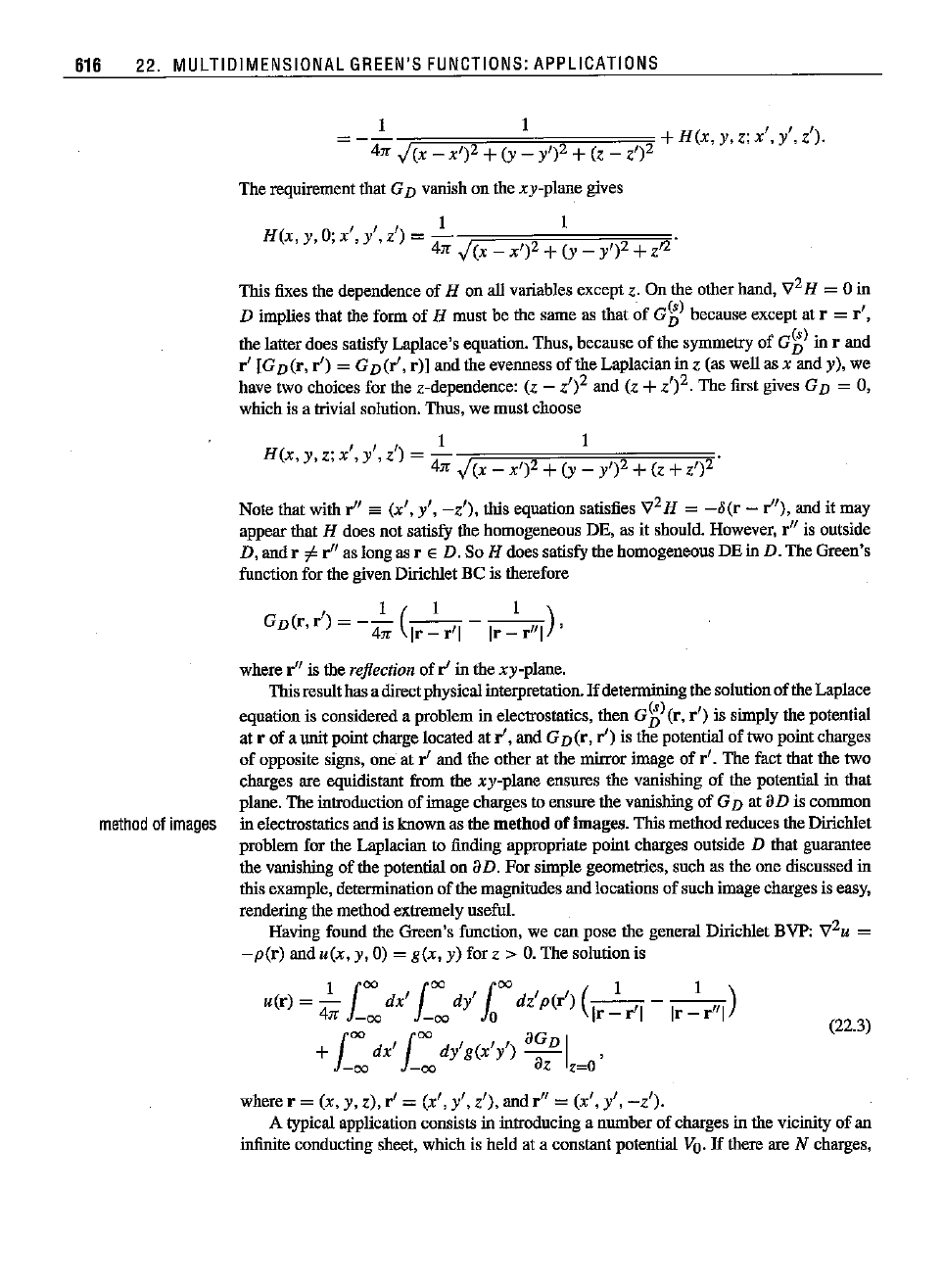
616
22. MULTIDIMENSIDNAL
GREEN'S
FUNCTIDNS:
APPLICATIDNS
I I
- -
411"
)(x
_
x')2
+(y - yl)2 +(z
The requirement that GD vanish on the xy-plane gives
2
+
H(x,
y,z:
x',
v',Zl).
Z')
(22.3)
, " 1 1
H(x,y,O;x
,y
,z)
=
411"
)(x
_ x
')2
+ (y _ yl)2 +
zrJ.
This fixes the dependence
of
H on all variables except z. On the other hand, V
2
H = 0 in
D implies that theform
of
H must be the same as that
of
Gg)
because except at r = t',
the latter does satisfy Laplace's equation. Thus, because
of
the symmetry
of
Gg)
in r and
r'
[GD(r,
r')
=
GD(r',
r)] and the evenness
of
the Laplacianin z (as well as x and y), we
have two choices for the z-dependence: (z -
z')2
and (z +z')2. The first gives GD = 0,
which is a trivial solution. Thus, we must choose
I " 1
--;===;,,;;==;,=I~7"F=:===;'7i
H(x,y,z;x
,y
,z)
=-
411"
.,j(x _ x
l)2
+(y - yl)2 +(z +z')2
Note that with
r"
ee (x', s'.
-z'),
this equation satisfies \72H =
-li(r
-
r"),
and it may
appear that
H does not satisfy the homogeneous DE, as it should. However,
r"
is outside
D,
andr
i=
r" as long as
rED.
So H does satisfy the homogeneous
DE
in D. The Green's
function for the given Dirichlet
Beis therefore
,1(1
I)
GD(r,r)=-411"
Ir-r'I-lr-r"l
'
where
r"
is the reflection of r' in the xy-plane.
This result hasa direct physicalinterpretation.
If
determiningthe solutionof theLaplace
equation is considered a problem in electrostatics, then
Gg>(r,
r')
is simply the potential
at r of a unitpoint charge located at
r', and
GD(r,
r')
is the potential of two point charges
of opposite signs, one at
r'
and the other at the mirror image
of
r', The fact that the two
charges are equidistant
from the xy-plane ensures the vanishing of the potential in that
plane. The introduction of image charges to ensure the vanishing of GD at 8
D is common
methodof images in electrostatics and
isknown as the methodofimages. This method reduces the Dirichlet
problem for the Laplacian to finding appropriate point charges outside
D that guarantee
the vanishing of the potential on
aD.
For simple geometries, such as the one discussed in
this example, determination of the magnitudes and locations of such image charges is easy,
rendering the method extremely useful.
Having found the Green's function, we can pose the general Dirichlet BVP: V
2
u =
-per)
and
u(x,
y, 0) =
g(x,
y) for z >
O.
The solution is
u(r)
=
4~
i:
dx'
i:
dy'
rdz'
per')
(Ir
~
r'1
- Ir
~
r'11)
+
1""
dxll""
dy'
g(x'
y')
8G
D I '
-00 -00
8z
z=o
where r = (x,
y,
z),
r'
= (x', s'.z'), and r' = (x',
v',
-z').
A typical application consists in introducing a number of charges in the vicinity of an
infinite conducting sheet, which is held at a constant potential
Vo.
If
there are N charges,

22.1
ELLIPTIC
EQUATIONS
617
{qil~l'
located
at{ril~l'
then
p(r)
=
I:~I
qj8(r
-
ri),
g(x, y) = const = Va, and we
gel
N 1
(qi
qi) 1
00
'1
00
,80
D
u(r)
= -
-----
+Vo
dx
dy
--
~
4rr [r -
ri
I [r -
r~
I
-00
-00
8z
Iz=o'
(22.4)
III
where ri =(Xi, Yi, Zi) and
~
= (Xi, Yi,
-Zi)·
That
the double integral in Equation (22.4)
is unity can be seen
by direct integration or by noting that the sum vanishes
when
z = o.
On the other hand,
u(x,
y, 0) =
Vo-
Thus, the solution becomes
u(r)
= t
~
(_q_i
--
----'!!...-)
+Va.
i=1
4".
Ir-ril Ir-ril
22.1.2.
Example.
Themethod of imagesisalsoapplicable whentheboondary isasphere.
Inside a sphere
of
radius a with center at the origin, we wish to solve this Dirichlet BVP:
V
2
u =
-p(r,
e,
rp)
for r < a, and u(a, e,rp)=
g(e,
rp).
The GF satisfies
v
2a
otr.
e,
rp;
r',
o',
rp') =
8(r
- r')
GD(a,
e,
ip; r', «,
cp')
=
o.
for r < a,
(22.5)
Thus, GD can again be interpreted as the potential
of
point
charges,
of
which one is in the
sphere and the others are outside.
Wewrite GD =
G~)
+ H and choose H in such a way that the secondequationin
(22.5) issatisfied. As in thecase of the xj-plane,let
l
H (r,
r")
= k
,,'
where k is
4".lr-r
I
a constantto be determined.
If
r"
is outside the sphere, V
2
H will vanisheverywhere inside
the sphere. The problem has been reduced to finding k and r" (the location of the image
charge). We want to choose
r"
such that
I I k I ' "
-I
-'I
= I _ r"1
=}
k(lr - r
I)r=
= (Ir - r
I)r~a.
rrr=a
r r=a
This shows that k must be positive. Squaring both sides and expanding the result yields
kZ(a
Z
+r'Z _ 2ar'
cosy)
=a
Z
+ r"Z - 2ar" cos y,
where
y is the angle between r and r', and we have assumed that r' and
r"
are in the
same direction.
If
this equation is to hold for arbitrary
y,
we must have kZr' = r'' and
k
2(a
2
+r,2)
= a
2
+r,l2. Combining these twoeqnations yieldsk
4r
12
_k
2
(a
2+r
12)+a2
=
0, whose positive solutions are k = 1 and k = aIr. The first choice implies that r" = r',
which is impossible because r" must be outside the sphere. We thus choose k =
air',
which gives
r"
= (a
2Ir'Z)r'.
We then have
,
1[
1
ar']
GD(r,r)=--
---
.
4". [r - r'l Ir,2
r
- a
2r'I
(22.6)
1Actually, to be general, we must add an arbitrary function
!(r")
to this. However, as the readercan easilyverify, the following
argument will show
that!
(r")
=O. Besides, we are only interested in a solution, not the most general one. All simplifying
assumptions that follow are made for the same reason.

618 22. MULTIDIMENSIDNAL
GREEN'S
FUNCTIDNS:
APPLICATIDNS
Substitutingthis in Equation (222), audnoting that
aG/any
=
(aG/ar')"=a,
yields
u(r) =
~
{a
rfldr'
t"
sinO'
so'
{2"
(~r'1
- 2
ar'
2 )
p(r')
dq/
411"
Jo
10 10
[r - IT' r - a
r'l
+ a(a
2
- r
2)
r:
dq/
("
siu0' dO'
g(O',q/)
, (22.7)
4"
1
0
10
[r - al
3
where
a = (a, 0',
q/)
is a
vector
from
the
origin
to apointonthe
sphere.
Forthe
Laplace
equation
p(r')
= 0, aud only thedouble integral in Equation (22.7) will contribute.
It cau
be shown that if g(O', '1") = const = Vo, then u(r) = Vo. This is the familiar
fact
shown
in
electromagnetism:
If
the
potential
ona
sphere
is
kept
constant,
the
potential
insidethespherewillbe constantandequalto thepotentialatthe surface. II
22.1.3.
Example.
Inthisexamplewefindthe DirichletGFfor acircleofradiusacentered
at
the origin.TheGFislogatithutic [seeEquation(21.22)]. Therefore,H isalsologatithutic,
and
itsmost
general
form is
H(r,
r") =
-~
In(lr -
r"l)
-
~
in[f(r")]
=
-~
In(lr
-
r"lf(r")),
2"
2n
2"
so that
,1
,1
""
1 1
r-r'
1
G
D(r,r)=-In(lr-rl)--In(lr-r
If(r
))=-In
r" "
2" 2"
2n
(r
-
)f(r)
ForGD to
vanish
atall
points
onthe
circle,
wemusthave
1
3-r'
I " ,
(a _
r")f(r")
= I
=}
[a -
r'[
= I(r - r
)f(r'
)1,
where
a is a
vector
from
origin
toapointonthe
circle.
Assuming
that
r" andr'
are
in the
same
direction,
squaring
bothsidesof thelast
equation
and
expanding
the
result,
we
obtain
(a
2
+r
'12
-
2ar"
cosy) f
2
(r")
= a
Z
+r
t2
-
2ar'
cos
y,
where
y is theangle
between
a
and
r' (orr").Thisequation
must
hold
for
arbitrary
y.
Hence,
we have
j2(r")r"
= r'
and
j2(r")(a
2
+r,12) =a
2
+r
f2
. Thesecanbe solvedfor
j(r")
and
r",
The
result
is
r',=a
2
r,
"a
r'
r
,2 '
f(r
) = " =
-.
r a
Substituting
these
formulas
inthe
expression
forGD, we
obtain
, I , I
(I
a
2
'1
r')
G
D(r,r)=-In(lr-rl)--1n
r--r
- .
23f 23f
r
12
a
Towrite the solutionto the Dirichlet BVP,we also need
aGD/an
=
aGD/ar'.
Using
polar
coordinates,
we
express
GD as
, I I r
2
+r,2 - 2rr'
cos(t9
-
19')
I
G
D(r,r)=-1n
.
4"
r
2,12
/a
2
+ a
2
-
2rr'
cos(O -
0')
Differentiation
with
respect
tor' yields
aGDI
aGD\
I a
2_r
2
---a;-
r'=a =
a;:r
r'=a = 23fa r
2
+a
2
- 2ra
cos(t9
-
19')'
22.1 ELLIPTIC
EQUATIONS
619
from
whichwe can
immediately
write
the
solution
to the
two-dimensional
Dirichlet
BVP
V
2u
= p,
u(r
= a) =
g(e')
as
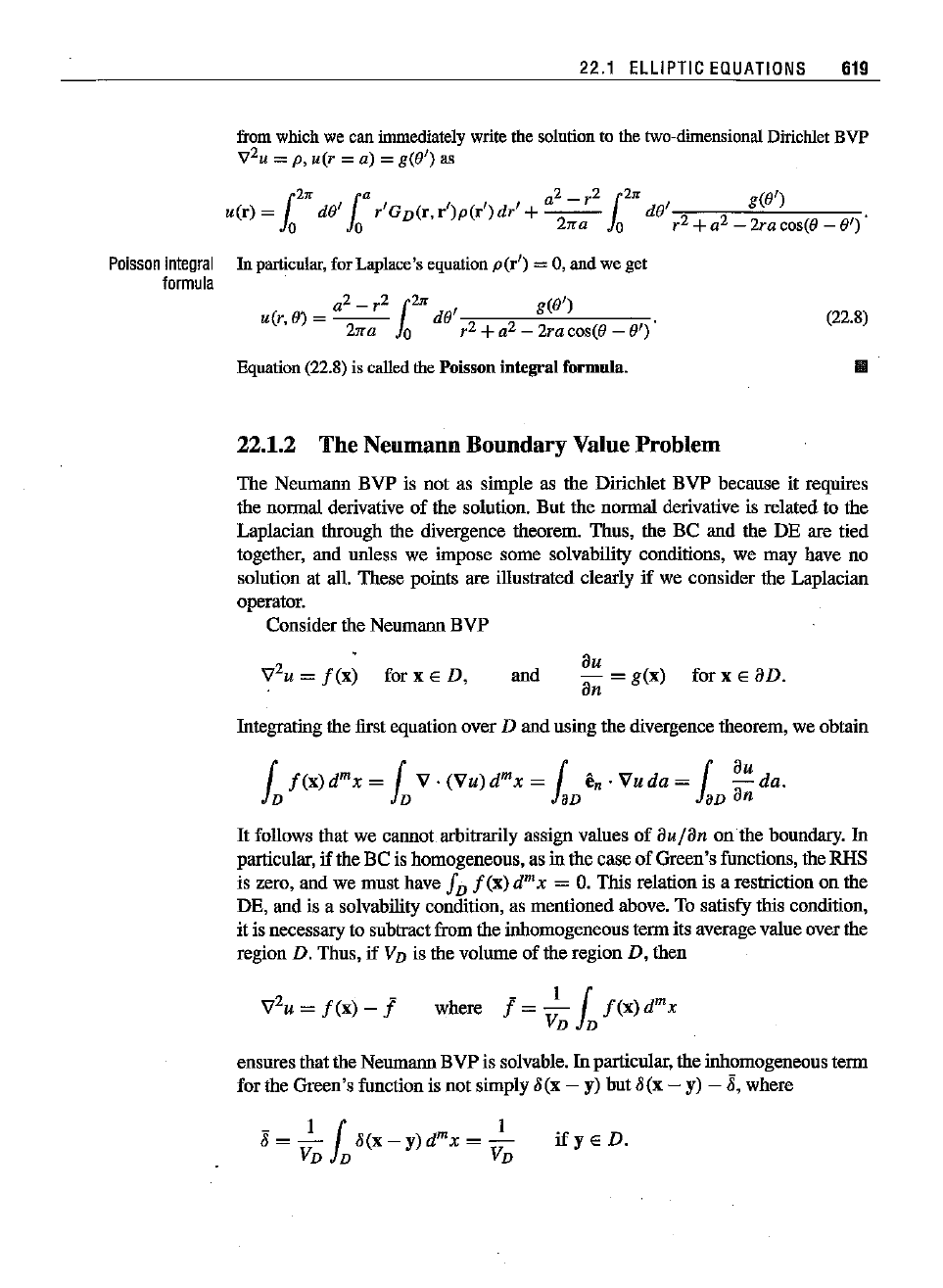
fo
2n loa a
2
- r
2
fo2n
g(e')
u(r)
=
de'
r'GD(r,r')p(r')dr'
+
-2--
de'
2 2 .
o 0
"a
0 r
+a
-2racos(e-e')
Poisson
integral
In
particular,
forLaplace's
equation
p(r')
= 0,
and
weget
formula
u(r, e) = a
2
- r
2
r:
de'
g(e')
.
2"a
10 r
2
+ a
2
- 2ra cos(e -
0')
Equation (22.8)is calledthePoissonintegral formula.
22.1.2 The Neumann Boundary Value Problem
(22.8)
11II
The Neumann BVP is not as simple as the Dirichlet BVP because it requires
the normal derivative of the solution.
But
the normal derivative is related to the
Laplacian through the divergence theorem. Thus, the BC and the DE are tied
together, and unless we impose some solvability conditions, we may bave no
solution at all. These points are illustrated clearly
if
we consider the Laplacian
operator.
Consider the Neumann BVP
v
2
u =
f(x)
for
xED,
and
au
an
=
g(x)
for x E
aD.
Integrating the first equation over D and using the divergence theorem, we obtain
It
follows that we cannot arbitrarily assign values
of
au/an
on the boundary. In
particular,
if
the BC is homogeneous, as in the case of Green's fuuctions, the RHS
is zero, and we must have
JD
f(x)
dmx =
O.
This relation is a restriction on the
DE, and is a solvability condition, as mentioned above. To satisfy this condition,
it is necessary to subtract from the inhomogeneous term its average value over the
region
D. Thus,
if
VD is the volume of the region D, then
- I J
where f = -
f(x)dmx
VD D
ensures that the Neumann BVP is solvable. In particnlar,the inhomogeneous term
for the Green's function is not simply 8(x -
y) but
8(x
- y) -
g,
where
-
11
m
I
8 = - 8(x - y) d x = -
VD D VD
if
y E D.

620 22. MULTIDIMENSIONAL
GREEN'S
FUNCTIONS:
APPLICATIONS
Thus, the Green's function for the Neumann BVP, GN(X,
y),
satisfies
I
V
2GN(X,
y) = 8(x - y) -
-,
Vn
aGN
--(x,
y) = a for x E aD.
an
Applying Green's identity, Equation (21.27), we get
u(x)
= (
dmyGN(X,
y)f(y)
- ( GN(X, y)
aau
da
+
ii,
In
laD
n
(22.9)
interior
vs
exterior
BVP
where ii =
(l/Vn)
In
u(x)
dmx
is the average value
of
u in D. Equation (22.9)
is valid only for the Laplacian operator, although a similar result can
be obtained
for a general self-adjointSOLPDO with constantcoefficients. We will not pursue
that result, however, since it is
of
little practical use.
Carl Gottfried Neumann
(1832-1925) was the son of
Franz
ErnstNeumann, aprofessorof physics andmineralogyat
Konigsberg;
his
mother,
Luise
Florentine
Hagen, was a sister-
in-law
of
the
astronomer
Bessel.
Neumann
receivedhis
primary
andsecondaryeducationin
Konigsberg,
attended
theuniversity,
andformedparticularly close friendships with the analyst F.J.
Richalot
andthegeometerL.a.Hesse.Afterpassingtheexamina-
tionfor
secondary-school
teaching,
he
obtained
his
doctorate
in
1855; in 1858 hequalifiedforiectnring inmathematics atHalle,
where
he
became
Privatdozent
and,
in 1663,
assistant
professor.
In the latteryearhe was called to Basel, and in 1865 to Tiibingen. Fromthe
autumn
of
1868 untiihis retirementin 1911 he was at the University of Leipzig.In 1864 he married
Hermine
Mathilde
Elise
Kloss;
shediedin 1875.
Neumann,
wholed a quietlife, was a successful
university
teacher
anda
productive
researcher.
More
than
two
generations
of
future
gymnasium
teachers
received
their
basic
mathematical
education
from
him.As a
researcher
hewasespecially
prominent
inthefield
of
potential
theory.
His
investigations
intoboundaryvalueproblems
resulted
in
pioneering
achievements;
in 1870he
began
to
develop
the
method
of the
arithmetical
mean
for
their
solution.
Healsocoinedthe
term
"logarithmic
potential."
Thesecond
boundary
value
prob-
lemof
potential
theory
still
bears
his
name;
a
generalization
ofitwas
later
provided
byH.
Poincare.
Neumann
wasa
member
of theBerlinAcademy andtheSocietiesofGoningen,
Munich,
and
Leipzig.
He
performed
a
valuable
service
in
founding
and
editing
the
important
German
mathematics
periodical
Mathematische Annalen.
Throughout the discussion so far we have assumed that D is bounded; thatis,
we haveconsideredpoints inside D with
Bes
on the boundary aD specified. This
is called an
interior
BVP.
In
many
physical situations we are interested in points

22.2
PARABOLIC
EQUATIONS
621
outside D. We are then dealing with an
exterior
BVP.
In
dealing with such a
problem, we
must
specify the behavior
of
the Green's function at infinity. In most
cases, the physics
of
the problemdictates such behavior. For instance, for the case
of
an exterior Dirichlet BVP, where
1
fa
aGD
u(x) =
d"'yGD(X,
y)f(y)
+
U(Yb)--(X,
Yb)
da
. D aD
any
and it is desired that u(x) --> 0 as [x] -->
00,
the vanishing
of
GD(X, y) at infinity
guarantees thatthe secondintegral vanishes, as long as
aD is a finite hypersurface.
To guaranteethe disappearance
of
the first integral, we mustdemandthat GD (x, y)
tendto zero faster than
f(y)d"'y
tends to infinity.
For
mostcases
of
physicalinter-
est, the calculation
of
the exterior Green's functions is not conceptually different
from that
of
the interior ones. However, the algebra may be more involved.
Later we will develop general methods for finding the Green's functions for
certain partial differential operators that satisfy appropriate BCs. At this point, let
us simply mention what are called mixed BCs for elliptic POEs. A general mixed
BC
is
of
the form
au
a(x)u(x)
+
fl(x)-(x)
=
y(x).
an
(22.10)
Problem 22.6 examines the conditions that the GF must satisfy in such a case.
22.2 Parabolic Equations
Elliptic partial differential equations arise in static problems, where the solution
is independent
of
time.
Of
the two major time-dependent equations, the wave
equation and the heat (or diffusion) equation.e the latter is a parabolic
POE
and
the former a hyperbolic POE. This section examines the heat equation, which is
of
the form "1
2u
= a
2au/at.
By changing t to
t/a
2
,
we
can
write the equation
as
Lx,,[u] sa
(a/at
- "1
2)u(x,
r) = 0, We wish to calculate the Green's function
associated with
Lx"
and the homogeneous BCs. Because
of
the time variable, we
must also specify the solution at
t =
O.
Thus, we consider the
BVP
Lx
,[u]
es
(~
- "1
2
)
u(x, r) = 0
, at
U(Xb,t) =0, u(x,O) =hex)
for
xED,
for
Xb
E aD,
xED.
(22.11)
To find a solution to (22.11), we can use a method that turns out to be useful
for evaluating Green's functions in
general-the
method
of
eigenfunctions. Let
2The heat equation turns into the Schrcdingerequation
if
t is changed to A t; thus, the following discussion incorporates
the Schrcdlnger equation as well.
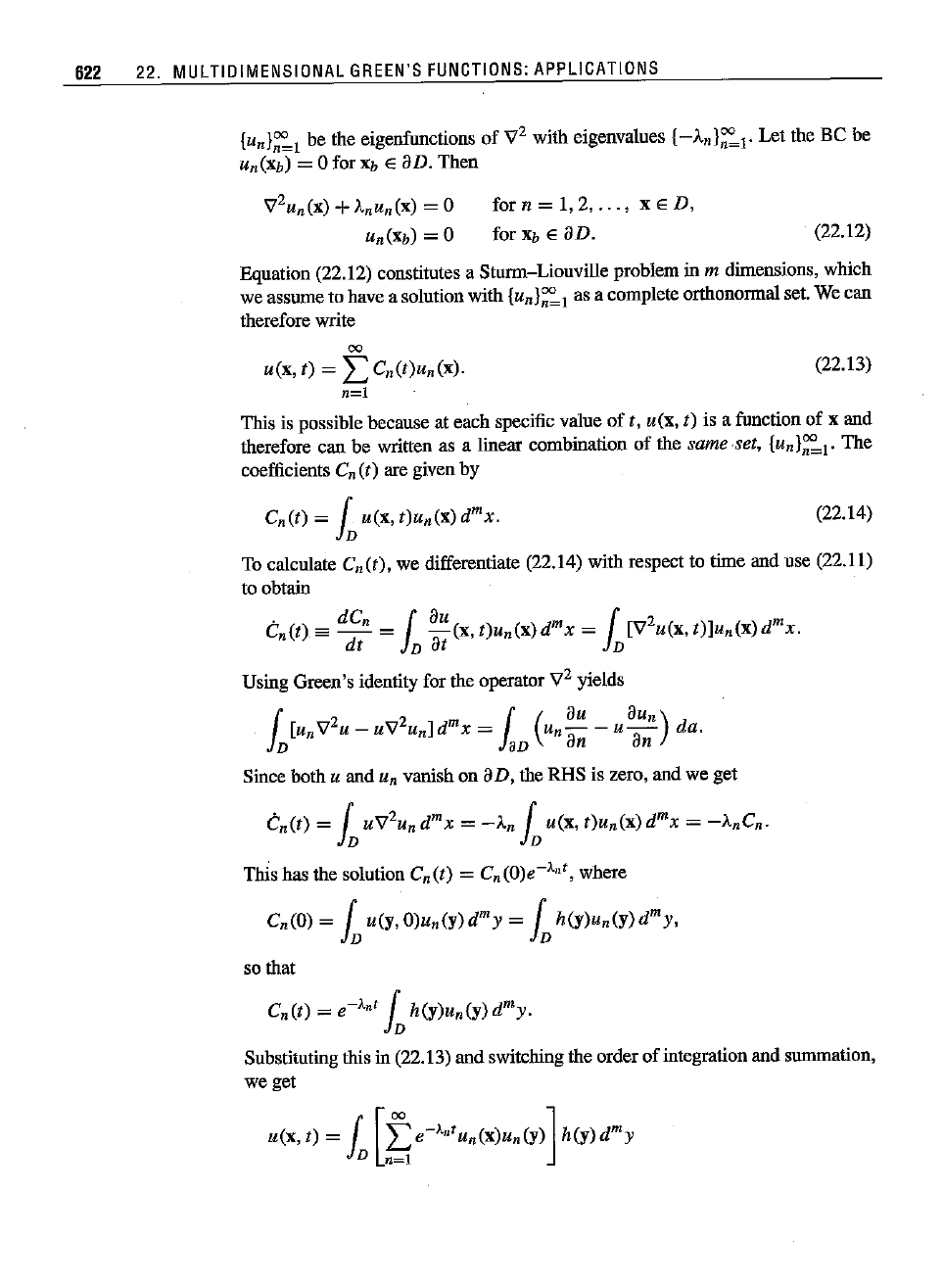
622 22. MULTIDIMENSIONAL
GREEN'S
FUNCTIONS:
APPLICATIONS
[un}~1
be the eigenfunctions of '1
2
with eigenvalues
[-An}~I'
Lei the Bebe
Un(Xb) = 0 for Xb E
an.
Then
'1
2
U
n
(X) +Anun(x) = 0
Un(Xb) = 0
for n =
1,2,
...
,
xED,
for
Xb
E
an.
(22.12)
Equation (22.12) constitutes a Sturm-Liouville problem io m dimensions, which
we assume to have a solution with
[un}~1
as a complete orthonormal set. We can
therefore write
00
u(x, I) = L
Cn(/)un(x).
n=l
(22.13)
This is possible because at each specific value of I, u(x,
I)
is a function of x and
therefore can be written as a lioear combioation of the sameset,
[un}~l'
The
coefficients C
n
(I) are given by
Cn(1) =
In
u(x, I)
Un
(x)
d"»,
(22.14)
To calculate
Cn(/),
we differentiate (22.14) with respect to time and use (22.11)
to obtaio
(;n(t)
==
ddCn
= [ au (x, I)U
n
(x)
dmx
= [ ['1
2
u(x,
I)]un(x)dmx.
I JD al JD
Usiog Green's identity for the operator '1
2
yields
[ [u
n'1
2u
_
u'1
2u
n]
dmx
= [ (Un au _ UaU
n)
da.
JD
Jan
an an
Sioce both Uand
Un
vanish on
an,
theRHS is zero, and we get
(;n(t) =
In
u'1
2u
n
dmx
=
-An
In
u(x, I)Un(X)
dmx
=
-AnC
n.
This has the solution
Cn(/)
= Cn(O)e-A"t, where
Cn(O) =
In
u(y,
O)un(y)dmy
=
In
h(y)un(y)dmy,
so that
Cn(t)
=
e-
Ant
In
h(y)un(y)
dmy.
Substitutiog this io (22.13) and switching the orderof integration and summation,
we get
u(X, I) = 1
[f:e-A"tun(X)Un(y)]
h(y)dmy
D
n=l
