Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations

Подождите немного. Документ загружается.

ignorable

coordinates

21.2 MULTIDIMENSIDNAL

GFS

AND

DELTA

FUNCTIONS

593

Thus, in the transformation from Cartesian to spherical coordinates, all spherical

coordinates (5,

tt,

rp),

with arbitrary tp, are mapped to the Cartesian coordinates

(0,0,

-5).

Similarly, the point

(0,0,0)

in the Cartesian coordinate system goes

to (0,

rp)

in the spherical system, with eand

arbitrary. A coordinate whose

value is not determined at a singular point is called an

ignorable

coordinate

that point. Thus, at the origin both

eand

are ignorable.

Among the

coordinates, let

{~i

li'=k+l

be ignorable at P with Cartesian co-

ordinates a. This means that any function, when expressed in terms

's, will

be independentof the ignorablecoordinates. A reexamination

Equation(21.10)

reveals that (see Problem 21.8)

I k

8(x

- a) =

-IJ

8(~i

ail,

k 1=1

where

= f

Jd~k+l'"

d~m'

(21.11)

particular,

the transformationis invertible, k = m and J

= J, and werecover

J8(x

- a) = 8(e -

a).

21.2.1.

Example.

In two

dimensions

the transformation betweenCartesian and polar

coordinates is given byXl

x = r eosB es gl

COs;z.

sinO

;1 sing

with

the

Jacobian

which vanishesatthe origin.The angle

f:}

is the only

ignorable

coordinate at the

origin.

Thus,k=2-1

= I, and

r: r:

J de =

r de = 21fr

8(r)

8(x)

8(x)8(y)

-2

1fr

three

dimensions, the transformation between

Cartesian

andspherical

coordinates

yields

Jacobian

J = r

sin().This

vanishes

atthe

origin

regardless

of the

values

of 8 and

tp, We

thus

havetwo

ignorable

coordinates

atthe

origin

(therefore,

k = 3 - 2 = 1),over

whichwe

integrate

to obtain

8(r)

8(x) = 41fr

III

21.2.1 Spherical Coordinates in m Dimensions

In discussing

Green's

functions

in m dimensions, a

particular

curvilinear

coor-

dinate system will prove useful. This system is the generalization of spherical

coordinates in three dimensions. The m-dimensional spherical coordinate system

594 21. MULTIDIMENSIONAL

GREEN'S

FUNCTIONS:

FORMALISM

is defined as

Xl = r sine!

Sillem-I,

X2 = r sin

(11

sine

_ 2 cos

em-I,

(21.12)

Xk = r sin

l:h

... sin{}m-k cos

(}m-k+I,

=rcos(h.

2::s:

k::s:

m - I,

(Note that for m = 3, the first two Cartesian coordinates are switched compared

to their nsual definitions.)

is not hard to show (see Example 21.2.2) that the Jacobian of the transfor-

mation

(21.12) is

m-t(.

)m-2(.

a_)m-3 (si 0

)m-k-l

. 0

::::::

r sm 1 SID V:l .

••

sm k

...

m~2

andthatthevolumeelementin termsof thesecoordinates is

dmx = J

dOl'" dOm-l =

rm-ldr

dQ.m,

element

ofthe where

m-dimensional

solid

angle

dQ.

= (sinOl)m-2(sin!Jz)m-3

...

sinOm_2dOld02'" dOm-l

is the element of the m-dimensional solid angle.

21.2.2. Example. Form =4 wehave

(21.13)

(21.14)

(21.15)

Xl = r sin

sin

sin93,

x3 =r sin

cos

Oz.

andthe

Jacobian

is givenby

X2 = r sin

sin8zcos63,

x4 =

rcos~h,

(21.16)

Itis

readily

seen(one

Can

use

mathematical

induction

prove

rigorously)

that

the

Jacobiaos

for m = 2

= r), m = 3

= r

Sinel), aod m = 4

= r

sin

el sine2)

generalize toEquation

(21.13).

III

Using the integral

Jf:

sin" 0 dO =

.Jii'r[(n

1)/2]/

r[(n

+2)/2], the total

solid angle in

m dimensions can be found to be

-:::-:---:;::-

m -

r(m/2)'

21.2 MULTIDIMENSIONAL

GFS

AND

DELTA

FUNCTIONS

595

An interesting result that is readilyobtained is an expression

the delta func-

tion in terms of spherical coordinates at the origin. Since

r = 0, Equation (21.12)

shows that

all the angles are ignorable. Thus, we have

which yields

(21.17)

21.2.2 Green's Function for the Laplacian

With the machinery developed above, we can easily obtainthe (indefinite) Green's

function for the Laplacian in

m dimensions. We will ignore questions

Bes

and

simply develop a function that satisfies '1

G(x, y) = 8(x - y). Without loss of

generality we let

y = 0; that is, we translate the axes so that y becomes the new

origin. Then we have

G(x) = 8(x).

spherical coordinates this becomes

2 8(r)

V G(x) =

Qm r

(21.18)

Since the RHS is a function of r only, we expect G to behave in the same way.

We now have to express

in terms of spherical coordinates. In general, this is

difficult; however, for a function

r = J

+...+

alone, such as

F(r),

have

aXi

a;:

aXi

a;:-;:

so that

and

For the Green's function, therefore, we get

~ (r

dG)

= 8(r).

dr dr Q

The solution, for m

::::

3, is (see Problem 21.9)

(21.19)

G(r)

f(m/2)

(I)

2(m - 2)rr

for

m::::

(21.20)

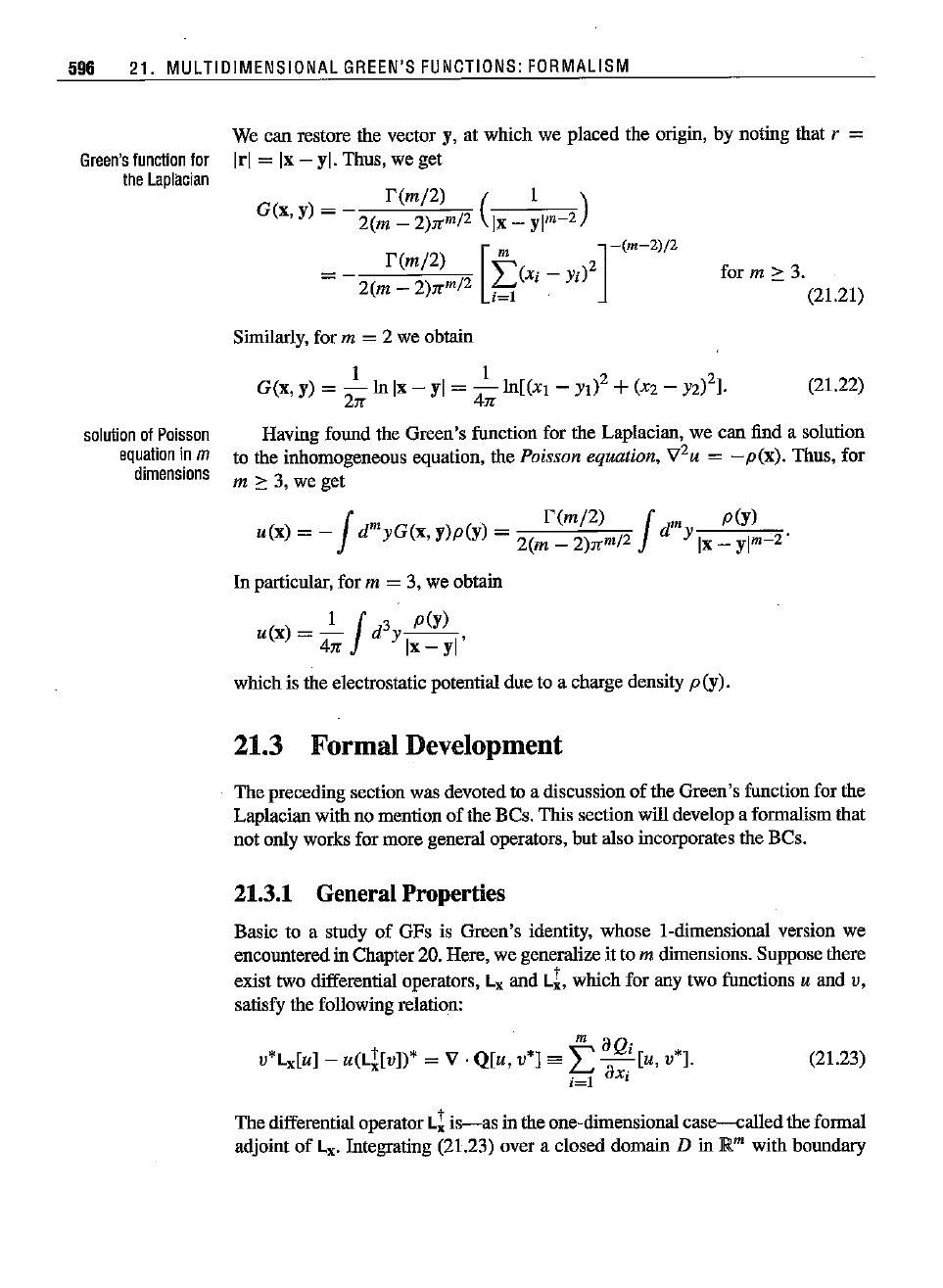
596 21. MULTIDIMENSIONAL

GREEN'S

FUNCTIONS:

FORMALISM

We can restore the vector y, at which we placed the origin, by notiog that r =

[r] = [x -

YI·

Thus, we

get

Green's

function

for

the

Laplacian

r(m/2)

( I )

G(x,

y) = /2 2

2(m - 2)Jt

[x _

ylm-

r(m/2)

[ m

2]-<m-2)/2

= - 2(m - 2)Jt

m/2

t;(Xi - Yi)

for m ::: 3.

(21.21)

(21.22)

solution

Poisson

equation

dimensions

Similarly, for m = 2 we obtain

I I 2 2

G(x, y) =

-10

[x -

-1o[(Xl

Yl)

+(X2 - Y2) ].

217:

417:

Having found the Green's function for the Laplacian, we

can

find a solution

to the inhomogeneous equation, the

Poisson equation, V

-pix).

Thus, for

3,we get

m (

r(m/2)

f m plY)

u(x)

= - d

yG(x,

y)p

y) = 2(m _ 2)Jtm/2 d Y [x _

ylm-2'

In par1icular, for m = 3, we obtaio

u(x) =

y plY) ,

417:

Ix-

whichis the electrostatic potential

due

to a charge density

ply).

21.3 FormalDevelopment

The

precediog section was devoted to a discussion

the Green's function for the

Laplacian with no mention

the BCs. This section will develop a formalism that

not

ouly works for more general operators, but also iocorporates the BCs.

21.3.1 GeneralProperties

Basic to a study

GFs is Green's identity, whose l-dimensional version we

encounteredio Chapter20. Here, we generalize it to

m dimensions. Supposethere

exist two differential operators, Lx and Lk,which for any two functions

u and v,

satisfy the following relation:

m aQ'

v*Lx[uJ -

U(L~[V])*

= V .

Q[u,

v*J sa L

[u, v*J.

i=l

aXi

(21.23)

The differential operator

is-as

io the one-dimensional

case--called

the formal

adjoint

Lx. Integrating (21.23) over a closed domaio D io R

with boundary

(21.24)

generalized

Green's

identity

21.3

FORMAL

DEVELOPMENT

597

aD,and using the divergeuce theorem, we obtain

d"'x{v*Lx[u] -

u(~[v])*}

= (

ell

da,

laD

where

is an m-dimensional unit vector normal to

aD,

and da is an etement

"area"of the m-dimensionalhypersnrface

aD.

Equation(21.24) is the generalized

Green's

identity

for m dimensions. Note that the weight function is set equal to

one for simplicity.

The differential operator

is said to be formally self-adjoint if the RHS of

Equation (21.24), the snrface term, vanishes.

such a case, we have Lx =

in one dimension. This relation is a necessary condition for the surface term to

vanish because u and v are, by assumption, arbitrary.

is called self-adjoint (or,

somewhatimprecisely, hermitian)

Lx =

and the domains of the two operators,

as determined by the vanishing of the snrface term, are identical.

can

use Equation (21.24) to study the pair of PDEs

Lx[u]

f(x)

and

L~[v]

h(x).

(21.25)

(21.26)

Green's

functions

are

symmetric

functions

their

arguments

As in one dimeusion, we let G(x, y) and g(x, y) denote the Green's functions for

Lx and

respectively. Let us assume that the

Bes

are such that the snrface term

in Equation (21.24) vanishes. Then we get Green's identity

d"'xv*Lx[u] =

d"'xu(~[v])*.

in this equation we let u =G(x, t) and v =g(x, y), where t, y

ED,

we obtain

d"'xg*(x,

y)~(x

- t) =

dmxG(x,

t)~(x

- y),

g*(t, y) = G(y, t). In particular, when Lx is formally self-adjoint, we have

G*(t, y)

= G(y, t), or

G(t,

y) = G(y, t), if all the coefficient functions of Lxare

real. That is, the Green's function will be symmetric.

we tet v = g(x, y) and use the first equation

(21.25) in (21.26), we get

u(y)

d"'xg*(x,

y)f(x),

which, using g*(t, y) =G(y, t) and interchanging

x and y, becomes u(x)

d"'yG(x,

y)f(y).

can similarty be shown that

v(x)

I»

d"'yg(x,

y)h(y).

21.3.2 Fundamental (Singular) Solutions

The inhomogeneousterm of the differentialequationto which G(x, y) is a solution

is the deltafunction,

(x- y).

wouldbe surprising

G(x, y) did not "takenotice"

this catastrophic source term and did not adapt itself to behave differently at

= y than at any other "ordinary" point. We noted the singular behavior of the

Green's function at

x = y in one dimension

when

we proved Theorem 20.3.4.

(21.27)

590 21. MULTIDIMENSIDNAL

GREEN'S

FUNCTIDNS:

FDRMALISM

There we introduced h(x,

y)-which

was discoutinuous at x =

y-as

a part of

the Green's function. Similarly, when we discussed the Green's functions for the

Laplacian in two and

m dimensions earlier in this chapter, we noted that they

behaved singularly at r

= 0 or x = y. In this section, we study similar properties

of the GFs for other differential operators.

Next to the Laplacian in difficulty is the formally self-adjoint elliptic

PD~

= V

q(x)

discussed in Problem 21.10. Substituting this operator in the

generalized Green's identity and using the expression for

Q given in Problem

21.10, we obtain

[ dmx{vLx[u] - u(Lx[v])} = [ (ve

•

Vii - ue

Vv)

da.

laD

Letting v = G(x, y) and denoting

en'

V by

a/an

gives

[ dmx[GL,.u _ uLxG] = [ [G

_ u

aG]

da.

Jan

an an

Wewanttouse this equationto findout about thebehavior

G(x,

y)as

Ix-YI

Therefore, assuming that y

ED,

we divide the domain D into two parts: one part

is aregion

DE bounded by an infinitesimal hypersphere

with radius Eand center

at y; the other is the rest of

Instead of D we use the region

sa D - DE' The

following facts are easily deduced for

D':(1) LxG(x, y) = 0 because x

yin

D';

(2) J

limHo

JD';

(3)

aD'

USE'

Suppose that we are interested in finding a solution to

Lx[U]=

q(x)]u(x)

f(x)

subject to certain, as yet unspecified, BCs. Using the three facts listed above,

Equation (21.27) yields

[ dmx[GL,.u - uLxG] = lim [

dmx[G

Lxu

-u

LxG]

E--+O

JD'

-----"-.,-'

. =1 =0

= lim [

dmxG(x,

y)f(x)

= [

dmxG(x,

y)f(x)

E--+O}DI J

= [

da+[

da.

Jan

an an

Js,

an an

We assume that the BCs are such that the integral over aD vanishes. This is

a generalization of the one-dimensional case (recall from Chapter 20 that this

is a necessary condition for the existence

Green's functions). Moreover, for

an m-dimensional sphere, da =

rm-1dfl

which for

reduces to Em-1dfl

Substituting in the preceding equation yields

[

dmxG(x,

y)f(x)

= [

au _ u

aG)

Em-1dfl

Js,

an an

21.3

FORMAL

DEVELOPMENT

599

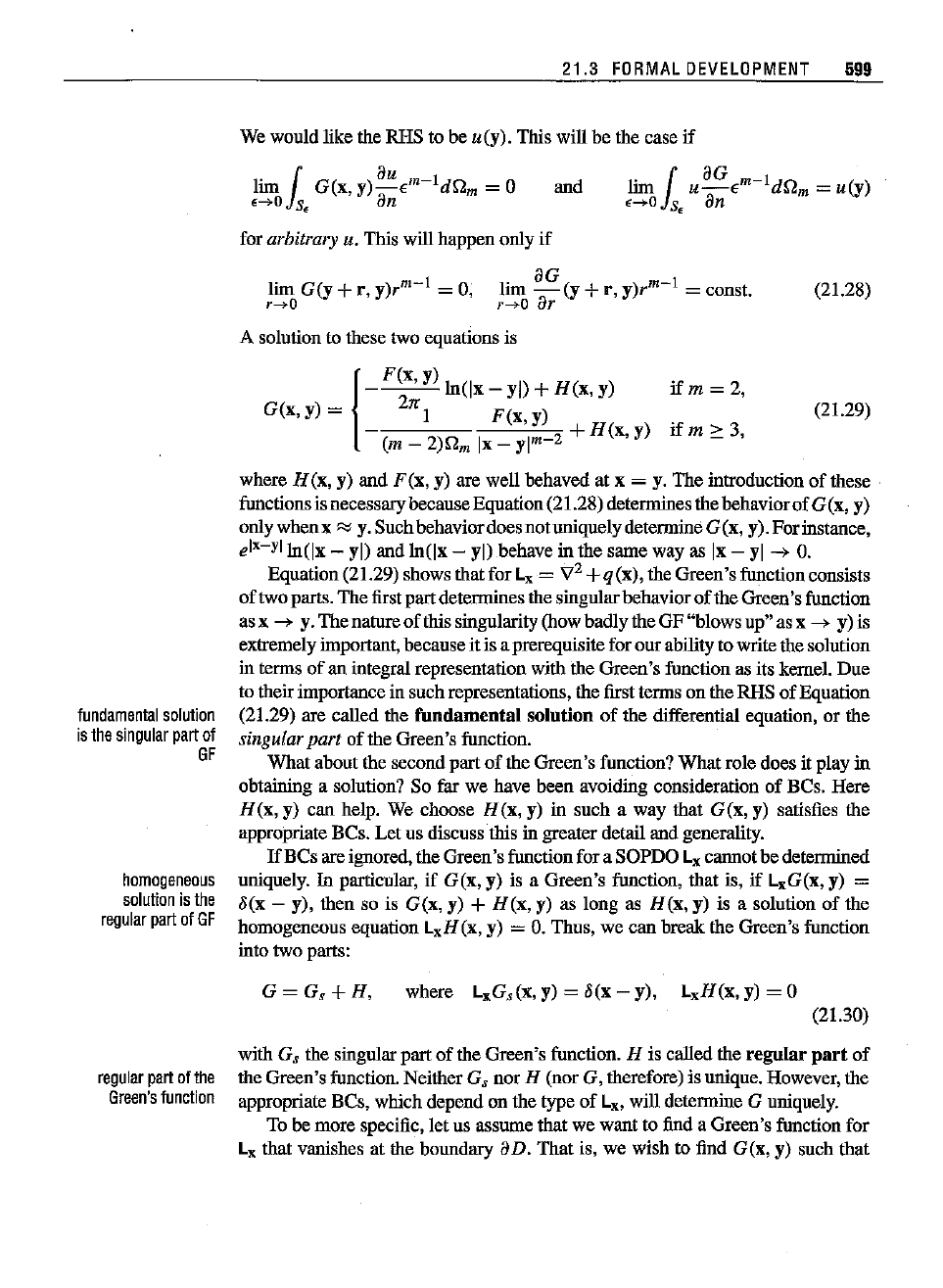
We would like the

RHS

to be u(y). This

will

be the case

and

for arbitrary u. This will

happen

only

lim

G(y

y)r

= 0,

r-+O

lim

-(y

y)r

= const.

r--+O

(21.28)

A solution tothesetwo

equations

{

F(x,

Intjx

yl)

H(x,

m = 2,

G(x,

y) =

F(x,

y) H

C"(--2"")-=n-

'1--'--',1"'

'-;.,2

+ (x, y)

m 2: 3,

m -

;:"(,m

x-y

(21.29)

lundamental

solution

isthe

singular

part

homogeneous

solution

isthe

regular

part

where

H(x,

and

F(x,

y) are well behaved at x = y.

The

introduction

these

functionsis necessarybecause

Equation

(21.28) determines

the

behavior

G(x,

only

whenx

"" y.

Such

behavior

does

not

uniquely

determine

G(x,

y).

For

instance,

elx-ylln(lx

- YI)

and

Intlx

- YI)behave in

the

same

way

as[x -

.....

Equation

(21.29) shows

that

for

Lx = V

+q(x), the

Green's

functionconsists

two

parts.

The

first

part

determines the

singular

behavior

the

Green's

function

asx

..... y.

The

natore

this singularity(how

badly

the

"blows

up"

asx

.....

y)is

extremelyimportant, becauseit is a prerequisitefor

our

ability to

write

the solution

in terms

an integral representation

with

the

Green's

function as its kernel.

Due

to their importancein

such

representations, the first terms on the

RHS

Equation

(21.29) are called the

fundamental

solution

the

differential equation, or the

singular

part

the

Green's

function.

What

about

the

second

part

the

Green's

function?

What

role

does

it play in

obtaining a solution? So far we have

been

avoiding consideration

BCs.

Here

H(x,

can

help. We

choose

H(x,

y) in

such

way

that

G(x,

y) satisfies the

appropriate BCs.

Let

us discuss this in greater detail

and

generality.

BCs

are iguored,the

Green's

function

for

SOPDO

cannot

be determined

uniquely.

In particular,

G(x,

y) is a

Green's

function,

that

is,

LxG(x, y) =

8(x

- y),

then

so is

G(x,

y) +

H(x,

y) as

long

H(x,

y) is a solution

the

homogeneous

equation

LxH(x,

y) =

Thus, we

can

break

the

Green's

function

into

two

parts:

G=G,+H,

where

LxG,(x,

y) =

8(x

- y),

LxH(x,

y) = 0

(21.30)

with

the singular

part

the

Green's

function. H is called the

regular

part

regular

part

the the

Green's

function.

Neither

nor

(nor

G, therefore) is unique. However, the

Green's

function

appropriate BCs,

which

depend

the

type

Lx,

will

determine G uniquely.

To be

specific,

let

us assume

that

want

to find a

Green's

function

for

Lx that vanishes at the boundary

aD.

That

is, we

wish

to find

G(x,

y) such

that

600 21. MULTIDIMENSIDNAL

GREEN'S

FUNCTIDNS:

FDRMALISM

G(Xb,y) = 0, where

is an arbitrary point of the boundary. All that is required

is to find a

and an H satisfying Equatiou (21.30) with the BC H(Xb, y) =

-G,(Xb,

y). The latter problem, involving a homogeneous differential equation,

can

be handled by the methods of Chapters 18 and 19. Since any discussion of

BCs is tied to the type

PDE, we have reserved the discussion of such specifics

for the next chapter.

21.4 Integral Equations

and

GFs

Integral equations are best applied in combination with Green's functions. In fact,

we can use a Green's function to tum aDE into an integral equation.

this integral

equation is compact or has a compact resolvent, then the problem lends itself to

the methods described in Chapters 16 and 17.

Let

Lx be a SOPDO in m variables. We are interested in solving the SOPDE

Lx[u] +J..V(x)u(x) =

fix)

snbject to some BCs. Here

J..

is an arbitrary constant,

and

V (x) is a well-behaved function on jRm. Transferring the second term on the

LHS to the RHS and then treating the RHS as an inhomogeneous term, we can

write

the

"solution"

tothePDEas

u(X) =

H(x)

+LdmyGo(x,

y)[f(y)

- AV(y)u(y)],

where D is the domain of Lxand Go is the Green's functiou for Lxwith some, as

yet unspecified, BCs. The functiou H is a solution to the homogeneous equation,

and it is present

to guarantee the appropriate BCs.

Combining the first term iu the integral with

H(x),

we have

u(x)

F(x)

J..

LdmyGo(x,

y)V(y)u(y).

(21.31)

Equation (21.31) is an m-dimeusioual Fredholm equation whose solution can be

obtained in the form of a Neumann series.

21.4.1. Example.

Consider

the

bound-state

Schriidinger

equation

in one

dimension:

'11

---

V(x)'II(x)

E'II(x),

2", dx

rewrite

this

equation

E<O.

(

) 2",

Lx['II]

'II(x)

1i2

V(x)'II(x),

whereK

-2",E/1i

Equation

(21.31)

givesthe

equivalent

integralequation

2",

joo

'II(x)

= 'IIo(x) +

Oo(x,

y)V(y)'II(y)dy

-00

21.4

INTEGRAL

EQUATIONS

AND

GFS

601

whereWo(x) is thesolution of

[Wol

=0,whichiseasily

found

tobeofthe

general

form

"Wo(x)

= Ae

lCx

Be-

•

we assume that

\I1o(x)

remains

finite as x

-....+

±oo,

wo(x)

will be zero.Furthermore, it canbe shownthatGo(x, y) =

-e-Klx-yI/2K

(see

Problem

20.12).

Therefore,

W(x) =

-4-

foo

e-Klx-YIY(y)W(y)dy.

-00

Now

consider

attractive

delta-functionpotential with

center

ata: V(x) = -

Vo8

(x -

a),

Vo >

Forsucha

potential,

the

integral

equation

yields

W(x) =

..!!:.-

fOO

e-Klx-YIYo8(y _

a)W(y)

dy =

'"'

e-Klx-aIW(a).

-00

Forthisequationtobeconsistent, i.e., to getanidentitywhenx = a, we musthave

,",Yo ,",Yo

,",Yo

lih

=1,*

K=1iJ

E=-21i2'

Therefore,

there

is onlyoneboundstateandoneenergylevelforan

attractive

delta-function

potential.

III

Tofinda

Neumann-series

solutionwe can

substitute

theexpression foru given

by the RHS of Equation (21.31) in the integral

that equation. The resulting

equation will have two integrals,

in the second

which u appears. Substituting

the new u in the second integral and continuing the process N times yields

N-l

(

u(x)

F(x)+

I)-A)n

dmyKn(x,

y)F(y)

n=l

(_A)N

dmyK

(x,

y)u(y),

where

K(x,

y) es Y(x)Go(x, y),

Kn(x,

dmtKn-t(x,

t)K(t,

for n 2: 2.

(21.32)

The Neumano series is obtained by lettiog

00:

u(x)

F(x)

+f(-A)n1

dmyKn(x,

y)F(y).

n=l

(21.33)

Exceptfor the fact that here the integrations are in m variables, Equation(21.33) is

the same asthe Neumanoseries derived

inSection 17.1.

exactanalogy, therefore,

we abbreviate (21.33) as

lu) = IF) +

L(-A)nK

IF).

n=l

(21.34)

602 21. MULTIDIMENSIONAL

GREEN'S

FUNCTIONS:

FORMALISM

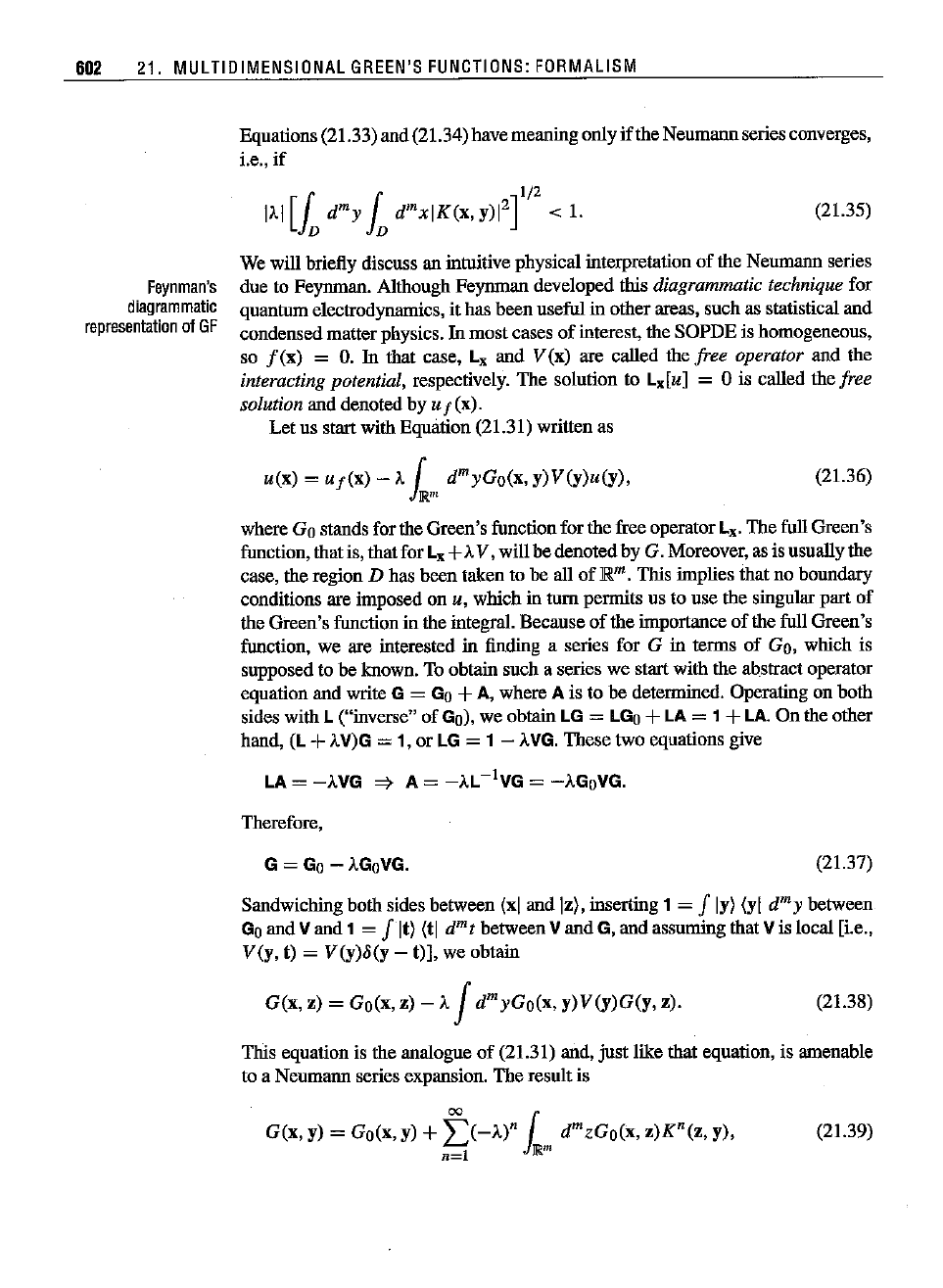
Equations (21.33) and (21.34) have meaningonly

the Neumannseries converges,

i.e.,if

(21.35)

Feynman's

diagrammatic

representation

We will briefly discuss an intuitive physical interpretation

the Neumann series

due to Feymuan. Although Feymuan developed this

diagrammatic technique for

quantum electrodynamics, it has been useful in other areas, such as statistical and

condensed matter physics.

In mostcases

interest, the SOPDE is homogeneous,

f(x)

In that case, .... and

V(x)

are called the free operator and the

interacting potential, respectively. The solution to Lx[u] = 0 is called the free

solution

and denoted by u

f(x).

Let

us start with Equation (21.31) written as

u(x) =

uf(x)

- A [ dmyGo(x, y)V(y)u(y),

JJRIll

(21.36)

where Go stands for the Green's function for the free operator Lx.The full Green's

function, that is, that for

Lx+AV, will be denotedby G. Moreover, as is usually the

case, the region

D has been taken to be all

Em. This implies that no boundary

conditions are imposed on

u, which in

turn

permits us to use the singular part of

the Green's function in the integral. Because of the importance of the full Green's

function, we are interested in finding a series for G in terms of Go, which is

supposed to be known. To obtain such a series we start with the abstract operator

equation and write

G = Go +A, where A is to be determined. Operating on both

sides with

L("inverse" of Go), we obtain LG =

LGo

+LA =1 +

LA.

On the other

hand, (L+

AV)G

= 1, or LG = 1 -

AVG.

These two equations give

LA = -AVG

A =

-AL

-IVG

= -AGoVG.

Therefore,

G = Go -

AGoVG.

(21.37)

Sandwiching both sides between (x] and [z), inserting 1

fly)

(YI

dmy between

Goand Vand 1 =

fit)

(tldmt between Vand G, and assuming that Vis local [i.e.,

V(y,

t) = V(y)8(y -

t)],

we obtain

G(x,z)

Go(x,z)

-A

dmyGo(x,y)V(Y)G(y,z).

(21.38)

This equation is the analogue

(21.31) and, just like that equation, is amenable

to a

Neumann

series

expansion.

Theresultis

G(x, y) = Go(x, y) +I)-A)n

dmzGo(x,

z)Kn(z,

y),

n=l

JRIll

(21.39)