Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations

Подождите немного. Документ загружается.

22.2

PARABOLIC

EQUATIONS

623

and read

off

the GF as

L~!

e-J.,IUn(x)Un(y)e(I), where we also introduced the

theta function to ensure that the solution vanishes for I <

generally, we

have

G(x, y; I -

= L e-J."CI-')Un(x)u

(y)e (I -

r).

n=l

Note the property

lim

G(x,

y; I -

"un(x)un(y)

8(x

y),

7:-+1

L.."

n=l

which is usually written as

G(x,

y; 0+) =

8(x

- y).

The reader may also check that

LX,IG(X,

y; I -

r).

8(x

- y)8(1 -

(22.15)

(22.16)

(22.17)

This is precisely

what

we expect for the

Green's

function

an operator in the

variables x and

Another property

G(x,

y; I -

is that it vanishes on aD, as

it should.

Havingfoundthe Green'sfunction and notedits properties, we are in a position

to solve the inhomogeneous analogue

Equation (22.11), in which the RHS

the first equation is f (x, I), and the zero on the

RHS

the second equation is

replaced by

g(Xf" I). Experience

with

similar but simplerproblems indicates that

to make any progress toward a solution, we

must

come

up with a form

Green's

identity involving

Lx,1

and

its adjoint.

is easy to show that

vLx,I[U]-

U~,,[V]

= al(uv) - V .

(vVu

uVv),

(22.18)

where

LIt

= -a/al - v

•

Now

consider the (m +I)-dimensional "cylinder" one

whose bases is at

I = E, where E is a small positive nmnber. This base is barely above the m-

dimensional hyperplane

The other base is at I = r - E and is a duplicate

D C IR

(see Figure 22.1).

Leta",

where",

= 0, 1,

...

, m, be the components

an (m +I)-dimensionalvector a = (ao,

ai,

...

, am). Define an inner product by

a·

b es L

a"b"

aObo

alb!

...

- amb

aObo

p,=O

and

the (m + I)-dimensional vector II by

UV,

Q =

vVu

uVv.

Then

(22.18) can be expressed as

aQ"

aQo

aQI

aQm

vLxt[u]

- uL

[v]

,,--

...

--.

X,I

L..

ax"

axl

,,~o

(22.19)

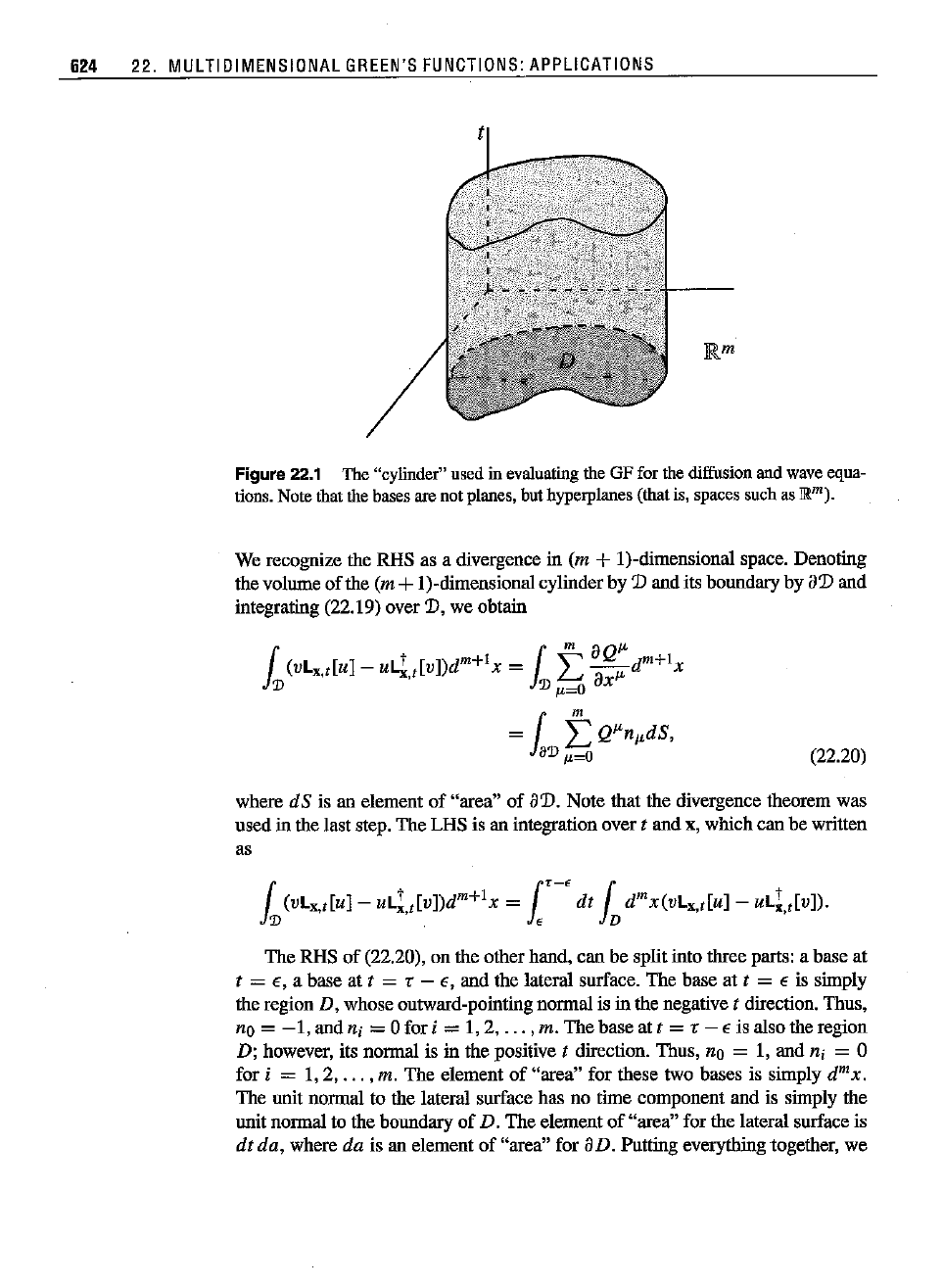
624 22. MULTIDIMENSIDNAL

GREEN'S

FUNCTIDNS:

APPLICATIDNS

Figure22.1 The

"cylinder"

usedin

evaluating

theGFforthediffusion

and

wave

equa-

tions.

Note

that

thebasesarenot

planes,

but

hyperplanes

(thatis,

spaces

suchas

JRrn).

We recognize the RHS as a divergence in (m + I)-dimensional space. Denoting

the volume of the

(m+I)-dimensional cylinder by D and its boundary by a:Dand

integrating (22.19)over D, we obtain

(22.20)

where dS is an element of "area" of a'D. Note that the divergence theorem was

used in the last step. The LHS is an integration over t and x, which can be written

The RHS

(22.20), on the other hand, can be split into three parts: a base at

t = E, a base at t = 'r - E, and the lateral surface. The base at t = E is simply

the region

D, whose outward-pointing normal is in the negative t direction. Thus,

no =

-1,

andn, = 0for i =

1,2,

...

,m. The base at t = r - E is also the region

however,

its

DOnna!

is in thepositivet

direction.

Thus,

= 1,

and

= 0

for i = 1,2,

...

,m. The element

"area"

for these two bases is simply dmx.

The unit normal to the lateral surface has no time component and is simply the

unit normal to the boundary

D. The element

"area" for the lateral surface is

dt da, where da is an element of "area" for

aD.

Putting everything together, we

(22.21)

(22.22)

(22.23)

22.2

PARABOLIC

EQUATIONS

625

can write (22.20) as

1'-'

dmx(vLx.'[u] - uLI., [v])

{(_QO)I,=,dmx+

{

QOI,~,_,dmx-

(

da1'-'dtQ.en.

In In

Jan

The minus sign for the last term is due to the definitiou

the inner product.

Substituting for

Qyields

1'-'

dmx(vLx,,[u] - uLI,,[v])

-in

u(x, f)V(X, f)dmx +

u(x, r - f)V(X, r - f)dmx

,-,

(au

av)

- da dt

aD,

an an

Let v be g(x, y; t -

r),

the GF associated with the adjoint operator. Theu

Equation (22.21) gives

1'-'

dmx[g(x, y; t -

r)f(x,

t) - u(x, t)8(x - y)8(t -

r)]

-in

u(x, f)g(X, y; f - r)dmx +

u(x, r - f)g(X, y;

-f)dmx

(

1'-'

[ au a

laD

da,

dt g(Xb,y; t -

an -

U(Xb,

We uow use the following facts:

8(t -

= 0 in the second integral on the LHS

Equation (22.22), because

t can never be equal to r in the range

integration.

2. Using the symmetry property of the Green's function and the fact that

Lx"

isreal, we have g(x, y; t -

= G(y, x; r

-t),

where we have used the fact

that t and r are the time components of x and y, respectively.

particular,

by (22.16), g(x, y;

-f)

= G(y, x;

8(x

- y).

3. Thefunctiong(x, y;

t-r)

satisfiesthe sarnehomogeneousBC as G(x, y;

r).

Thus, g(Xb,y; t -

= 0 for x, E aD.

Substituting all the above in (22.22), taking the limit f --> 0, and switching x

and y and t and

we obtain

u(x,

t) =

fa'

dmyG(x, y; t -

r)f(y,

u(Y, O)G(x, y; t)dmy

U(Yb,

r)-(x,

Yb;

t -

da,

et»

any

(22.24)

626 22. MULTIDIMENSIONAL

GREEN'S

FUNCTIONS:

APPLICATIONS

where a/any in the last integral means

normal

differentiation with respect to the

second argument

the Green's function.

Equation

(22.23) gives the complete solution to the BVP associated with a

parabolic PDE.

(Y,

= 0 and U vanishes on the hypersurface

aD,

then

Equation

(22.23) gives

u(x,

t) = Lu(y, O)G(x,

t)dmy,

evolution

operator

propagator

which is the solution to the BVP of Equation (22.11), which led to the general

Green's function of

(22.15). Equation (22.24) lends itself nicely to a physical

interpretation. The RHS can be thought of as an integral operator with kemel

G(x, y;

t).

This integral operator acts on

u(y,

0) and gives u(x,

t);

that is, given

the shape

the solution at t = 0, the integral operator prodnces the shape for

all subsequent time. That is why G(x, y;

t) is called the evolution

operator,

propagator.

22.3 Hyperbolic Equations

The hyperbolic equation we will discuss is the wave equation

Lx.t[u]

(::2 -

'1,72)

u(x, t) = 0,

(22.25)

where we have set the speed of the wave equal to unity.

We wish to calculate the Green's function for

Lx,t

subject to appropriate

Bes.

Let us proceed as we did for the parabolic equation and write

G(x, y; t) = LCn(y;

t)un(x)

n=l

Cn(y; t) = 1G(x, y;

t)un(x)

dmx,

D (22.26)

where

(x) are orthonormal eigenfunctions

'1,72

with eigenvalues

-An,

satisfy-

ing certain, as yet unspecified,

Bes.

As usual, we expect G to satisfy

)

Lx,t[Gj = at

'1,72

G(x, y; t -

=8(x -

y)8(t

r),

(22.27)

Substituting (22.26) in (22.27) with r =0 and using

'I,72

-Anu

gives

where we used

8(x

- y) =

L::'1

(x)Un(y) on the RHS. The orthonormality

now gives

tn(y;

t) +AnCn(y; t) =

un(y)8(t).

follows that Cn(y; t) is

22.3

HYPERBOLIC

EQUATIONS

627

separable. In fact,

C,,(y;

t) = u,,(y)T,,(t)

where

(:t:

An)

Tn(t) = 8(t).

This equation describes a one-dimensional Green's function and can be solved

using the methods of Chapter 20. Assuming that

Tn(t) = 0 for t :'" 0, we obtain

T,,(t) = (sinw"t/w,,)e(t), where

= An. Substituting all the above results in

(22.26), we obtain

sinwnt

G(x, y; t) =

L..,un(x)un(y)--O(t),

n=l

Of,

generally,

sinwn(t -

G(x, y; t -

= L..,un(x)un(y) O(t -

c).

n=l

liJ

We note that

(2228)

G(x, y;

0+)

= 0

and

eo I

-(x,

y; t) = 8(x -

y),

t--).O+

(22.29)

as can easily be verified.

With the Green's function for the operator

Lx,1

Equation (22.25) at our

disposal, we can attack the BVP given by

(:t

) u(x,

!(x,

r) for

xED,

U(Xb, t) =h(Xb, r), u(x,O) =

t/J(x)

for Xb E

aD,

xED,

(x, t) I =

1/r(x)

for

xED.

t t=O

(22.30)

As in the case of the parabolic equation, we first derive an appropriate expression

of Green's identity. This can be done by noting that

t a

(av

au)

vL flu] - U" [v] = -

- V .

(uVv

vVu).

-X,I

at at at

Thus,

Lx,1

is formally self-adjoint. Furthermore, we can identify

o av au

=u--v-

at at

and

uVv

vVu.

(22.31)

628 22. MULTIDIMENSIONAL

GREEN'S

FUNCTIONS:

APPLICATIONS

Following the procedure nsed for the parabolic case step by step, we can easily

derive a Green's identity and show that

u(x, t) =

fot

dt:

dmyG(x,

y; t -

7:)f(y,

7:)

(

+1D [1ft(y)G(x, y;

- </>(Y)iJt(x, Y;

t)]dmy

-r

r h(Yb, 7:)

(x, Yb; t -

7:)

da.

laD

any

The details are left as Problem 22.11.

For the homogeneons PDE with the homogeneous BC

h = 0 =

1ft,

we get

aG d

u(x, r) = -

</>(y)-(x,

Y;t) y.

Note the difference between this equation and Equation (22.24). Here the prop-

agator is the time derivative of the Green's function. There is another difference

between hyperbolic and parabolic equations. When the solution to a parabolic

equation vartishes on the boundary and is initially zero, and the

PDE

is homoge-

neous

[f(x,

t) = 0], the solutionmustbe zero. This is clear from Equation(22.23).

On the other hand, Equation (22.31) indicates that under the same circumstance,

there may be a nonzero solutionfor a hyperbolic equation if

1ft

is nonzero. 10such

acase we obtain

u(x, r) =

1ft

(y)G(x, y;

t)dmy.

This difference in the two types

equations is due to the fact that hyperbolic

equations have second-order time derivatives. Thus, the initial shape of a solution

is not enough to uniquely specify it. The initial velocity profile is also essential.

We saw examples

this in Chapter 19. The discussion of Green's functions has

so far been formal, The main purpose of the remaining sections is to bridge the

gap between founalism and concrete applications. Several powerful techniques

are used in obtainingGreen's functions, hnt we will focus only on two: the Foutier

transfoun technique, and the eigenfunction expansion technique.

22.4 The Fourier TransformTechnique

Recall that any Green's function can be written as a sum

a singo1ar part and

a regular part: G =

Since we have already discussed homogeneous

equations in detail in Chapter 19, we will not evaluate H in this section but will

concentrate on thesingular

parts

various

Green'8 functions.

The BCs play no role in evaluating

G,.

Therefore, the Foutiertransfoun tech-

nique (FTT), which involves integration over all space, can be utilized. The FTT

(22.32)

22.4

THE

FOURIER

TRANSFORM

TECHNIQUE

629

has a

drawback-it

does not work if the coefficient functions are not constants.

For

most physical applications treated in this book, however, this will not be a

shortcoming.

Let us consider the most general SOLPDO with constant coefficients,

m a m

= ao +

~:>Fa-:

+ L

bjkaT'

)=1

j,k=l

XJ Xk

where ao,

aj,

and bjk are constants. The corresponding Green's function has a

singular partthat satisfies the usual PDE with the delta function on the RHS. The

FIT

starts with assuming a Fourier integral representation in the variable xfor the

singular part

andfor the delta function:

G,(x,y)

= I /2

jdmkG,(k,y)e

(21f)m

8(x - y) =

_1_

keik.(x-y).

(21f)m

Substituting these equations in the PDE for the GF, we get

and

(22.33)

(22.34)

we can evaluate the integral in (22.33), we

can

find G.

The following examples apply Equation (22.33) to specific problems. Note

that (22.33) indicates that

depends only on x - y. This pointwas mentioned in

Chapter 20, where it was noted that such dependence occurs when the BCs play

no part in an evaluation

the singular part

the Green's function

a DE with

constant coefficients; and this is exactly the situation here.

22.4.1 GF for them-Dimensional Laplacian

We calculated the GF for the m-dirnensional Laplacian in Section 21.2.2 using a

different method. With

ao = 0 =

aj,

bjl = 8jz, and r = x - y, Equation (22.33)

reduces

I j e

.•

G,(r)

= (21f)m dmk

_k2'

where k

= kr +...+

= k . k. To integrate (22.34), we choose spherical

coordinates in the m-dirnensional k-space. Furthermore, to simplify calculations

630 22.

l TIDI

MENS

IONAl

GREEN'S

'FUNCTIONS:

APPll

CATIONS

we let the km-axis lie along r so that r =

(0,0,

...

, [r[) and k . r =

klrl

cos{h

[see Equation (21.12)]. Substitutiog this in (22.34) and writiog

dmk

in spherical

coordinates yields

From Equation (21.15) we note that

drl.

(sinet)m-2deldrl.m_l.

Thus, after

integrating over the angles

ez,

...

em-t,

Equation (22.35) becomes

G (r)

I_r1.

(00

3dk

("(sine

)m-2eiklrlcoSOlde .

s (21f)m m

t 1

The inner integral can be looked up in an integral table (see [Grad 65, p. 482]):

r (2

)m/2-t

(sinet)m-2eiklrlcosOldel

=.fii

--T-

/2- 1(kr).

Substitutiog this and (21.16) in the preceding equation and using the result (see

[Grad 65, p. 684])

we obtain

f(m/2

(_1_)

4nrn/2

m-2

for m > 2,

which agrees with (21.20) since

f(m/2)

(m/2

1)r(m/2

- I).

22.4.2 GF for the m-Dimensional Helmholtz Operator

For the Hehnholtz operator

'17

J.t2,

Equation (22.33) reduces to

Gs(r)

= - (21f)m

dmk

J.t2

Following the same procedure as in the previous subsection, we find

22.4 THE

FOURIER

TRANSFORM

TECHNIQUE

"631

Here we can use the integral formula (see [Grad 65, pp. 686 and 952])

where

to obtain

(2)m/2-1

:n:

(r)

rrr

- r

--"

1_e,mn/4H(I)

(inr)

s (2:n:)""'" r

2""

2 m/2-1 "" ,

which simplifies to

:n:/2

(fh)m/2-1

imn/4

(I)

G,(r)

= -

(2:n:)m/2

e H

('W)·

(22.36)

(22.37)

(22.38)

Non-local

potentials

depend

not

only

the

observation

point,

but

also

some

other

"non-local"

variables.

e-/l-

can be shown(see Problem22.8)that form = 3this reduces to

G,(r)

--4

:n:r

which is the Yukawa potential due to a unit charge.

We can easily obtainthe GF

for \72

+fh

by substituting ±ifh for

in Equation

(22.36). The result is

.m+!

:n:/2

(fh)m/2-1

(I)

G,(r)

/2 - H

(±W)·

(2:n:)m

r -

For m = 3 this yields

G,(r)

= _e±i/U/(4:n:r).

The

two signs in the exponent

correspondto the so-called incoming

and

outgoing "waves."

22.4.1.

Example.

Foranon-localpotential, thetime-independentSchriidingerequation

Then,

the

integral

equation

associated

withthis

differential

equation

is (see

Section

21.4)

\II(r) = Ae

ikor

---.!:!:..-

{ d

r, i

k1r

- r'1 ( d

3r"V(r',

r")lJ1(r").

2rr1i

JR'

[r -

r']

JR'

Foraseparablepotential,forwhich

V(r

r")

-g2U(r

')U(r"),

wecansolve(22.38)

exactly.

Wesubstitutefor

V(r

r")

in (22.38)10 obtain

(22.39)

632 22. MULTIOIMENSIONAL

GREEN'S

FUNCTIONS:

APPLICATIONS

(22.40)

(22.41)

andsubstituting themin(22.39) yields'I1(r)=

ik·r

+CQ(r). Multiplyingbothsidesof

thisequationby

U(r) andintegrating over

we get

C = A [

e'k.rU(r)d

+C [

U(r)Q(r)d

JR3

(2".)3/2Ail(-k)+C

[

U(r)Q(r)d

3r,

lJW.3

from wbich we obtain C = (2Jl")3/2Ail(-k)/[1 -

JJW.3

U(r)Q(r)d

3r],

leading to the

solution

3/2 -

'I1(r)_

ik.r

+ (2".)

AU(-k)

Q(r)

I-JJW.3U(r

l)Q(r

l)d

'·

ptinciple,

U(-k)

[theFouriertransform of U(r)] and Q(r) can be calculatedoncethe

functional

form of U(r) is

known.

Equations(22.40) and (22.41) givethe solutionto the

Schriidinger

equation

in closed

form.

IlII!I

Whendealing with parabolic and hyperbolic equations, we will find it conve-

nient to consider the "different" variable (usually

r) as the zeroth coordinate.

the Foutier transfonu we then use

-ko

and write

()

1 1

d !dmkG- (k ) i(k·r-wt)

s r, t = (m+l)J2 (J)

we.

(2".)

-00

8(r)8(t)

= I

dW!dmkeirJ<.r-wt)

(2".)m+t

-00

where

r is the

m-dimensional

position

vector.

22.4.3 GF for the m-Dimensional Diffusion Operator

We substitote from (22.42) in

(a/at

- V

2)G,(r,

8(r)8(t)

to obtain

1 ! .1

iwt

G,(r,

t) = +1

dmke,k.r

dco .

(21f)m

-00

W +

(22.42)

(22.43)

where as usual,

I:;:1

kr.

The

to integration can be done using the calculus

residues.

The

integrand has a simple pole at

-ik

that is, in the lower

half

the complex w-plane (LHP). To integrate, we must know the sign

t > 0, the exponential factor dictates thatthe contour be closedin the LHP, where

there is a pole and, therefore, a contribution to the residues.

On the otherhand, if

t < 0, the contour must be closed in the UHP.

The

integral is then zero because