Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations
Подождите немного. Документ загружается.


n-dimensional
complex
coordinate
space
n-dimensional
reai
coordinate
space,
or
Cartesian
n-space
linear
independence
defined
linear
combination
of
vectors
1.1
VECTOR
SPACES
21
6. Let
:Pe[t]
be the set of all polynomials with complex coefficientsin a variable t.
Then pC[t] is a vector space under the ordinary addition of polynomials and the
multiplication of a polynomial by a complex number.
In this case the zero vector is
the zeropolynomial.
7. For a given positive integer n, let
P~[t]
be the set of all polynomials with complex
coefficients of degree less than or equal to
n. Again it is easy to verify that
P~[t]
is a vector space under the usual addition of polynomials and their multiplication
by complex scalars.
In particular. the sum of two polynomials of degree less than
or equal to
n is also a polynomial of degree less than or equal to n, and multiply-
ing a polynomial with complex coefficients by a complex number gives another
polynomial of the same type. Here the zero polynomial is the zero vector.
8. The set
~[t]
of polynomials of degree less than or equal to n with real coefficients
is a vector space over the reals, but it is
not
a vector space over the complexnumbers.
9.
Let
en
consist of all complex a-tuples such as la) =
("1,
"2,
...
,
"n)
and Ib) =
(!!I,
fJ2,
...
,
fJn)·
Let"
be a complexnumber.Then we define
la) + Ib) =
("t
+ fJI, "'2 +
fJ2,""
"n +
fJn),
ala}
=
(ceq,
aa2,
...•
aa
n),
10)
=
(0,0,
...
, 0),
-Ia)
=
(-"10
-"2,···,
-"n)·
It is easy to verify that
en
is a vector space over the complex numbers. It is called
the n-dimensional complex coordinate space.
10. The set of all real n-tuples lR
n
is a vector space over the real numbers under the
operations similar to that of
en
.
It
is called the n-dimensional real coordinate space,
or Cartesian n-space.
It
is not a vector space over the complex numbers.
11. The
set
of all complex matrices
withm
rows
andn
columns
JY[mxn
is a vector space
under the usual addition of matrices and multiplication by complex numbers. The
zero vector is the
m x n matrix with all entries equal to zero.
12. Let Coo be the set of all complex sequences
la}
= {a;
}~l
such that
L~l
la;1
2
<
00.
One can show that with addition and scalar multiplication defined component-
wise, Coo is a vector space over the complex numbers.
13. The set of all complex-valued functions of a single real variable that are continuous
in the real interval
(a, b) is a vector space over the complex numbers.
14. The set
en
(a, b) on (a, b) of all real-valuedfunctions of a single real variable that
possess continuous derivatives of all orders up to n forms a vector space over the
reals.
15. The set eOO(a, b) of all real-valuedfunctions on (a, b) of a single real variablethat
possess derivatives of all orders forms a vector space over the reals. II
It
is ciearfrom the
example
above
that
the
existence
nf
a vector
space
depends
as
much
on the nature
of
the vectors as nn the nature
of
the scalars.
1.1.3.
Definition.
The vectors
lal),
la2),
...
,
Ian},
are said to be linearly inde-
pendent
iffor
"i
EC, the relation
L:f=t
a; lai) =0 implies a; =0
for
all i. The
sum
L:f=l
a; lai) is calleda linear combination
of{lai
)}f=l'

22 1.
VECTORS
AND
TRANSFORMATIONS
subspace
The
intersection
of
two
su
bspaces
is
also
a
subspace.
1.1.4. Definition. A subspace W
of
a vector space V is a nonempty subset
of
V
with the property that iJla} ,
Ib)
E W,then
ala}
+
fJ
Ib)also belongs to Wfor all
a,
fJ
E C.
A subspaceis a vector space in its own right. The reader may verify that
the
intersection
of
two subspaces is also a subspace.
span
ofa
subset
ofa
vector
space
1.1.5. Theorem.
If
S is any nonempty set
of
vectors in a vector space V,then the
set
Ws
of
all linear combinations
of
vectors in S is a subspace
of
V. We say that
Ws is the span
of
S, or that S spans WS, or that Ws is spanned by S. Ws is
sometimes denoted by
Span{S}.
The proof ofTheorem 1.1.5is left as Problem 1.8.
basis
defined
1.1.6. Definition. A basis
of
a vector space Vis a set B
of
linearly independent
vectors that spans all
ofV.
A vector space that has afinite basis is calledjinite-
dimensional; otherwise,
itis injinite-dimensional.
We statethe following withoutproof (see [Axle96, page 31]):
components
ofa
vector
in
a
basis
1.1.7.Theorem. All bases
of
a given finite-dimensional vector space have the
same number
of
linearly independentvectors. This number is called thedimension
of
the vector space. A vector space
of
dimension N is sometimes denoted by VN.
If
10)
isavectorinan N-dimensionalvectorspaceVand B = {Ia,}
}~1
a basis
in that space,then by the definitionof a basis, there exists a nnique (seeProblem
1.4) set of scalars{aI,
a2,··.,
an} such that
10)
=
L~l
a, 10,).The set
{ail~l
is called the components of la} withrespect to the basis B.
1.1.8. Example. Thefollowingare
subspaces
of someof thevectorspaces
considered
in
Example 1.1.2.
• The
"space"
of
real
numbers
is a
subspace
of C overthereals,
•
1R
isnota
subspace
of
Coverthecomplex
numbers,
becauseasexplainedin
Example
1.1.2,
1R
cannot
be
a
vector
spaceoverthe
complex
numbers.
• The setof all
vectors
alonga given line going through theorigin is a
subspace
of
arrows
intheplane(orspace)over
JR.
•
P~[t]
is a subspace ofpC[t].
• C
n
-
1
is a
subspace
of
en
whene
n
-
1
is
identified
withall
complex
a-tuples with
zerolast
entry.
In
general,
em
isa
subspace
of
en
form < n when
em
is
identified
withalla-tuples whoselastn - m
elements
are
zero.
•
:MY
XS
isa
subspace
of:M
m
xn forr
::::
m ors
~
n.
Here,
we
identify
anr x s
matrix
withanm x n
matrix
whoselastm - r
rows
andn - s
columns
areall
zero.
•
:P~
[t] is a
subspace
of
p~
[t] form < n.
•
P~[t]
is a
subspace
ofP~[t]
form < n. Note
that
both
P~[t]
and
P~n[t]
are
vector
spaces
Over
the
reals
only.

1.2
INNER
PRODUCT
23
•
IR
m
is a
subspace
of
R"
form
< n. Therefore,lR
2•
the plane,is a subspace
oflR3~
the
Euclidean space. Also, R
1
==
IRis a subspace
of
both
the plane]R2 and the Euclidean
space
IR
3
• II
1.1.9. Example.
The
following are bases
for
the
vector
spaces given in
Example
1.1.2.
•
The
number
1 is a basis for
JR,
which
is therefore one-dimensional.
•
The
numbers 1 and i = .J=Iare basis vectors
for
the
vector
space
Cover
R Thus,
this space is two-dimensional.
• The number 1 is a basis for
Cover
C, and the space is one-dimensional. Note that
although the vectors are the
same
as in the
preceding
item, changing the nature
of
the scalars changes the dimensionality
of
the space.
• The set {ex,ey, cz} of the unit vectors in the directions of the three axes forms a
basis
in space.
The
space is three-dimensional.
• A
basis
of
pert]
can
be
fanned
by the monomials 1, t, (2,
....
It is
clear
that
this
space
is infinite-dimensional.
• A
basis
of
en
is given by
el,
e2,
...,
en,
where ej is an n-tuple that has a 1 at the
standard basis of
en
jth
position and zeros everywhere else.
This
basis
is called the standard
basis
of
en.
Clearly, the space has n dimensions.
• A basis
of
JY[mxn is given by 811, e12,
...
,
eij,
...
, e
mn•
where 8U is the m x n
matrix
with
zeroseverywhere
except
at the intersection
of
the
ithrow
and
jth
column,
where
it has a one.
• A set consisting
of
the monomials 1, t, t
2
,
•••
, t
n
fOnTIS a
basis
of:P~[t].
Thus, this
space
is (n +1)-dimensional.
•
The
standard basis
of
en
is a
basis
of
R"
as well. It is also calledthe standardbasis
oflll
ff
•
Thus,
IR
n
is n-dimensional.
•
If
we assume that a < 0 < b,
then
the
set
of
monomials 1,
x,
x
2
,
...
forms a basis
for e""(a, b), because, by Taylor'stheorem, any fuuctionbelonging to e""(a, b)
can
be expandedin an infinite
power
series
about
x = O.Thus, this space is infinite-
dimensional.
..
Givena space V with a basis B =
{lai)}i~l'
the span of any mvectors (m < n)
of B is an m-dimensional subspace of V.
1.2 Inner Product
A vector space, as given by Definition 1.1.1, is too general and structureless to be
of much physical interest. One useful structure introduced on a vector space is a
scalarproduct. Recallthat the scalar(dot) productof vectorsin the planeor
inspace
is a rule that associates with two vectors a and b, a real number. This association,
denoted symbolically by
g : V x V -->
E,
with
g(a,
b) = a . b, is symmetric:
g(a, b) =
g(b,
a), is linearin the first (and by symmetry, in the second) factor:"
g(aa
+tJb, c) =
ag(a,
c) +tJg(b, c) or
(aa
+tJb) . c =
aa·
c +
tJb·
c,
4A function that is linear in both of its arguments is called a
bilinear
function.

24 1.
VECTORS
AND
TRANSFORMATIONS
gives the "length" of a vector: lal
2
=g(a, a) = a . a 2: 0, and ensures that the
only vector with zero length'' is the zero vector:
g(a, a) = 0 if and only if a =
O.
We want to generalize these properties to abstract vector spaces for which the
scalars are complex numbers. A verbatim generalization of the foregoing proper-
ties, however, leads to a contradiction. Using the linearity in both arguments and
anonzero
la), we obtain
g(i
la),
i
la»
=i
2g(la}
, la» =
-g(la)
, la}).
(1.1)
Dirac
"bra,"
( I,
and
"kef'I ),
notation
Is
used
for
Inner
products.
inner
product
defined
Either the right-hand side (RHS) or left-hand side (LHS) of this equation must be
negative! But this is inconsistent with the positivity of the "length" of a vector,
which requires
g(la) , la» to be positive for
all
nonzero vectors, including i la}.
The source of the problemis the linearity in both arguments.
If
we
can
change this
property in such a way that one of the
i's
in Equation (1.1) comes
out
complex-
conjugated, the problem may go away. This requires linearity in one argument
and complex-conjugate linearity in the other. Which argument is to be complex-
conjugate linear is a matter of convention. We choose the first argument to be so.6
We thus have
g(a
la) +
fJ
Ib), Ie}) =
a*g(la)
, Ie}) +fJ*g(lb) , Ic}),
where a* denotes the complex conjugate. Consistencythen requires us to change
the symmetry property as well.
In fact, we must demand that g(la} ,
Ib»
=
(g(lb) ,
la»)*,from which the reality
of
g(la)
,
la})-anecessary
conditionfor its
positivity-follows
innnediately.
The question of the existence
of
an inner product on a vector space is a deep
problem in higher analysis. Generally,
if
an inner product exists, there may be
many ways to introduce one on a vector space. However, as we shall see in Section
1.2.4, afinite-dimensional vector space always has an innerproduct and this inner
productis unique." So, for all practicalpurposes we can speak of
the inner product
on a finite-diroensional vector space, and as with the two- and three-dimensional
cases, we can omit the letter
g and use a notation that involves only the vectors.
There are several such notations in use, but the one that will be employed in this
book is the
Dirac bra(c)ket notation, whereby g(la} , Ib}) is denoted by (al b).
Using this notation, we have
1.2.1. Definition.
The
inner
product
a/two
vectors, la)
and
Ib), in a vector space
Vis a complex number, (a Ib) E
<C,
such that
1.
(alb)
=
(bla)*
2. (al
(fJ
Ib) +Y Ie}) =
fJ
(al b) +y (al c)
SInour
present
discussion, we are
avoiding
situations
inwhicha
nonzero
vectorcanhavezero
"length."
Suchoccasionsarise
in
relativity,
andwe shalldiscussthemin
Part
VII.
6In some books,
particularly
inthemathematical literature. thesecond
argument
is chosento be
linear.
7TIris
uniqueness
holdsupto a
certain
equivalence
of
inner
products
thatwe shallnotget intohere.

positive
definite,
or
Riemannian
inner
product
sesquilinear
1.2
INNER
PRODUCT
25
3.
(ala)
,,::0,
and
(ala)
=0
ifandonlyif
ja) = 10).
The last relationis calledthe positivedefiniteproperty
of
the innerproduct.
8
Apos-
itive definite inner product is also called a
Riemannian innerproduct, otherwise
it
is calledpseudo-Riemannian.
Note that linearity in the first argument is absent, because, as explainedearlier,
it would be inconsistent with the first property, which expresses the "symmetry"
of the inner product. The extra operation of complex conjugationrenders the true
linearity in the second argumentimpossible. Becauseof this complex conjugation,
the inner product on a complex vector space is not truly bilinear; it is commonly
called sesqnilinear.
A shorthand notation will be useful when dealing with the inner product of a
linear combination of vectors.
1.2.2. Box.
We write the
illS
of
the secondequation in the definition above
as (alPb
+
vc).
This has the advantage of treating a linear combination as a single vector. The
second property then states that
if
the complex scalars happen to be in a ket,
they
"split out" unaffected:
(alpb
+
vc)
= P(al b) +V (al c) .
(1.2)
On the other hand, if the complex scalars happen to be in the first factor (the bra),
then they should be conjugated when they are "split out":
(Pb +
vcla)
=
P*
(bl a) +V* (cla) .
(1.3)
natural
inner
product
forC"
A vector space V on which an inner product is defined is called an
inner
product
space. As mentioned above, all finite-dimensional vector spaces can be
turned into inner product spaces.
1.2.3.Example. Inthis
example
we introduce
some
of
the
most
common
inner
products.
The
reader
is urged to verify that in all cases, we
indeed
have an
inner
product
.
• Let la) , Ib) E
cn,
with
la) =
(ej
, a2,
...
, an) and Ib) = (fJj,
f32,
...
,
f3n),
and
define an
inner
product on
en
as
n
(alb)
=aif31
+a2f32+
..
·+a~f3n
=
I>if3j·
i=l
That this product satisfies all the required properties of an inner product is easily
checked. For example,
if
Ib) = la), we obtain
(al
a) = lal1
2
+
la21
2
+...+
la,,1
2,
which
is clearly nonnegative.
8The positive definiteness must be relaxed in the space-time
of
relativity theory, in which nonzero vectors can have zero
"length."

26 1.
VECTORS
ANO
TRANSFORMATIONS
• Similarly, for la} • Ib) E
JRn
the same definition (without the complex conjugation)
satisfies all the properties
of
an inner product.
• For Ie},Ib} E
<coo
the natural inner product is defined as (al b) =
Lr:;,1
aifJi'The
question of the convergence of this sum is thesubject of Problem 1.16.
• Let
x(t),
y(t)
E
:Pe[t],
the space of all polynomials in t with complex coefficients.
Define
weight
function
of
an
inner
product
defined
in
terms
of
integrals
(xl
y) es L
b
w(t)x*(t)y(t)dt,
(t.4)
naturai
inner
product
for
complex
functions
where a and b are real
numbers--or
infinity-for
which the integralexists, and wet)
is a real-valued, continuous function that is alwaysstrictlypositivein the interval
(a, b). Then Equation (1.4) defines an inner product. Depending on the so-called
weight function
w(t),
there can be many different inner products defined on the
infinite-dimensional space
pC[t].
• Let I, g E
<C(a,
b) and define their inner product by
UI
g)
'"
L
b
w(x)!*(x)g(x)
dx.
It is easily shown that
(II
g) satisfies alt the requirements of the inner product if,
as in the previous case, the weight function
w(x)
is always positive in the interval
(a, b). This is called the standard innerproduct on
<C(o,
b). III
1.2.1 Orthogonality
The
vectors
of
analytic
geometry
and
calculus
are
often
expressed
in
terms
of
unit
vectors
along
the
axes, i.e.,
vectors
that
are
of
unit
length
and
perpendicular
to
one
another.
Such
vectors
are
also
important
in
abstract
inner
product
spaces.
orthogonalilydefined
1.2.4,
Definition.
Vectors la)
,Ib)
E V are
orthogonal
if
(al
b)
=0.
A
normal
vector,or
normalized
vector,
Ie}is
one
for
whicb
(e] e) = 1.A basis B =
[lei)}~1
orthonormal
basis
in an
N-dimensional
vector
space
V is an
orthonormal
basis
if
{
I
ifi
=j,
e, e,
-8"-
( ,I J) - 'J = 0
if
i
'I
i.
Kronecker
delta
where 8ij, defined by the lastequality, is
called
the
Kronecker
delta.
1.2.5.
Example.
Here are examples of orthonormal bases:
• The standard basis of R" (or C"}
leI} =(1,0,
...
,0),
le2)= (0, I, ....
,0),
...
, len} =
(0,0,
...
,1)
is orthonormal under the usual innerproduct
of
those spaces.
(1.5)
(a)
!az)
1.2
INNER
PRODUCT
27
laz)
Ia,)-l
=1£'1)
\
'-
~
I
e2)
\ \
'- '-
~I~)
\ \
I~)
~
~"'a,)11
\------"'1~)
Ie,)
(b) (e) (d)
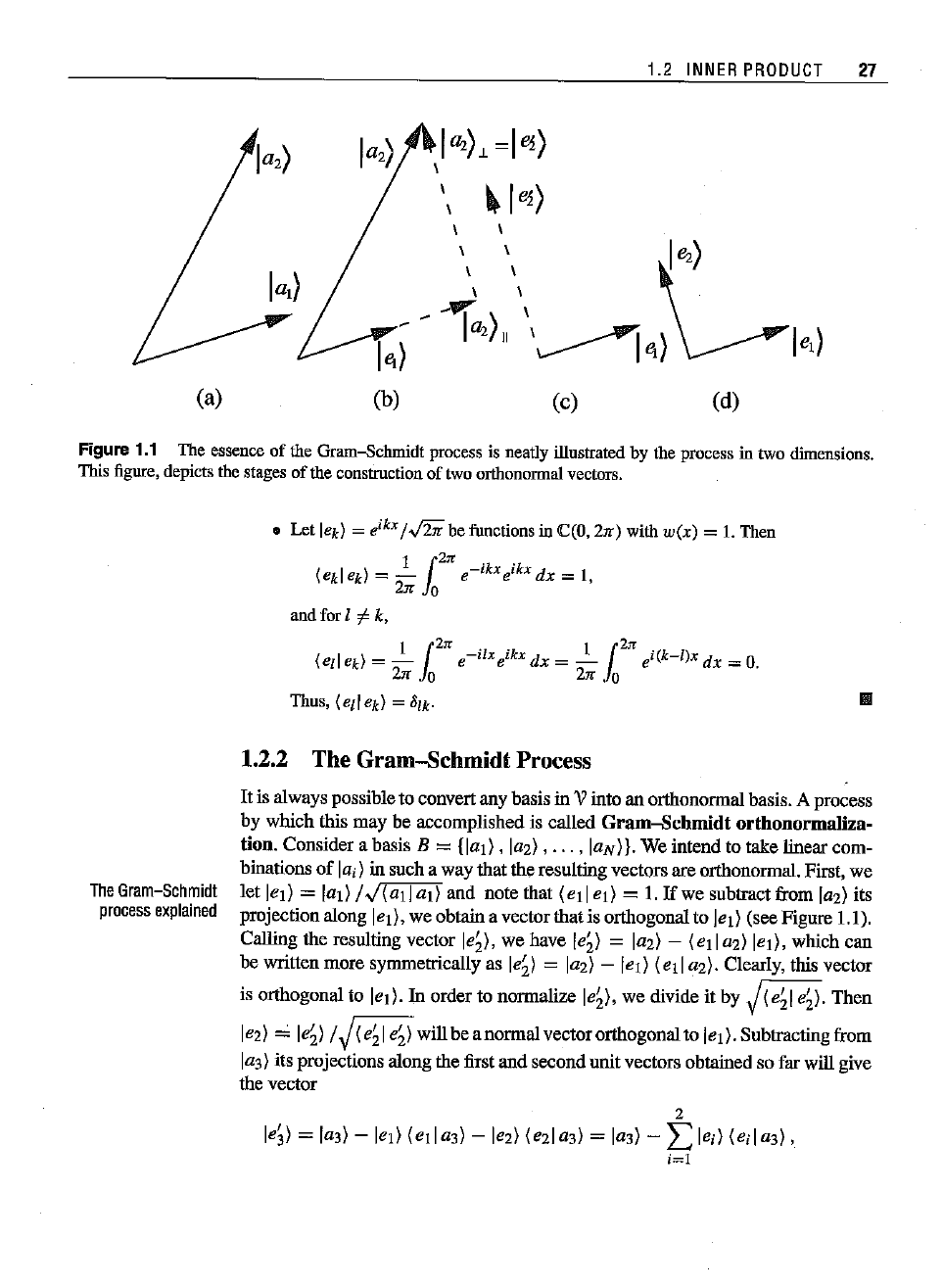
Figure 1.1 The essence of the Gram-Schmidt process is neatly illustrated by the process in two dimensions.
This figure, depicts the stages of the construction of two orthonormal vectors.
•
Lellek)
=e
ikx
/
v'2n'
befunctions in
iC(O,
2".) withw(x) = 1. Then
1
fozn
. .
(ekl ek) =-
e-tkXe,kx
dx
=1,
2".
0
andforI
i'
k,
(ell ek) =
~
f21r
e-ilxi
kx
dx
=
~
f2tr
ei(k-l)x
dx =
o.
2".k 2".k
Thns,
(etlek)
=
~lk'
1.2.2 The Gram-Schmidt Process
III
The
Gram-Schmidt
process
explained
It
is always possibleto convert any basis
in
Vinto an orthonormal basis. A process
by wbich this may be accomplished is called
Gram-Schmidt
orthonormaliza-
tion. Consider a basis B = {lal} , laz},
...
, IaN)}. We intend to take linear com-
binations
of
lai) in such a way that the resulting vectors are orthonormal. First, we
let
leI) =lal)
/v'(atlal)
and note that
(ell
el)
=1.
If
we subtract from laz) its
projectionalong
leI), we obtaina vectorthat is orthogonal to leI) (see Figure 1.1).
Ca1ling the resulting vector
le
z
)'
we have lez) = laz} -
(ell
az) let), which can
be written more symmetrically as
102)
= laz) - leI)
(ell
az). Clearly, this vector
is orthogonal to Iet}.In order to normalize
lez}, we divide it by (ezl e
z).
Then
lez}
==
lez)
/ (ezl ez)will be anormalvectororthogonalto leI). Subtracting from
la3)its projections along the first and second unit vectors obtained so far will give
the vector
z
Ie;) = la3)
-leI}
(ella3)
-lez}
(ezla3) = la3)- L lei} (eil
a3)
,
i=l

28 1.
VECTORS
ANO
TRANSFORMATIONS
la,)
(a)
(b)
(c)
Figure
1.2
Once the orthonormal vectors in the plane
of
two vectors are obtained, the thirdorthonormal vector
is easily constructed.
which
is
orthogonal
to
both
lei)
and
le2)
(see
Fignre
1.2):
=1
~O
I
,..-'-, ,..-'-,
(ell
e3) =
(ell
a3) -
(eli
el)
(ell
a3) -
(ell
e2)(e21a3) = O.
Similarly,
(e21
e~)
= O.
Erhard
Schmidt
(1876-1959) obtained bis doctorate
nnder
the supervision of
David
Hilbert. His
main
interest was in in-
tegral equations and Hilbert spaces. He is the "Schmidt"
of
the
Gram-8chmldtorthogonalization
process, which takes
a basis
of
a space and constructs an orthonormal one from
it. (Laplace had presented a special case of this process
long
before Gram or Schntidt.)
In 1908 Schmidt worked on infinitely many equations in
infinitelymanyunknowns, introducingvariousgeometricno-
tations and terms that are still in use for describing spaces
of
functions. Schmidt's ideas were to
lead
to the geometry
of
Hilbert spaces. This was motivated by the study
of
integral equations (see Chapter 17) and
an attempt at their abstraction.
Earlier, Hilbert regarded a function as given by its Fourier coefficients. These satisfy
the condition that
L~l
a~
is finite. He introduced sequences
of
real numbers {xn} such
that
L~l
x~
is finite.
Riesz
and Fischer showed that there is a one-to-one correspondence
between square-integrable functions and square-summable sequences of their Fourier co-
efficients.
In 1907 Schmidt and Frechet showed that a consistent theory
could
be obtained
if
the square-summable sequences were regardedas the coordinates of points in an infinite-
dimensionalspacethat
isa generalization of n-dimensionalEuclideanspace. Thusftmctions
can be regarded as points
of
a space, now called a
Hilbert
space.

1.2
INNER
PRODUCT
29
In general, if we have calculated m orthonormal vectors
lell,
...
, !em), with
m < N, then we can find the next one using the following relatious:
m
le~+l)
= la
m+!)
-
Lie,)
(eila
m
+,) ,
i=l
!e~+l)
(e~+,1
e~'+l)
(1.6)
Even thoughwehavebeendiscussing finite-dimensionalvectorspaces, the process
of
Equation (1.6) can continue for infinite-dimensions as well. The readeris asked
to pay attention to the fact that, at eacb stage
of
the Gram-Schmidt process,
one
is taking linear combinations of the original vectors.
1.2.3 The SchwarzInequality
Let us now consider an important inequality that is valid in both finite and infinite
dimensions and whose restriction to two and three dimensions is equivalent to the
fact that the cosine of the angle between two vectors is always less
than one. .
1.2.6. Theorem. For any
pair
of
vectors la) , Ib) in an inner
product
space V, the
Schwarz
inequality
Schwarz inequality holds:
(al
a)
(bl
b) 2: 1
(al
b) 1
2
.
Equality holds when la) is
proportional
to Ib).
Proof
Let [c) = Ib) -
((al
b) /
(al
a))
la), and note that
(al
c) =
O.
Write Ib) =
((al
b) /
(al
a») la) +Ic) and take the inner product of Ib) with itself:
l
(alb)1
2
(bib)
=
(ala) (ala)
+
(clc).
Sincethelast
term
isnever
negative,
wehave
(bl
b) > I
(al
b) 1
2
=}
(al
a)
(bl
b) 2: 1
(al
b) 1
2
•
-
(al
a)
Equality holds
iff
(c] c) = 0 or Ic) =
O.
From the definition
of
[c), we conclude
that la) and Ib) must be proportional. 0
Noticethe power of abstraction: Webavederived the Schwarzinequality
solely
from the basic assumptions of inner product spaces independent of the specific
nature of the inner product. Therefore, we do not have to prove the Schwarz
inequality every time we encounter a new inner product space.
Karl HermanAmandusSchwarz(1843-1921) thesonof an
architect,
was
born
inwhat
isnowSobiecin,
Poland.
After
gymnasium.
Schwarz
studied
chemistry
in
Berlin
foratime

30 1.
VECTORS
ANO
TRANSFORMATIONS
before switching to mathematics,receivinghis doctorate in 1864. He was greatly influenced
by the reigning mathematicians in Germany at the time,especially Kummer
and
Weierstrass.
The lecture notes that
Schwan
took while attending Weierstrass's lectures on the integral
calculus still exist. Schwarzreceivedan initial appointmentat Halle
and
later appointments
in
Zurich
and
Gotringen beforebeing
named
as Weierstrass'g successorat Berlin in 1892.
These later years, filled with students and lectures, were not Schwarz's most productive,
buthis earlypapersassurehis place in mathematicshistory.
Schwarz's favorite tool was geometry, which he soon
turnedto the studyof analysis.He conclusively provedsome
of
Riemann's results that
had
been
previously(and justifiably)
challenged. The primaryresult in question was the assertion
that every simply connected region in the plane could be con-
formally mapped onto a circular area. From this effort came
several well-known results now associated with Schwarz's
name, including the principle of reflection and Schwarz's
lemma. He also worked on surfaces of minimal area, the
brancbof geometrybelovedbyallwhodabblewithsoapbub-
bles.
Schwarz's most important work, for the occasion
of
Weierstrass's seventieth birthday,
again dealt with minimal area, specifically whether a minimalsurface yields a minimal area.
Along the way, Schwarz demonstrated second variation in a multiple integral, constructed
a function using successive approximation, and demonstrated the existence of a "least"
eigenvalue for certain differential.equations. This work also contained the most famous
inequality in mathematics, which bears his name.
Schwarz's success obviously stemmed from a matching of his aptitude and training to
the mathematicalproblems of the day. One of his traits, however, couldbe viewed as either
positive or
negative-his
habit of treating all problems, whethertrivial or monumental, with
the same level of attention to detail. This might also at least partly explain the decline in
productivity in Schwarz's later years.
Schwarz had interests outside mathematics, although
his marriage was a mathematical
one, since he married Kummer's daughter. Outside mathematics he was the captain of the
local voluntary fire brigade, and he assisted the stationmaster at the local railway station by
closing the doors of the trains!
1.2.4 Length of a Vector
norm
ofa
vector
defined
In
dealing with objects such as directed line segments in the plane or in space, the
intuitive idea
of
the length
of
a vector is used to define the dot product. However,
sometimes it is moreconvenientto introducethe innerproductfirst
and
then define
the length, as we shall do now.
1.2.7. Definition.
The norm, or length,
of
a vector la) in an innerproduct space
is denoted
by
lIall
and defined as
lIall
==.J"(£il<i).
We use the notation lIaa +
,Bbll
for
the norm
of
the vector a la) +
,B
Ib).
One
can
easily show that the
norm
has the following properties:
