Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations
Подождите немного. Документ загружается.


0.4
CARDINALITY
11
His father urged him to study engineering, and Cantor entered the University
of
Berlin in
1863 with that intention. There he
came
under the influence
of
Weierstrass and turned to
pure mathematics. He became Privatdozent at Halle in 1869 and professor
in 1879. When
hewastwenty-nine hepublished his firstrevolutionarypaper on the theory ofinfinitesetsin
the JournalfUrMathematik.Although some
of
its propositions were deemed faulty by the
older mathematicians,its overalloriginality andbrilliance attracted attention.He continued
to publishpaperson the theoryof sets and on transfinitenumbersuntil 1897.
One
of
Cantor's main concerns was to differentiate among
infinite sets by "size" and, like Balzano before him, he decided
that one-to-one correspondence should be the basic principle.
In his correspondence with
Dedekind
in 1873, Cantorposed the
question
of
whetherthe set
of
real numbers can be
put
into one-
to-one correspondence with the integers, and some weeks later
he answeredin the negative. He gave two proofs.The first ismore
complicated than the second, which is the one most often used
today.
In
1874 Cantor occupiedhimselfwith the equivalence
of
the points
of
a line and the points
of
R" and sought to prove
that a one-to-one correspondence between these two sets was
impossible. Three years later he proved that there is such a correspondence. He wrote to
Dedekind, "I see
it but I do not believe it." He later showed that given any set, it is always
possible to create a new set, the set
of
subsets
of
the given set, whose cardinal number is
larger than that of the given set.
If
1'::0
is the given set, then the cardinal number
of
the set
of
subsets is denoted by
2~o.
Cantor proved that
2~O
= C, where c is the cardinal number
of
the continuum; i.e., the set
of
real numbers.
Cantor's work, which resolved age-old problems and reversed much previous thought,
could hardly be expected to receive immediate acceptance. His ideas on transfinite ordi-
nal and cardinal numbers aroused the hostility
of
the powerful
Leopold
Kronecker, who
attacked Cantor's theory savagely over more
than a decade, repeatedly preventing Cantor
from obtaining a more prominent appointment in Berlin. Though Kronecker died in 1891,
his attacks left mathematicians suspicious
of
Cantor's work. Poincarereferred to set theory
as an interesting "pathologicalcase." He also predicted that "Latergenerations
will regard
[Cantor's]
Mengenlehre as 'a disease from which one has recovered." At one time Cantor
suffered a nervous breakdown, but resumed work in 1887.
Many prominentmathematicians,however, were impressedby the uses towhichthe new
theoryhadalreadybeenpatinanalysis,measuretheory,andtopology.
Hilbert
spreadCantor's
ideas in Germany, and in 1926 said,
"No
one shall expel us from the paradise which Cantor
created for us." He praised Cantor's transfinite arithmetic as "the most astonishing product
of
mathematical thought, one
of
the most beautiful realizations
of
human activity in the
domain
of
the purelyintelligible." BertrandRusselldescribedCantor'swork as "probablythe
greatest
of
which the age can boast." The subsequentutility
of
Cantor's work in formalizing
mathematics-a
movement largely led by
Hilbert-seems
at odds with Cantor's Platonic
view that the greater importance
of
his work was in its implications for metaphysics and
theology. That his work could be so seainlessly diverted from the goals intended by its
creator is strong testimony to its objectivity and craftsmanship.

12
O.
MATHEMATICAL
PRELIMINARIES
countably
infinite
uncountable
sets
Cantor
set
constructed
Now consider the set of natnral numbers N =
{l,
2, 3,
...
}.
If
there exists a
bijection between a set
A and N, then A is said to be
countably
infinite. Some
examples of countablyinfinite sets are the set of all integers,the set
of
even natnral
numbers, the set of odd natnral numbers, the set of all prime numbers, and the set
of energy levels
of
the bound states of a hydrogen atom.
It
may seem surprising that a subset (such as the set
of
all even numbers)
can be put into one-to-one correspondence with the full set (the set of all natural
numbers); however, this is a property shared by all
infinite sets. In fact, sometimes
infinite sets are
defined as those sets that are in one-to-one correspondence with at
leastone of their proper subsets.
It
is also astonishing to discover that there are as
many rational numbers as there are natnral numbers. After all, there are infinitely
many rational numbers
just
in the interval (0, I
)-or
betweenany two distinctreal
numbers.
Sets that are neitherfinite
nor
countablyinfinite are said to be
uncountable.
In
some sense they are "more infinite" than any countable set. Examples of uncount-
able sets are the points in the interval
(-1,
+1), the real numbers, the points in a
plane, and the points in space.
It
can
be shown that these sets have the same cardinal-
ity: There are as many points in three-dimensional
space-the
whole
universe-as
there are in the interval
(-I,
+1) or in any other finite interval.
Cardinalityis avery intricatemathematicalnotionwith many surprisingresults.
Consider the interval [0, 1].Remove the openinterval
(~,
~)
from its middle. This
means that the points
~
and
~
will not be removed. From the remaining portion,
[0,
~]
U
[~,
1], remove the two middle thirds; the remaining portion will then be
[0,
~]
U
[~,
~]
U
[~,
~]
U
[~,
1]
(see Figure 5). Do this indefinitely. What is the cardinality
of
the remaining set,
which is called the
Cantor
set? Intuitively we expect hardly anything to be left.
We might persuade ourselves into accepting the fact that the number of points
remainingis at mostinfinite but countable. The surprisingfact isthat the cardinality
is that
of
the continuum! Thus, afterremoval of infinitely many middle thirds, the
set that remains has as many points as the original set!
0.5 Mathematical Induction
Many a time it is desirable to make a mathematical statement that is true for all
natural numbers. For example, we may want to establish a formula involving an
integerparameterthat will hold for all positiveintegers. One encounters this situa-
tion when, after experimenting with the first few positive integers, one recognizes
a pattern and discovers a formula, and wants to make sure that the formula holds
for all natural numbers.
For
this purpose, one uses
mathematical
induction. The
induction
principle
essence
of
mathematical induction is stated as follows:
(2)
0.5
MATHEMATICAL
INDUCTION
13
0------------------
1------
2--
3-
4--
Figure5 The
Cantor
set
after
one,two,
three,
and
four
"dissections."
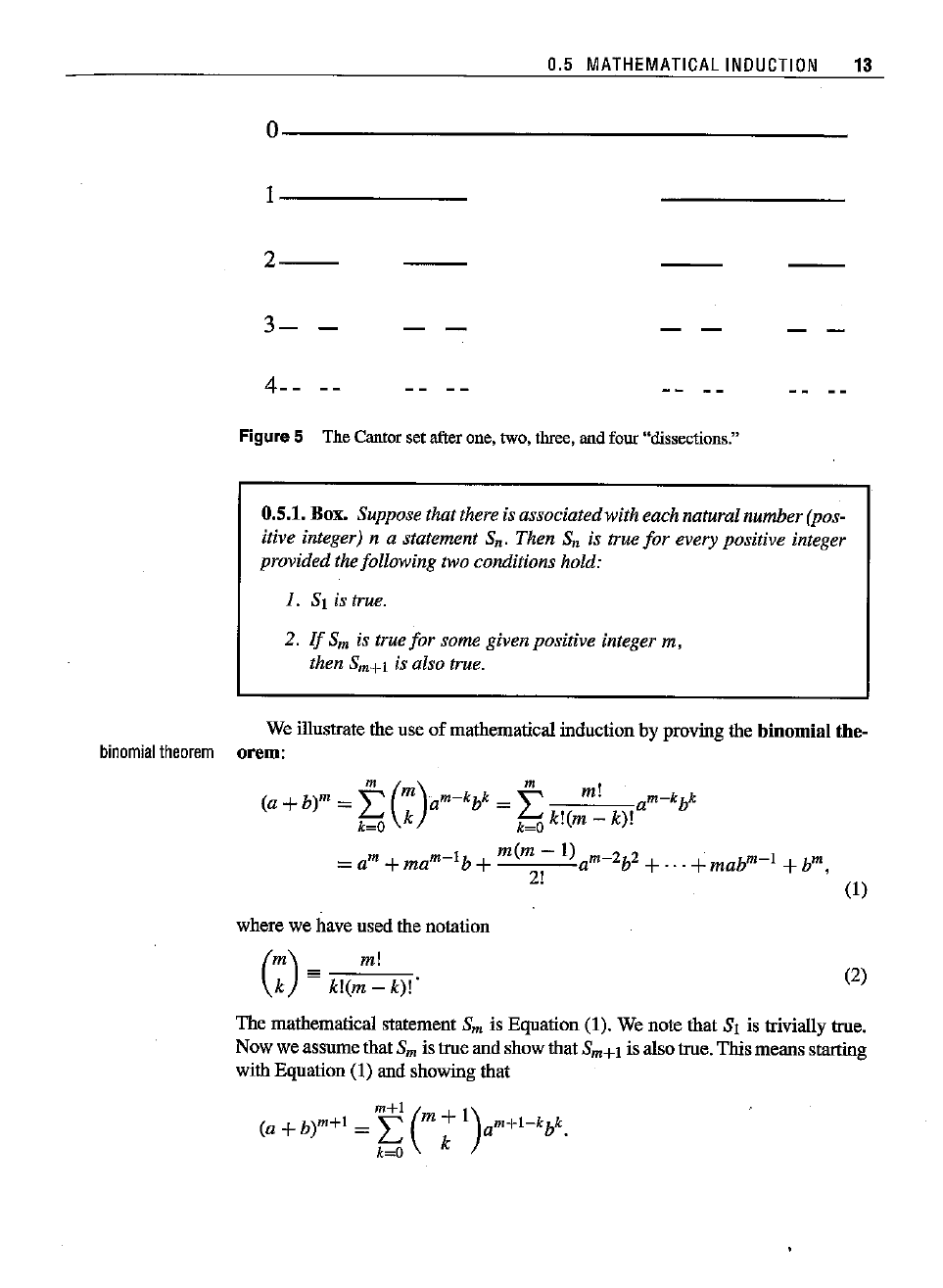
0.5.1. Box. Suppose that there is associatedwitheach naturalnumber(pos-
itive integer) n a statement Sn. Then Sn is true
for
every positive integer
provided the following two conditions hold:
I.
S, is true.
2.
If
Sm is true
for
some given positive integer m,
then Sm+l is also true.
We illustrate the use
of
mathematical induction by proving the
binomial
the-
binomial
theorem
orem:
where we have used the notation
(;)
'"
k!(mm~
k)!'
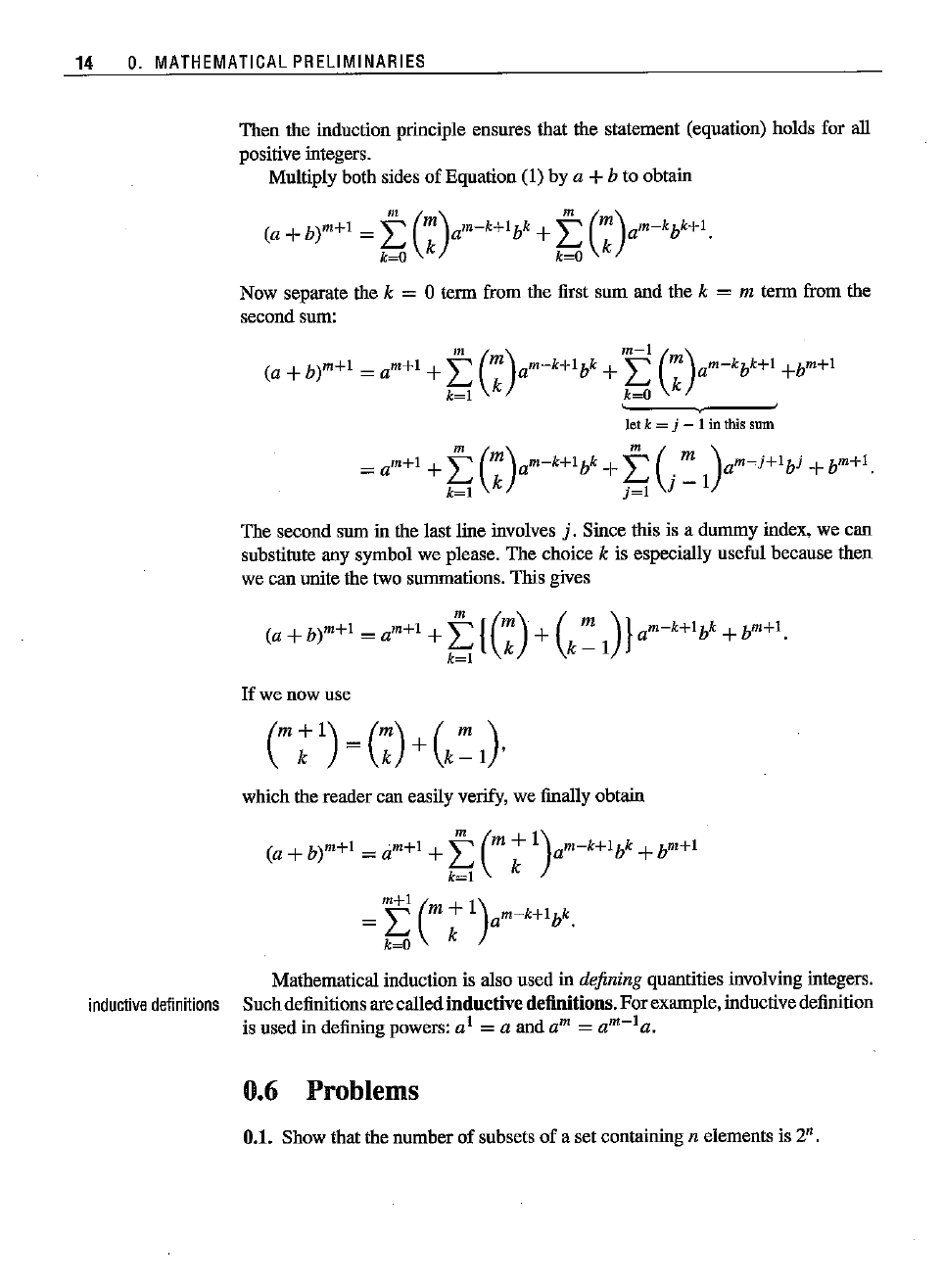
The mathematical statement Sm is Equation (1). We note that SI is trivially true.
Now we assume that
Smis true and show that
Sm+1
is also true. This means starting
with Equation (1) and showing that
14
O.
MATHEMATICAL
PRELIMINARIES
Then the induction principle ensures that the statement (equation) holds for all
positive
integers.
Multiply both sides
of
Eqnation
(I)
by a +b to obtain
(a
+b)m+l
= t
(rn)a
m
- k+l
b
k
+t
(rn)a
m
-
k
b
k
+
1
.
k~O
k k=O k
Now separate the k = 0 term from the first sum and the k =
rn
term from the
second
sum:
letk=j-linthissum
The
second sum in the last line involves
j.
Since this is a dummy index, we can
substitute any symbol we please.
The
choice k is especially useful because then
we canunitethetwo
summations.
Thisgives
If
we now use
which the reader can easily verify, we finally obtain
Mathematical induction is also used in
defining quantities involving integers.
inductive
definitions
Suchdefinitions are called
inductive
definitions.
For
example,inductivedefinition
is used in defining powers: a
l
= a and am =
am-lao
0.6 Problems
0.1. Show that the number
of
subsets
of
a set containing n elements is 2".

tk=
n(n+
I).
k=O 2
0.6
PROBLEMS
15
0.2. Let A, B, and C be sets in a universal set U. Show that
(a)
A C
BandB
C
CimpliesA
C C.
(b)
AcBiffAnB=AiffAUB=B.
(c) A c
Band
B C C implies (A U B) c C.
(d)
AU
B = (A
~
B) U (A nB) U (B ~ A).
Hint: To show the equality
of
two sets, show that each set is a subset of the other.
0.3. For each n E N, let
In = I
xlix
- II < n and Ix +II >
~}
.
Find UnI
n
and nnIn.
0.4. Show that a' E [a] implies that [a'] = [a].
0.5. Can you define a binary .operatiou
of
"multiplication" on the set of vectors in
space? What about vectors in the plane?
0.6. Show that
(f
a
g)-1
=
g-1
a
1-1
when I and g are both bijections.
0.7. Take any two open intervals (a, b) and (c,
d),
and show that there are as many
points in the first as there are in the second, regardless of the size of the intervals.
Hint: Find a (linear) algebraic relation between points of the two intervals.
0.8. Use mathematical induction to derive the Leibniz rulefor differentiating a
Leibniz
rule
product:
d
n
n
(n)
d
k
I
dn-kg
dx
n
(f.
g) =
'E
k
-d
k d
n-k'
k=O x x
0.9. Use mathematical induction to derive the following results:
n k r
n
+
1
-
1
'E
r
= ,
k=O r - I
Additional Reading
1. Halmos, P.Naive Set Theory, Springer-Verlag, 1974. A classic text on intu-
itive (as opposed to axiomatic) set theory coveriog all the topics discussed
in this chapter and much more.
2. Kelley, J. General Topology,Springer-Verlag, 1985. The introductory chap-
ter of this classic reference is a detailed introduction to set theory and map-
pings.
3. Simmons,
G.lntroduction to TopologyandModernAnalysis, Krieger, 1983.
The first chapter
of
this book covers not only set theory and mappings, but
also the Cantor set and the fact that integers are as abundant as rational
numbers.


Part I _
Finite-Dimensional Vector Spaces

j
j
j
j
j
j
j
j
j
j
j
j
j
j
j

1 _
Vectors
and
Transformations
Two- and three-dimensional
vectors-undoubtedly
familiarobjectsto
the
reader-
can easily be generalized to higher dimensions. Representing vectors by their
components,
onecanconceiveof
vectors
having
N
components.
Thisis themost
immediate generalization
of
vectors in the plane and in space, and such vectors
are called N-dimensional
Cartesian vectors. Cartesian vectors are limited in two
respects: Their components are real, and their dimensionality is finite. Some ap-
plications
in physics require the removal
of
one
or both
of
these limitations.
It
is
therefore convenient to study vectors stripped
of
any dimensionality or reality
of
components. Such properties become consequences
of
more fundamental defini-
tions. Although we will be concentrating on finite-dimensional vector spaces
in
this part
of
the book, many
of
the concepts and examples introduced here apply to
infinite-dimensional spaces as well.
1.1
Vector
Spaces
Let us begin with the definition
of
an abstract (complex) vector space.'
1.1.1.Definition. A vector space Vover
<C
is a set
oj
objects denoted by 10), Ib),
vector
space
defined
[z),
and
so on, called vectors, with the following propertiesr:
1. Toevery
pair
ofvectors la) and Ib) in Vthere correspondsa vector la)+Ib),
also in
V,
called the sum
oj
la)
and
Ib), such that
(a) la)
+
Ib)
= Ib) +la),
1Keepinmind
that
C is thesetof complex
numbers
andR thesetof
reels.
2Thebra, (I,andket, I),
notation
for
vectors,
invented
by
Dirac,
is veryusefulwhendealingwithcomplexvectorspaces.
However,
itis
somewhat
clumsyfor
certain
topicssuchasnannand
metrics
andwill
therefore
be
abandoned
inthosediscussions.

20 1.
VECTORS
ANO
TRANSFORMATIONS
(b) la} +(Ib) +Ie)) = (Ia) +Ib)) +[c),
(c) There exists a unique vector
10}
E V, called the zero vector, such that
la}
+
10)
=
Ja}
for every vector la),
(d) Toevery vector la}
E Vthere corresponds a unique vector -
Ja}
(also
in
V) such that la) +
(-Ia})
= 10).
scalars
are
numbers
complex
VS.
real
vector
space
concept
of
field
summarized
2. To every complex number:
a-{llso
called a scalar-s-and every vector la)
there corresponds a vector a la) in V such that
(a) a(f3la})
= (af3) la),
(b) J ]a) = la).
3. Multiplication involving vectors and scalars is distributive:
(a) a(la) +Ib}) =
ala)
+
alb).
(b) (a +
13)
la} =
ala)
+f3la).
The
vector space defined above is also called a
complex
vector
space. It is
possibleto replace
iC
with
IR-the
set
of
realnumbers-s-in whichcase the resulting
space will be calleda
real
vector
space.Real and complexnumbers are prototypes
of
a mathematical structure called field. A field is a set
of
objects with two binary
operations called addition and multiplication.
Each
operation distributes with re-
spect to the other, and each operation has an identity.
The
identity for addition is
denoted by
°and is called additive identity. The identity for multiplication is de-
noted by I and is called
multiplicativeidentity. Furthermore, every elementhas an
additiveinverse, and every elementexceptthe additive identity has a multiplicative
inverse.
1.1.2.
Example.
SOME VECTOR SPACES
1.
1R
is a
vector
space
over
the
fieldof
real
numbers.
2. C is a
vector
space
overthefieldofreal
numbers.
3. C is a vector space over the
complex
numbers.
4. LetV= R
and
letthefieldof
scalars
heC.Thisisnot a
vector
space,
because
property
2
of
Definition
1.1.1
is not
satisfied:
A complex
number
timesareal
number
is not
areal
number
and
therefore
doesnotbelongtoV.
5. Theset
of"arrows"intheplane(orin
space)
forms
a
vector
space
over
1R
under
the
parallelogram lawof additiooof planar(or spatial)vectors.
3Complex
numbers,
particularly
whentheyare
treated
as variables, areusually
denoted
by z, andwe shall
adhere
to this
convention
in
Part
ITI.
However,
inthediscussionof vectorspaces,we havefoundit more
convenient
touse lowercase Greek
letters
todenotecomplex
numbers
as
scalars.
