Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations

Подождите немного. Документ загружается.

1.4

ALGEBRAS

1.3.19.

Example.

Let

andVbe vectorspaceswithbasesBU =

Iluj

)}i'~l

and BV =

(IVj}}j=l'

respectively. Consider an mn-dimensional vector space W whose basis

is in one-to-one correspondence with the pairs

(lUi),

IVj»,

and let !UiVj) be the vector

corresponding to

(Iuj),

IVj»' For lu)

Ell

with components

10I;}i'=1

in Bu and [v) E V

with components {11jlj=l in

, definethe vector

lu,

v} E W whosecomponents in

are

{OIi~j}'

One can easily sbowthat if lu), lu'), and lu") are vectorsin

and lu") =

01lu) +

lu'), then

[a". v) = 01 lu, v) +

lu', v)

ThespaceW thus

defined

is calledthe tensor product oflL andVanddenotedby

<8>

Onecan also

define

tensorproductof three or more vector

spaces.

Of specialinterest

are tensor products

a vector space and its dual. The tensor product space Vr,s of type

(r,s) is definedas

follows:

V,., = V

<8>

...

V<8>V*

<8>V*

<8>

...

<8>

'-v-"

, .

r times s times

Wesballcomebackto thisspacein Cbapter25.

1.4 Algebras

III

In many physical applications, a vector space has a natural "product," the prime

examplebeing the vector space of matrices.

is therefore useful to considervector

spaces for which such a product exists.

algebra

defined

1.4.1. Definition. An algebra A over

(or IR)is a vector space over

(or

IR),

together with a binary operation /L : V x V -+

called multiplication, that

satisfies

a(pb

+ye) =

pab

yac

Va,

b, e E

Vp,Y E

(or IR),

dimension

the

algebra;

associativity;

commutativity;

identity;

and

right

and

left

inverses

with a similar relation

for

multiplication on the right. The dimension

the vector

space is called the dimension

the

algebra. The algebra is calledassociative if

the product satisfies a(bc) = (ab)e and commutative if it satisfies

= ba. An

algebra with identity is an algebra that has an element1 satisfying

=a.

An element b

an algebra with identity is saidto be a

left

inverse

ifba

= 1.

Right

inverse is defined similarly.

1.4.2.

Example.

Definethefollowing producton

]Rz:

(xj

, XZ)(Yl, yz) = (XIYl - XZYZ,

X1YZ

+XZYl)·

The reader is urged to verifythat this productturns

JR2

into acommutativealgebra.

15Weshall abandon the Dirac bra-and-ket notation in this section due to its clumsiness; instead we use boldface roman letters

to denote vectors.

is customary to write ab for p,(a, b).

42 1.

VECTORS

ANO

TRANSFORMATIONS

Similarly,

thevector(cross)

product

lR.

turns

itinto anonassociative,

noncornmu-

tative

algebra.

The

paradigm

of all

algebras

is thematrix algebra whose

binary

operation

ordinary

multiplication of n x n

matrices.

This

algebra

is associative butnot

commutative.

All

the

examples

above

are

finite-dimensional

algebras.

example

of an

infinite-

dimensional

algebra

eOO(a,

b), the vectorspaceof infinitely

differentiable

real-valued

functions

onareal

interval

(a, b). The

multiplication

defioed

pointwise:

H f E

eOO(a,

andg E

eOO(a,

b),

then

This

algebra

commutative

andassociative.

fg(x)

f(x)g(x)

v x E (a, b).

derivation

algebra

defined

The last item in the example abovehas a feature that turns out to be of great

significancein

all algebras,the product rule for differentiation.

1.4.3.Definition. A vectorspace endomorphismD : A

--+

A iscalledaderivation

A ifit has the additionalproperty

D(ab) = [D(a)]b +a[D(b)].

1.4.4. Example. LetA bethesetofn x n

matrices.

Definethe

binary

operation,

denoted

bye,

AoB"'AB-BA,

where

the RHSis

ordinary

matrix

multiplication. The

reader

may checkthatA

together

withthis

operation

becomesan

algebra.

Nowlet Abe a fixed

matrix,

anddefinethe

linear

transformation

Thenwe note

that

DA(B

0 C) =

(B.

C) =

A(B.

C) - (B 0 C)A

=A~-~-~-C~=~-_-_+~

Onthe

other

hand,

(DAB). C +

(DAC)

(AoB)

.C+B.

(AoC)

(AB-

BA)

.C+B.

(AC

- CAl

(AB

- BA)C- C(AB-

BA)

B(AC

- CAl - (AC- CA)B

= ABC+ CBA- BCA-

ACB.

So,

is a

derivation

on A.

Thelineartransformations connectiogvectorspacescanbemodifiedslightlyto

accommodatethebinaryoperationofmultiplicationofthecorrespondingalgebras:

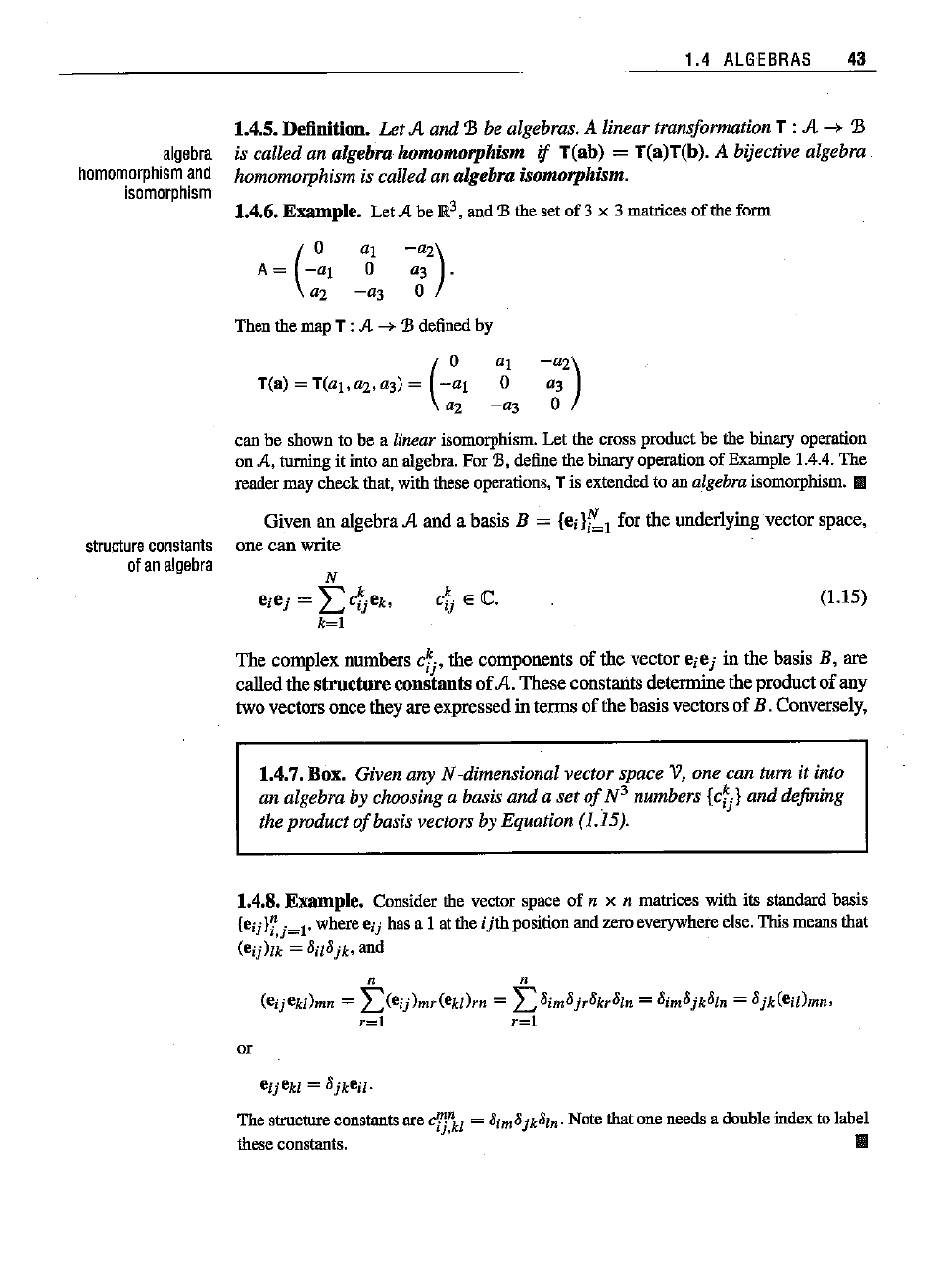
(1.15)

algebra

homomorphism

and

isomorphism

structure

constants

ofan

algebra

1.4

ALGEBRAS

1.4.5.Definition. Let A and

be algebras. A linear transformation T : A .....

is called an algebra homomorphism if

T(ab)

= T(a)T(b). A bijective algebra

homomorphism is called an algebra isomorphism.

1.4.6.Example. LetA be

]R3,

and

:8 thesetof3 x 3

matrices

ofthe

form

Then

the

map

T : A

defined

canbe

shown

to be a linear

isomorphism.

Let the cross

product

be the

binary

operation

onA,

tnming

itintoan

algebra.

For B,

define

the

binary

operation

Example

1.4.4. The

reader

maycheck

that,

withthese

operations,

Tis

extended

toanalgebra

isomorphism.

IlIlI

Given an algebraA and a basis B =

(e;}~1

for the underlyingvector space,

onecan

write

eiej

Lc~ekl

k~1

The complex numbers ef

the components of the vector

eiej

in the basis

are

calledthe structureconstantsof

Theseconstantsdeterminetheproductofany

twovectorsoncethey

are expressedinterms of thebasisvectorsof B. Conversely,

1.4.7.Box. Given any N-dimensional vector space

one can turn it into

an algebra by choosing a basis

and

a set

ofN

numbers

{c~}

and

defining

the product

basis vectors by Equation (1.15).

1.4.8.

Example.

Consider the vector space of n x n matrices with its

standard

basis

{ei]'}~

"-I'

wheree., hasa 1atthe ijthpositionandzero

everywhere

else.This

means

that

l,j_

(eij)zk = 8il8jko

and

n n

(eijekl)mn =

L(eij)mr(ekl)rn

L~im~jr8kr8In

lJimlJjk8In

= 8jk(eU)mn,

r=1 r=1

eijekl

8jk

eil·

The

structure

constantsare

cjj~kl

8im8jk8ln.

Note thatone needs adoubleindex to label

these constants. II

44 1.

VECTORS

AND

TRANSFORMATIONS

1.4.9. Example. In the

standard

basis{eil ofR4,

choose

the

structore

constants

fol-

lows:

222

et = -02 =

-e

-e4

=ej,

elei

= eie! = ei for i = 2, 3, 4,

eie

j = L Eijkek for i, j = 2, 3, 4,

algebra

quaternions

[<ijk is

defined

Equation

(3.19)].

The

reader

may

verify

that

these

relations

turn R

intoanassociative, but

noncommutative,

algebra,

calledthealgebraof quaternions

and

denoted

1HI.

this

context,

ej is

usually

denoted

byI, ande2,e3,ande4byi,

andk,

respectively,

and

one

writes

q = x +iy +iz +kw foran

element

oflBI.

It thenbecomes

evident

that

1HI

isa

generalization

ofC.

analogy

withC,x is

called

therealpart ofq, and

(y, z, w) thepurepart ofq.

Similarly,

theconjugateofq isq* = x -

- iz - kw.

III

Algebras have a surprisingly rich structure and are used extensively in many

branches of mathematics and physics. We shall see their usefulness in our dis-

cussion of group theory in Part vn. To ciose this section, and to complete this

introductory discussionof algebras, we cite a few more useful notions.

left,

right,

end

1.4.10. Definition.

Let

A be an algebra. A subspace

A is calledasubalgebra

two-sided

ideals

A if'B contains the products

all its members. If'B has the extra property that

it contains ab

for

all a E A

and

b E

'B,

then 'Bis called a left ideal

A. A right

ideal and a two-sided ideal are defined similarly.

is ciear from the definition that an ideal is automatically a subalgebra, and

that

1.4.11. Box. No proper ideal

an algebra with identity can contain the

identity element.

In fact, no proper left (right) ideal can contain an element that has a left (right)

minimal

ideal

inverse.An ideal can itselfcontain a proper (sub)ideal.

anideal doesnot contain

any proper subideal, it is called a minimal ideal.

1.4.12. Example. The

vector

space

eO(a, b) ofall

contiuuous

real-valued

functions

interval

(a, b) is

turned

into a commutative algebra by pointwise

multiplication:

f, g E eO(a,b), thenthe

product

defined

fg(x)

f(x)g(x)

forallx E (a, b).

Theset of

functions

that

vanish

at a given fixedpointC E (a, b)

constitutes

ideal

eO(a, b). Sincethe

algebra

commutative,

theidealis two-sided. II

ideals

generated

element

algebra

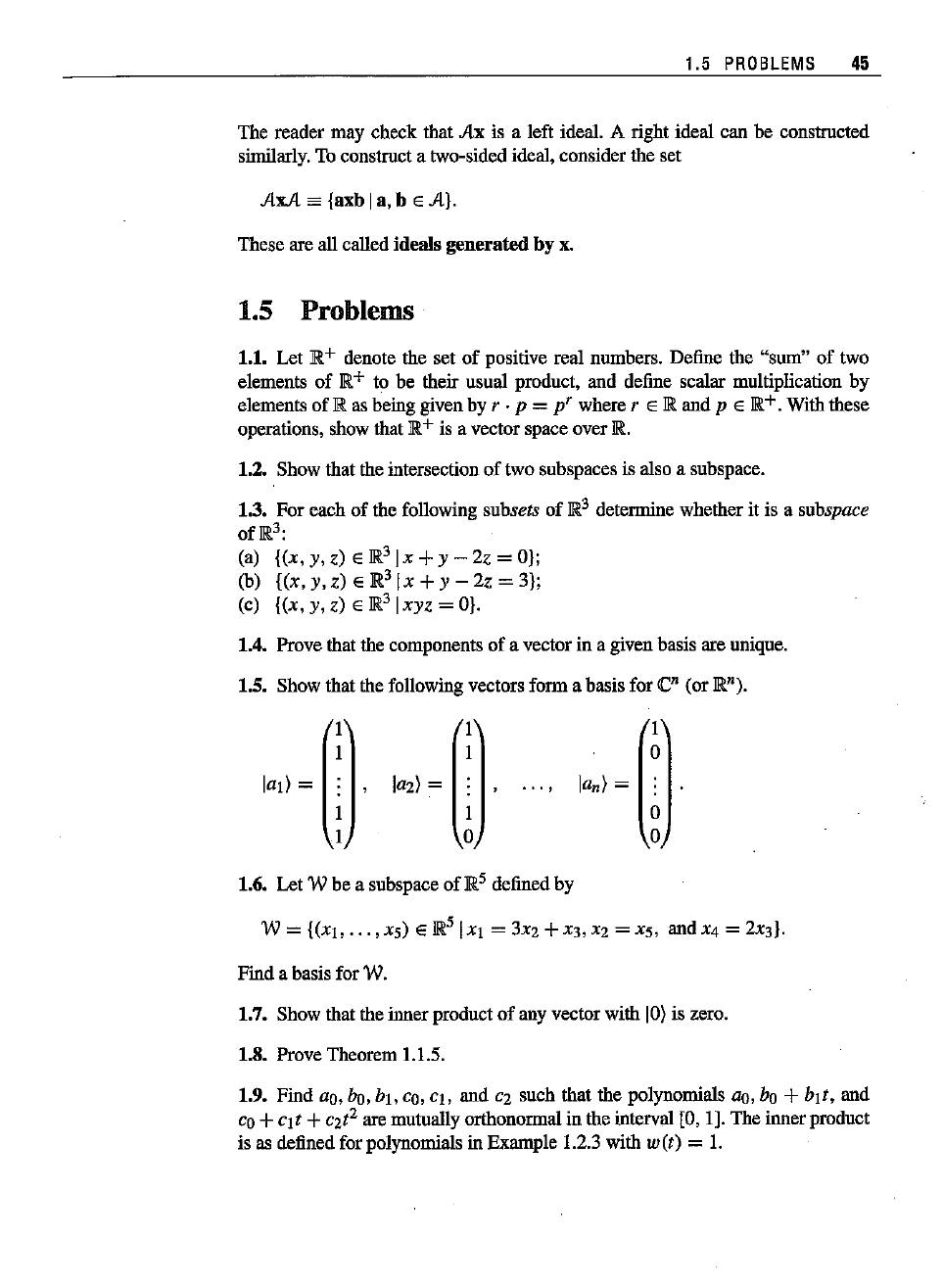
One can easily constructleft ideals for an algebra A: Takeany element x E A

and consider the set

Ax",

{ax Ia E

A}.

1.5

PROBLEMS

The reader may check that .Ax is a left ideal. A right ideal can be constructed

similarly. To construct a two-sided ideal, consider the set

.AKA

{axb Ia, h E .A}.

These are all called ideals generated

1.5 Problems

1.1. Let R+ denote the set

positive real nnmbers. Define the "sum" of two

elements of

R+ to be their usual product, and define scalar multiplication hy

elements of

R as being given by r . p =

where r E R and p E R+. With these

operations, show that

R+ is a vector space over R

1.2. Show that the intersection of two subspaces is also a subspace.

1.3.

For

each of the following subsets of R

determine whether it is a subspace

ofR

(a) {(X, y, z)

jx + y - 2z = 0};

(b)

{(x,y,z)ER

3Ix+y-

2z=3);

xyz

OJ.

1.4. Prove that the components of a vector in a given basis are unique.

1.5. Show that the following vectors form a basis for

(or R

laz)

...,

1.6. Let W be a subspace of R5 defined by

{(Xl,

...

,X5) E R

1xI = 3xz

+X3,

Xz = X5, and xa = 2x3}.

Find a basis for W.

1.7. Show that the inner product

any vector with

10)

is zero.

1.8. Prove Theorem 1.1.5.

1.9. Find

aD,

bo,

bl,

CO,

Ci, and cz such that the polynomials

aD,

+bIt, and

Cit

czt

are mutually orthonormal in the interval [0, I]. The inner product

is as defined for polynomials in Example 1.2.3 with

wet) = 1.

46 1.

VECTORS

AND

TRANSFORMATIONS

1.10. Given the linearly independent vectors

x(t)

= t", for n = 0, 1,2,

...

in pc[I], use the Gram-Schmidt process to find the orthonormal polynomials

eo(t),

(t),

and e2(t)

(a) when the inner product is defined as

(xl

y) =

J~l

x*(t)y(t)

dt.

(b) when the inner product is defined with a nontrivial weight function:

(xl y) =

e-t'x*(t)y(t)

dt.

Hint: Use the following result:

..;n

e-ttndt=

ioo

·3·5

· (n - 1)

..;n

ifn

= 0,

n is odd,

if n is even.

1.11.

(a) Use the Gram-Schmidt process to find an orthonormal set of vectors

out of

(I,

-I,

1),

(-1,0,

I), and (2,

-1,2).

(b) Are these three vectors linearly independent?

not, find a zero linear combi-

nation

them by using part (a).

1.12.

(a) Use the Gram-Schmidt process to find an orthonormal set

vectors

out

(I,

-I,

2),

(-2,

-1),

and

(-1,

-I,

4).

(b) Are these three vectors linearly independent?

not, find a zero linear combi-

nation

them by using part (a).

1.13. Show that

1.14. Show that

dy (x

- x

+2x

- 2)(y5

-l

2)e-(x

)

dy (x

l)(i

+4)e-(x

+y').

Hint: Define an appropriate inner product and use the Schwarz inequality.

1.15. Show that for any set

n complex numbers

ai,

a2,

...

, an, we have

la1+a2 +...+an1

(la112

la21

+...+

Ian

Hint: Apply the Schwarz inequality to

(I,

...

,1)

and (ai, a2,

...

, an).

1.5

PROBLEMS

1.16. Using the Schwarz inequality show that

(a;}f,;;,

and

ltl;}f,;;,

are iu

iCoo,

then

Lf,;;,

fJ;

is convergent.

1.17. Show thatT :

-->

given

byT(x,

y) = (x

y2,x

+ y, 2x - y) is not

a linear mapping.

1.18. Verify that all the transformations

Example 1.3.4 are linear.

1.19.

Let

17:

be the permutation that takes

(1,2,3)

to (3, 1, 2). Find

Ie;}, i =

1,2,3,

where {Ie;

}}T=,

is the standard basis ofIR

(or

and A" is as defined in Exam-

ple 1.3.4.

1.20. Show that

TEI:,(iC,

C),

then there exists a E iCsuch that T la} =

ala}

for all la) E C.

1.21. Showthatif

(la;}}f~l

spans Vand

TEI:,(V,

is surjective,then(T la,

}}f=l

spans W.

1.22. Give an example

a function f :

-->

suchthat

f(a

la}) =

af(la})

and la} E

but f is not linear. Hint: Consider a homogeneous function of degree 1.

1.23. Show that the following transformations are linear:

(a) V is

ic over the reals and C [z) = [z"). Is C linear

instead

real numbers,

complex numbers are

used

as scalars?

(b) V is

pelt]

and T

Ix(t)

Ix(t

+ 1) -

Ix(t)}.

1.24. Verify that the kernel

a transformation T : V --> W is a subspace

and that T(V) is a subspace

1.25. Let V and W be finite dimensional vectorspaces. Show

thatifTEI:,(V,

is surjective, then dim W :::dim V.

1.26. Suppose that V is finite dimensional and

TEl:,

(V,

is not zero. Prove

that there exists a subspace U

V such that ker T nU =

(OJ

and T(V) =T(U).

1.27. Show that

is a subspace

V* and

dim V

dimW+

dimWo.

1.28. Show that T and

have the same rank. In particular, show that

T is

injective, then

is surjective, Hint: Use the dimension theorem for T and T* and

Equation (1.11).

48 1.

VECTORS

ANO

TRANSFORMATIONS

1.29. Show that (a) the product on

defiued by

turns

into an associative and commutative algebra, and (b) the cross product

IR.3

turns

it intoanonassociative,

noncommutative

algebra.

1.30. Fix a vector a E

and define the linear transformation

D. :

IR3

-4

D.(h)

= a x b. Show that D. is a derivation ofIR

with the cross product as

multiplication.

1.31. Show that the linear transformation of Example 1.4.6 is an isomorphism of

the two algebras

A and

11.

1.32. Write down all the structure constants for the algebra of quaternions. Show

that this algebra is associative.

1.33. Show that a quaternion is real iff it commntes with every quaternion and

that it is pure

iff

its square is a nonpositive real number.

1.34.

Let

p and q be two quaternions. Show that

(a) (pq)* =

q*p*.

(b) q E

IRiffq*

= q,

andq

iffq*

-q.

1.35. Show that no

properleft (right) ideal of an algebrawith identity can contain

an element that has a left (right) inverse.

1.36.

Let

A be an algebra, and x E A. Show that

is a left ideal, xA is a right

ideal, and

AxA

is a two-sided ideal.

Additional Reading

I. Alder, S. LinearAlgebra Done Right, Springer-Verlag, 1996. A small text

packed with information. Lots of marginal notes and historical remarks.

2. Greub,

W.LinearAlgebra, 4th ed., Springer-Verlag, 1975. Chapter V has a

good discussion of algebras and their properties.

3. Halmos, P.Finite DimensionalVector Spaces, 2nd ed., VanNostrand, 1958.

Another great bookby the master expositor.

2 _

Operator

Algebra

Recall that a vector space in which one can multiply two vectors to obtain a third

vector is called an algebra.

this cbapter, we want to iuvestigate the algebra of

linear transformations. We have already established that the set of linear trans-

formations

L (V,W) from V to W is a vector space. Let us attempt to define a

multiplication as well. The best candidateis the composition of linear transforma-

tions.

T : V

--+

U and S : U

--+

W are linear operators, then the composition

SoT:

--+

W is also a linear operator, as can easily be verified.

This product, however, is not defined on a single vector space, bnt is such

that it takes an element in

L(V,

U) and another element in a second vector space

L(U, W) to give an element in yet another vector space

L(V,

W). An algebra

requires a single vector space. We can accomplish this by letting V

= U = W.

Then the three spacesoflineartransformationscollapsetothe single space

L (V, V),

the set of endomorphisms of V, which we abbreviate as

,c,(V)

and to which T, S,

ST sa

SoT,

andTS sa

S belong. The space ,c,(V) is the algebra of the linear

operators on V.

2.1 Algebra

'c(V)

Operator algebra encompasses various operations on, and relations among, op-

erators. One

these relations is the equality of operators, which is intuitively

obvions; nevertheless, we make it explicit in (see also Box 1.3.3) the following

defiuition.

operator

equality

2.1.1.Definition.

Two

linear

operators

T,UE

,c,(V)

areequalifT

10)

= Ula)Ior

aUla)

E V.

Because of the linearity

T and U, we have

50 2.

OPERATOR

ALGEBRA

2.1.2. Box. Two endomorphisms T, U E L(V) are equal

ifT

lai} = Ulai}

for aUla,}

E B, where B is a basis

ofV.

Therefore, an endomorphism is

uniquely determined by its action on the vectors

a basis.

The equality of operators

can

also be established by other, more convenient,

methods when an inner product is defined on the vector space. The following two

theorems contain the essence of these alternatives.

2.1.3.

Theorem.

An endomorphism T

an inner product space is 0

and only

if!

{blT la} es (bl Ta) = Ofor aUla) and

Ib).

Proof Clearly,

ifT

= 0 then {blT la} =

Conversely,

(blT la) = 0 for all ]a)

and Ib), then, choosing Ib)

=T la) =ITa), we obtain

(TaITa)=O

Via) *

Tla)=O

Via) *

T=O

by positive definiteness of the inner product.

2.1.4.

Theorem.

A linear operator T on an inner product space is 0 ifand only if

(alT la) =

or aUla}.

Proof

Obviously,

ifT

= 0, then (alT la) =

Conversely, choose a vectora la)+

fJ Ib), sandwich T between this vector and its dual, and rearrange terms to obtain

polarization

identity

what is known as the

polarization

identity:

a*fJ (alT

Ib)

+afJ*(blT la) = (aa +

fJbl

T laa +

fJb)

-lal

(alTla) -

IfJI

(blT Ib).

According to the assumption

the theorem, the RHS is zero. Thus, if we let

a = fJ = 1 we obtain (alT

Ib)

+ (hiT la) =

Similarly, with a

and fJ = i

we get i (alT

Ib)

- i (blT la) =

These two equations give (al T Ib) = 0 for all

la) , Ib). By Theorem 2.1.3, T =

To show that two operators U and T are equal, one can either have them act

on an arbitrary vectorand show that they give the same result, or one verifies that

U- Tis the zero operatorby means

one

the theorems above. Equivalently, one

shows that

(alT

Ib)

= (al U

Ib)

or (alT la) = (al U la) for all le},

Ib}.

In addition

to the zero element, which is presentin

all algebras, L(V) has an identity element,

1, which satisfies the relation1

la) = la) for all ]«) E V. With 1 in our possession,

we can ask whether it is possible to find an operator

T-

with the property that

T-

T =

= 1. Generally speaking, ouly bijective mappings have inverses.

Therefore,

only automorphisms

a vector space are invertible.

1It is convenient here to use the notation ITa) for T la). This would then allow us to write the dual

the vector as (Tal,

emphasizing that

it is indeed the bra associated with T la).