Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations
Подождите немного. Документ загружается.


14.4
THE
HYPERGEOMETRIC
FUNCTION
413
The uniqueness of the Riemann DE allows us to derive identities for solutions
and reduce the independentparameters of Equation (14.20) from five to three. We
first note that if
w(z)
is a solution of the Riemann DE corresponding to (AI, A2),
(J.'I,
J.'2),and (VI,
112),
then the function
u(z) = z},(z - I)JLw(z)
has branchpoints at z = 0,
1,00
[because
w(z)
does]; therefore, it is a solution
of
the Riemann DE. Its pairs of characteristic exponents are (see Problem 14.10)
(AI
+A,A2+A),
In particular,
if
we let A =
-AI
and J.'=-J.'1, then the pairs reduce to
(0,
J.'2
- J.'I),
Defining a sa
VI
+ Al +
J.L!,
fJ
es V2 + Al + J.'I, and y
==
I -
A2
+ AI, and using
(14.19), we can write the pairs as
(O,I-y),
(0, y - a - fJ), (a, fJ),
which yield the third version
of
the Riemann DE
w"
+
(1:'.
+
1-
Y + a + fJ)
w'
+ afJ w =
O.
z z - I
z(z
- 1)
hypergeometric
DE
This important equation is commonly written in the equivalent form
z(1 -
z)w"
+ [y - (1 + a + fJ)z]w' -
afJw
= 0
(14.21)
where
ao =
I.
and is called the
hypergeometric
differential equation (HGDE). We will study
this equation next.
14.4 The Hypergeometric Function
The two characteristic exponents of Equation (14.21) at z = 0 are 0 and I - y.
It follows from Theorem 14.2.6 that there exists an analytic solution (correspond-
ing to the characteristic exponent 0) at z
=
O.
Let
us denote this solution, the
hypergeomelric
hypergeometric function, by
F(a,
fJ;
y;
z) and write
function
00
F(a,
fJ;
y;
z) =
I>kZk
k~O
Substituting in the DE, we obtainthe recurrence relation
(a +k)(fJ +k)
ak+I
= (k +
I)(y
+k) ak
for k 2:
O.

(14.23)
414
14.
COMPLEX
ANALYSIS
OF
SOLOES
Thesecoefficientscanbe
determined
successivelyif y is
neither
zeronora
negative
hypergeometric
integer:
series
. . _
~
a(a
+
1)···
(a + k -
1)f:l(f:l
+
1)·
..
(f:l
+ k - 1) k
F(a,f:l,y,z)-l+t;r
k!y(y+1)
...
(y+k-1)
z
r(y)
~
r(a
+
k)r(f:l
+ k) k
=
r(a)r(f:l)
6
r(k
+
l)r(y
+ k) z . (14.22)
The series in (14.22) is called the
hypergeometric
series, because it is the gener-
alization of
F(l,
f:l;
f:l;
z), which is simply the geometric series.
We note immediately from (14.22) that
14.4.1. Box. The hypergeometric series becomes a polynomial ifeither a
or
f:l
is a negative integer.
Tbisis
becausefor k < lal
(ork
<
If:ll)bothr(a+k)
[orr(f:l+k)]and
I'(o)
[or r(f:l)] have poles that cancel each other. However,
r(a
+ k) [or r(f:l +
k)]
becomes finitefor k > lal (or k >
If:ll),
and the pole in
I'(o)
[or r(f:l)] makes the
denominator infinite. Therefore,
all terms of the series (14.22) beyond k = la I(or
k =
If:lll
will be zero.
Many of the properties of the hypergeometric function can be obtaineddirectly
from the HGDE, Equation (14.21). For instance, differentiating the HGDE and
letting
v = w', we
obtain
z(l
-
z)v"
+ [y + 1 - (a +
f:l
+
3)zlv'
- (a + 1)(f:l+
l)v
= 0,
which shows that
F'(a,
f:l;
y; z) =
CF(a
+ 1,
f:l
+ 1; y + 1; z), The constant C
can be determined by differentiatingEquation (14.22), setting z
= 0 in the result.'
and noting that
F(a
+ I,
f:l
+ 1; y + 1; 0) = 1. Then we obtain
af:l
F'(a,f:l;
y; z) =
-F(a
+ I,
f:l
+ 1; y + 1; z).
y
Now assume that y
'"
1, and make the substitution w =
Zl-Yu
in the HGDE
to obtain"
z(l-z)u"
+[Yl -
(al
+f:ll +
l)z]u'
-alf:llu
=0, where
al
=a
-Y
+ 1,
f:ll
=
f:l-
Y+
I,
and Yl =2 -
y.
Thus,
u =
F(a
- y + I,
f:l-
Y+ 1; 2 - y; z),
3Note
that
the
hypergeometric
function
evaluates
to 1atZ = 0
regardless
of its
parameters.
4Inthefollowingdiscussion,
aI,
Ih,andYlwill
represent
the
parameters
of thenewDEsatisfied bythenew
function
defined
in
terms
of theold.

14.4 THE
HYPERGEOMETRIC
FUNCTION
415
and u is therefore analytic at z =
D.
This leads to an interesting resnIt. Provided
that y is not an integer, the two functions
Wt(z)
==
F(a,
fJ;
y;
z),
W2(Z)
==
zt-y
F(a
- y +1,
fJ
- Y +I; 2 - y; z)
(14.24)
form a canonical basis
of
solutions to the
HGDE
at Z =
D.
This follows from
Theorem 14.2.6 and the fact that
(D,
I -
y)
are a
pair
of
(different) characteristic
exponents at
z =
D.
Johann CarlFriedrichGauss (1777-1855)wasthegreatestof
all mathematiciansandperhapsthemostrichlygifted geniusof
whom
thereis any record. He was bornin the city
of
Brunswick
in northern Germany, His exceptional skill with numbers was
clear at a very early age, and in laterlife he
joked
thathe knew
how to
count
before he could talk. It is said that Goethe wrote
anddirectedlittleplaysfora puppettheaterwhenhe was6 and
that Mozart composed his first childish minuets when he was
5, but Gauss correctedan error in his father's payroll accounts
at the age of 3. At the age of seven, when he started elemen-
tary school, his teacher was amazed when Gauss summed the
integersfrom 1 to 100instantlyby spottingthat the som was
50 pairs
of
numbers each pair summing to 1Oi.
His longprofessional life is so filled
withaccomplishments thatit is impossible to give
a full account
of
them in the short space available here. All we
can
do is simply give a
chronology
of
his almost uncountable discoveries.
1792-1794: Gauss reads the works
of
Newton, Euler, and Lagrange; discovers the prime
number
theorem (at the age
of
14 or 15); invents the method
of
least squares; conceives the
Gaussian law
of
distribution in the theory
of
probability.
1795:(only 18yearsold!) Proves
tbataregularpolygon
withn
sides is constructible(by ruler
and compass)
if
and only
if
n is the product
of
a
power
of2
and distinctprimenumbers
of
the
form
Pk = 2
2k
+1, and completely solves the 200D-year old problem
of
ruler-and-compass
construction
of
regular polygons. He also discovers the law
of
quadratic reciprocity.
1799: Proves the
fundamental
theorem
ef
algebra
in his doctoral dissertation using the
then-mysterious complex numbers with complete confidence.
1801: Gauss publishes his
Dtsquistitones
Arithmeticaein which he creates the
modem
rig-
orous approach to mathematics; predicts the exact location
of
the asteroid Ceres.
1807:Becomesprofessor
of
astronomyand the director
of
the new observatoryat Gottingen,
1809:Publisheshis secondbook, Theoria motus cor
porum
coelestium, a majortwo-volume
treatise on the motion
of
celestialbodies and the bible
of
planetary astronomers for the
next
100years.
1812: Publishes Dlsqulsitiones generales circa seriem infinitam, a rigorous treatment
of
infinite series, and introduces the
hypergeometric
function
for the first time, for whichhe
uses the notation
F(et,
fJ;
Y; z): an essay on approximate integration.
1820-1830: Publishes over 70 papers, including Disquisitiones generales circa superficies
curvas,
in which he creates the intrinsic
differential
geometry
of
general curvedsurfaces,

416 14.
COMPLEX
ANALYSIS
OF
SOLOES
theforerunnerofRiemanniangeometryandthegeneraltheoryofrelativity. Fromthe
18308
on, Gauss was increasingly occupied with physics, and he enriched every branch
of
the
subjecthe touched.
In
the theory
of
surface
tension,
he developed the fundamental
idea
of
conservation
of
energy and solved the earliestproblemin the
calculus
of
variations.
In
op-
tics,he introducedtheconceptof thefocallengthof a systemoflenses.Hevirtuallycreated
the science
of
geomagnetism,
and in collaboration
with
his friend and colleague Wilhelm
Weberhe inventedthe electromagnetic telegraph. In 1839 Gauss publishedhis fundamental
paperon the generaltheory
of
inverse squareforces,
which
established
potential
theory
as
a coherent branch
of
mathematics and in which he establishedthe
divergence
theorem.
Gauss
had
many opportunities to leave Gottingen, but he refused all offers and remained
there for the rest
of
his life, living quietly and simply, traveling rarely, and working with
immense energy on a wide variety
of
problems in mathematics and its applications. Apart
from science
andhis
family-he
marriedtwice and had six children, two
of
whom
emigrated
to
America-his
main
interests werehistory and worldliterature, internationalpolitics, and
publicfinance. He owned
alarge
libraryof about6000volumesin manylanguages,including
Greek, Latin, English, French, Russian, Danish, and
of
course German. His acuteness in
handlinghis own financial affairs is shownby the fact thatalthoughhe started
with
virtually
nothing, he left an estate over a hundred times as great as his average annual income
during the last
half
of
his life. The foregoing list is the published portion
of
Gauss's total
achievement; the unpublished and private part is almost equally impressive. His scientific
diary, a little booklet of 19 pages, discovered in 1898, extends from 1796 to 1814 and
consists
of
146 very concise statements of the results
of
his investigations, which often
occupied
him
for weeks or months.
These
ideas were so abundant and so frequent that he
physically did not have time to publish them.
Some
of
the ideas recorded in this diary:
Cauchy
Integral
Formula:
Gauss discovers it
in
1811, 16 years before Cauchy.
Non-Euclidean
Geometry:
After failing to prove
Euclid's
fifth postulate at the age
of
15,
Gauss came to the conclusion that the Euclidean form
of
geometry cannot be the only
one
possible.
Elliptic
Functions:
Gauss had found many
of
the results
of
Abeland Jacobi (the two
main
contributors to the subject) before these
men
were born. The facts became known partly
throughJacobihimself. His attention was caught by a cryptic passagein the
Disquisitiones,
whosemeaning
can
only be understood if one knows something aboutelliptic functions. He
visited Gauss on several occasions to verify his suspicions and tell
him
abouthis own
most
recentdiscoveries, and each time Gausspulled 30-year-oldmanuscripts out
of
his deskand
showed Jacobi
what
Jacobi had
just
shown him. After a week's visit with Gauss in 1840,
Jacobi wrote to his brother, "Mathematics would be in a very different position
if
practical
astronomy had not diverted
thiscolossal genius from his glorious career."
A possible explanation for not publishing such important ideas is suggested by
his
comments in a letter to
Bolyai:
"It
is not knowledge but the act
of
learning, not possession
but the act of getting there, which grants the greatest enjoyment.
When
I have clarified and
exhausted a subject, then I
turn away from it in order to go into darkness again." His was
the temperament
of
an explorer
who
is reluctant to take the time to write an account
of
his
last expedition
when
he could be starting another. As it was, Gauss wrote a great deal, but
to have published every fundamental discovery he
made
in a form satisfactory to
himself
would have required several long lifetimes.

14.4
THE
HYPERGEOMETRIC
FUNCTION
417
A thirdrelationcan be obtainedby makingthe snbstitntionw =
(I-z)y-a-
p
u.
Tltis leads to a hypergeometric equation for u with
al
=Y - a, fh = Y - fJ, and
Yl
=
y.
Furthermore, w is analytic at z = 0, and w(O) =
I.
We couclude that
w =
F(a,
fJ; Y; z), We therefore have the ideutity
F(a,
fJ; Y; z) = (I -
z)y-a-
p
F(y
- a, Y - fJ; Y; z), (14.25)
To obtainthe canonicalbasis at z =
I,
we makethe substitution t =
1-
z, and
notethattheresultisagaintheHGDE,withal
=
a,fJI
=fJ,andYI =
a+fJ-y+1.
It
follows from Equatiou (14.24) that
W3(Z) sa
F(a,
fJ; a +fJ - Y +
I;
1-
z),
W4(Z)
==
(I
-
z)y-a-
p
F(y
- fJ, Y - a; Y - a - fJ+
I;
I - z)
(14.26)
form a canonical basis
of
solutious to the
HGDE
at z = I.
A symmetry
of
the hypergeometric functiou that is easily obtained from the
HGDEis
F(a,
fJ; Y; z) = F(fJ, a; Y; z).
The six functions
(14.27)
F(a
± I, fJ; Y; z),
F(a,
fJ ±
I;
Y; z),
F(a,
fJ; Y ±
I;
z)
(14.28)
(14.29)
are called hypergeometric functions
contiguous
to
F(a,
fJ; Y; z),
The
discussiou
above showed how to obtain the basis
of
solutions at z = I from the regular
solutiou to the HDE
z = 0,
Fta,
fJ; Y; z).We
can
show that the basis
of
solutious
at
z =
00
can also be obtained from the hypergeometric fuuctiou.
Equatiou (14.16) suggests a function
of
the form
v(z) =
z'
F
(ai,
fJI; Yl; Dsa
z'w
G)
=}
w(z) =
z'v
G),
where r,
ai,
fJl' and Ylare to be determined. Since w(z) is a solution
of
the HGDE,
v will satisfy the following DE (see Problem 14.15);
z(l-
z)v"
+[I - a - fJ
-2r
- (2 - Y -
2r)z]v'
-
[r
2
-
r +
ry
-
~(r
+
a)(r
+fJ)] v =
O.
This reduces to the HGDE
if
r =
-a
or
r =
-fJ.
For r =
-a,
the parameters
become
al
=a, fJl = I
+a
- Y, and
YI
=a - fJ+
I.
For r =
-fJ,
the parameters
are
al
= fJ, fJI = I +fJ - Y, and Yl =fJ - a +I. Thus,
VI(Z) =
z-a
F
(a,
I +a - Y; a - fJ +
I;
~)
,
V2(Z) =
z-p
F (fJ, I +fJ - Y; fJ - a +
I;
D (14.30)

(14.31)
418
14.
COMPLEX
ANALYSIS
OF
SOLDES
form a canonical basis of solutions for the
HGDE
that are valid about z =
00.
As the preceding discussion suggests, it is possible to obtain many relations
among the hypergeomettic functions with different parameters and independent
variables. In fact, the nineteenth-centurymathematicianKummershowed that there
are 24 different (but linearly dependent, of course) solutions to the HGDE. These
Kummer's
solutions
are collectively known asKummer's solutions,
and
six of them were
derived
above. Another important relation (shown in Problem 14.16) is that
z·-y
(1 -
z)Y-.-p
F (Y - a, I - a; I - a +
fJ;
~)
also solves the HGDE.
Many of the functions that occur in mathematical physics are related to the
hypergeomettic function. Even some
of
the common elementary functions can be
expressed in terms of the hypergeometric function with appropriate parameters.
For example, when
fJ
=
y,
we obtain
. . _
~
r(a
+k) k _
_.
Fta,
fJ, fJ,
z) -
f;;o
r(a)r(k
+ I) z -
(I
- z) .
Similarly,
F(~,
~;
~;
z2) =
sin-
1
zl
z, and
F(I,
I; 2;
-z)
= In(1 +
z)lz.
How-
ever, the real power of the hypergeomettic function is that it encompasses almost
all
of
the nonelementary functions encountered in physics. Let us look briefly at a
Jacobi
functions
few of these.
Jacobi
functions are solutions of the DE
2 d
2u
du
(I
- x
)-2
+
[fJ
- a - (a +
fJ
+
2)x]-
+ ).(). + a +fJ +
I)u
= 0
dx dx
(14.32)
Defining x = I - 2z changes this DE into the
HGDE
with parameters
al
=
).,
fJl
=
).
+ a +
fJ
+ I, and Yl = I + a. The solutions of Equation (14.32), called
the
Jacobi
functions
of
the
first
kind,
are, with appropriate normalization,
c.
R)
r().
+a +1) ( I -
Z)
P
op
(z) = F
-).
).+ a +
fJ
+
I'
I +
a'
--
.
A
r().
+
l)r(a
+
I)'
" 2
When).
= n, anonnegativeinteger, the Jacobifunction turns into a polynomial
of degree
n with the following expansion:
pC
a,PJ(z) =
r(n+a+l)
~r(n+a+fJ+k+I).(Z-I)k
n
r(n
+
I)r(n
+ a +
fJ
+ I)
f;;o
I'(o
+ k +
I)
2
Theseare the Jacobipolynomialsdiscussedin Chapter7.
Infact, the DE satisfied by
pJ.,PJ
(x)
ofChapter7
is identicalto Equation(14.32). Note that the transformation
x = I - 2z translates the points z = 0 and z = 1 to the points x = I and x =
-I,
respectively. Thus the regular singular points of the Jacobi functions of the first
kind are at
±1
and
00.
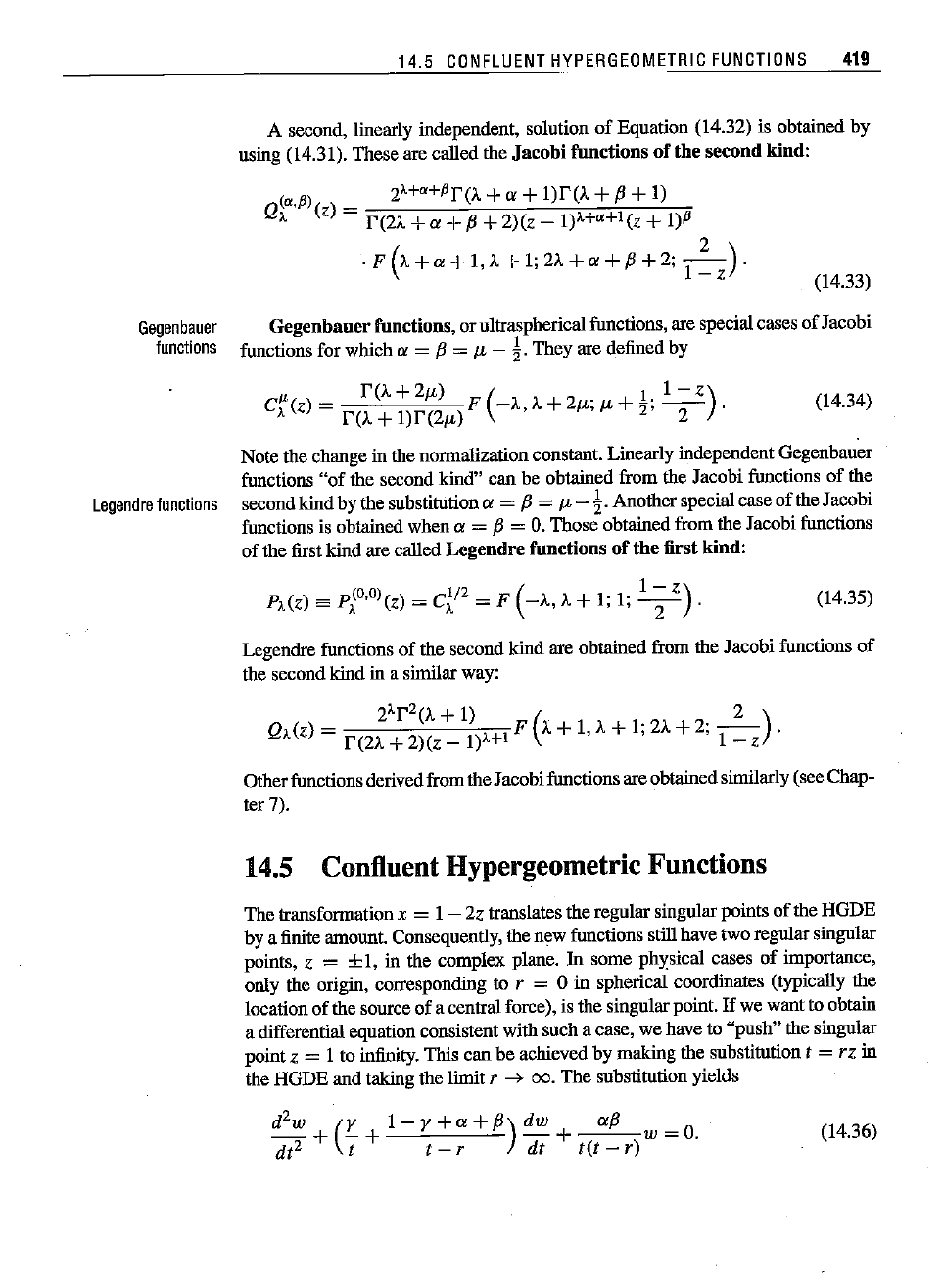
14.5
CONFLUENT
HYPERGEOMETRIC
FUNCTIONS
419
A second, linearly independent, solution
of
Equation (14.32) is obtained by
using (14.31).These are called the
Jacobi
functions
of
the
second kiud:
lO,P)
_ 2,,+o+Pr(A +
ex
+ 1)r(A +
fJ
+ I)
Q,
(z) - r(2A +
ex
+
fJ
+ 2)(z - 1),,+o+l(z + I)P
. F (A+ex +
I,A
+ 1;
2A
+ex + fJ
+2;
_2_).
1-z
(14.33)
(14.34)
(14.35)
(14.36)
Gegenbauer
Gegeubauer
functions, orultrasphericalfunctions, are special cases ofJacobi
functions
functions for which
ex
=
fJ
= /-' -
~.
They are defined by
/L _
r(A+2/-,)
( . 1.
1-Z)
c, (z) -
rCA
+ 1)r(2/-,) F
-A,
A+
2/-"
/-'+
2'
-2-
.
Note the change in the normalization constant. Linearly independent Gegenbauer
functions
"of
the second kind" can be obtained from the Jacobi functions of the
Legendre
functions
second kind by the substitution
ex
=
fJ
=
/-'
-~.
Anotherspecial case of the Jacobi
functions is obtained when
ex
=
fJ
=
O.
Those obtainedfrom the Jacobi functions
of the first kind are called
Legendre
functions
of
the
first kind:
_ (0,0)
1/2
( . . 1 -
Z)
F,(z)
=
F,
(z) = c, = F
-A,
A+1, 1,
-2-
.
Legendre fuuctions of the second kind are obtained from the Jacobi functions
of
the second kind in a similar way:
2'r
2(A
+ 1) ( 2 )
Q,(z)
= r(2A + 2)(z _ 1)"+1F A+ 1, A+ 1; 2A+ 2; 1 _ z .
Otherfunctions derived from the Jacobifunctions are obtainedsimilarly (see Chap-
ter7).
14.5 ConfluentHypergeometric Functions
The transformation x = 1- 2ztranslates the regular singular points
of
the HGDE
by a finite amount. Consequently, the new functions still have two regular singular
points,
z =
±I,
in the complex plane.
In
some physical cases of importance,
only the origin, corresponding to r = 0 in spherical coordinates (typically the
location of the source of a centralforce), is the singularpoint.
If
we want to obtain
a differential equation consistent with such a case, we have to "push" the singular
point
z = 1 to infinity. This can be achieved by making the substitution t = rz in
the HGDE and taking the limit
r -->
00.
The
substitution yields
d
2w
(Y
I - Y +
ex
+
fJ)
dw
exfJ
0
dt
2
+ t + t - r
at
+
t(t
_ r) w = .
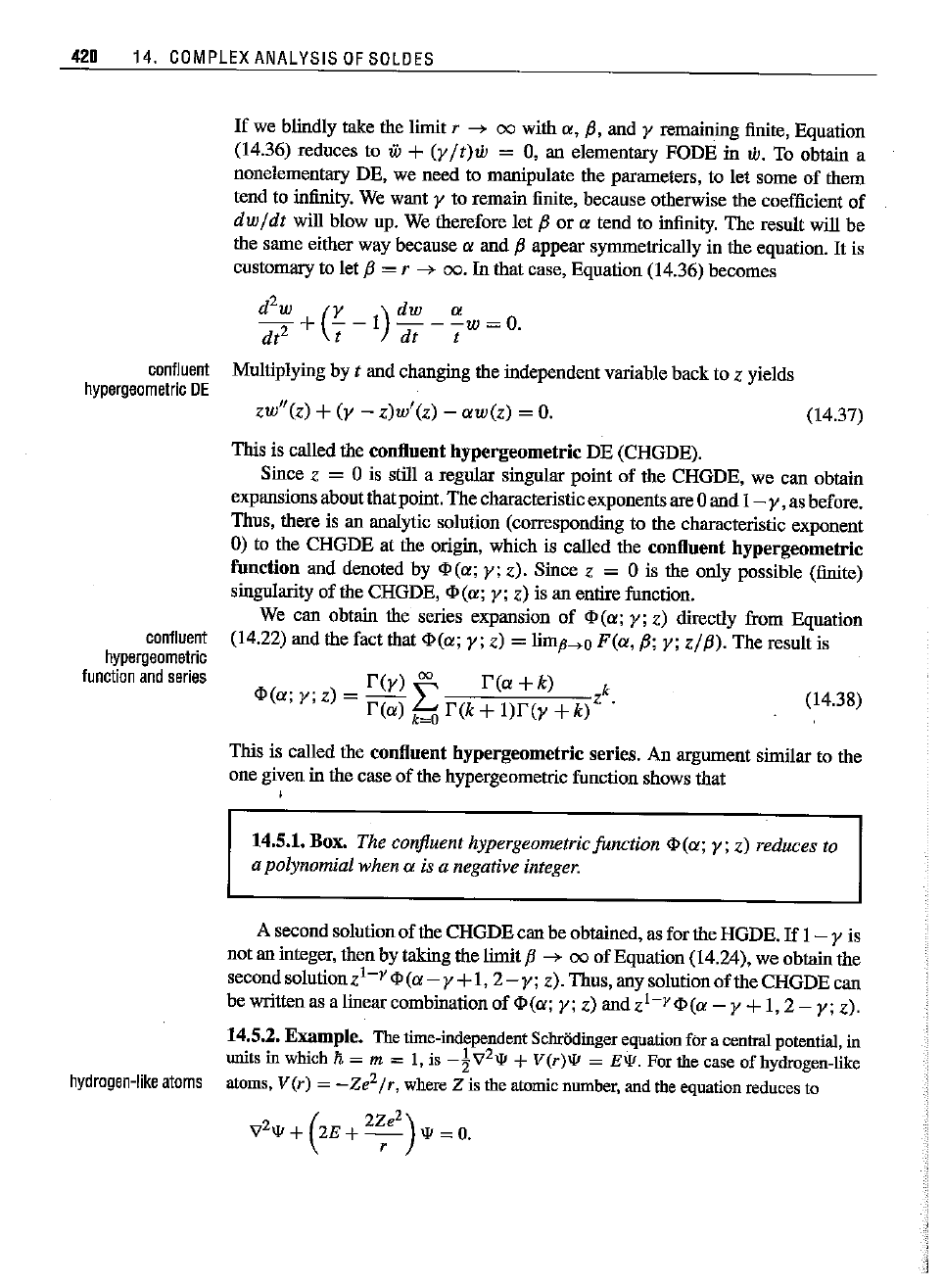
420 14.
COMPLEX
ANALYSIS
OF
SOLOES
(14.38)
confluent
hypergeomelric
OE
confluent
hypergeometric
function
and
series
If
we blindly take the limit r --+
00
with
a,
fJ,
and )I remaining finite, Equation
(14.36) reduces to
w+
()I/t)th
= 0, an elementary FODE in th. To obtain a
nonelementary DE, we need to manipulate the parameters, to let some of them
tend to infinity. We want
)I
to remain finite, because otherwise the coefficient of
dw/dt
will blow up. We therefore let
fJ
or a tend to infinity. The result will be
the same either way because a and
fJ
appear symmetrically in the equation.
It
is
customary to let
fJ
=r --+
00.
In that case, Equation (14.36) becomes
d
2w
+
(l'.
_
I)
dw
_
':W
=
O.
dt
2
t
dt
t
Multiplying by t and changing the independent variable back to Z yields
zw"
(z) +
()I
-
z)w'
(z) -
OIW(Z)
=
O.
(14.37)
This is called the confluent
hypergeometric
DE (CHGDE).
Since Z = 0 is still a regular singular point of the CHGDE, we can obtain
expansions about thatpoint. The characteristicexponentsare
0 and
1-)1,
as before.
Thus, there is an analytic solution (corresponding to the characteristic exponent
0) to the CHGDE at the origin, which is called the confluent
hypergeomelric
function and denoted by
<p(0I;
)I;
z). Since z = 0 is the ouly possible (finite)
singularity of the CHGDE,
<P(OI;
)I;
z) is an entire function.
We can obtain the series expansion of
<P(OI;
)I;
z) directly from Equation
(14.22) and the fact that
<p(0I;
)I; z) = limp-+o
F(OI,
fJ;
)I; z/
fJ).
The result is
<P
a.
. _
r()I)
f'...
r(OI +k) k
(
,)I,z)
-
I'(c)
f;;Q
r(k+
1)r()I
+k)z
.
This is called the conflnent
hypergeometric
series. An argument similar to the
one given in the case
of
the hypergeometric function shows that
,
14.5.1. Box. The confluent hypergeometricfunction <P(a;)I; z) reduces to
a polynomial when a is a negative integer.
A second solutionof the CHGDEcan be obtained, as for the HGDE.
If
1
-)I
is
not an integer, then by taking the limit
fJ
--+
00
of Equation (14.24), we obtain the
second solution
Zl-Y
<P
(a
-)I
+
I,
2
-)I;
z). Thus, any solution
of
the CHGDEcan
be written as a linear combination of
<p(0I;
)I; z) and
zl-y
<P(a
-)I
+
I,
2
-)I;
z),
14.5.2. Example. Thetime-independent Schrodinger equation for acentralpotential, in
unitsin whichIi = m = I, is -!V
2
1jf +
V(r)1jf
= E1jf. Forthe case of hydrogen-like
hydrogen-like
atoms
atoms,
V(r)
=
-Z;'
[r,
where Z is theatomic number, andtheequation reducesto
(
2Ze2)
V
2
1jf + 2E +
-r-
1jf =O.

(14.39)
quantization
of
the
energy
of
the
hydrogen
atom
14.5
CONFLUENT
HYPERGEOMETRIC
FUNCTIONS
421
The radial part of this equation is given by Eqnation (12.14)with
fer)
=
2E
+2Ze21r.
Definingu =
rR(r),
we maywrite
e, ( a
b)
- + A+- - - u =0,
dr
2
r r
2
where A = 2E, a =
2Ze
2,
and b =
1(1
+ I). This equation can be further simplified by
defining
r
==
kz (k is an
arbitrary
constant
tobe
determined
later):
d
2u
( 2 ak
b)
- + Ak +- - - u =
O.
dz
2
Z z2
Choosing Ak
2
=-! and introducing a
==
al(2H)
yields
d2u+(_!+~_~)u=0.
dz
2
4zz
2
Equations
ofthisform canbe
transformed
intothe
CHGDE
by
making
the
substitution
u(z) =
z"e-
vz
f(z).1t
theu follows that
d
2f
+(2fJ.
_2v)df
+[_!+fJ.(fJ.-
I
)
_2fJ.v
+~-~+v2]f=0.
dz
2
z dz 4 z2
zzz2
Cboosing v
2
= !and
fJ.(fJ.
- I) =b reduces this equation to
I" +
c:
-
2v)
r -
2fJ.
V
z
- a f
= 0,
which is in the form of
(14.37).
Onphysical
grounds,
we expectu(z) --+ 0 as z --+
00.
5
Therefore,
v=
!.
Similarly,
with
fJ.(fJ.
- I) = b =
1(1
+
I),
we obtain the two possibilities
fJ.
=
-I
and
fJ.
= I +
I.
Againonphysical
grounds,
we
demand
that
u(O)be
finite
(thewave
function
must
not
blow
up at r = 0). This implies'' that
fJ.
= I +
I.
We thus obtain
fff +
[2(1;
I)
_ I] t' _ I +
~
- a f =
O.
Multiplying by z gives
zf"
+
[2(1
+ I) - zlr" - (I + I -
a)f
=
O.
Comparing this with
Equation
(14.37)
shows that f is proportional to
cf>(I
+
I-a,
21
+2;
z). Thus, the solution
of
(14.39)
can be written as
u(z) =Cz
1
+
te-
z/
2
cf>
(1+
1-
a,
21
+2; z).
An
argument similar to that used in Problem 13.20 will reveal that the product
e-
z/
2
cf>
(I+
I-a,
21+2; z) willbeinfiniteunless thepower seriesrepresenting
cf>
terminates
(becomes a polynomial).
It
follows from Box
14.5.1
that this will take place
if
I +
I-a
=-N
(14.40)
5Thisis becausethevolume
integral
of
1\111
2
overallspacemustbe
finite.
The
radial
part
ofthis
integral
is simplythe
integral
of r
2
R2(r)
= u
2(r).
This
latter
integral
will notbe
finite
unlessu(oo) =
o.
6Recall
that
JL
is the
exponent
of z =r/ k.

422 14.
COMPLEX
ANALYSIS
OF
SOLOES
for some integerN
::::
O.
In that case we obtain the Laguerre polynomials
L
j
=
r(N+j+l)
<1>(-N'
I')
N -
I'(N
+
I)r(j
+
I)
,J
+ ,z ,
where j = 21+
I.
Condition (14.40) is the quantization rule for the energy levels of a hydrogen-like
atom. Writing everything in
tenus
of
the original parameters and defining n = N +I + 1
yields-after
restoring all the
m's
and the
!i's-the
energy levels
of
a hydrogen-like atom:
E = _
Z2
me4
=
_Z2
(me
2
)
a2~,
21i
2
n
2
2 n
2
where a =
eO
1(1ic) = 1/137 is the fine
structure
constant.
The
radial wave functions can now be written as
R
n
I(T) = Un,I(T) = CTle-bj(naO)<1>
(-n
+1+1,21 +2;
2ZT)
,
, r naO
where
an
= 1i
2
/ (me
2)
= 0.529 x
10-
8
em is the
Bohr
radius.
III
Friedrich
Wilhelm
Bessel (1784-1846) showed no signs
of
unusual academic ability in school, although he did show a
liking. for mathematics and physics. He left school intending
to become a merchant's apprentice, a desire that soon mate-
rialized with a seven-year unpaid apprenticeship
with
a large
mercantile
finn in Bremen. The young Bessel proved so adept
at accountingand calculationthat he was grantedasmallsalary,
with raises, afteronly the first year.
An interestin foreign trade
led
Besselto studygeographyaod laoguagesat uight,astonish-
ingly learning to read and write English in only three months.
He also studiednavigationin orderto qualify as a cargo officer
aboard ship, but his innate curiosity
soon
compelledhim to investigate astronomy at a more
fundamentalleveI. Still serving his apprenticeship, Bessel learned to observe the positions
of
stars with sufficientaccuracy to determine the longitude
of
Bremen, checkinghis results
against professional astronomical journals. He then tackled the more formidable problem
of
determining the orbit
of
Halley's comet from published observations. After seeing the
close agreementbetweenBessel'scalculations and those
of
HaIley, the
German
astronomer
Olbers encouragedBessel to improve his already impressive work
with
more observations.
The improved calculations, an achievement tantamount to a
modem
doctoral dissertation,
were published with Olbers's recommendation. Bessel later received appointments with
increasing authority at observatories
near
Bremen and in Konigsberg, the latter position
being accompanied by a professorship. (The title
of
doctor, required for the professorship,
was granted by the University
of
Gottingen on the recommendation
of
Gauss.)
Besselprovedhimselfan excellentobservationalastronomer. His careful measurements
coupled
with
his mathematical aptitude allowed
him
to produce accurate positions for a
number
of
previously mapped stars, taking account
of
instrumental effects, atmospheric
refraction, and the position and motion
of
the observation site.
In
1820 he determined the
position
of
the vernal equinox accurate to 0.01 second, in agreement
with
modern values.
