Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations
Подождите немного. Документ загружается.


(14.4)
14.1
ANALYTIC
PROPERTIES
OF
COMPLEX
DES
403
14.1.2 The Circuit Matrix
The method used in Example 14.1.1 can be generalized to obtain a similar result
for the NOLDE
dnw
dn-1w
dw
L[w] =
dz
n
+
Pn-I(Z)
dz
n-
I
+...+PI(Z)
dz
+
po(z)w
= 0
where all the Pi(Z) are analytic in rl < [z - zol <
ri-
Let {w
j(z)}J~1
be a basis
of
solutions
of
Equation(14.4),
andletz-zo
=
re'",
Start at z and analyticallycontinuethe functions W j (z) one completetum toe+
2IT
.
Let
W
j(z)
'"
Wj (eo+re
ie
)
es Wj (zo +rei(9+2"J). Then, by a generalization of
Proposition 14.0.1, {Wj(z)}J=1 are not ouly solutions, but they are linearly inde-
pendent (because they are
W
j'S
evaluated at a differentpoint). Therefore, they also
form abasis
of
solutions. Onthe otherhand, Wj (z) can be expressedasalinearcom-
binationoftheWj (z). Thus,
Wj(z)
=Wj (zo +re
i(9+2"»)
=
Lk=1
ajkwk(Z).The
circuit
matrix
matrix A = (a
jk),
called the
circnit
matrix
of
the NOLDE, is invertible, because
it transforms one basis into another. Therefore, it has ouly nonzero eigenvalues.
We let
Abe one such eigenvalue, and choose the column vector C, with entries
{c,
}7~1'
to be the corresponding eigenvector
of
the transpose of A (note that A
and At, have the same set
of
eigenvalues). At least one such eigenvector always
exists, because the characteristic polynomial
of
At has at least one root. Now we
let
w(z)
=
LJ=I
CjW
j(z).
Clearly, this
w(z)
is a solution
of
(14.4), and
n
w(z)
'"
w(zo
+rei (9+2>t
J
)
=
:~::>jWj(zo
+re
i(9+2>t
J)
j=l
n n n
=
LCj
LajkWk(z)
=
L(At)kjCjWk(Z)
=
LACkWk(Z)
= AW(Z).
j=l
k=l
j,k
k=l
If
we define O! by A=
e2>tia,
then w(zo +
re
i(9+2>tJ)
=e
2"ia
w(z).
Now we write
f(z)
ea (z -
zo)-aw(z).
Following the argument used in Example 14.1.1, we get
f(zo
+
re
i(8+2"»)
=
f(z);
that is,
f(z)
is single-valued around
ZOo
We thus have
the following theorem.
14.1.2.
Theorem.
Any
homogeneous
NOWE
with analytic coefficientfunctions
in
ri < [z-
zo
1 <
rz
admits a solution
of
the
form
W(z) = (z -
zo)"
f(z)
where
f(z)
is single-valuedaround zo in rl < lz- eo! <
rz-
An isolated singular point zo near which an analytic function
w(z)
can be
written as
w(z)
= (z - zo)"
f(z),
where
f(z)
is single-valued and analytic in
the punctured neighborhood
of
zo. is called a simple
branch
point
of
w(z).
The
arguments leading to Theorem
14.1.2 imply that a solution with a simple branch
404 14.
COMPLEX
ANALYSIS
OF
SOLDES
point exists if and only
if
the vector C whose components appear in
w(z)
is an
eigenvector of
At, the transpose of the circuit matrix. Thus, there are as many
solutions with simple branch points as there are linearly independent eigenvectors
of
At
14.2 ComplexSOLDEs
canonical
basis
of
the
SOLOE
Let us now consider the SOLDE ui" +
p(z)w
t
;t-q
(z)w
=
O.
Given two linearly
independent solutions
WI (z) and Wz(z), we form the2 x 2 matrix A and
try
to
diagonalize it. There are three possible outcomes:
1. The matrix A is diagonalizable, and we can find two eigenvectors,
F(z)
and
G(z),
corresponding, respectively, to two distinct eigenvalues, Al and
AZ.This means that
F(zo
+rei(O+z,,)) = Al
F(z)
and
G(zo
+rei(O+h)) =
AZG(Z). Defining Al = e
h i"
andAz = e
h iP,
we get
F(z)
= (z - zo)"
fez)
andG(z)
=
(z-zo)P
g(z),asTheoremI4.1.2suggests.
The set
{F(z),
G(z)}
is called a canonical basis
of
the SOLDE.
2. The matrix Ais diagonalizable, and the two eigenvalues are the same.
Inthis
case both
F(z)
and
G(z)
have the same constant
ex:
F(z)
= (z - zo)"
fez)
and
G(z)
= (z- zo)"
g(z).
3. We cannot find two eigenvectors. This corresponds to the case where A
is not diagonalizable. However, we can always find one eigenvector, so
A has only one eigenvalue,
A.
We let WI (z) he the solution of the form
(z -
zo)"
fez),
where
fez)
is single-valued and A = eZ"i". The existence
of such a solution is guaranteed by Theorem 14.1.2. Let
wz(z)
be any other
linearly independent solution (Theorem 13.3.5 ensures the existence of such
a second solution). Then
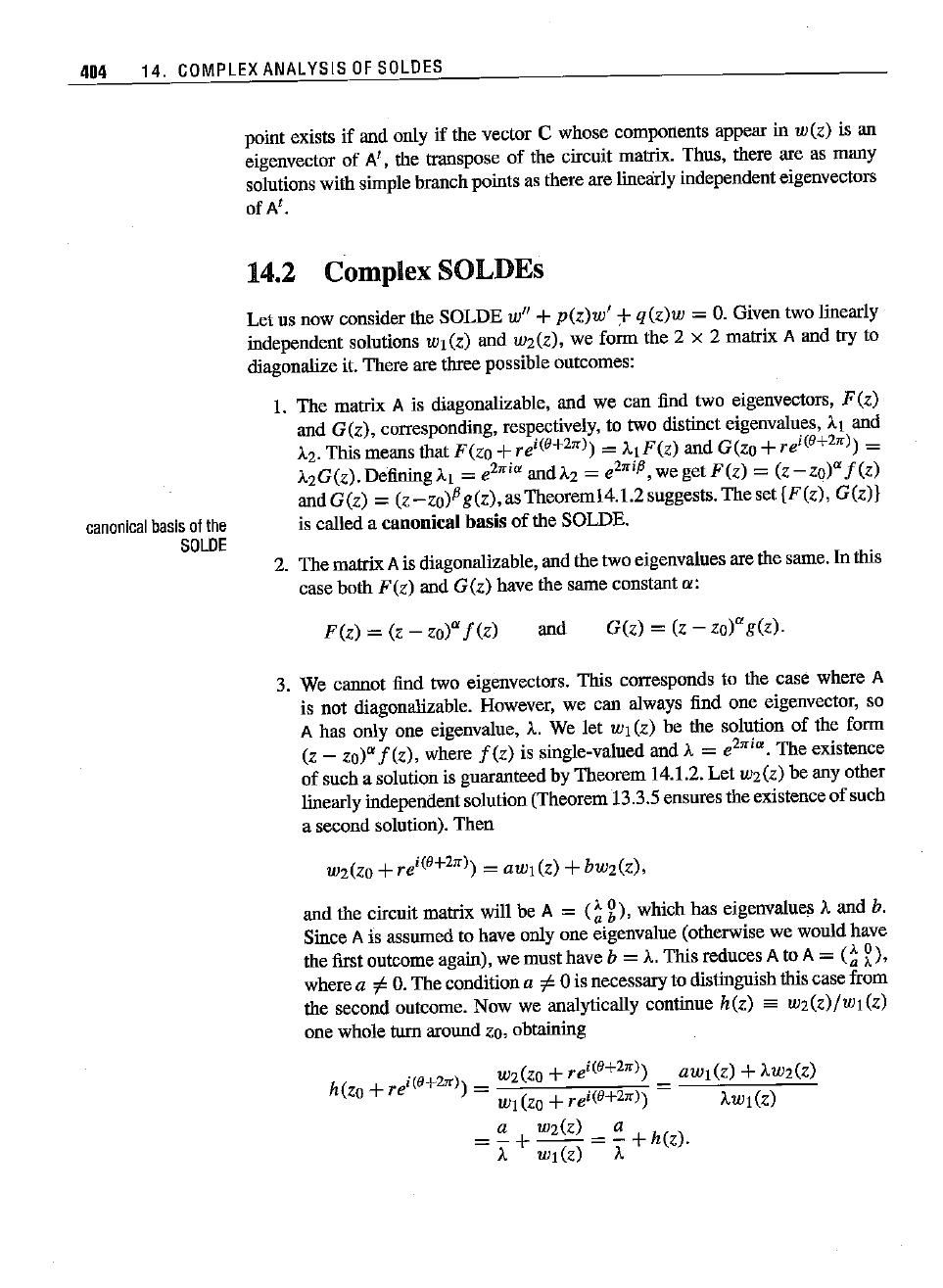
and the circuit matrix will be A
=
(~g),
which has eigenvalues Aand b.
Since Ais assumed to have only one eigenvalue (otherwise we would have
the first outcome again), we must have
b =
A.
This reduces Ato A=
(~
~),
where a i"
O.
The condition a i" 0 is necessaryto distinguishthis case from
the second outcome. Now we analytically continue
h(z)
sa wZ(Z)/WI(Z)
one whole
tum
around zo, obtaining

14.2
COMPLEX
SOLOES
405
It
then follows that the functionI
a
gl
(z)
==
h(z)
-
2:n:iJ..
In(z - zo)
is single-valued in TJ < [z - zn] <
rz-
If
we redefine
gl
(z) and
Wz
(z) as
(2:n:iJ..ja)gl (z) and (2:n:iJ..ja)wz(z), respectively, we have the following:
14.2.1.
Theorem.
If
p(z)
and
q(z)
are analytic in the annular region rl < [z -
zol
< rz. then the
SOWE
w" +
p(z)w'
+
q(z)w
= 0 admits a basis
of
solutions
{WI,
wz}
in the neighborhood
of
the singular
point
zo.
where either
WI(Z)
= (z - zn)"
fez),
or, in exceptional cases (when the circuit matrix is not diagonalizable],
WI (z) = (z- zo)"
fez),
Thefunctions f (z).
g(z),
and
gl
(z) are analytic and single-valuedin the annular
region.
This theorem allows us to factor out the branch point zo from the rest of the
solutions. However, even though
fez),
g(z),
and
gl
(z) are analytic in the annular
region
rl <
Iz
- zoI<
r2,
they may very well have poles
of
arbitrary orders at
zoo
Can we also factor out the poles?
In
general, we cannot; however, under special
circumstances, described in the following definition, we can.
14.2.2. Definition.
ASOWEoftheform
w"+p(z)w'+q(z)w
= othat isanalytic
regular
singular
point
in 0 < [z-
zol
< r has a regularsingular
point
at zo if
p(z)
has at worsta simple
ofa
SOLDE
defined
pole and
q(z)
has at worst a pole
of
order 2 there.
In a neighborhood of a regular singularpointzo, the coefficient functions
p(z)
and q (z) have the puwer-series expansions
00
a-I
'"
k
p(z)
=
--
+L..., ak(Z -
zo)
,
z
-zo
k~O
b-z
b_1
~
k
q(z)
= ( )Z +
--
+L...,bk(z - zo) .
z-zo z-zo
k~O
Multiplying both sides of the first equation by z - zo and the second by (z - zo)z
and introducing
P(z)
sa (z -
zo)p(z),
Q(z)
es (z - zo)Zq(z), we obtain
00
P(z)
=
Lak-I(Z
-
ZO)k,
k=O
1Recall
that
In(z - zo)
increases
by21ri foreach
turn
around
zoo
00
Q(z)
=
Lbk-z(z
- zo)k.
k~O

406 14.
COMPLEX
ANALYSIS
OF
SOLDES
It
is also convenient to multiply the
SOLDE
by (z -
ZO)2
and write it as
(z -
ZO)2
w" +(z -
zo)P(z)w'
+
Q(z)w
=
o.
Inspired by the discussion leading to Theorem 14.2.1, we write
(14.5)
00
w(z)
= (z -
zo)"
LCk(Z -
ZO)k,
k=O
Co =
I,
(14.6)
where we have chosen the arbitrary multiplicative constant in such a way that
Co
= 1. Substitute this in Equation (14.5),
and
change the
dummy
variable-s-so
that
all sums start at
O-to
obtain
~
{ (n +
v)(n
+v -
I)C
n
+
~[(k
+
v)an-k-I
+b
n-
k-
2]Ck
}
. (z - zo)"+v = 0,
which
results
inthe
recursion
relation
For n = 0, this leads to
what
is known as the
indicial
equation
for the exponent
v:
indicial
equation,
indicial
polynomial,
characteristic
exponents
n
(n +
v)(n
+v -
I)C
n
= -
L[(k
+v)an_k_1 +b
n-k-2]Ck·
k=O
ltv)
ss
v(v
-I)
+a_Iv
+b_2 =
O.
(14.7)
(14.8)
The roots
of
this equationare calledthe
characteristic
exponents
of
zo,
and I (v) is
calledits
indicial
polynomial.
In
terms
of
this polynomial, (14.7) can be expressed
as
n-I
I(n
+
v)C
n
= -
L[(k
+
v)an-k-I
+b
n-k-2]Ck
k=O
forn
=
1,2,
....
(14.9)
Equation (14.8) determines
what
values
of
v are possible,
and
Equation (14.9)
gives
CI,
C2, C3,
...
, which in turn determine w(z). Special care
must
be taken
if the indicial polynomial vanishes at
n +v for
some
positive integer n, that is,
if
n +v, in addition to v, is a root
of
the indicial polynomial: I (n +v) = 0 = I
(v).
If
uj and
V2
are characteristic exponents
of
the indicialequation and
Re(vI)
>
Re(
V2),then a solutionfor VI alwaysexists. A solutionfor
V2
also existsif VI- v2 #
n for any (positive) integer n. In particular, if Zo is an ordinary
point
[a point at
which
both
p(z)
and
q(z)
are analytic], then only one solution is determined by
(14.9). (Why?) The foregoing discussion is summarized in
thefollowing:

14.2
COMPLEX SOLDES 407
14.2.3.
Theorem.
If
the dif.ferential equation w" +
p(z)w'
+
q(z)w
= 0 has a
regular singular point at
Z = zo, then at least one power series
of
the form
of
(14.6) formally solves the equation.
If
VI
and
V2
are the characteristic exponents
of
zo.
then there are two linearly independent formal solutions unless VI -
V2
is
an
integer.
14.2.4.
Example.
Let us
consider
some
familiar
differential
equations.
(a)TheBessel
equation
is
" I , ( a
2
)
w +
-w
+
1-
- w·=
O.
Z z2
In thiscase, theoriginis a
regular
singular
point,
a-I
= I, and
b-z
=
_a
2.
Thus,
the
indicia!
equation
is v(v - 1) + v - a
2
= 0, andits solutions are vI = a and
112
=
-a.
Therefore,
there
are
two
linearly
independent
solutions to theBessel
equation
unless
VI
- v2 =2a is an
integer,
i.e., unlessa is eitheranintegerora half-integer.
(b) For the
Coulomb
potential f
(r)
= f3I
r,
the most generalradial eqoation [Equation
(12.14)] reducesto
"
2,
(fJ
a)
w +
-w
+ - - - w =
O.
z z z2
Thepointz = 0 is a
regular
singular pointatwhich
a-I
= 2 and
b-z
=
-ct.
The
indicia!
polynomialis lev) = v
2
+v
-a
with
characteristic
exponents
vi = -i+
iJl
+4a and
V2
= -! -
!oJI
+4a. Thereare two independentsolutionsunless
vI
-
VZ
= oJI +4a
is an
integer.
In
practice,
a =
1(1
+ I),
where
I is some
integer;
so VI ""
vz
=
21
+ I, and
onlyone solution is
obtained.
(c)Thehypergeometric
differential
equation
is
w" + y - (a +
fJ
+I)z w' _
~w
=
O.
z(1 - z) z(1 - z)
A
substantial
nwnberof functions in
mathematical
physicsaresolutions of this
remarkable
equation,
with
appropriate
valuesfor
a,
fJ,
andy. The
regular
singular
points- arez = 0and
z = 1.
Atz
= O,a_1 = y andb_2 =
O.
Theindicialpolynomialis I(v) =
v(v
+y
-I),
whose roots
are
VI
= 0 andV2 = 1 -
y.
Unless y is an
integer,
we havetwo formal
solutions.
..
It
is shown in differential equation theory [Birk 78, pp. 40-242] that as long
as
VI
- V2 is not an integer, the series solution
of
Theorem 14.2.3 is convergent
for a neighborhood
of
zoo
What happens when
VI
-
VZ
is an integer? First, as a
convenience, we translate the coordinate axes so that the point
zo coincides with
the origin. This will save us some writing, because instead
of
powers
of
z -
zo,
we will have powers
of
z. Next we let
VI
=
V2
+n with n a positive integer. Then,
since it is impossible to encounter any new zero
of
the indicialpolynomialbeyond
2Thecoefficientof w neednothaveapoleof
order
2. Itspole canbeof
order
oneas well.

408 14.
COMPLEX
ANALYSIS
OF
SOLDES
VI, the recursion relation, Equation (14.9), will be valid for all values
ofn,
and we
obtaina solution:
WI (z) =
ZVI
f(z)
=
ZVI
(I
+f
Ckl)
,
k=1
which is convergent in the region 0 < IzI < r for some r > 0 To investigate the
nature and the possibility
of
the second solution, write the recursion relations
of
Equation (14.9) for the smaller characteristic root
V:2:
=PII(V2+I)
~
I(V2 +
I)CI
=
-(V:2aO
+
b_l)
Co
=}
CI = PI,
1
(V2
+2)C2 = -(V:2al +bo)Co -
[(V2
+I)ao +
b_IlCI
=}
C2 sa P2,
(14.10)
1(V:2
+n - I)C,,_I ""
p,,-II(V2
+n - I)Co
=}
C,,_I =
P,,-I,
1(V:2
+
n)C"
=
I(VI)C"
= p"Co
=}
0 = P",
where in each step, we have used the result
of
the previous step in which Ck is
given as a multiple of
Co = 1. Here, the
p's
are constauts depending (possibly in
a very complicated way) on the
ak's and bk'S.
Theorem 14.2.3 guarantees two power series solutions only when VI -
V2
is
not an integer. When
VI -
V2
is an integer, Equation(14.10) shows that ariecessary
conditionfor a second
power
series solutionto exist is that
Pn
=
O.
Therefore,
when p" i 0, we have to resort to other means
of
obtaining the second solution.
Let us define the second solution as
=WI(Z)
,......-"-,
W2(Z) "" WI
(z)h(z)
=
ZVl
f(z)h(z)
(14.11)
(14.12)
and substitute in the SOLDE
to obtain a
FOLDE
in h', namely, h" + (p +
2w;lwI)h
'
=O,or, by substituting
w;lwI
=
vi/z-}
f'
If,
the equivalentFOLDE
h"+
C~I
+ 2;' +
p)
h'=
O.
14.2.5.
Lemma.
The coefficient
of
h'
in Equation (14.12) has a residue
ofn
+1.
Proof
Recall that the residue
of
a function is the coefficient of
Z-I
in the Laurent
expansion of the function (about
Z = 0).
Let
us denote this residue for the coef-
ficient of
h'
by
A-I.
Since
f(O)
= I, the ratio
f'
If
is analytic at z =
O.
Thus,
the simple pole at
z = 0 comes from the other two terms. Substituting the Laurent
expansion of
p(z)
gives
2vI 2vI
a-I'
-+p=
-+-+aO+alz+·
...
Z Z Z

i =
1,2,
14.2
COMPLEX
SOLOES
409
This shows that
A-I
= 2vI
+0_1.
On the otherhand, comparing the two versions
of
the indicialpolynomial v
2+(a_1
-1)v+b_2
and (v
-VI)(V-
V2)
= v
2
-
(VI
+
1I2)V
+
VI
V2
gives
VI
+
V2
=
-(a-I
-
I),
or 2vI - n =
-(a-I
- I). Therefore,
A_I=2vI+a_l=n+1.
0
14.2.6. Theorem. Suppose that the characteristic exponents
of
a
SOWE
with a
regular singular
point
at z = 0 are VI
and
1J:2.
Consider three cases:
1.
VI - Vz is not an integer.
2.
V:2
= VI - n wheren isa nonnegativeinteger.and
Pit,
as definedinEquation
(14.10), vanishes.
3. V2 = VI- n wheren is a nonnegativeinteger, and
Pn,
as definedinEquation
(14.10), does not vanish.
Then, in the first two cases, there exists a basis
of
solutions {WI,
W2}
of
theform
Wi
(z) =
zV'
(I
+f Cki1zk) ,
k~1
and in the third case, the basis
of
solutions takes theform
Wt(z)
=
ZV!
(1
+
~akZk),
W2(Z)
=zV'
(1
+
~bkl)
+
CWt(z)lnz,
where the power series are convergent in a neighborhood
of
z =
o.
Proof. The first two cases have been shown before. For the third case, we use
Lemma 14.2.5 and write
2vI
2f'
n +I
~
k
- +
-f
+p =
--
+L... CkZ ,
z Z
k~O
and the solutionfor the FOLDEin h' will be [see Equation (14.3) and the discussion
preceding it]
h'(z) =
z-n-t
(1
+f
bkl)
.
k~t
For n = 0, i.e., whenthe indicial polynomial has a double root, this yields h'(z) =
l/z+
I:~1
bkZ
k-
l,
or
h(z)
=
Inz+
gl (z), where gl is analytic in aneighborhood
ofz
=
O.
Forn
oF
0, we have
h'(z)
=
bn/z+
I:i4n
bkZ
k-
n-
1
and, by integration,
00
b
h(z)
= b
n
ln z +L
_k_l-
n
k;fnk-n
b In
-n
~
bk k b In
-n
()
= n
z+z
L...k_nz
= n
z+z
g2Z,
kim
(14.14)
410 14.
COMPLEX
ANALYSIS
OF
SOLOES
where gz is analytic in a neighborhoodof z =
O.
Substitutingh in Equation(14.11)
and recalling that Vz = VI - n, we obtain the desiredresults
of
the theorem. 0
14.3 Fuchslan Differential Equations
In
many cases of physical interest, the behavior of the solution of a SOLDE at
infinity is important. For instance, bound state solutions of the Schrodinger equa-
tion describing the probability amplitndes of particles in quantum mechanics must
tend to zero as the distance from the center
of
the binding force increases.
We have seen that the behavior of a solution is determined by the behavior
of
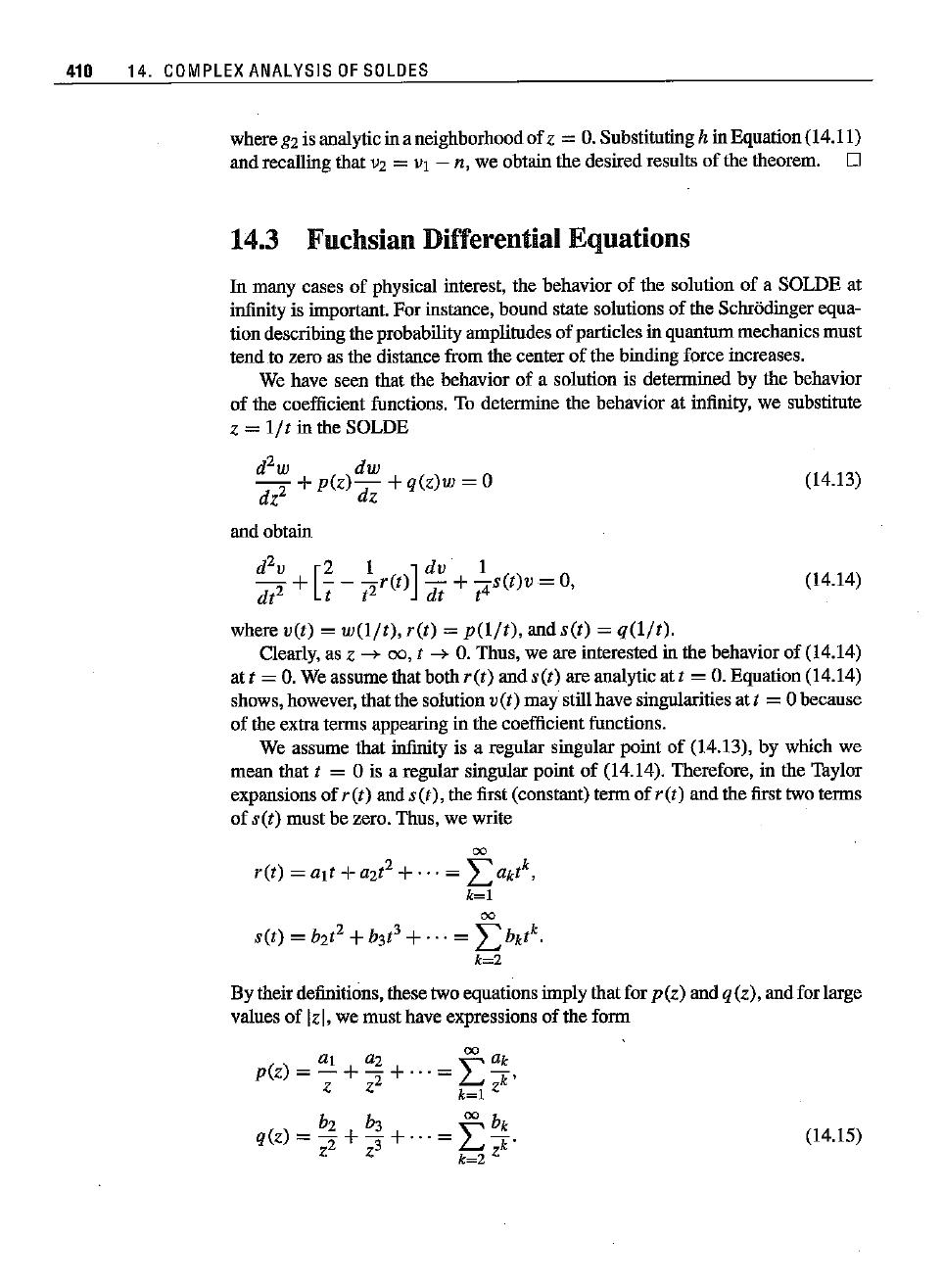
the coefficient functions. To determine the behavior at infinity, we substitnte
z =
lit
in the SOLDE
dZw
dw
-z
+
P(z)-d
+
q(z)w
= 0 (14.13)
dz z
and obtain
dZv
[2
1 ]
dv
1
- + - -
-r(t)
- +
-s(t)v
= 0,
dt
Z
t t
Z
dt t
4
where
v(t)
=
w(1lt),
r(t)
=
p(llt),
ands(t)
=
q(llt).
Clearly, as z --+
00,
t -->
O.
Thus, we are interested in the behavior of (14.14)
at
t =
O.
Weassume that both
r(t)
and
s(t)
are analytic at t =
O.
Equation (14.14)
shows, however, that the solution
v(t)
may still have singularities
att
= 0 because
of the extra terms appearing in the coefficient functions.
We assume that infinity is a regular singular point of (14.13), by which we
mean that
t = 0 is a regular singular point
of
(14.14). Therefore, in the Taylor
expansions
ofr(t)
and
s(t),
the first (constant) term
ofr(t)
and the first two terms
of
s(t)
must be zero. Thus, we write
00
r(t)
=
alt
+azt
Z
+... =
Lakl,
k~l
00
s(t)
=
bzt
Z
+b3t3
+... =
Lbktk.
k=Z
By their definitions, these two equations imply that for
p(z)
and
q(z),
and for large
values of [z],we must have expressions of the form
(14.15)

(14.16)
(14.17)
Fuchsian
DE
A
second-order
Fuchsian
DE
with
two
regular
singular
points
leads
to
uninteresting
solutions!
A
second-order
Fuchsian
DE
with
three
regular
singular
points
leads
to
Interesting
solutions!
14.3
FUCHSIAN
DIFFERENTIAL
EQUATlDNS
411
When infinity is a regular singular point
of
Equation (14.13), or, equiva-
lently, when the origin is a regular singular point
of
(14.14), it follows from
Theorem 14.2.6 that there exists at least one solution
of
the form VI(t) =
t"
(I
+
L~l
ckrk)
or, in terms
of
z,
-"
(I
~
Ck)
WI (z) = z +
L..J
k .
k=l
Z
Here a is a characteristic exponents at t = 0 of(14.14), whoseindicialpolynomial
is easily found to be
a(a
- I) +(2 -
al)a
+b: =
o.
14.3.1. Definition. A homogeneous differential equation with single-valued an-
alytic coefficient functions is called a Fuchsian differential equation (FDE)
if it
has only regular singular points in the extended complex plane, i.e., the complex
plane including the pointat infinity.
It
turns out that a particnlarkind of FDE describes a large class of nonelemen-
tary
functions
encountered
in
mathematical
physics.
Therefore,
itis
instructive
to
classify various kinds
of
FDEs. A fact that is used in such a classification is that
complex functions whose only singularities in the
extended complex plane are
poles are rational functions, i.e., ratios
of
polynomials (see Example 10.2.2). We
thus expect FDEs to have only rational functions as coefficients.
Consider the case where the equationhas at most two regular singnlar points at
Zl and Z2.Weintroduce
anew
variable
Hz)
= Z - Zl . The regnlar singular points
Z
-Z2
at
zt
and Z2 are mapped onto the points
~I
=
~(ZI)
= 0 and
~2
=
HZ2)
=
00,
respectively, in the extended
~-plane.
Equation (14.13) becomes
d
2u
du
d~2
+
<I>(~)
d~
+
e(~)u
= 0,
where u,
<1>,
and e are functions
of
~
obtained when Z is expressed in terms
of
~
in
w(z),
p(z),
and
qtz),
respectively. From Equation (14.15) and the fact that
~
= 0 is at most a simple pole
of
<I>(~),
we obtain
<I>(~)
=
al/~.
Similarly,
e(~)
=
b2/~2.
Thus, a SOFDE with two regular singular points is equivaleut
to the DE
wIt +
(al/Z)w'
+
(b2/Z
2)W
=
O.
Mnltiplying both sides by Z2, we
obtain
Z2
w"
+
alzw'
+b2W = 0, which is the second-order Euler differential
equation. A general nth-orderEuler differential equationis equivalent to aNOLDE
with constant coefficients (see Problem 13.28). Thus, a second order Fuchsian DE
(SOFDE) with two regular singularpoints is eqnivalent to a SOLDE with constant
coefficients and produces nothing new.
The simplest SOFDE whose solutions may include nonelementary functions
is therefore one having three regular singnlar points, at say
ZI,
Z2, and Z3. By the
transformation
~(z)
= (z - ZI)(Z3 - Z2)
(z - Z2)(Z3 -
ZI)

412 14.
COMPLEX
ANALYSIS
OF
SOLOES
(14.18)
Riemann
differential
equation
we can map Zl, Z2, and Z3 onto
~I
=0,
~2
=00, and
~3
= 1. Thus, we assume
that the three regular singular points are at
Z =0, z = I, and z =00.
It
can be'
shown [see Problem (14.8)] that the most geuera!
p(z)
and
q(z)
are
Al
BI
A2 B2 A3
p(z)
= - +
--1
and
q(z)
=
2:
+ ( 1)2 ( I)
z
z-
z
z-
zz-
We thus have the following theorem.
14.3.2.
Theorem.
The mostgeneral secondorder Fuchsian DE with three regular
singularpoints can be transformed into the form
/I
(AI
BI)
I
[A2
B2
A3]
0
w+-+--w+-+
-
w=,
z Z - I z2 (z - 1)2
z(z
- I)
where
AI,
A2, A3,
BI,
and B2 are constants. This equation is calledthe
Riemann
differential equation.
We can write the Riemaruo DE in terms
of
pairs of characteristic exponents,
(;\,1,1.2), (1-'1,1-'2), and (VI, V2), belonging to the singular points 0, 1, and 00,
respectively. The indicia! equations are easily found to be
1.
2
+
(AI
- 1)1.+A2 = 0,
1-'2
+
(BI
-
1)1-'
+B2 = 0,
v
2
+(I -
Al
-
BI)v
+A2 +B2 - A3 =
O.
By writing the indicia! equations as (A - 1.1)(1. - 1.2) = 0, and so forth and
comparing coefficients, we can find the following relations:
Al
= I - Al - 1.2,
BI
= I -
1-'1
-
1-'2,
AI+BI
=VI+V2+1,
A2 =
1.11.2,
B2 =
1-'11-'2,
A2 +B2 - A
3
= VIV2.
These equations lead easily to the Riemann identity
Al +1.2+
1-'1
+
1-'2
+VI +
V2
= 1.
(14.19)
(14.20)
Substituting these results in (14.18) gives the following result.
14.3.3.
Theorem.
A secondorder Fuchsian DE with three regular singuiarpoints
in the extendedcomplex plane is equivalent to the Riemann DE,
(
1 - 1.1 - 1.2
1-
"1-
"2)
wI!+ +
t'"
r-
w'
Z
z-I
[
1.11.2
1-'11-'2
VI
V2
-
1.11.2
-1-'11-'2]
+
--+
+
w-O
Z2 (z - 1)2
z(z
- I) - ,
which is uniquely determined by the pairs
of
characteristic exponents at each
singularpoint. The characteristicexponents satisfy the Riemann identity, Equation
(14.19).
