Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations
Подождите немного. Документ загружается.


Schriidinger
equation
describes
a
classical
statistical
mixture
when
Ii -e-
O.
13.9 NUMERICAL
SOLUTIONS
OF
DES
383
The
first equation
of
(13.51) gives an interesting result when
1i,
--+ °because
in this limit, the RHS
of
the equation will be zero, and we get
as
1 2
-+-mv
+V
=0.
at
2
Taking the gradient
of
this equation, we obtain
(:t
+v'V)mv+VV=O,
which is the equation
of
motion
of
a classicalfluid with velocity field v = VS/ m.
We thus have the following:
13.8.2.Proposition. In the classical limit, the solution
of
the Schriidinger equa-
tion describes a fluid (statistical mixture)
of
noninteracting classicalparticles
of
mass m subject to the potential
V(r).
The density
and
the Currentdensity
of
this
fluid are, respectively, the probabilitydensity p = I'"1
2
and
theprobability current
density
J
of
the quantum particle.
13.9 Numerical Solutionsof DEs
The majority
of
differential equations encountered in physics do not have known
analytic solutions. One therefore resorts
tonumericalsolutions. Thereare a variety
of
methods having various degrees
of
simplicity
of
use
and accuracy. This section
considers a few representatives applicable to the solution
of
ODEs. We make
frequent use
of
techniques developed in Section2.6. Therefore,the reader is urged
to consult that section as needed.
Any normal differential equation
of
nth
order,
dnx
_ . (n-e
l},
--F(x,x,
...
,x
,t),
dt
n
can be reducedto asystem
of
nfirst-orderdifferentialequationsby defining
Xl
= x,
Xz =
i,
...
X
n
=
x(n-l).
Thisgivesthesystem
We restrict ourselves to a
FaDE
of
the form i =
f(x,
t)
in which f is a well-
behavedfunction
of
two realvariables.
At
the
end
of
the section, we briefly outline
a technique for solving second-order DEs.
Two general types
of
problems are encountered in applications. An initial
value problem (IVP) gives
X (t) at an initial time to and asks for the value
of
X at
other times.
The
second type, the boundary value problem (BVP), applies ouly to
differential equations
of
higher order thanfirst. A second-order
BVP
specifies the
value
of
x(t)
and/or
i(t)
at
one
or more points and asks for X or i at other values
of
t. We shall consider only IVPs.

384 13.
SECOND-ORDER
LINEAR DIFFERENTIAL
EQUATIONS
13.9.1 Using the Backward Difference Operator
Let us consider the IVP
i =
f(x,
r),
x(to) = xo·
(13.54)
(13.55)
(13.56)
The problem is to find
{Xk
= x(to +kh)}£"=I' given (13.54).
Let us begiu by integrating (13.54) between t
n
aud t
n
+h:
l
to
+
h
x(tn +h) -
x(t
n)
=
i(t)
dt.
to
Chauging the variable of integration to s = (t - tn)/ h aud using the shift operator
Eintroduced in Section 2.6 yields
x
n+'
- X
n
= h
fa'
i(t
n
+
sh)
ds =h
fa\E
S
i(t
n)]
ds.
Since a typical situation involves calculating x
n+'
from the values of
x(t)
aud
i(t)
at preceding steps, we waut au expression in which the RHS of Equation
(13.55) contains such preceding terms. This suggests expressing E in terms of the
backward difference operator.
It
will also be useful to replace the lower limit of
integration to -
p, where p is a numberto be chosen later for convenience. Thus,
Equation (13.55) becomes
Xn+t
= x
n-
p
+h
[i~
(1 -
V)-S
dS]
in
[I
'
00
r(-s
+
l)ds
k] .
= x
n
-
p
+h
_p
~
k!r(-s
_ k +1)
(-V)
X
n
= x
n-
p
+h
(f>iP)V
k
)
in,
k=O
where
(p)
(-l)kl'
r(-s+l)ds
III
a
k
=--
=-
s(s+l)
..
·(s+k-l)ds.
k! r P
r(-s
- k +1) k!
-p
(13.57)
Keeping the first few terms for p = 0, we obtain the useful formula
Due to the presence of V in Equation (13.58), finding the value of
x(t)
at
tn+1
requires a knowledge of
x(t)
aud
i(t)
at points to, tl,
...
, tn' Because
of
this,
formulas
of
open
and
closed
type
13.9
NUMERICAL
SOLUTIONS
OF
DES
385
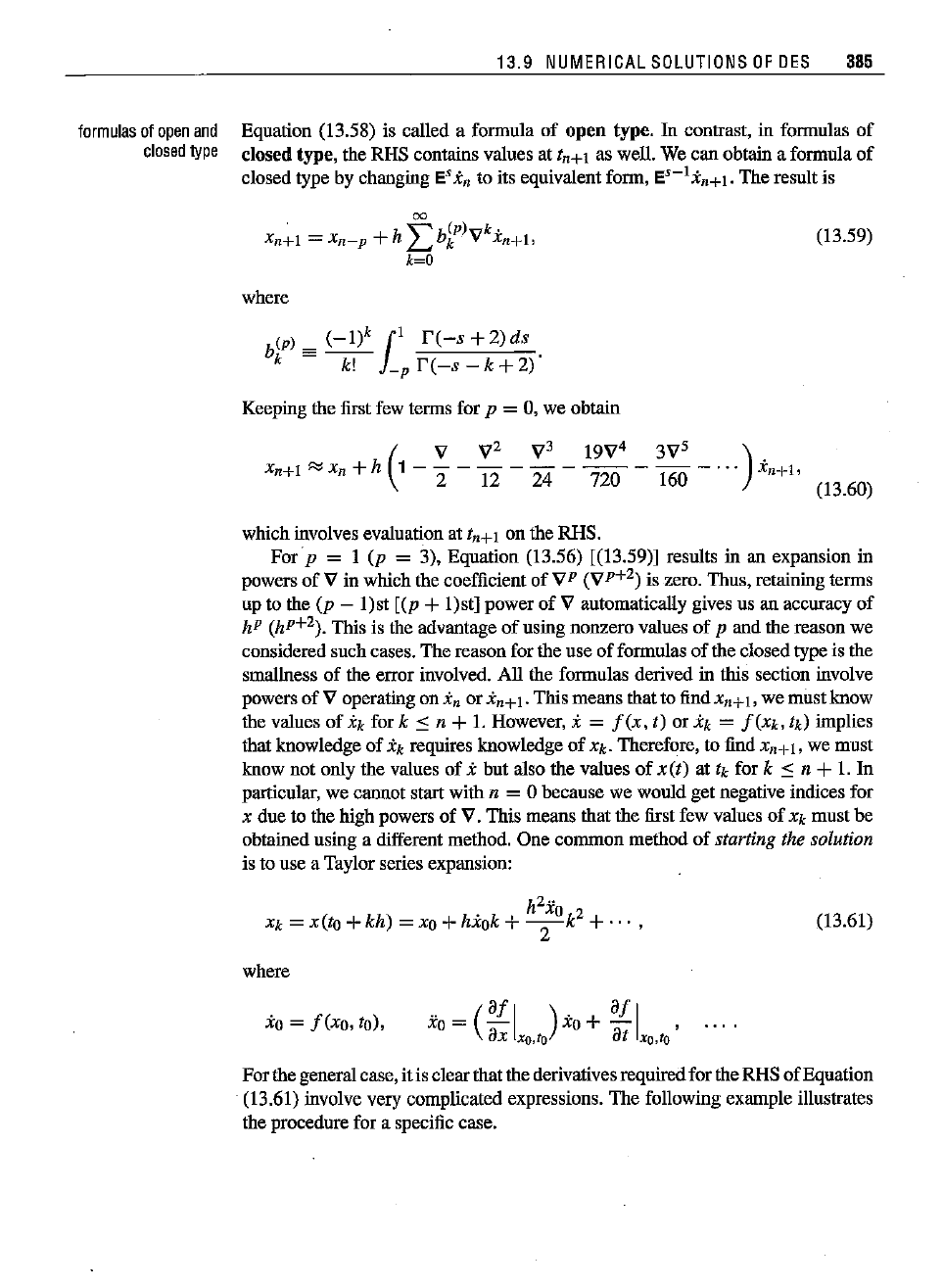
Equation (13.58) is called a formula of
open
type. In contrast, in formulas
of
closed type, the RHS contains values at t
n
+1 as well. We can obtain a formula
of
closed type by changing E' x
n
to its equivalent form,
E,-l
xn
+
1.
The result is
00
.
h"
bCP)V
k
.
X
n+l
= x
n-
p
+
L-
k'
Xn+b
k~O
where
b
CP)
es
(_l)k
1
1
r(-s+2)ds.
k k! _P I'{
-s
- k +2)
Keeping the first few terms for p = 0, we obtain
x +1 "" x +h
(1
_ V _ V
2
_
V
3
_
19V
4
_
3V
5
_
...
) X +1
n n
2 12 24 720 160 " ,
(13.59)
(13.60)
which involves evaluation at
t
n
+l on the RHS.
For
p = 1
(p
= 3), Equation (13.56) [(13.59)] results in an expansion in
powers
of
V in which the coefficient of VP (VP+2)is zero. Thus, retaining terms
up to the
(p
-
l)st
[(p
+l)st] power
of
V automatically gives us an accuracy of
hl' (h
p
+2). This is the advantage
of
using nonzero values of p and the reason we
considered such cases. The reason for the use of formulas of the closed type is the
smallness of the error involved. All the formulas derived in this section involve
powers
of V
operating
on
x,
orX
n
+l . Thismeans
that
tofindx
n
+l , wemustknow
the values of Xk for k :0 n + 1. However, x =
f(x,
t) or Xk =
f(xk,
tk) implies
that knowledge of
Xkrequires knowledge
of
Xk.Therefore, to find X
n+l,
we must
know not ouly the values of x but also the values
of
x(t)
at tk for k :0 n +1. In
particular, we cannot start with
n = 0 because we would get negative indices for
x due to the high powers of V. This means that the first few values
of
Xkmust be
obtained using a different method. One common method of
starting the solution
is touse a
Taylor
series
expansion:
h2xo 2
Xk = x (to
+kh)
=xo
+hxok+
-2-k
+... ,
where
(13.61)
Xo
=
Itx«;
to),
..
(8
f
I ). +
8f
I
xo=
- XQ - ,
ax Xij,to
at
XQ,to
For the general case, it is clearthat the derivatives requiredfor the RHS of Equation
.(13.61) involve very complicated expressions. The following example illustrates
the procedure for a specific case.

386 13.
SECOND-ORDER
LINEAR
DIFFERENTIAL
EQUATIONS
13.9.1.
Example.
Letus solvethe IVPx+x +e'x
2
= 0 withx(O) =
I.
Wecanobtain
a Taylor series expansion for x by noting that
. 2
xQ=
-xo
-xO'
xo = x(O) = -XO -
2xOXO
- x5,
... .. ".,2 2 ..
4'
2
xo=-xO-£...\,o-
xQxO-
xQxO-xo'
Continuing in this way. we can obtain derivatives
of
all orders. Substituting xQ = 1 and
keeping terms up to the fifth order, we obtain
iO =
-2,
iQ = 5,
x·o
=
-16,
d4XI
=65,
dt
4
,=0
d
5
x I =
-326.
dt
5
,~O
(13.62)
Substituting thesevalues
in a Taylorseriesexpansionwithh = 0.1 yields
Xk
= I - 0.2k +0.025k
2
-
0.0027k
3
+(2.7 x 1O-4)k
4
- (2.7 x 1O-5)k
5
+....
Thus,Xt = 0.82254,
X2
= 0.68186, and
X3
= 0.56741. Thecorresponding values
oU
can
be calculatedusingtheDE.Wesimplyquotetheresult:
Xt = -1.57026, x2 = -1.24973,
X3
= -1.00200. III
Once the starting values are obtained, either a formula
of
open type or one
of
closed type is used to find the next x value. Ouly formulas
of
open type will
be discussed here. However, as mentioned earlier, the accuracy
of
closed-type
formulas is better. The price one pays for having
x,,+! on the RHS is that using
closed-type formulas requires
estimating Xn+t. This estimate is then substituted
in the RHS, and an improved estimate is found.
The
process is continued until no
further improvement in the estimate is achieved.
The
use
of
open-typeformulas involvessimple substitution
of
the knownquan-
tities
Xo,
xi,
...
, X
n
on the RHS to obtain x,,+t.
The
master equation (for p = 0)
is (13.58).
The
number
of
powers
of
V that are retained gives rise to different
Euler's
method
methods. For instance, when no power is retained, the method is called
Euler's
method,
for which we nse xn+t
'"
x" +
hin.
A more commouly used method
is
Adam's
method,
for which all powers
of
V up to and including the third are
Adam's
method
retained. We then have
or,
in
tenus
of
values
of
i,
h
Xn+t
'"
X
n
+24
(55i
n
-
59i
n_t
+
37i,,_2
-
9i,,_3).
Recall
thatik
=
f(xk,
tk). Thus, if we know the values xs,
Xn-I,
X,,-2, andx,,_3,
we can obtain
x
n
+1-
13.9.2.
Example.
Knowing
xo,
xI,
X2,
andX3,we canuseEquation(13.62)to calculate
X4
forExample13.9.1:
0.1
X4
""
X3
+ 24 (
55x
3 - 59x2+37xl - 9xo) = 0.47793.

13.9 NUMERICALSOLUTIONS
OF
DES
387
With
X4 at our
disposal,
wecan
evaluate
x4 = - X4 -
xl
e
t4
, and
substitute
itin
to
find
X5.
and
so on.
A crucial fact about such methods is that every value obtaiued is in error by
some
amount,
and
using such
values
to
obtain
new
values
propagates
the
error.
Thus, errorcau accumulate rapidly aud make approximatious worse at each step.
Discussiou
of
error propagation aud error
aualysis-topics
that we have not, aud
shall not,
cover-is
commouin the literature (see, for example, [Hild 87, pp.
267-
268]).
13.9.2 The Runge-Kutta Method
The FODE
of
Equatiou (13.54) leads to a unique Taylor series,
h
2
x(to +h) = xo +
hio
+
2io
+... ,
where
io,io,
aud all the rest of the derivatives cau be evaluated by differeutiatiog
i = lex, t). Thus, theoretically, the Taylor series gives the solution (for to +h;
but to +2h, to +3h, aud so on cau be obtaioed similarly). However, in practice,
the Taylor series converges slowly, aud the accuracy involved is not high. Thus
oue resorts to other methods of solution such as described earlier.
Runge-Kutta
method
Another method, known as the
Runge-Kulla
method,
replaces the Taylor
series
with
Xn+l
= Xn +h
[ao!(xn,
tn) +
i-»-
+
bjh,
t
n
+/Ljh)] ,
J~t
(13.63)
(13.64)
where ao aud
{aj,
b],
/Lj
}}=t
are constauts choseusuch that
iftheRHS
of (13.64)
were expauded in powers of the spacing
h, the coefficients of a certaio number of
the leading terms would agree with the corresponding expausion coefficieuts of
the RHS
of
(13.63).
It
is customary to express the b's as linear combinations of
preceding values of
!;
i-I
hb, =
LAirkr,
r=O
i = 1,2,
...
, p.

(13.65)
388
13.
SECOND-ORDER
LINEAR
DIFFERENTIAL
EQUATIONS
The k; are recursively defined as
ko =
hf(x,,,
In),
k; =
hf(x"
+b-h, In +P,rh).
Then
Equation (13.64) gives
Xn+l
= X
n
+
L~=oarkr'
The
(nontrivial)
task
now
is to determine the parameters a-,
J-Lr,
and
Aijo
CarleDavid TalmoRunge (1856-1927),afterreturningfrom
a six-month vacation in Italy, enrolled at the University
of
Munich to study literature. However, after six weeks
of
the
course he changed to mathematics and physics.
Rungeattended
courses
with
Max
Planck,
andtheybecame
close friends. In 1877 both went to Berlin, but Runge turned
topuremathematicsafterattendingWeierstrass'slectures. His
doctoraldissertatioo(1880)dealt withdifferentialgeometry.
Aftertakinga secondary-schoolteacherscertificationtest,
he returned to Berlin, where he was influenced
by Kronecker.
Runge then worked on a procedure for the numerical solution
of algebraicequationsin which the roots were expressedas infiniteseriesof rationalfunc-
tions
of
the coefficients. In the area
of
numerical analysis, he is credited with an efficient
method
of
solving differential equations numerically,
work
he did with Martin Kutta.
Runge published little at that stage, but after visiting
MitlagMLeffler
in Stockholm in
September 1884 he produced a large nnmber of papers in Mittag-Leffler'sjonmal Acta
mathematica.
In 1886. Runge obtained a chair at Hanover and remainedthere for 18 years.
Within a year Runge had moved away from pure mathematics to study the wavelengths
of
the spectral lines of elements other than hydrogen. He did a great deal
of
experimental
work and published a great quantity
of
results, including a separation of the spectral lines
of
helium in two spectral series.
In 1904 Klein persuaded Gottingen to offer Runge a chair
of
applied mathematics, a
post that Rnngeheld nnti!he retired in 1925.
Runge was always a fit and active man, and on his 70th birthday he entertained his
grandchildren by doing handstands. However, a few months laterhe
had
a heart attack and
died.
In general, the determination
of
these constants is extremely tedious.
Let
us
consider the very simple case where
p = I,
and
let
A es
AOl
and IL sa ILl.
Then
we obtain
Xn+l
= x" +aoko +
alkj,
where ko =
hf(x
n
, In)
and k
j
=
hf(x
n
+Ako, In +p,h).
Taylor-expanding
kj,
a function
of
two variables, gives?
k: =
hf
+h
2(lLft
+
Vfx)
+
h;
(1L
2
I« +2Ap,ffxt +A
2
f2
fxx) + O(h
4
) ,
9ThesymbolO(h
ln
) meansthatalltermsof orderhlll·and higherhavebeenneglected.
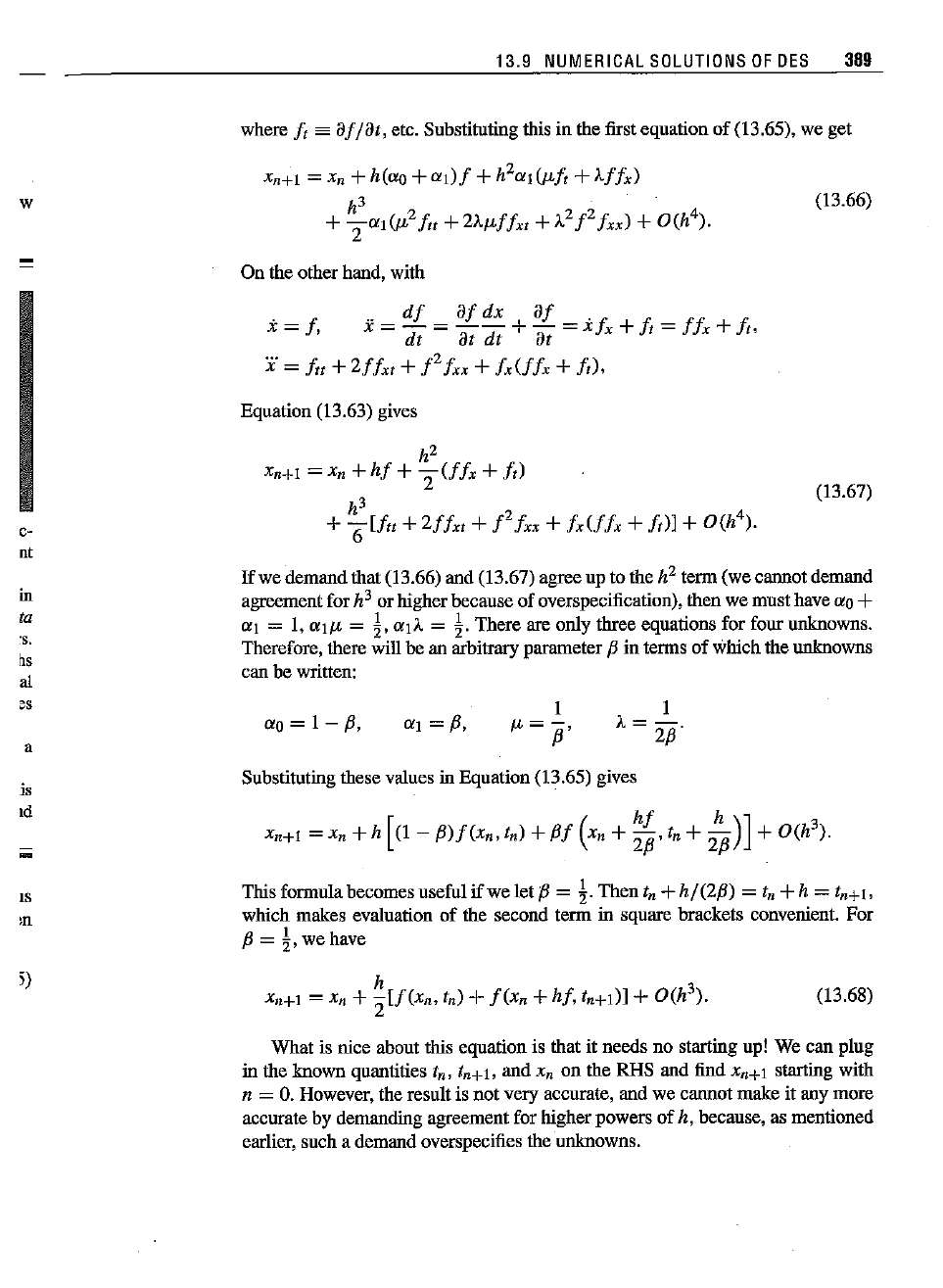
13.9 NUMERICAL SOLUTIONS
OF
OES
389
where It es anal,etc. Substituting this in
the
first equation
of
(13.65), we get
w
c-
nt
Xn+l
= x" +h(ao +
al)I
+h
2a\(J-LIt
+
Allx)
+ h;
al(J-L2
Itt +
2AJ-Lllxt
+A
2
1
2
Ixx)
+O(h
4
).
On
the
otherhand, with
..
df
al dx al .
x=
I,
x = dt = at
dl
+at=
xIx
+It =
IIx
+
It,
x'
= Itt +
2IIxt
+1
2
Ixx
+Ix<Jlx +
It),
Equation (13.63) gives
h
2
x
n+!
= X
n
+
hI
+
z<Jlx
+
It)
h
3
2 4
+
6[Itt
+
2IIxt
+I Ixx +Ix<Jlx +
ft)]
+O(h ).
(13.66)
(13.67)
lfwe
demand
that(13.66) and (13.67) agree up to
the
h
2
term
(we
cannot
demand
agreementfor h
3
or higher because
of
overspecification),
then
we
mnst
have ao+
al
= 1,
alJ-L
=
~,aIA
=
~'
There
are
ouly
three equations for four unknowns.
Therefore, there will be an arbitrary parameter
f3
in terms
of
which
the
unknowns
canbe
written:
in
ta
~S.
hs
al
es
a
ao =
1-
f3,
al
=
f3,
1
A = 2f3'
is
td
IS
m
Substituting these values in Eqnation (13.65) gives
This formula becomes useful
if
we
let
f3
=
~'
Then
In
+hf(2f3) =
In
+h = In+l,
which makes evaluation
of
the second
term
in square brackets convenient.
For
f3
=
~,we
have
i)
h 3
X,,+l
= x
n
+"2[f(X
n,
In)+
I(x
n
+
hI,
In+I)]
+O(h ).
(13.68)
What
is nice about this equation is that it needs no starting up! We
can
plug
in the
known
quantities
In,
t
n
+
1,
and
X
n
on
the
RHS and find X
n+
I starting with
n =
O.
However,
the
result
is notvery
accurate,
and
we
cannot
makeitanymore
accurate by demanding agreement for higher
powers
of
h, because, as mentioned
earlier,
sucha
demand
overspecifies the
unknowns.

390
13.
SECOND-ORDER
LINEAR
DIFFERENTIAL
EQUATIONS
Martin
Wilhelm
Knlla
(1867-1944)losthis parents whenhe was still a child, and together
with his brother
went
to his unclein Breslauto go to
gymnasium.
He attended the University
of
Bres1au from 1885-1890, and the University
of
Munich from 1891-1894 concentrating
mainly on mathematics, but he was also interested
in
languages, music, and art. Although
he completedthe certification for teachingmathematics and physics in 1894, he did not start
teachingimmediately. Instead, he assisted von Dyckat the Technische HochschuleMiinchen
until 1897 (and then again from 1899 to 1903).
From 1898 to 1899 he studied at Cambridge, and a year later, he finished his Ph.D.
at the University
of
Munich. In 1902, he completed his habilitation in pure and applied
mathematicsat
the
TechnischeHochschule
Munchen,
where
he
became
professorof applied
mathematics five years later. In 1909 he accepted an offer from University
of
Vienna, but
a year later he went to the Technische Hochschule Aachen as a professor. From 1912 until
his retirement in 1935 he worked at the Technische Hochschule Stuttgart.
Kutta's name is well known not only to physicists, applied mathematicians, and en-
gineers, but also to specialists in aerospace science
and
fluid mechanics.
The
first group
use the
Runge-Kutta method, developed at the beginning
of
the twentieth century to obtain
numerical solutions to ordinary differential equations.
The
second group use the
Kutta-
Zhukovskii formula for the theoretical description
of
the buoyancy
of
a body immersed in
a nonturbulent moving fluid. Kutta's
work
on
the
application
of
conformal mapping to the
study
of
airplane wings was later applied to the flight
of
birds, and further developed by L.
Prandtl in the theory
of
wings.
Kutta obtained the motivation for his first scientific publication from
Boltzmann
and
others(includinga historian
of
mathematics)
when
workingon
the
theoreticaldetermination
of
the
heat
exchangedbetween two concentric cylinders
kept
at constant temperatures. By
applying the conformal mapping technique, Kutta
managed
to obtain numerical values for
the
heat
conductivity
of
air
that
agreed
well with
the
experimental values
of
the time.
Three
ofKutta's
publicationsdealtwiththe history
of
mathematics,for
which
he profited
greatly because
of
his knowledge
of
the Arabic language.
One
of
the
most
importanttasks
of
appliedmathematics is to approximate numerically
the initial value problem
of
ODEs
whose solutions
cannot
be found in closed form. After
Euler(1770)
had
alreadyexpressedthe basic idea, Runge (1895) and Heun(1900) wrote down
the appropriate formulas. Kutta's contribution was to considerably increase the accuracy,
and allow for a larger selection
of
the parameters involved. Afteraccepting a professorship
in Stuttgart in 1912, Kutta devoted all his time to teaching. He was very
much
in demand
as a teacher,
and
it is
said
that his lectures were so
good
that
even engineering students took
an interest in mathematics.
(Taken from W. Schulz,
"Martin
Wilhelm Kutta," Neue Deutsche Biographie 13, Berlin,
(1952-) 348-350.)
Formulas
that
give
more
accurate
results
can
be
obtained
by
retaining
terms
beyondp
= 1.
Thus,
for
p = 2,
if
we
writex
n
+ ! = x
n
+
I:~~o
OI,k"
there
will
be
eight
unknowns
(three
01
's,
three
Aij
's,
and
two
u
's),
and
the
demand
for
agreement
between
the
Taylor
expansion
and
the
expansion
of
f
up
to h
3
will
yield
only
six
equations.
Therefore,
there
will
be
two
arbitrary
parameters
whose
specification

13.9 NUMERICAL
SOLUTIONS
OF
OES
391
results iu variousformulas.
The
details
of
this
kind
of
algebraic derivationare very
messy,
so we will
merely
consider twospecific
formulas.
Onesuch
formula,
due
to Kutta, is
Xn+l = X
n
+
~(ko
+4kl +k2) +
O(h
4
),
where
ko =
hf(x
n
, In),
kl =
hf(x
n
+
!ko,
In +
!h),
k2 =
hf(x
n
+2kl - ko, In +
h).
(13.69)
A second formula, dne to Heun, has the
form
X
n
+! = X
n
+
!(ko
+3k2) +
O(h
4
),
where
ko =
hf(x
n,
In),
kl =
hf(x
n
+
~kO,
In
+
~h),
ka =
hf(x
n
+
~kl
- ko, In +
~h).
These
two formulas are
of
about
the
sarne
order
of
accuracy.
13.9.3.Example. LetussolveIheDEofExample 13.9.1usingIheRunge-Kuua meIhod.
WiIh
to = 0,
xO
= I, h = 0.1, and n = 0, Equation (13.69) gives ko =
-0.2,
kl =
-0.17515, k2 = -0.16476. so Ihat
XI = 1 +
~(-0.2+4(-0.17515)
- 0.16476) = 0.82244.
This
Xl, h =0.1, and tl =to+ h = 0.1 yieldIhefollowingnew values:ko = -0.15700,
kl
= -0.13870, k2 = -0.13040, whicb in turn give
x2 = 0.82244 +
~[-0.15700
- 4(0.13870) - 0.13040] = 0.68207.
Wesimilarlyobtain
X3 =0.56964andX4 =0.47858. OntheoIherhand, solvingIheFODE
analyticallygives Iheexactresult
x(t) =
e-
t
/(1+ t).
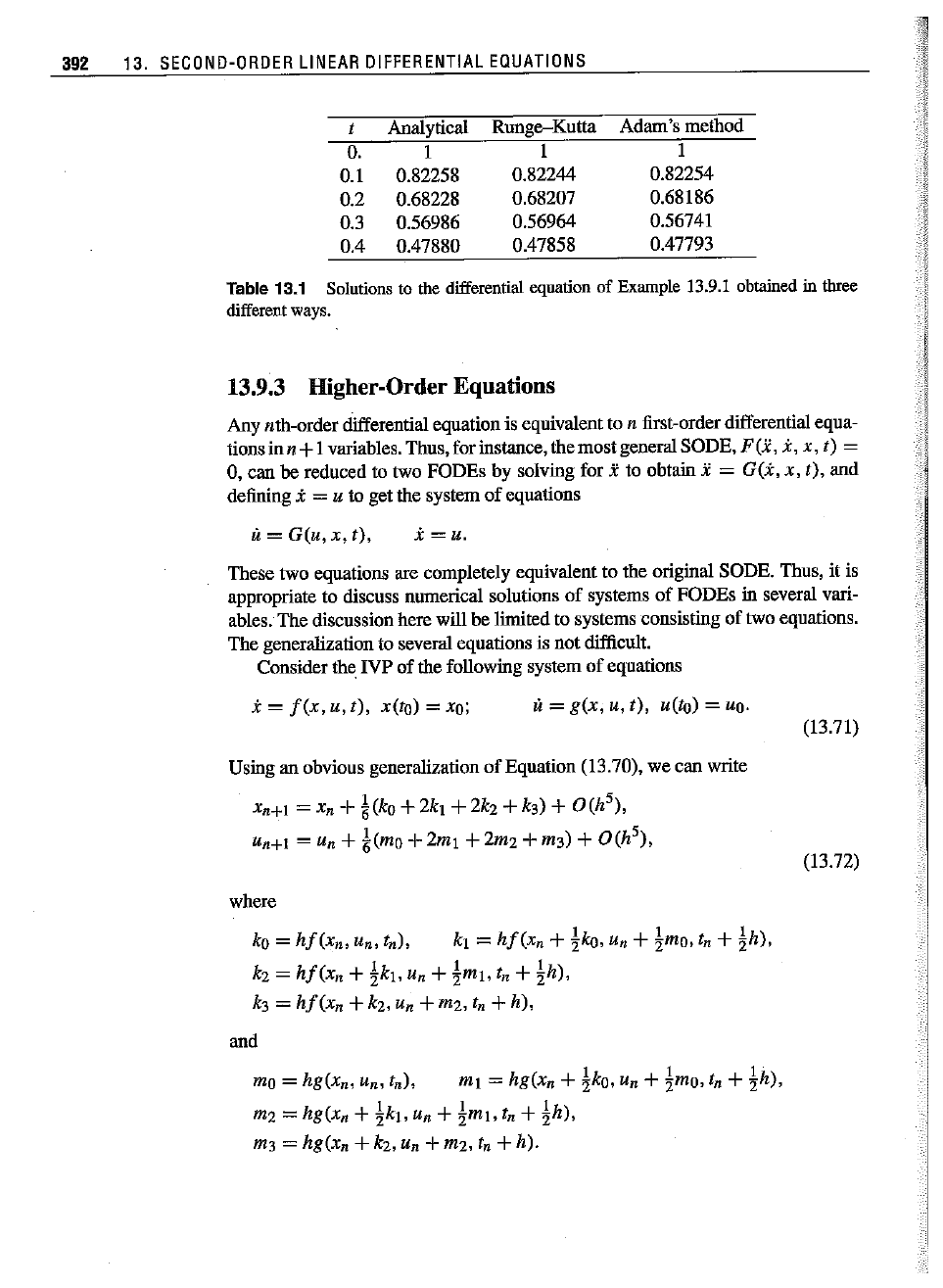
Table
13.1
compares
the
values
obtained
here,
those
obtained
using
Adam's
method,
and
theexact
values
tofive
decimal
places.It is
clear
that
the
Runge-Kutta
method
is
more
accurate
than
the
methods
discussed
earlier.
II1II
The
accuracy
of
Ihe
Runge-Kutta
meIhodand
the
fact Ihat it requiresno startup
procedure
make
it one
of
the
most
popularmethods for solving differential equa-
tions. The
Runge-Kutta
method
can
be
made
more
accurateby usinghighervalues
of
p,
For
instance, a formula that is used for p = 3 is
Xn+1 = X
n
+
~(ko
+2kl +2k2 +k3) +
O(h
5
) ,
where
(13.70)
ko =
hf(x
n
, In),
k2 =
hf(x
n
+
!kl,
In
+
!h)
kl =
hf(x
n
+
!ko,
In +
!h),
k3 =
hftx;
+k2, In +h).
392 13.
SECONO-ORDER
LINEAR
DIFFERENTIAL
EQUATIONS
I Analytical
O.
1
0.1 0.82258
0.2 0.68228
0.3 0.56986
0.4 0.47880
Runge--Kutta
1
0.82244
0.68207
0.56964
0.47858
Adam's method
1
0.82254
0.68186
0.56741
0.47793
Table 13.1 Solutions to the differential equation of Example 13.9.1 obtained in three
different
ways.
13.9.3 Higher-OrderEquations
Any nth-order differential equationis equivalent to n first-order differential equa-
tionsinn+
1variables. Thus, for instance, the mostgeneral SOOE,
F(i,
X,x, I) =
0, can he reduced to two FOOEs by solving for i to obtain i =
G(x,
x, z), and
defining x= U to get the system
of
equations
u=
G(u,
x,
I),
x=u.
These two equations are completely equivalent to the original SOOB. Thus, it is
appropriate to discuss numerical solutions
of
systems of FOOEs in several vari-
ables. The discussion here will be limited to systems consisting of two equations.
The generalization to several equations is not difficult.
Consider the IVP of the following system
of
equations
x=
f(x,
u, I), x(lo) =
xo:
u=
g(x,
u, I), u(to) =uo.
(13.71)
Using an obvious generalization
of
Equation (13.70), we can write
X
n
+l = X
n
+
!(ko
+
2kl
+ 2k2 + k3) +
O(h
s),
U
n+l
=
Un
+
!(mo
+
2ml
+ 2m2 + m3) +
O(h
s),
where
ko =
hf(x
n,
Un, In), kl =
hf(x
n
+ !kO, Un +
!mo,
In +
!h),
k2 =
hf(x
n
+
!kl,
Un +
!mj,
In +
!h),
k3 =
hf(x
n
+ ka.
Un
+ m2, In +
h),
and
mo =
hg(x
n,
Un, t
n
),
mj
=
hg(x
n
+
!k
o,
Un
+
!mo,
In
+
!h),
m2 =
hg(x
n
+
!kj,
Un +
!ml,
In +
!h),
m3 =
hg(x
n
+ k2, Un +
m2,
t
n
+
h).
(13.72)
