Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations
Подождите немного. Документ загружается.


(6.6)
(6.7)
III
step
function
orB
function
8function
as
derivative
01
B
function
6.1
CONTINUOUS
INDEX
163
of
Dr
(x -
x')
around
x'
is given, roughly, by the distance between the points at which
DT (x -
x')
drops to zero: T (x -
x')
=
±1l'.
or X -
x'
=
±1l'
/T. This width is roughly
Ax = 2n/ T. whichgoestozeroasT grows.Again,thisisasexpectedofthedeltafunction.
III
The
precedingexample suggestsauotherrepresentation
of
the Diracdeltafunc-
tion:
I
joo.(
')
8(x -
x')
= - e'
x-x
'dt.
2".
-00
6.1.3.
Example.
A third representation of the Dirac delta function involvesthe step
function
e(x
-
x'),
which is defined as
I
{O
if
x e
x',
B(x
-x)
==
1
if
x>x'
and is discontinuous at x = x', We can approximate this step function by many continuous
functions, such as
T
E
(x -
x')
defined by
[
0
if
X~X'_,.
T,(x-x')==
2~(X-x'+')
if
x'-,
~x~x'+,.
1
if
x
~
x'
+€,
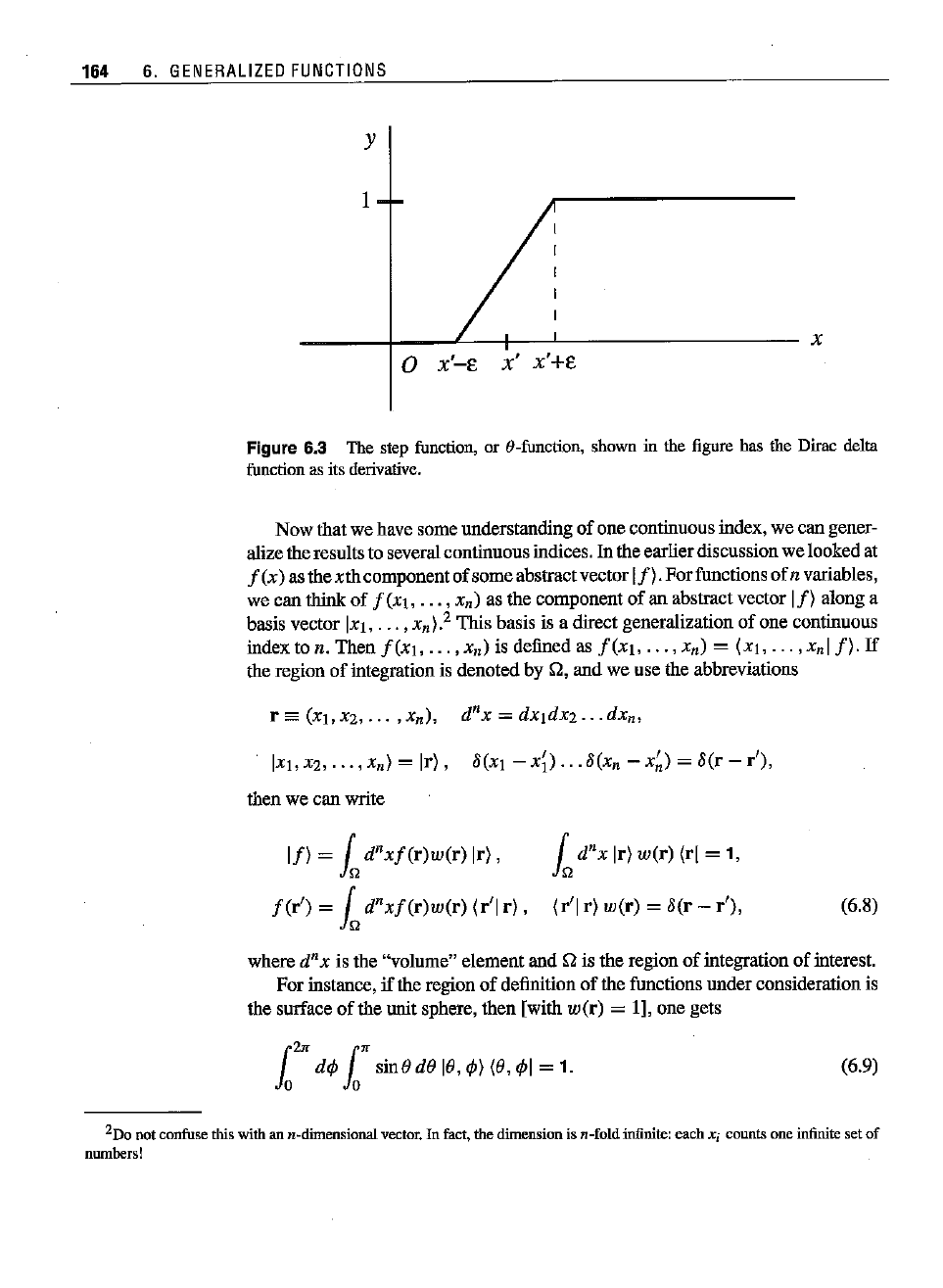
where e is a small positive number as shown in Figure 6.3. It is clear that
B(x-x')=
tiro
T,(x-x').
,--+0
Now
let
us consider the derivative
of
T
e
(x -
x')
with
respect to x:
r
if
x
<X'-f,
at; , 1
if
x'
- € < X <
x'
+E,
-(x-x)=
-
dx
2,
0 if
x >
x'
+E.
We note that the derivative is not defined at x =
x'
- e and x =
x'
+ E. and that dT€/dx
is zero everywhere except when x lies in the interval (x' - E,
x'
+
f),
where it is equal to
1/(2f)
and goes to infinity as e
.....,.
O.Here againwe see signs
of
the deltafunction. In fact,
we also note that
1
00
(dT,)
t:
(dT,)
l
X
'+E
1
- dx = - dx =
-dx
=
I.
-00
dx x'
-,
dx
x'
_,
2<
It is
not
surprising, then, to find that
lim€......,)oo
dT€(x -
x')
= 8(x -
x').
Assuming that the
dx
interchange
of
the order
of
differentiation and the limiting process is justified, we obtain
theimportantidentity
d I I
-B(x
-
x)
= 8(x -
x).
dx
164 6.
GENERALIZED
FUNCTIONS
y
1
x
o
X'-E
x'
x'+E
Figure 6.3 The step function, or 8-function, shown in the figure has the Dirac delta
function
asits
derivative.
Now
that
wehavesome
understanding
of one
continuous
index,we can
gener-
alizethe
results
to
several
continuous
indices. In the
earlier
discussionwelookedat
j
(x)
as thexthcomponentof someabstractvector
If).
For
functions of n variables,
we can
think of
j(X!,
...
, x
n)
as the component of an abstract vector
If)
along a
basis vector Ix!,
...
, x
n
}.2 This basis is a direct generalization of one continuous
index to
n. Then
j(XI,
...
,x
n)
is defined as
j(X!,
...
, x
n)
= (Xl,
...
, X
n
I
f).
If
the region of integration is denoted by n,and we use the abbreviations
thenwe canwrite
If)
=
In
dnxj(r)w(r)
[r},
In
dnx [r)
w(r)
(r] = 1,
j(r')
=
In
dnxj(r)w(r)
(r']
r),
(r'l
r)
w(r)
=
8(r
-
r'),
(6.8)
where d"X is the "volume" element and n is the region
of
integration of interest.
For instance,
if
the region
of
definition of the functions under consideration is
the surface of the unit sphere, then [with
w(r)
= 1], one gets
r
dq,
fan
sinO
so
10,
q,HO,
q,1
=1.
(6.9)
2Donotconfusethiswithanu-dimensional
vector.
Infact,thedimension is a-fold
infinite:
each
xi
countsone
infinite
setof
numbers!

(6.10)
6.2
GENERALIZED
FUNCTIONS
165
This will be used in our discussion of spherical harmonics in Chapter 12.
An important identity using the three-dimensional Dirac delta function comes
from potential theory. This is (see [Bass 99] for a discussion
of
this equation)
2 ( 1 ) ,
V
--
=
-411"8(r-r).
Ir-r'l
6.2 Generalized Functions
Paul Adrian Maurice Dirac discovered the delta function in the late 1920s while
investigating scaltering problems in quantum mechanics. This "function" seemed
to violate most properties
of
otherfunctions knownto mathematicians at the time.
Furthermore, the derivative
of
the delta function, 8'(x -
x')
is such that for any
ordinary function
f(x),
i:
f(x)8'(x
-
x')dx
=-
i:
f'(x)8(x
-
x')
dx
=-
f'(x').
We can define 8'(x -
x')
by this relation.
In
addition, we can define the derivative
of any function, including discontinuous functions, at any point (including points
of discontinuity, where the usual definition
of
derivative fails) by this relation. That
is, if
cp(x) is a "bad"function whose derivative is not defined at some point(s), and
f(x)
is a "good" function, we can define the derivative
of
cp(x) by
i:
f(x)cp'(x)dx
==
-
i:
f'(x)cp(x)dx.
The integral on the RHS is well-defined.
Functionssuch as theDiracdeltafunctionand its derivatives of all orders are not
functions in the traditionalsense.
What
is commonamongall
of
them is thatin most
applications they appearinsidean integral, and we saw in Chapter1that integration
can be considered as a linear functional on the space
of
continuous functions.
11
is therefore natura1to describe such functions in terms of linear functionals. This
idea was picked up by LaurentSchwartz in the 1950s who developed it into a new
branch
of
mathematics called
generalized
functions, or distributions.
A distribution is a mathematical entity that appears inside an integral in con-
junction with a well-behaved
test
function-which
we assume to depend on n
variables-such
that the result of integrationis a well-definednumber. Depending
on the type
of
test function used, different kinds of distributions
can
be defined.
If
we want to include the Dirac delta function and its derivatives
of
all orders,
then the test functions must be infinitely differentiable, that is, they must
be e
oo
functions on R
n
(or C"), Moreover, in order for the theory of distributions to be
mathematicallyfeasible,all the test functions mustvanishoutsidea finite "volume"
of
R
n
(or C
n
).3
One common notation for such functions is e1i'(Rn) or e1i'(cn)
3S
uch
functions are
saidtobeof compactsupport.

166 6.
GENERALIZED
FUNCTIDNS
generalized
functions
and
distributions
defined
(F
stands for "finite").
The
definitive property
of
distributions concerns the way
they combine with test functions to give a number.
The
test functions
used
clearly
form
a vector space over R or C.
In
this vector-space language, distributions are
liuear functionals.
The
linearity is a
simple
consequence
of
the
properties
of
the
integral. We therefore have the following definition
of
a distribution.
6.2.1. Definition. Adistribution, orgeneralizedfunction, isa continuous" linear
functional on the space ef"(Rn) or
ef"(C
n).
Iff
E
ef"
and
<p
is a distribution,
then
<p[J]
= Coo'
,!,(r)f(r)
dnx.
Another notation
nsed
in
place
of
<p[J]
is
(<p,
f).
This' is more appealing not
only becanse
<p
is linear, in
the
sense that
<p[af
+
,Bg]
=
a<p[J]
+
,B<p[g],
bnt
also because the set
of
all such liuear functionals forms a vector space; that is,
the
liuear combination
of
the
<p's
is also defined. Thus,
(<p,
f)
suggests a mutual
"democracy" for both
!'s
and
<p's.
We now have a shorthand
way
of
writing integrals.
For
instance,
if
8
a
repre-
sents the Dirac delta function
8(x - a), with an integration over x understood,
then
(8
a
,
f)
=
f(a).
Similarly,
(8~,
f)
=
-/,(0),
and
for linear combinations,
(a8
a
+
,B8~,
f)
=
af(a)
- ,B/,(a).
6.2.2.
Example.
An ordinary(continnons)functinng coobe thonghtof asa specialcase
of a
distribution, The linear functional g :
ef"(R)
--+ Ris simpty definedby (g,
f)
ea
g[f]
=
1:"'00
g(x)f(x)
dx.
III
6.2.3.
Example.
An interesting applicationof distributions (generalizedfunctions)oc-
curs
whenthenotionof
density
is
generalized
to
include
notonly
(smooth)
volume
densities,
butalsopoint-like,linear, and
surface
densities.
Apointcbargeq locatedat
rO
coobethougbtofashavingacharge densityper) =
q8(r-
rO)'Inthelanguage oflinear functionals,weinterpretp asadistribution,p :
ef"
(E
3
)
--+ E,
whichforan
arbitrary
function
f gives
p[f]
= (p,
f)
=
qf(ro)·
(6.11)
Thedelta
function
character
of p canbe
detected
fromthis
equation
by
recalling
that
the
LHSis
On the RHS of this
equation,
the only volume element
that
contributes
is the one
that
contains
the point to: all the rest
contribute
zero. As b.Vi
-+
0, the only way
that
the
RHScangivea nonzero
number
is forp(rO)!(rO) tobe infinite.
Since!
is awell-behaved
function, p(rO) must be infinite, implying that per) acts as a delta function. This shows
thatthe definitionof Equation
(6.11) ieads to a delta-functionbehavior for p. Similarlyfor
linearand
surface
densities.
III
4See
[Zeidler,
95],pp.27. 156-160, fora
formal
definition
of the
continuity
of
linear
functionals.

"The
amount
of
theoretical
ground
one
has
to
cover
before
being
able
to
solve
problems
of
real
practical
value
is
rather
large,
but
this
circumstance
is
an
inevitable
consequence
of
the
fundamental
part
played
by
transformation
theory
and
is
likely
to
become
more
pronounced
inthe
theoretical
physics
of
the
future."
P.A.M.
Dirac
(1930)
6.2
GENERALIZED
FUNCTIONS
167
The example above and Problems 6.5 and 6.6 suggest that a distribution that
confines an integral to a lower-dimensional space mnst have a delta function in its
definition.
"PhysicalLaws should have mathematicalbeauty."Thisstatement
was
Dirac's
response to the question
of
his philosophy
of
physics,
posed to
him
in Moscow in 1955. He wroteit on a blackboard that
is still preserved today.
Paul
Adrien
Maurice
Dirac
(1902-1984), was
born
in 1902
in Bristol, England, of a Swiss, French-speaking father and an
English mother. His father, a taciturn
man
who refused to receive
friends at home, enforced young
Paul's
silence by requiring that
only
French
be spoken at the dinner table. Perhaps this explains
Dirac's later disinclination toward collaboration and his general
tendency to be a lonerin most aspects
of
his life.
The
fundamental
nature
of
his
work
madethe involvement
of
students difficult, so perhaps
Dirac's
personality
was well-suited to his extraordinary accomplishments.
Dirac
went
to Merchant Venturer's School,
the
public school where his father taught
French,
and
while there displayed great mathematical abilities.
Upon
graduation, he fol-
lowed in his older brother's footsteps and
went
to Bristol University to study electrical
engineering. He was 19
when
he graduated Bristol University in 1921. Unable to find a
suitable engineering position due to the economic recession that gripped post-World War I
England, Dirac accepted a fellowship to study mathematics at Bristol University. This fel-
lowship, together with a grant from the Department
of
Scientific
and
Industrial Research,
made it possible for Dirac to go to Cambridge as a research student
in 1923.
At
Cambridge
Dirac was exposed to the experimental activities
of
the
Cavendish Laboratory, and he be-
came
a
member
of
the intellectual circle over
which
Rutherford
and
Fowler presided. He
took his Ph.D.
in 1926 and was elected in 1927 as a fellow. His appointment as university
lecturer
came
in 1929. He
assumed
the
Lucasian
professorship following Joseph
Larmor
in 1932
and
retired from it in 1969. Two years laterhe accepted a position at Florida State
University where he lived out his remaining years.
The
FSU
library now carries his name.
In
the
late 1920s the relentless
march
of
ideas and discoveries
had
carriedphysics to a
generally acceptedrelativistic theory
of
the electron. Dirac, however, was dissatisfied with
the prevailing ideas and, somewhat in isolation, sought for a
better
formulation. By 1928
he succeeded
in finding an equation,
the
Diracequation,
that
accorded with his own ideas
and
also fit
most
of
the established principles
of
the time. Ultimately, this equation, and
the physical theory behind it, proved to
be one
of
the great intellectual achievements
of
the
period. It was particularly remarkable for the internal
beauty
of
its mathematical structure,
which not only clarified previously mysterious
phenomena
such as
spin
and the
Fermi-
Dirac
statisticsassociatedwith it,
but
alsopredictedthe existence
of
an electron-likeparticle
of
negative energy, the antielectron, or positron,and,
more
recently, it has
come
to
playa
. role
of
great importance in
modem
mathematics, particularly in
the
interrelations between
topology, geometry, and analysis.
Heisenberg
characterized the discovery
of
antimatter by
Dirac as "the
most
decisive discovery in connection with the properties or
the
nature
of
elementaryparticles
....
This discovery
of
particles and antiparticlesby
Dirac.
..
changed
our whole outlook on atomic physics completely."
One
of
the interesting implications
of

168 6.
GENERALIZED
FUNCTIONS
his
work
that
predicted
the
positron
wasthe
prediction
of amagnetic monopole.
Dirac
won
the Nobel Prize in 1933for this work.
Dirac
is not only one of thechief
authors
of
quantum
mechanics,
buthe is also the
creator
of
quantum
electrodynamics andone of the
principal
architects
of
quantum
field
theory.
While
studying
the
scattering
theory
of
quantum
particles, he
invented
the
(Dirac)
deltajunction; in his attempt at quantizing the geueral theory of relativity, he founded
constrained
Hamiltonian
dynamics,
whichis one of themost
active
areas
of
theoretical
physics
research
today.
Oneof his
greatest
contributions
is the
invention
of bra ( I
and
ket
I)·
Whileat
Cambridge,
Diracdidnot
accept
many
research
students.
Thosewho
worked
with him
generally
thought
that
he was a good supervisor; but one who didnot
spend
muchtimewithhis
students.
A
student
neededtobe
extremely
independent
to
work
under
Dirac.
Onesuch
student
was
Dennis
Sciama,
who
later
became
the
supervisor
of
Stephen
Hawking,
the
current
holder
of the
Lucasian
chair.
Salam
and
Wigner,
in
their
preface
tothe
Festschrift
that
honors
Diraconhis
seventieth
birthday
and
commemorates
his
contributions
to
quantum
mechanics
succinctly
assessedthe
man:
Diracis one of thechief
creators
of
quantum
mechanics
....
Posterity
will
rateDiracasoneofthe
greatest
physicists of alltime.The
present
generation
valueshimas one of its
greatest
teachers
..
..
Onthose
privileged
to know
him, Dirachas left his
mark
. .. by his
human
greatness.
He is
modest,
affectionate,
and
setsthehighestpossible
standards
of
personal
and
scientific
integrity.
Heisa legendinhis ownlifetimeand
rightly
so.
(Takenfrom Schweber, S. S. "Some chapters for a history of quantum field theory: 1938-
1952", in Relativity,
Groups,
and
Topology
II vol. 2, B. S. Dewitt and R. Stora, eds.,
North-Holland,Amsterdam, 1984.)
We
have
seen
thatthe
delta
functiottCattbe
thought
of
as
the
limit
of
att ordinary
function,
This
idea
can he generalized,
6.2.4. Defittition. Let
{'Pn
(x)} be a sequence
of
functions such that
n~i:
'Pn(x)f(x)dx
exists for all f E
e~(JR).
Then the sequence is said to converge to the distribution
'P,defined by
('P,
f)
= j}!'!"J:
'Pn(x)f(x)dx
Vf.
This convergence is denoted by
'Pn
->
'P.
For
example, it Cattbe verified
that
n 2 2
_e-
n
x
->
8(x)
.,fii
attd
1 -
cosnx
->
8(x)
nnx
2
and
so
Ott.
The
proofs
are
left
as exercises.

6.3
PROBLEMS
169
derivative
ofa 6.2.5. Definition. The derivative
of
a distribution
rp
is another distribution
rp'
distribution
defined by
(rp',
f)
= -
(rp,
f')
Vf E
e~.
6.2.6. Example. Wecan
combine
thelasttwo
definitions
toshow
that
ifthe
functions
en
are
defined
as
{
a
if x <
_1
B,,(x)
==
(nx + 1)/2 if
--k
~"~
s
k.
1 if x
~
k,
tbenB~(x)
-+
8(x).
Wewritethe
definition
of the
derivative,
(B~,
f) = -
(B
n
•
j'),
in
terms
of
integrals:
1
00
, 1
00
df
1
00
B,,(x)f(x)dx
= -
B,,(x)-dx
= -
Bn(x)df
-00 -00
dx
-00
=_(1-
1
/" B
n(X)d
f+l
l
/
n
Bn(x)df+
roo
Bn(X)d
f)
-00
-lin
11/n
= _
(0
+1
1
/"
nx
+I
df
+
roo
d
f)
-I/n
2 11/n
=
_::1
1
/
n
xdf
_
~
1
1
/ "
df
_
roo
df
2
-I/n
2
-I/n
11/n
=
-~
(xf(x)I~I~"
-
i:
f(X)dX)
I
-
2:
(f(lfn)
-
f(-I/n»
-
f(oo)
+
f(l/n).
Forlarge n. wehave
I/n
'"
0 and
f(±I/n)
'"
frO). Thus,
1
00
, n
(I
I I I 2 )
B,,(x)f(x)dx
'"
--
-
f(-)
+-
f(--)
-
-frO)
+frO)
'"
frO).
-00
2nnnnn
The
approximation
becomes
equality
in thelimitn
-+
00.
Thus,
1
00
, ,
lim
Bn(x)f(x)dx
= frO) = (80.
f)
=>
B
n
-+
8.
n"""*oo
-00
Note
that
/(00)
= 0
because
of the
assumption
that
all
functions
must
vanish
outside
a
finitevolume. II
6.3 Problems
6.1. Write a density function for two point charges q; and qz located at r =
rl
and r = r2. respectively.
6.2. Write a density function for four point charges
ql = q, q2 =
-q,
q3
= q
and q4 =
-q,
located at the comers of a square
of
side 2a, lying in the xy-plane,
whose center is at the origin and whose first
comer
is at (a.
a).

170 6.
GENERALIZED
FUNCTIONS
I
6.3. Show that 8
(f(x))
= 8(x - xo), where xo is a root of f and x is
1f'(xo)1
confined to values close to xo. Hint: Make a change
of
variable to Y =
f(x).
6.4. Show that
where the
Xk'S
are all the roots of f in the interval on which f is defined.
6.5. Define the distribution p : e
oo
(R
3)
--+
R by
(p,
f)
= f
<J(r)f(r)da(r),
S
where <J(r) is a smooth function on a smooth surface S in R
3
•
Show that
per)
is
zero
ifr
is not on S and infinite
ifr
is on S.
6.6. Define thedisttibution p : e
oo
(R
3)
--+
R by
(p,
f)
=
fc
)..(r)f(r)
di(r)
,
where )..(r) is a smooth function on a smooth curve C in R
3
•
Show that
per)
is
zero if r is not
00
C and infinite if r is on C.
6.7. Express the three-dimensional Dirac delta function as a product of three one-
dimensional delta functions involving the coordinates in
(a) cylindtical coordinates,
(b) spherical coordinates,
(c) general curvilinear coordinates.
Hint: The Dirac delta function in R
3
satisfies
JJJ
8
(r)d
3
x = 1.
6.8. Show that
J""oo
8'(x)f(x)
dx
= - f'(O) where
8'(x)
sa
!x8(x).
6.9. Evaluate the following integrals:
(a)
i:
8(x
2
- 5x
+6)(3x
2
-7x
+2)
dx.
(e)
roo
8(sin]fx)(~)X
dx.
1
0
.5
Hint: Use the result of Problem 6.4.
(b)
i:
8(x
2
-
]f2)cosxdx.
1
00
2
(d)
-00
8(e-
X
)lnxdx.
6.10. Consider IxIas a generalized function and find its derivative.

6.3
PROBLEMS
171
6.11. Let
~
E
eOO(JRn)
be a smooth function on
JR
n,
and let
<fJ
be a distribution.
Showthat
~<fJ
is alsoa distribution. Whatis thenaturaldefinitionfor
~<fJ?
Whatis
(~<fJ)/,
the derivative of
~<fJ?
6.12. Showthat eachof the following sequencesof functionsapproaches8
(x)
in
the senseof Definition6.2.4.
n 2 2
(a)
.,jiie-
n
x •
(b)
1-
cosnx
1tnx
2
(d)
sinnx.
nx
Hint:Approximate
<fJn(x)
forlargen andx
""
0, andthenevaluatethe appropriate
integral.
6.13. Showthat
~(1
+tanhnx)
-->
O(x) as n -->
00.
6.14. Show
thatx8'(x)
=
-8(x).
Additional Reading
1. Hassani,S.Mathematical Methods, Springer-Verlag, 2000.An elementary
treatmentofthe Dirac deltafunction with many examplesdrawnfromme-
chanicsand electromagnetism.
2. Rudin, W.
Functional Analysis, McGraw-Hill,1991.Part II of this mathe-
maticalbut(forthosewithastrongundergraduatemathematicsbackground)
veryreadablebookis devotedto the theory of distributions.
3. Reed, M. andSimon,B.
FunctionalAnalysis, AcademicPress, 1980.
7 _
Classical Orthogonal Polynomials
The last example of Chapter5 discussedonly one of the many types of the so-called
classicalorthogonalpolynomials. Historically, these polynomials were discovered
as solutions to differential equations arising in various physical problems.
Suchpolynomials can beproducedby startingwith I,
x, X
2,
...
and employing
the Gram-Schmidt process. However, there is a more elegant, albeit less general,
approach that simultaneously studies most polynomials of interest to physicists.
We will employ this approach.'
7.1 General Properties
for n = 0, 1,2,
...
,
Most relevant properties of the polynomials
of
interest are contained in
7.1.1.
Theorem.
Consider thefunctions
1 d
n
Fn(x) = w(x) dx
n
(uis")
where
1. F, (x) is a first-degreepolynomial in x,
(7.1)
2. s(x) is a polynomial in x
of
degree less than or equal to 2 with only real
roots,
3.
w(x)
is a strictly positive function, integrable in the interval (a, b), that
satisfiesthe boundaryconditions w(a)s(a) = 0 = w(b)s(b).
IThis
approach
is duetoF.G.
Tricomi
[Tric
55].See also[Denn 67].
