Hassani S. Mathematical Physics: A Modern Introduction to Its Foundations
Подождите немного. Документ загружается.


4.7
REAL
VECTOR
SPACES
131
(this is the fundamental theorem of algebra). A polynomial over the reals, on the
otherhand, does not necessarily have all its roots in the real number system.
It
may therefore seem that vectorspaces over the reals will not satisfythe nseful
theorems and results developed for complex spaces. However, through a process
called
complexification of a real vector space, in which an imaginarypartis added
to such a space, it is possible
to prove (see, for example, [Balm 58]) practically all
the results obtained for complex vector spaces. Only the resnlts are given here.
4.7.1.
Theorem.
Arealsymmetricoperatorhasaspectraldecompositionasstated
in Theorem4.4.6.
This theorem is especially useful in applications of classical physics, which
deal mostly with real vector spaces. A typical situation involves a vector that
is related to another vector by a symmetric matrix.
It
is then convenient to find
a coordinate system in which the two vectors are related in a simple mauner.
This involves diagonalizing the symmetric mattix by a rotation (a real orthogonal
matrix). Theorem 4.7.1 reassures us that such a diagonalization is possible.
4.7.2.
Example.
For a systemof N pointparticles constituting a rigid body,the total
angular momentum L =
'Lf::l
mj(rj
x Vi) is related to the angular frequency via L =
'Lf::l
mi[rj
x (w x Ii)] =
'Lf::l
mj[wfj
.
fj
-
fieri
. W)], or
(
Z;)
=
(~;:
~;~
t.,
I
zx
/zy
where
IX,)
(WX)
I
yz
Wy.
I
zz
{Oz
N
i..
=
L:mjC
rl-
xl>.
;=1
N
I
xy
=
-LmjXiYi,
i=1
N
I
yy
=L mi
(r1-
Y1),
i=1
N
[xz
= -
LmjXjZi.
i=1
N
I
zz
=
EmjC
r
l- zf),
i=1
N
I
yz
=-
LmiYiZi,
i=1
with I
xy
= I
yx
•
[xz
=
[zx.
and I
yz
=
flY'
The 3 x 3 matrix is denoted by I and is called the moment
of
inertia matrix. It is
symmetric,andTheorem4.7.1permitsits diagonalizationby anorthogonal transformation
(the counterpart of a unitary transformation in a real vector space). But an orthogonal
transformation in threedimensions is merely a rotation of coordinares.? Thus, Theorem
4.7.1 says that
it
is always possible to choose coordinate systems in which the moment of
inertia matrix is diagonal.
In such a coordinate system we have
Lx
= Ixxwx. L
y
= Iyywy,
and
L
z
= Izzwz,simplifying the equations considerably.
Similarly, thekineticenergyof therigidrotatingbody,
N. N N
T = L !mjVr = L
!miVj
. (w x
rj)
= L
!mjw.
(r,
x Vj) =
!W.
L = !wt!W,
i=1 i=1
i=1
71bis is not entirely true! There are orthogonal transformations that are composed
of
a rotationfollowedby a reflection about
the origin. See Example 3.5.8.

132 4.
SPECTRAL
DECOMPOSITION
which in general has off-diagonal terms involving I
xy
and so forth, reduces to a simple
&.
I 2
II
2
II
2 III
rorm: T =
2.
Ixxw
x
+
2"
yyW
y
+ '2 zzw
z·
4.7.3.
Example.
Another application
of
Theorem 4.7.1 is in the study
of
conic sections.
The most general form of theequation of a conic section is
alx
2
+
azy2
+
a3xy
+a4x +a5Y +a6 = 0,
where
aI,
...
, a6 are constants. ITthe coordinate axes coincide with the principal axes of
the conic section, the
xy
term will beabsent, and the equation
of
the conic section takes
the familiar form, On geometrical grounds we have to be able to rotate xy-coordinates to
coincide with the
principalaxes. We shall do this using the ideas discussed in this chapter.
First, wenotethatthegeneralequationfora conic sectioncanbe writtenin matrixform
as
(x
as)
(~)
+a6 =
o.
The 2 x 2 matrixis symmetricandcanthereforebe diagonalizedby meansofan orthogonal
matrix R. Then R/R = 1, and we can write
Let
a3/2) Rt = (ai
a2 0
Then we
get
(x' y') (aJ
~)
(;:)
+(a
4
a~)
(;;)
+a6 = 0;
or
"2
"2
""
0
alx
+a2Y
+a
4
x
+asy
+a6
= .
The cross term has disappeared. The orthogonal matrix R is simply a rotation. In fact,
it rotates the original coordinate system to coincide
with
the principal axes
of
the conic
section. II
4.7.4.
Example.
In this example we investigate conditions under which a multivariable
function has a maximum or a minimum.
A point a
=
(aI,
a2,
...•
an) E lR
n
is a maximum (minimum)
of
a function
f(xj,
X2,
...
, xn) ee
f(r)
if
vflx,=a, =
(aa
f
,
aa
f
,
...
,
aa
f
)
= 0
Xl x2 xn
Xj=aj

4.7
REAL
VECTOR
SPACES
133
and for small Xi -
ai,
the difference
fer)
-
/(a)
is negative (positive). To relate this
difference to thetopicsof thissection, write theTaylorexpansionofthe functionarounda
keeping terms
up
to the second order:
n
al
1 n ( a
2
I )
l(r)=/(a)+L(Xi-ai)(-a.)
+-2
L(Xi-
ai)(Xj-aj)
-a·a·
+... ,
i=l
X,
r=a
i,j
XI XJ
r=a
or, constructing a column vector
out
of
8j ea Xi - ai
and
a symmetric matrix
Dij
out
of
the
second derivatives, we
can
write
1 t
I(r)
-
I(a)
=
-8
08 +...
2
because
the
first derivatives vanish.
For
a to be a
minimum
point
of
f,
the
RHS
of
the last
equation must be positive for arbitrary 8. This means that D must be a positive matrix.
S
Thus, all
its eigenvalues mustbe positive (Corollary4.4.8). Similarly, we can show
that
for
a to be a
maximum
point
of
f.
-0
must
be positive definite.
This
means
that
D
must
have
negative eigenvalues.
Whenwespecialize theforegoing discussion totwodimensions, weobtainresultsthat
are familiar
from
calculus.
For
the
function f (x, y) to
have
a minimum, the eigenvalues
of
the
matrix
(
Ixx Ixy)
Iyx
Iyy
must be positive.
The
characteristic polynomial
det
(Ixx - A
Iyx
Ixy
)
=0
Iv»
-A
yields two eigenvalues:
Ixx
+I
yy
+JUxx - l
yy
)2
+4!A
At = 2 '
Ixx
+I
yy
- JUxx - l
y
y)2
+4!ly
1.2 = 2 .
These eigenvalues will be both positive
if
Ixx
+I
yy
> jUxx:-
/yy)2
+4!ly.
and
both
negative
if
Ixx
+
Ivv
<
-JUxx
-
lyy)2
+41;y.
Squaring these iuequalities and simplifying yields
2
Ixxlyy > I
xY
'
SNote that Dis already
symmetric-the
real analogue of hermitian.

134 4.
SPECTRAL
DECOMPOSITION
which showsthat
fxx
and
/yy
musthave the same sign. ITtheyare both positive (negative),
wehaveaminimum (maximum).This is the familiarconditionforthe attainmentofextrema
by a function of two variables. II
Although the establishment
of
spectral decomposition for symmetric opera-
tors is fairly straightforward, the case
of
orthogonal operators (the counterpart
of
unitary operators in a real vector space) is more complicated.
In
fact, we have
already seen in Example 4.5.1 that the eigenvalues
of
an orthogonal transforma-
tion in two dimensions are,
in general, complex. This is in contrast to symmetric
transformations,
Think
of
the orthogonal operator 0 as a unitary operator.? Since the absolute
value
of
the eigenvalues
of
aunitary operatoris I, the only realpossibilities are ±I.
To find the other eigenvalues we note that as a unitary operator. 0 can be written
as
e
A
,
where Ais anti-hermitian (see Problem 4.22). Since hermitian conjugation
and transposition coincide for real vector spaces, we conclude that A
=
_At,
and
A is antisymmetric.
It
is also real, because 0 is.
Let us now consider the eigenvalues
of
A.
If
A is an eigenvalue
of
A corre-
sponding to the eigenvector
la), then (al
Ala)
= A
(al
a). Taking the complex
conjugate
of
both sides gives (al
At
la) = A*
(al
a);
but
At
= At =
-A.
because
A is real and antisymmetric. We therefore have
(alAla)
=
-A*
(ala),
which
gives
A* =
-A.
It
follows that
if
we restrict A to be real. then it can only be zero;
otherwise. it must be
purely imaginary. Furthermore, the reader may verify that
if
Ais an eigenvalue
of
A. so is
-A.
Therefore, the diagonal form
of
Alooks like this:
Amag = diag(O. 0,
...•
O.WI.
-WI.
ifh.
-ilh
•...•Wk,
-iek).
which gives 0 the following diagonal form:
Od
' = e
Adiag
= diag(e
O
eO eO
eifft e-i91 eilh
e-ifh
eiOk
e-Uh)
lag , ,
...
" , , , ,
.••
, ,
with el •fh•....ekall real.
Ilis
clearthat if 0 has
-I
as an eigenvalue, then some
of
the e's
must
equal
±1f.
Separating the
1f'S
from the rest
of
e's and putting all
of
the above arguments together, we get
O'
- diagt l 1 1
-1 -1 -1
Uft
-Uh
i(h
-iBz
iBm
-iBm)
dlag-
, ,
...
,
,~,e
,e
,e,e
,
...
,e
,e
N+ N_
whcre
v.,
+N_
+2m
= dimO.
Getting insightfrom Example4.5.1, we can argue. admittedlyin a nonrigorous
way. that corresponding to eachpair
e±iBj is a 2 x 2 matrix
of
the form
-Sine})
==
R (e.)
COse}
2 J
(4.17)
9This can always be done by formally identifying transposition with hermitian conjugation, an identification that holds when
the underlying field of numbers is real.

4.7
REAL
VECTOR
SPACES
135
We therefore have the following theorem (refer to [Hahn 58] for a rigorous treat-
ment).
4.7.5.
Theorem.
A real orthogonal operator on a real inner product space V
cannot, in general, be completelydiagonalized. The closestit can get to a diagonal
form is
Odiag
=
diag(l,
1,
...
,1",-1,
-1,
...
,
-1,
R2(11j), R2(!h),
...
,
R2(e
m
))
,
where N+ +N_ +2m =
dim
V and
R2(ej)
is as given in (4.17). Furthermore,
the matrix that transforms an orthogonal matrix into the form above is itselfan
orthogonal matrix.
The last statement follows from Theorem 4.5.2 and the fact that an orthogonal
matrix is the real analogue
of
a unitary matrix.
4.7.6.
Example.
AninterestingapplicationofTheorem
4.7.5
occursinclassical
mechan-
ics,whereitisshown
that
themotionofarigidbodyconsistsof a
translation
anda
rotation.
'The
rotation
is
represented
bya 3 x 3
orthogonal
matrix.
Theorem
4.7.5
states
that
byan
appropriate
choiceof
coordinate
systems(l.e., by
applying
thesame orthogonal
transfor-
mation
that
diagonalizes
the
rotation
matrix
of therigidbody),one can
"diagonalize"
the
3 x 3
orthogonal
matrix.
The
"diagonal"
formis
o
±l
o
or
o
cosB
sinO
-s~e)
.
cosB
Excluding
the
reflections
(corresponding
to
-1
's)
and
the
trivial
identity
rotation,
we con-
clude
that
any
rotation
of arigidbodycanbe
written
as
(
I 0
o cosB
o sine
-s~e)
,
cosO
whichis a
rotation
through
theangleB
about
the(new)x-axis.
III
Combining the rotation of the example above withthe translations, we obtain
the following theorem.
4.7.7.
Theorem.
(Euler) The general motion
of
a rigid body consists
of
the trans-
lation
of
one point
of
that body
and
a rotation about a single axis through that
point.
Finally, we quote the polar decomposition for real innerproduct spaces.
4.7.8.
Theorem.
Any operator A on a real inner product space can be written as
A = OR, where R is a (unique) symmetric positive operatorand 0 is orthogonal.

136 4.
SPECTRAL
DECOMPOSITION
4.7.9.
Example.
Let us decompose the following matrix intoits polarform:
The
procedure
is thesameasin thecomplexcase. Wehave
2 t
(2
3)(2
0)
(13
R = A A= 0
-2
3
-2
=
-6
witheigenvalues
A.l
= 1 and).2 = 16and
normalized
eigenvectors
and
The
projection
operators
are
PI =
le1)(eIl
=
~
G
;),
Thus,
we have
-2)
1.
Wenote
that
Ais
invertible.
Thus,Ris also
invertible,
and
R-
I
=
2~
(~
I~)'
Thisgives0 = AR-
1,
or
Itisreadily
verified
that
0 is indeed
orthogonal.
-2)
=
~
(17
1
5-6
-6)
8 .
III
Our excursion through operator algebra and matrix theory bas revealed to us
the diversity of diagonalizable operators. Could it be perhaps that all operators are
diagonalizable?
In
other words, given any operator, can we find a basis in which
the matrix representing that operator is diagonal? The answer is, in general, no!
(See Problem 4.27.) Discussion of this topic entails a treatment
of
the Hamilton-
Cayly theorem and the Jordan canonical form of a matrix, in which the so-called
generalized eigenvectors are introduced. A generalizedeigenvectorbelongs to the
kernel
of
(A - A1)m for some positive integer m.
Then
A is called a generalized
eigenvalue. We shall not pursue this matter here. The interested reader can find
such a discussion in books on linearalgebraand matrix theory. We shall, however,
seethe applicationof this notionto specialoperatorson infinite-dimensionalvector
spaces in Chapter 16. One result is worth mentioning at this point.
4.7.10. Proposition.
If
the roots
of
the characteristic polynomial
of
a matrix are
all simple, then the matrix can be brought to diagonal form by a similarity (not
necessarily a unitary) transformation.
4.7
REAL
VECTOR
SPACES
137
4.7.11.
Example.
As a finat example of the application of the results of this section, tet
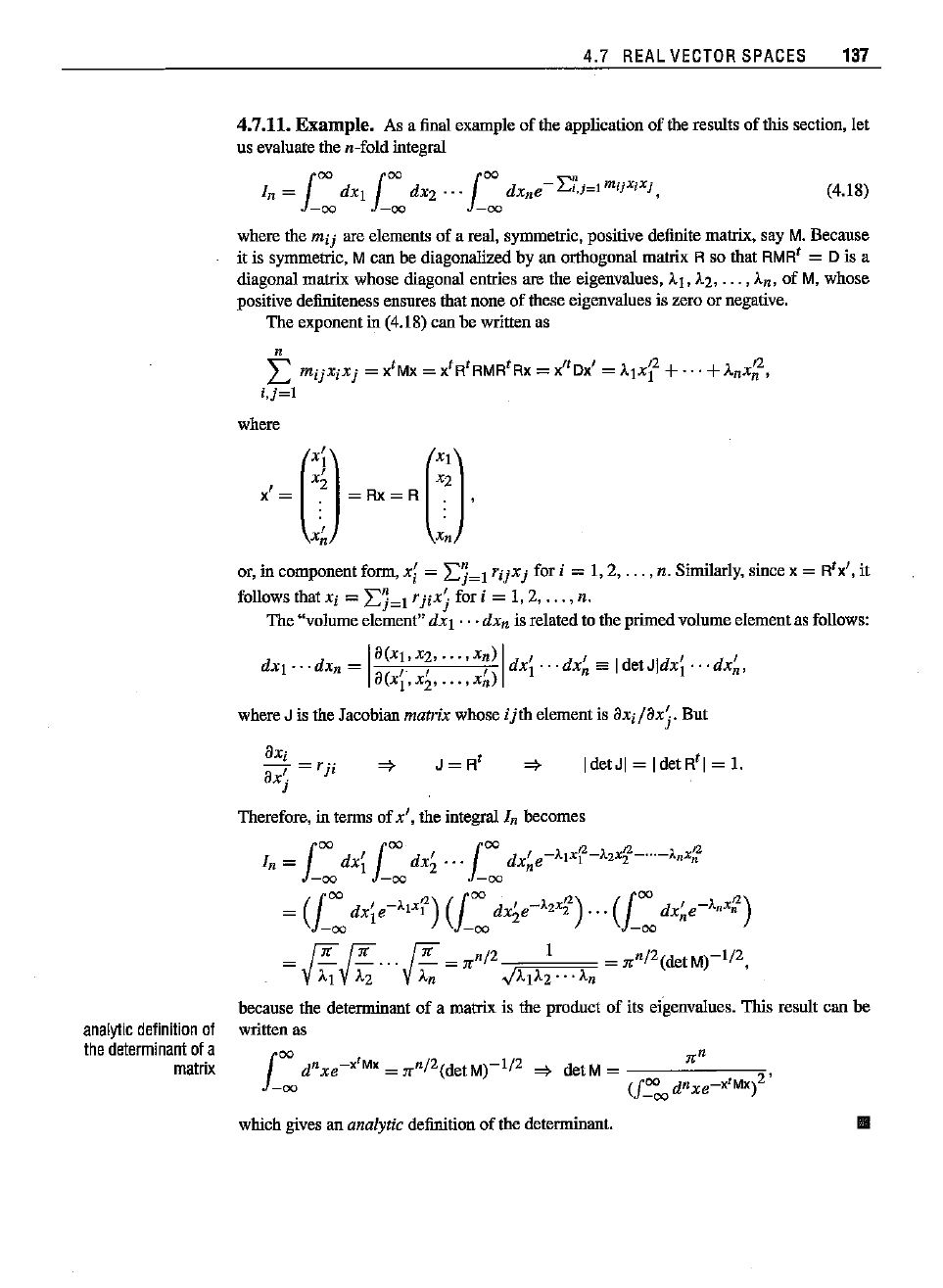
us evaluate the n-fold integral
1
00
100
1
00
-'"
In =
dXl
dX2
...
dXn
e
- L..i,j=l
lnijXiXj,
-00
-00
-00
(4.t8)
[det J] = IdetR'1 =
I.
III
analytic
definition
of
the
determinant
ofa
matrix
where the
mij
are elements of a real, symmetric, positive definite matrix. say
M.
Because
it is symmetric, M can be diagonaIized by an orthogonal matrix R so that
RMR
t
=D is a
diagonal matrix whose diagonal entries are the eigenvalues, AI.
).,2,
...
, An,of M,whose
positive definiteness ensures
that
none
of
these eigenvalues is
zero
or negative.
The
exponent
in (4.18)
can
be written as
n
L
mijXjXj
= xtMx
=xtRtRMRtRx
= xttDx' =
)"1X12
+...
+).nx~2.
i,j=l
where
or, in component form, x; =
L:j=l
TijX
j for t =
1,2,
...
,n. Similarly, since x = Rtx'. it
follows that Xi =
LJ=l
rjixj
for i =
1,2,
...
, n.
The "volume element"
dx!
dXn is related to the primedvolume elementas follows:
1
8
(X
l>
X2,
,xn)1
I I I d I
dXl···dxn
=
f"'
f dX
l
···dx
n
es
IdetJldxl
...
x
n'
8
(xl'
x
2
. · · · ,
x
n)
where J is the Jacobian matrix whose
ijth
element is
8xi/8xj.
But
8Xi
8'
=rji
x
j
Therefore, in terms of
s',
the integral In becomes
In = 1
00
dx~
l°Odx~
...
1
00
dx~e-J..IX?-J..2Xq--
..
·-J..nX~2
-00
-00
-00
=
(/_:
dx~e-J..1X?)
(i:dx2e-J..2X22)
...
(i:
dx~e-J..nX~)
=
~ ~
...
~
= "n/2 1 =
"n/2(detM)-t/2,
VAt V1.2
vi;.
.JA1A2"·
An
because the determinant of a matrix is the product of its eigenvalues. This result can be
written as
1
00
t nn
dnxe-
X
Mx
=
"n/2(detM)-1/2
=}
detM = ,
-00
(J~oo
d
n
xe-
xt MX
)2
which gives an analytic definition of the determinant.

138 4.
SPECTRAL
DECOMPOSITION
4.8 Problems
4.1. Let 11
j
and
11z
be subspaces
of
V. Show that
(a) dim(l1j + 11z)= dim
111
+ dim
11z
-
dim(111
n11z).Hint: Extend a basis of
111
n
11z
to both
111
and
11z.
(b) If111 +
11z
= Vand dim
111
+ dim
11z
= dim V,then V=
111
m11z.
(b)
If
dim
111
+ dim
11z
> dim V,then
111
n
11z
oF
{OJ.
4.2. Let P be the (hermitian) projection operator onto a subspace
Jye
Show that
1 - P projects onto M1-. Hint: You
need
to show that (ml
Pia)
= (ml a) for
arbitrary
la) and
1m)
E M; therefore, consider (ml P la)*, and use the hermiticity
ofP.
4.3. Show that a subspace M
of
an inner product space V is invariant under the
linear operator
A
if
and only if M1-is invariant under At.
4.4. Show that the intersection
of
two invariant subspaces
of
an operator is also
an invariant subspace.
4.5.
Let
n be apermutation of the integers
(I,
2,
...
, nj. Findthe spectrum of An,
if
for Ix) =
(aj,
az,
...
,an)
E
en,
we define
An [x) =
(an(I),
...
, an(n»)'
4.6. Show that
(a) the coefficient of
J..N
in the characteristic polynomial is
(_l)N,
where N =
dim V, and
(b) the constant in the characteristic polynomial
of
an operatoris its determinant.
4.7. Operators
A and B satisfy the commutationrelation [A, B] = 1. Let Ib)be an
eigenvector
of
B with eigenvalue
J...
Show that
e-'fA
Ib) is also an eigenvector
of
translation
operator
B,but witheigenvalue
J..
+ r , This is why
e-'fA
is called the
translation
operator
for B. Hint: First find [B,
e-'fA].
4.8. Find the eigenvalues of an
involutive
operator, that is, an operator A with the
property A
Z
= 1.
4.9. Assume that A and A' are similar matrices. Show that they have the same
eigenvalues.
4.10.
In each of the following cases, determine the counterclockwise rotation
of
the xy-axes that brings the conic sectioninto the standard form and determine the
conic section.
(a)
llx
z
+
3l
+
6xy
- 12 = 0
(e) 2x
z
<s">
4xy
- 3 = 0
(e) 2x
z
+
sl-
4xy
- 36 = 0
(b) Sx
Z
- 3
yz+6xy+6=O
(d)
6x
z
+
3l-
4xy
- 7 = 0
4.B
PROBLEMS
139
4.11. Show that
if
A is invertible, then the eigenvectors
of
A-I
are the same as
those
of
A and the eigenvalues
of
A
-I
are the reciprocals
of
those
of
A.
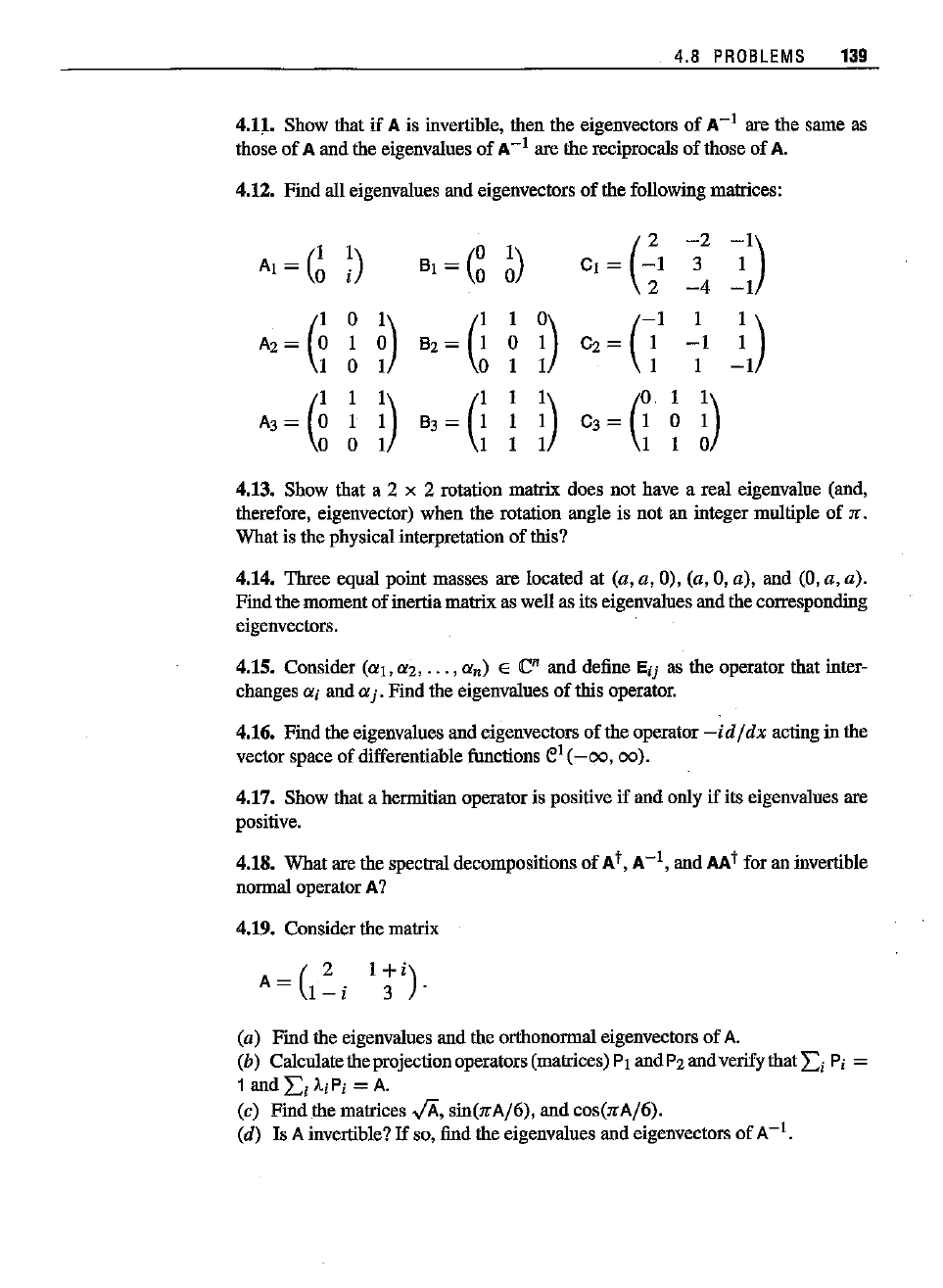
4.12. Find all eigenvalues and eigenvectors
of
the following matrices:
Al =
(~
~)
81 =
(~
~)
Cl=
CI
-2
~I)
3
-4
-I
A2=
G
0
~)
82=
G
I
D
C
I I
JJ
I 0
C2 = :
-I
0 I
I
A3=
G
I
D
83 = G
I
D
(
I
D
I I
C3 = :
0
0 I I
4.13. Sbow that a 2 x 2 rotation matrix does not have a real eigenvalue (and,
therefore, eigenvector) when the rotation angle is not an integer multiple
of
tt
.
What is the physicalinterpretation
of
this?
4.14. Three equal point masses are located at
(a, a, 0), (a, 0, a), and (0, a, a).
Findthe moment
of
inertiamatrix as well as its eigenvalues and the corresponding
eigenvectors.
4.15. Consider
(aI,
a2,
...
,
an)
E
en
and define
Eij
as the operator that inter-
changes
a: and ai- Find the eigenvalues
of
this operator.
4.16. Findthe eigenvalues and eigenvectors
of
the operator
-id/dx
acting in the
vector space
of
differentiable functions e
l
(-00,00).
4.17. Show that a hermitian operatoris positive
if
and only if its eigenvalues are
positive.
4.18.
What
are the spectral decompositions
of
At, A
-I,
and AAt for an invertible
normal operatorA?
4.19. Considerthe matrix
I +
i)
3 .
(a) Find the eigenvalues and the orthonormal eigenvectors
of
A.
(b) Calcnlatethe projectionoperators(matrices) PI andP2andverifythat
Li
Pi =
1 and
Li
AiPi = A.
(c) Find the matrices.,fA,
sin(nA/6),
and
cos(nA/6).
(d) Is Ainvertible?
If
so, find the eigenvalues and eigenvectors
of
A-I.

140
4.
SPECTRAL
OECOMPOSITION
4.20. Consider the matrix
A=
(~i
~
~}
(a)
Find
the eigenvalnes of A.Hint: Try A= 3 in the characteristic polynomial
of
A.
(b) For each A,find a basis for
M,
the eigenspace associated with the eigenvalue
A.
(c) Use the Gram-Schmidt process to orthonormalize the above basis vectors.
(d) Calculate the projection operators (matrices) Pi for each subspace and verify
that
LiPi =1 and LiAiPi =A.
(e) Find the matrices.,fA, sin(rrAj2), and cos(rrAj2).
(f)
Is Ainvertible?
If
so, find the eigenvalues and eigenvectors of
A-I.
4.21. Show that
if
two hermitian matrices have the same set of eigenvalues, then
they are unitarily related.
4.22. Prove that corresponding to every unitary operator U acting on a finite-
dimensional vector space, there is a hermitian operator H such that U
= exp iH.
4.23. Find the polar decomposition of the following matrices:
(
2i
0)
A="fi3'
(
41
B = 12i
-12i)
34 '
(
1 0
c=
0 1
I i
4.24. Showthat an arbitrary matrix Acan be "diagonalized" as D =
UAV,
where U
is unitary and Dis a real diagonal matrix with only nonnegative eigenvalues. Hint:
Consider
AA
t.
4.25. Show that (a)
if
A is an eigenvalue of an antisymmetric operator, then so
is
-A,
and (b) antisymmetric operators (matrices)
of
odd dimension cannot be
invertible.
4.26. Find the unitary matrices thatdiagonalize the following hermitianmatrices:
Al =
C1
2
_i
-1+
i)
A2=C·
;)
,
A3 = G
-i)
-1
'
-,
o '
BI =
(~I
-1
~)
B2 =
(~.
0
~i)
.
0
, ,
-1
-i
-I
-,
Warning! You may have to resort to numerical approximations for some of these.
4.27.
For
A =
(b
1),where x
t=
0, show that it is impossible to find an invertible
2 x 2 matrix Rsuch that RAR-
1
is diagonal. (This shows that not
all operators are
diagonalizable.)
