Gottstein G., Shvindlerman L.S. Grain Boundary Migration in Metals: Thermodynamics, Kinetics, Applications

Подождите немного. Документ загружается.

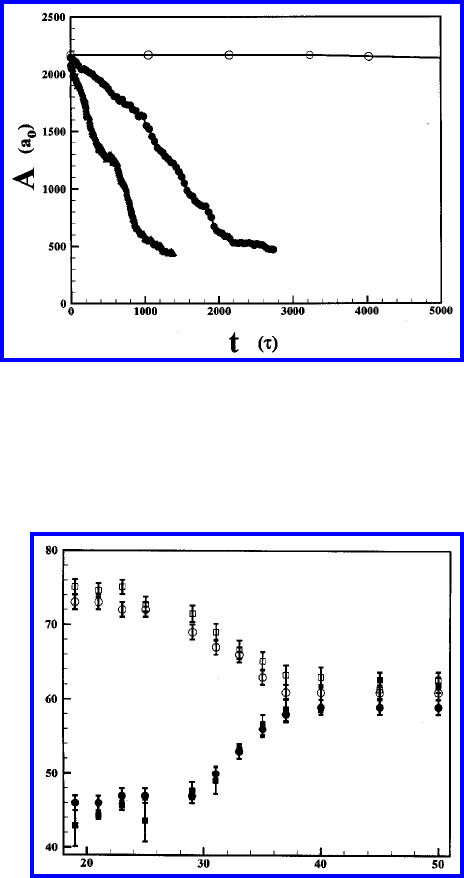
506 5 Computer Simulation of Grain Boundary Motion

FIGURE 5.41

The rate of change in the area of the half-loop grain with and without triple

junctions for the same conditions as in Fig. 5.39. The ﬁlled and open circles

indicate the “+” and “–” geometries, respectively, and the shaded triangles

indicate the results of the bi-crystal simulation [440].

θ (

)

a (

)

θ (

)

a (

)

FIGURE 5.42

The dynamic triple junction angle θ

plotted as a function of the half-loop

grain width a, for both the “+” (ﬁlled symbols) and “–” (open symbols)

geometries and for simulation conditions ϕ =38.2

◦

and T =0.125ε/k

The circles indicate the directly measured dynamic angles while the squares

correspond to θ

−

from Figs. 5.41(a) and (b) [440].

5.9 Simulation of Triple Junction Motion 507

a low of 60

◦

± 1

◦

toahighof74

◦

± 1

◦

for the “–” geometry. Of more im-

portance than the absolute value of the dynamic triple junction angle is the

deviation of this angle from its equilibrium value |θ

−θ

|. While little or no

deviations (within the error bars) of the dynamic triple junction angles from

the static value of 60

◦

are common in the simulation performed, signiﬁcant

deviations do occur at or very near low Σ misorientations: |θ

−θ

| =16

◦

and

|θ

−

−θ

| =14

◦

for Σ13 (ϕ =32.2

◦

)and|θ

−θ

| =13

◦

and |θ

−

−θ

| =1

◦

for Σ7 (ϕ =38.2

◦

). The observation of large deviations of the dynamic angle

from their equilibrium values appears strongly correlated with large devia-

tions of

from

The variation of the dynamic angle θ

(as measured from ﬁgures such as

Figs. 5.36 and 5.37) with half-loop width is shown in Fig. 5.42. As the half-

loop width increases, the deviation θ

− θ

decreases for both the “+” and

“–” geometries. For widths above approximately 40r

, the dynamic angle θ

is nearly indistinguishable from the static equilibrium value θ

=60

◦

± 1

◦

These data suggest that at small half-loop width triple junction drag is sig-

niﬁcant.

Fig. 5.43 shows the variation of θ

with temperature T ,whereθ

has been

directly measured from images of the migrating triple junction and deduced

from Figs. 5.39(a) and (b). Within the error bars of the determination of θ

both methods yield the same values. As the temperature increases, the devi-

ation of the dynamic angle from the static angle |θ

−θ

| decreases. Similar

trends are also observed for the “–” geometry of Fig. 5.40(b). Substantial

deviations of θ

from its equilibrium value θ

only occur for special misori-

entations, small grain size and relatively low temperature.

The results show that for low Σ misorientations and misorientations near

these, the dynamic triple junction angles deviate signiﬁcantly from their equi-

librium values. This results in signiﬁcantly slower boundary migration in the

tri-crystal geometries, as indicated by the extracted values of the rates of

change in area of the half-loop grain

The simulations conﬁrm the experimental observations of non-equilibrium

triple junction angles and substantial triple junction drag seen in recent exper-

iments [183, 436]. One discrepancy between the experiments and simulations

is the conditions under which triple junction drag is signiﬁcant. In the simu-

lations, triple junction drag was never found to substantially retard boundary

migration at grain sizes above approximately ﬁfty inter-atomic spacings. On

the other hand, the experiments have demonstrated triple junction drag for

grain sizes in excess of 10 μm. This diﬀerence is likely attributable to the

presence of impurities on the grain boundaries in the experiments, while the

simulations model (intrinsic) migration in an ideally pure material. This sug-

gests that impurity eﬀects, even in extremely high purity materials, may sub-

stantially inﬂuence grain boundary and triple junction migration because of

segregation eﬀects. Such an explanation is meaningful since impurities aﬀect

the triple junction and grain boundary mobility diﬀerently. Nonetheless, it is

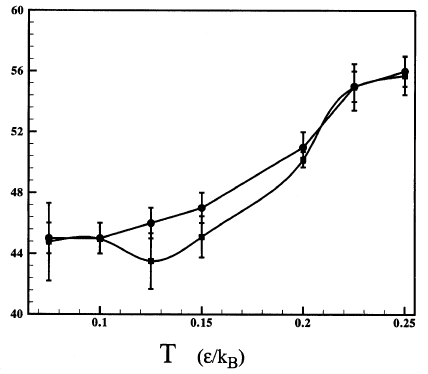
508 5 Computer Simulation of Grain Boundary Motion

θ (

)θ (

)

FIGURE 5.43

The dynamic triple junction angle β

vs. temperature T , for the “+” geometry

and for simulation conditions ϕ =38.2

◦

and T =0.125ε/k

.Thecircles

indicate the directly measured dynamic angles while the squares correspond

to θ

from Figs. 5.41(a) and (b) [440].

emphasized that according to experimental results and computer simulation

data that triple junction drag must always be considered for the thermal be-

havior of modern nano-structured materials.

Applications

“Pooh felt that he ought to say something helpful

about it, but didn’t quite know what. So he decided

to do something helpful instead.”

— A.A. Milne

6.1 Characterization of Microstructure and Texture

6.1.1 Introductory Remarks

Because of the complexity of microstructures in crystalline solids, it is vir-

tually impossible to comprehensively characterize and, accordingly, represent

microstructures. The microstructure of commercial materials like hardened

steels or heavily deformed metals consists of complex morphological features,

defect arrangements and phase distributions even under high magniﬁcation,

i.e. on a nanoscale level.

For many applications only the macroscopic behavior of a material is of

practical interest and, therefore, the common approach in the past was the

correlation of macroscopic properties with average values of dominant mi-

crostructural features, usually features to be identiﬁed by optical microscopy,

like grain size and macroscopic phase distribution besides overall chemistry.

In crystalline solids the macroscopic properties are not necessarily isotropic,

i.e. equal for all specimen directions. This is due to the fact that the individual

crystals are non-random arrangements of atoms. On the contrary they con-

sist of strictly aligned atoms on a long-range periodic pattern. Therefore, the

physical properties of a crystal depend on the considered crystal direction, i.e.

physical properties of crystallites are anisotropic, which requires their repre-

sentation by a second rank tensor. A crystalline solid usually consists of many

crystallites (typically 10

/mm

). If the grains in a polycrystal are randomly

arranged, then the anisotropic properties of the individual crystallites aver-

age out, and the polycrystal behaves isotropically. During the processing of

a material to the ﬁnal product, however, usually non-random distributions of

crystallite orientations are generated, which cause an anisotropic behavior of

509

510 6 Applications

the macroscopic specimen. Therefore, in addition to the grain size and shape

as well as the phase distribution and morphology, the orientation distribution

is also of interest for macroscopic material behavior. The orientation distribu-

tion in crystalline solids is referred to as crystallographic texture, or simply

texture

The texture of a material is by deﬁnition a macroscopic average of the ori-

entations in a polycrystalline aggregate (macrotexture). On a more local scale,

the orientation distribution (microtexture) may be diﬀerent from the macro-

scopic average, if orientation correlations exist, i.e. clustering of orientations

or preferred next neighbors. Evidently, in deformed microstructures the ori-

entation distribution in deformation inhomogeneities may be quite diﬀerent

from the homogeneously deformed matrix.

While the macrotexture determines the anisotropy of a material property,

the microtexture provides information on the mechanisms of material behavior

and microstructure evolution. More speciﬁcally, the microtexture encompasses

the grain boundary character distribution so that all mechanisms involving

grain boundary processes will be primarily aﬀected by this microtextural char-

acteristic.

In the framework of this text we will consider the eﬀect of grain boundary

motion on microstructure and texture development. Correspondingly, we shall

conﬁne microstructure characterization mainly to grain structures in single

phase materials, i.e. characterized by grain size distribution and morphology

as well as crystallographic texture, both on a macroscopic and microscopic

level. The main features of both characterization groups, grain structure and

texture, are textbook knowledge and thus will be only concisely reviewed in

the following.

6.1.2 Microstructure

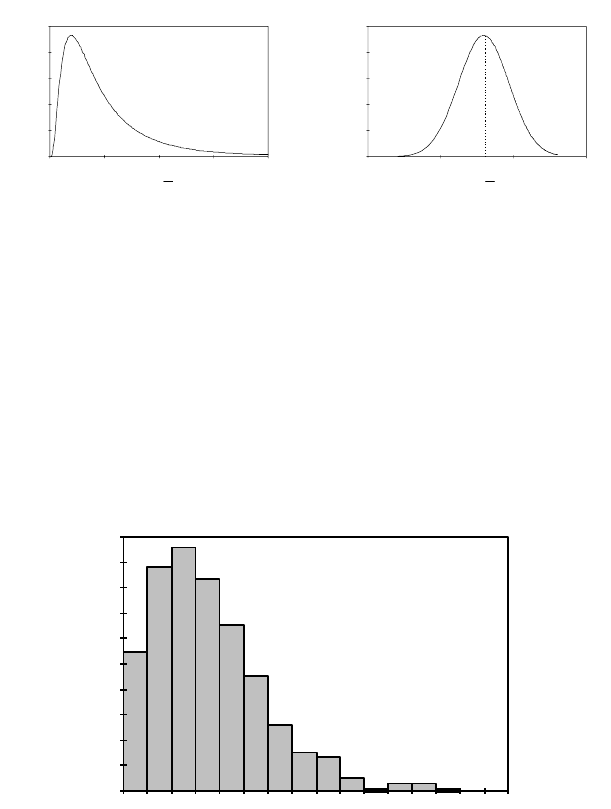
The grain size y of a crystalline solid is practically never uniform; rather, there

is a grain size distribution Ψ (Fig. 6.1), which usually can be described by a

log-normal distribution

Ψ(y)dy =

√

2πσ

exp

⎛

⎜

⎝

−

⎡

⎣





⎤

⎦

⎞

⎟

⎠

d (ln y) (6.1)

We note at this point that the term texture is not understood unambiguously among the

disciplines concerned with crystalline solid. While in the materials science community tex-

ture is synonymous with crystallographic texture, in mineralogy or geology the term texture

stands for the morphological appearance of the usually complex minerals or rocks. There-

fore, geologists choose the term “preferred orientation” rather than texture. Of course, there

is also texture in textile fabrics, food and art with quite diﬀerent meanings, but because

we are so far removed from these disciplines there is no need to diﬀerentiate meanings. We

shall refer to texture as crystallographic texture in the following.

6.1 Characterization of Microstructure and Texture 511

Häufigkeit w(D)

1,0

0,8

0,6

0,4

0,2

1234

(D/D

)

Häufigkeit w(D)

1,0

0,8

0,6

0,4

0,2

0,01 0,1 1 10(ln D)

ln (D/D

)

(a) (b)

frequency w(D)

1.0

0.8

0.6

0.4

0.2

1234

frequency w(D)

1.0

0.8

0.6

0.4

0.2

0.01 0.1 1 10

(a) (b)

DD/

Häufigkeit w(D)

1,0

0,8

0,6

0,4

0,2

1234

(D/D

)

Häufigkeit w(D)

1,0

0,8

0,6

0,4

0,2

0,01 0,1 1 10(ln D)

ln (D/D

)

(a) (b)

Häufigkeit w(D)

1,0

0,8

0,6

0,4

0,2

1234

(D/D

)

Häufigkeit w(D)

1,0

0,8

0,6

0,4

0,2

0,01 0,1 1 10(ln D)

ln (D/D

)

(a) (b)

frequency w(D)

1.0

0.8

0.6

0.4

0.2

1234

frequency w(D)

1.0

0.8

0.6

0.4

0.2

0.01 0.1 1 10

(a) (b)

DD/

FIGURE 6.1

(a) Logarithmic normal distribution as given by Eq. (6.1) plotted linearly

(normalized to mean grain size

D). The maximum is not at D/

D =1.(b)

Plot of the distribution in (a) over the logarithm of grain size. The distribution

is symmetrical.

201816

12108642

22 24 26 28 30 32

frequency

D [μm]

201816

12108642

22 24 26 28 30 32

frequency

D [μm]

FIGURE 6.2

Histogram of grain size distribution in recrystallized Fe-17%Cr commercial

sheet metal (70% rolled, annealed 250 min. at 1050

◦

C).

512 6 Applications

Here, lny

is the mean value of lny and σ is the width of the distribution and

Ψ(y

∗

)dy represents the frequency of grains of size y with y

∗

≤ y ≤ y

∗

+ dy.

In a linear plot Ψ(y)vs.y the distribution is asymmetrical (Fig. 6.2) and,

therefore, the most frequent value y

(median) does not coincide with the

average value (mean) ¯y.Rather,y

and ¯y are related by

=exp



ln ¯y − σ



(6.2)

i.e. y

< ¯y. The log-normal distribution does not follow easily from fundamen-

tal considerations. On the contrary, from theoretical models of grain growth,

the stationary grain size distribution, which would satisfy







=0 (6.3)

does not yield a log-normal distribution but a distribution, which is not ob-

served in real grain structures. This contradiction is commonly reconciled by

the assumption that the kinetic processes, which modify the grain structure

toward a steady-state, cease to operate before a stationary distribution is at-

tained, and the log-normal distribution represents an approximation of the

non-stationary structure.

The mathematical grain size distribution as expressed in terms of Eq. (6.1)

and displayed in Fig. 6.1 relates to an inﬁnitely large assembly of grains.

Despite the large number of grains in a polycrystal (10

/mm

) one would

probably not ﬁnd any grain with a precisely given grain size. Therefore, ex-

perimental grain size distributions are represented in terms of histograms,

where the frequency of grains in a given size interval is plotted as a bar graph

(Fig. 6.2).

Neither the average grain size nor the grain size distribution can provide

a comprehensive description of polycrystalline aggregates. Grain morphology

and topology are rarely accounted for, since stereology requires laborious spec-

imen handling for a 3D image of the grain assembly. Grain shape — in terms

of ellipticity — or distribution of the number of grain edges and corners can

be determined to more adequately represent the grain structure. However,

owing to the extraordinary eﬀorts involved in gaining this information exper-

imentally, it is only collected on particular need, e.g. if grain topology plays a

role for the problem under consideration, as in a comprehensive treatment of

grain growth. Nowadays, powerful image analysis systems, which allow digital

storage of metallurgical micrographs, i.e. sections through grain assemblies,

are now becoming available to facilitate stereological evaluation.

The quantitative evaluation of heterogeneous microstructures from 2D sec-

tions is the subject of textbooks on stereology and image analysis systems.

In the context of grain boundaries in single phase materials areas of primary

interest usually include grain size, grain shape and spatial orientation of grain

boundary planes. There are two basic methods to determine the average grain

size: lineal and areal analysis. An automated image analysis system will reveal

6.1 Characterization of Microstructure and Texture 513

the boundaries in a planar section and determine the mean cross section

A of

a grain, from which the mean grain size

D can be derived, assuming equiaxed

grain shape

D =

A (6.4)

For manual evaluation of micrographs from planar sections, it is most common

to superimpose parallel lines to the microstructure such that any single grain

is intersected only once by a line. Then the number N of intersections of grain

boundaries with the total line length L is counted to yield the average grain

size

D =

(6.5)

as a mean linear intercept. In the case of nonequiaxed microstructures the

linear intercepts in three perpendicular directions

have to be

determined, requiring two mutually perpendicular sections, to yield

D =





1/3

(6.6)

The ratios

denote the respective ellipticity or aspect

ratio.

A quantity of particular interest with regard to grain boundaries is the

surface area A

per unit volume, which is found to be [565]

=2·

≡ 2N

(6.7)

In the case of non-equiaxed microstructures

=0.429 N

+2.571N

− N

∼



0.429

+2.571

− 1



(6.8)

where N

represents the respective inverse linear intercepts or boundary

counts per unit length [566].

6.1.3 Crystallographic Texture

6.1.3.1 Deﬁnitions

Metallic materials are crystalline, i.e. within a crystallite the atoms are ar-

ranged in a strictly periodic pattern, which is represented by the crystal-

lographic unit cell of the particular crystal structure. While all grains of a

polycrystal have the same crystal structure, the orientation of the unit cell

with respect to the macroscopic specimen coordinate system changes from

grain to grain. This relationship between spatial orientation of the crystal-

lographic unit cell as deﬁned by its fundamental crystallographic axes and

the macroscopic specimen system as deﬁned by its characteristic directions is

referred to as crystallographic orientation. The distribution of orientations in

514 6 Applications

(a)

(b)

(a)

(b)

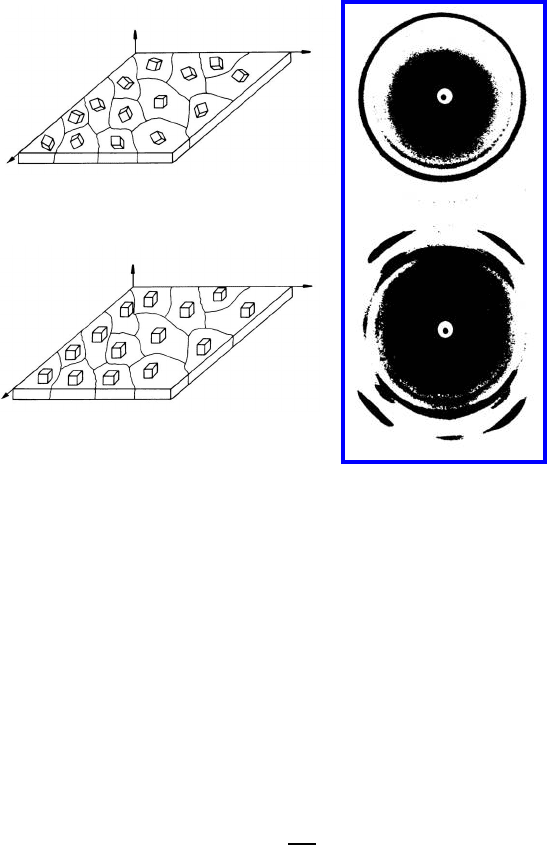
FIGURE 6.3

Schematic representation of the orientation distribution and the resulting

Debye-Scherrer images in sheet metal with (a) random distribution; (b) when

a preferred orientation exists (here: Cube texture).

a polycrystal is termed its crystallographic texture, or simply texture. Real

textures comprise very diﬀerent cases, from a random orientation distribution

(random or gray texture) to a single component texture, the extreme of which

would be a single crystal (Fig. 6.3).

Quantitatively, the texture is represented by the orientation distribution

function (ODF) f (g) [567, 568], where f (g

∗

)dg is the specimen volume fraction

dV/V with orientation g

∗

in the interval g

∗

≤ g ≤ g

∗

+ dg, i.e.

f(g)dg =

(6.9)

Since the orientation of a cubic crystal is deﬁned as the relation between the

(orthonormal) crystal axes and the (orthonormal) specimen axes, it is mathe-

matically represented by the rotation that makes the specimen axes align with

the crystal axes. There is an inﬁnite number of ways to prescribe a procedure

of rotations that makes two coordinate systems coincide. Irrespective of the

procedure, however, the rotation is mathematically formulated in a unique

way, namely by the rotation matrix g, which is deﬁned as



= gr (6.10)

6.1 Characterization of Microstructure and Texture 515

where r represents any vector of the unrotated system and r



is the corre-

sponding vector in the rotated system. By using the bases of the two systems

to deﬁne the vector r or r



it is obvious that the columns of the (3 x 3) matrix

g correspond to the direction cosines of the base vectors of the rotated system

with respect to the unrotated system.

Three quantities are necessary to describe a rotation in three dimensions,

for example three angles of subsequent rotations around prescribed axes. Since

there is an inﬁnite set of three axes, there is an inﬁnite number of ways to

put up the rotation matrix. There are three ways which are most convenient

and, therefore, most commonly used to describe an orientation relationship

(Fig. 6.4c):

a) Axis and angle of rotation: ω[HKL] (Fig. 6.4a). It is easy to imagine and

readily handled in stereographic projection. The notation is especially useful

for the treatment of problems related to grain boundaries, as the type of grain

boundary is commonly deﬁned by the orientation of the boundary plane with

respect to the axis of rotation

(see Chapter 2).

b) Miller indices (Fig. 6.4c): 2 crystallographic directions in coordinate system

1, which are parallel to the base vectors of coordinate system 2. For instance,

for rolling geometry parallel to rolling plane normal: (hkl) and rolling direc-

tion: [uvw]. It is commonly used when the crystal lattice is to be related to

the specimen coordinate system, e.g. in a tensile sample with respect to the

tensile axis or for rolling geometry with respect to rolling plane and rolling

direction.

c) The three Euler angles (Fig. 6.4b): ϕ

,Φ,ϕ

.Thisiswidelyusedforan

analytical treatment of rotations. It is standard for the calculation and repre-

sentation of textures in terms of the three-dimensional orientation distribution

function (ODF).

The rotation matrix g is only unique if there is no crystal symmetry [568].

In cubic crystals there is a 24-fold symmetry, i.e. there are 24 diﬀerent rota-

tions S

which do not change the position of lattice points. Each rotation g

may, therefore, be superimposed by any combination of S

to give an equiva-

lent orientation of the rotated lattice. Any of the 24 diﬀerent orientations of

a vector r

in cubic lattices is, therefore, obtained by



= S

· g · r

(6.11)

This deﬁnition of the grain boundary character is not unambiguous in crystal structures of

high symmetry, like the cubic lattice. Depending on the choice of the 24 possible symmetry

elements in a cubic lattice, a grain boundary may be either tilt or twist boundary. An

example gives the coherent twin plane in fcc crystals. Its Miller indices (plane normal) are

{111}, in particular (111). The twin orientation can be described by a 60

◦

[111] rotation. In

this case the (111) boundary plane is a twist boundary. A symmetrically equivalent rotation

is 70.6

◦

10]. The coherent twin boundary is now a tilt boundary. Therefore, for uniqueness

in highly symmetric lattices additional rules of selection have to be applied. Unambiguity

is attained by selection of the smallest angle of rotation.