Gottstein G., Shvindlerman L.S. Grain Boundary Migration in Metals: Thermodynamics, Kinetics, Applications

Подождите немного. Документ загружается.

4.2 Thermodynamics of Triple Junctions 335

tension there mentioned may have a negative value. This would be the case

with respect to a line in which three surfaces of discontinuity are regarded

as meeting, but where nevertheless there really exists in stable equilibrium

a ﬁlament of diﬀerent phase from the three surrounding masses” [400]. The

triple junction with a negative linear tension should manifest unusual ther-

modynamic properties. In particular, the adsorption of impurities on a triple

junction, as a rule, should be negative and, therefore, deﬁnitely lower than at

grain boundaries. Further, the behavior of a triple junction with negative line

tension can be manifested in the attempt to increase its length. The descrip-

tion given above complies with this requirement. Computer simulations of the

energy of a grain boundary triple junction indicate the feasibility of such an

eﬀect [405].

4.2.1 Grain Boundary Triple Line Tension — Experimental

Approach

The inﬂuence of grain boundary junctions on grain growth, stability and evo-

lution of the grain microstructure was recognized recently [360, 361, 411]. This

eﬀect is especially pronounced in ﬁne-grained and nanocrystalline materials.

The inﬂuence of the line tension of grain boundary triple junctions on grain

growth and stability of nanocrystalline materials is not conﬁned to its contri-

bution to the driving force of grain growth but determines the adsorption on

the triple junctions and in turn their mobility, as well.

The problem of triple line energy was discussed by Gibbs who came to

the conclusion that the excess free energy of a triple line between ﬂuid phases

might be positive or negative [1, 362]. McLean [23] contended that triple junc-

tions should have a positive energy owing to the inﬂuence of three connected

grain boundaries. The attempts to extract the triple line energy from simple

geometrical model were undertaken in [406]–[408]. More recent computational

studies by Srinivasan et al. [364] and Van Swygenhoven [363, 365] came to con-

tradictory conclusions. The authors of [364] concluded that a negative triple

line energy is possible although the value of the triple line tension obtained

in the simulation studies [365] was always positive. Unfortunately, there is a

poor body of experimental data to resolve this issue by properly conducted

experiments. This is mainly due to both the experimental diﬃculties and the

lack of a rigorous theoretical basis of such measurements [366].

The theoretical foundations of the measurement of triple line tension along

with the experimental technique were put forward and developed in [366, 421].

It is well known that the equilibrium at the straight root of a thermal groove

satisﬁes the condition

γ = γ

sinΘ

+ γ

sinΘ

(4.21)

where Θ

,Θ

and γ

, γ

are the respective angles and surface energies to

both sides of the groove. In the case that the grain boundary is curved in the

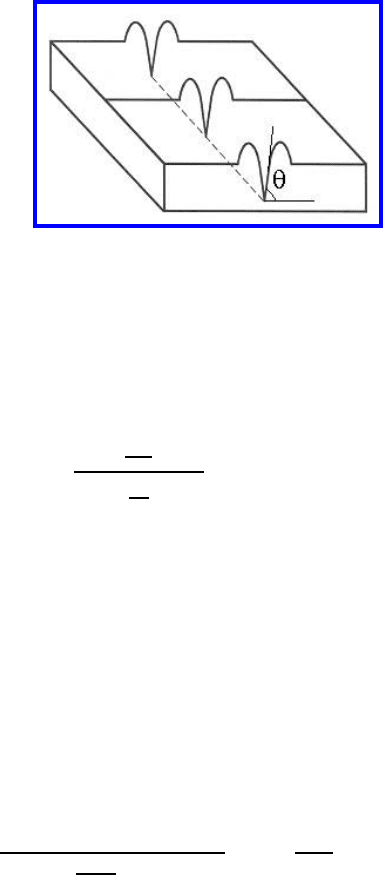
336 4 Thermodynamics and Kinetics of Connected Grain Boundaries

FIGURE 4.2

Grain boundary formed at a ﬂat grain boundary with a curved groove root

[366].

root of the groove (Fig. 4.2), one has to take into account an additional term,

the so-called line tension γ



of the groove root triple line, i.e.

γ − γ



∂

∂r





∂u

∂r





3/2

= γ

sinξ

+ γ

sinξ

(4.22)

The term u(r) mathematically describes the proﬁle of the groove root. The

multiplier of γ



is the Laplace curvature. The dihedral angles denoted by ξ

and ξ

have the same meaning like Θ

and Θ

in Eq. (4.21) but are diﬀerent

in magnitude owing to the curvature. The topography of a triple junction

[366] after thermal annealing is formed by three grain boundaries which meet

in a way schematically shown in Fig. 4.3. The three groove roots are curved

towards the triple junction but remain straight far away from this point.

If one associates a line tension with the triple line, an equilibrium of the

four competing line tensions (thethreegroovelinetensionsγ



i−j

and the triple

line tension γ



) at the triple junction will be established. The line tension of

these groove roots at the triple junction is



i−j

− γ

sinξ

− γ

sinξ

∂

∂r

r=0





∂u

∂r

r=0





3/2

(4.23)

From the equilibrium of the four line tensions it follows for the triple line

tension



= γ



1−2

sinζ

1−2

+ γ



1−3

sinζ

1−3

+ γ



2−3

sinζ

2−3

(4.24)

Eq. (4.24) constitutes the theoretical basis of the presented approach and can

be used to calculate the triple line energy from the geometry of the boundaries

merging at the triple junction [366].

The ﬁrst measurements were performed on polycrystalline copper with

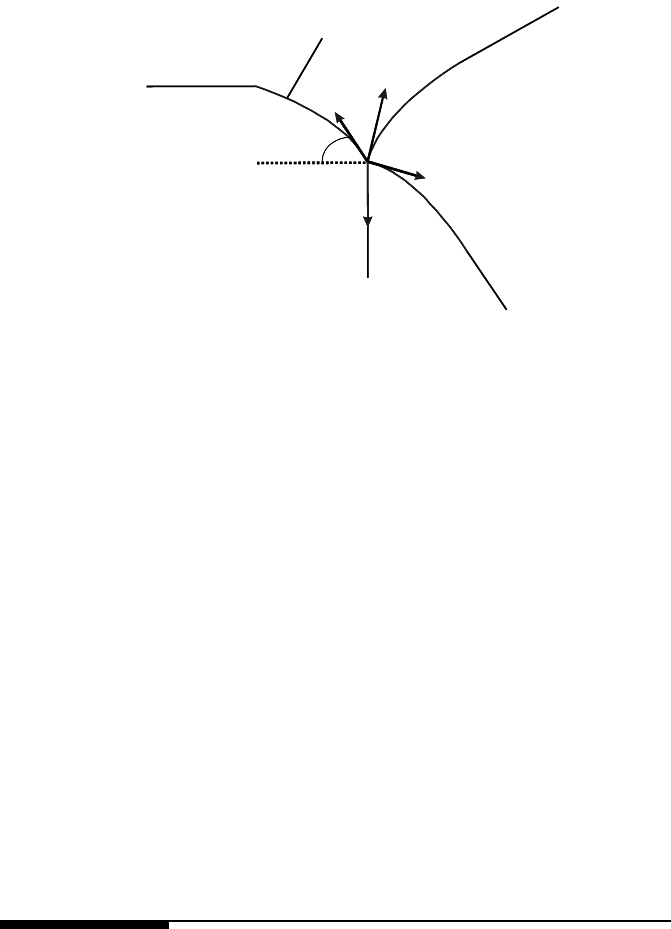
4.3 Motion of a Grain Boundary System with Triple Junctions 337

Triple line

Groove root 1-2

()ur

−

1-3

1-2

2-3

1-2

Triple line

Groove root 1-2

()ur

−

1-3

1-2

2-3

1-2

FIGURE 4.3

Schematic 3D view of the line tension equilibrium in the triple junction [366].

rather large grains [366]. The topography in the close vicinity of the triple

junctions was measured by means of atomic force microscopy. An example for

groove formation at grain boundaries intersecting the crystal surface is given in

Fig. 4.4. In the center of the ﬁgure there is a triple junction of the three adjoin-

ing grain boundaries (Fig. 4.4(a)) [366]. From AFM measurements (Fig. 4.4)

all necessary parameters can be derived to extract the triple line tension, such

as the grain boundary groove angles Θ

, the groove root angles in the center

of the triple junction ξ

, and the proﬁle of the groove roots. The measured

value of the line tension of grain boundary groove root γ



i−j

and grain bound-

ary triple junction γ



are positive and equal to (17.0 ± 7.0) · 10

−9

J/m and

6.5 ± 2.5) ·10

−9

J/m, respectively. The analysis given in [366] shows that the

negative value of γ



can be expected only for the line tension of grain bound-

ary groove root γ



i−j

smaller than −4·10

−7

J/m. A comprehensive description

of the approach and the experimental technique of the measurements was put

forward in [366].

4.3 Motion of a Grain Boundary System with Triple

Junctions

It is usually tacitly assumed in all studies of grain boundary migration and

grain growth that the line tension of a triple junction is of minor importance

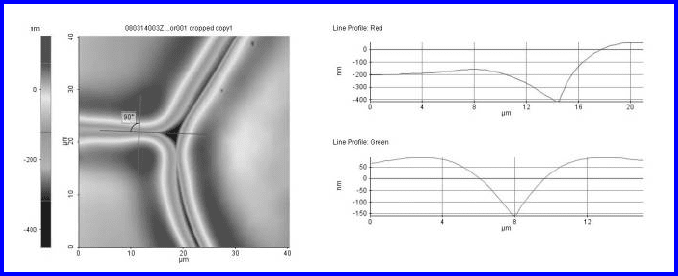
338 4 Thermodynamics and Kinetics of Connected Grain Boundaries

(a) (b)(a) (b)

FIGURE 4.4

Top view (a) of an AFM topography measurement in the vicinity of a triple

junction; (b) surface proﬁles along lines indicated in (a) [366].

and that a triple junction does not drag grain boundary motion. Thus, their

role in the migration process is reduced to maintaining the thermodynamic

equilibrium in terms of ﬁxed dihedral angles during grain boundary motion.

However, as shown in [403], the movement of triple junctions caused by

boundary migration can involve additional energy dissipation, in other words,

a triple junction can have a ﬁnite mobility. The concept of a speciﬁc triple

junction mobility was ﬁrst introduced in [403].

Steady-state motion of a system of boundaries with a triple junction is only

possible in a narrow range of geometrical conﬁgurations. One of them is shown

in Fig. 4.5 [403]. The triple junction in Fig. 4.5 is rectilinear and perpendicular

to the plane of the diagram. Far from the triple junction all three boundaries

are planar, their planes parallel to each other and perpendicular to the plane

of the diagram. This makes the problem quasi-two-dimensional.

Of course, this is very diﬀerent from situations that arise in a polycrystal,

and real triple junctions are unlikely to experience steady-state motion. But a

detailed analysis of this problem allows us to isolate the main physical aspects

of triple junction motion.

The next assumption relates to the driving forces of grain boundary and

triple junction motion in this system. It is commonly assumed that the system

only migrates under the action of the surface tensions γ of the adjoining grain

boundaries. The problem can be considered in the framework of a uniform

boundary model, i.e. all grain boundaries possess equal surface tensions and

mobilities irrespective of the misorientation of the adjacent grains and the

crystallographic orientation of the boundaries, and the mobilities of grain

boundary and triple junction are independent of their velocity.

The given assumptions require symmetry with respect to the x-axis. The

normal displacement of each element of a boundary, the velocity of grain

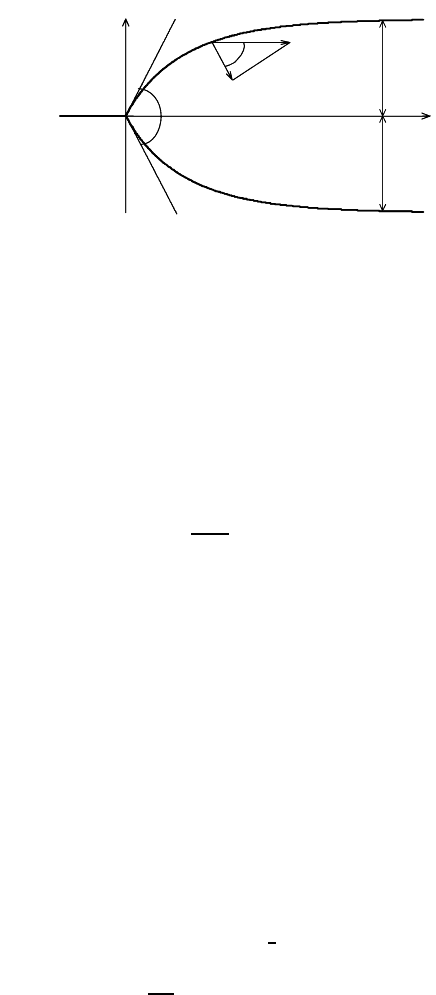
4.3 Motion of a Grain Boundary System with Triple Junctions 339

a/2

GB I

GB II

GB III

GB II

a/2

GB III

GB I

a/2

GB I

GB II

GB III

GB II

a/2

GB III

GB I

FIGURE 4.5

Geometry of the grain boundary system with triple junction in the course of

steady-state motion.

boundary motion, the diﬀerential equation of the shape y(x)ofamoving

grain boundary, and the boundary conditions are identical to the problem of

the shape of a moving boundary (Chapter 3, Eqs. (3.117)-(3.119)) and are

repeated here for convenience:



= −





1+y

2



(4.25)

y(0) = 0 (4.26)

y(∞)=a/2



(0) = tan Θ

The meaning of the length a and angle Θ is clear from Fig. 4.5.

Since a driving force γ (2 cos Θ − 1) is applied to the triple junction, the

velocity of its motion can be expressed as

V = m

γ (2 cos Θ − 1) (4.27)

where m

is the mobility of the junction.

Eqs. (4.25)–(4.27) deﬁne the problem completely. The shape of a steady-

state moving grain boundary was already described by Eq. (3.143):

y(x)=ξ arc cos



−



+ C

(4.28)

ξ =

2Θ

=ln(sinΘ)

= ξ (π/2 − Θ)

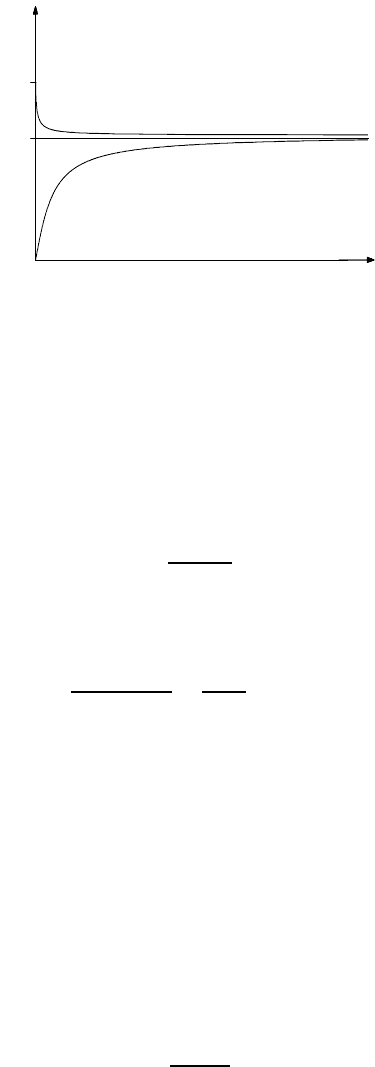
340 4 Thermodynamics and Kinetics of Connected Grain Boundaries

(a)

(b)

π/2

π/3

(a)

(b)

(a)

(b)

π/2

π/3

(a)

(b)

FIGURE 4.6

Geometry of the grain boundary system with triple junction in the course of

steady-state motion.

As can be seen, a steady-state motion of the grain boundary system with a

triple junction is possible indeed. The velocity of steady-state motion V of

the system is equal to

V =

2Θm

(4.29)

From (4.27) and (4.29) we arrive at the equation which determines the steady-

state value of the angle Θ:

2Θ

2cosΘ− 1

= Λ (4.30)

This equation associates the angle Θ with the value of the dimensionless

parameter Λ = m

a/m

, which characterizes the inhibiting inﬂuence of the

triple junction on grain boundary migration.

It should be stressed that the dimensions of m

, triple junction mobility,

and m

, the grain boundary mobility, are diﬀerent, so that their ratio m

has the dimension of a length.

For small Λ, m

a/m

 1, the contact angle Θ approaches zero. For large

Λ the junction does not inﬂuence boundary migration, and the angle Θ tends

to its equilibrium value π/3. The dependency of Θ vs. Λ is shown in Fig. 4.6.

When Λ  1thevalueV is independent of the mobility of the junction and

governed only by the boundary properties and the driving force, which is

proportional to 1/a

V =

2πm

(4.31)

4.4 Triple Junctions Motion in the Presence of Impurities 341

When Λ  1 the velocity is controlled by the mobility of the junction

V = γm

(4.32)

These relations comprise the theoretical background for experimental studies

of triple junction mobility and how it aﬀects grain boundary migration.

4.4 Triple Junctions Motion in the Presence of Impuri-

ties

Until now we have considered the migration of a grain boundary system which

does not interact with impurities. In the following we analyze the main eﬀects

introduced by impurities on the motion of a grain boundary system with

a triple junction. First of all we would like to emphasize that, contrary to

the motion of a single grain boundary, there are two aspects of the problem

where impurity inﬂuence can manifest itself, namely grain boundary motion

and triple junction motion. Let us consider more comprehensively the latter

aspect of the problem.

The theories of impurity inﬂuence on grain boundary motion also can be

applied, with certain corrections, to triple junction motion. Actually, if a triple

junction moves under the action of a driving force P with a certain number of

impurity atoms Γ



(per unit line length) the velocity of such a triple junction

is given by

= m

eﬀ

(4.33)

where the P

eﬀ

is the eﬀective driving force. (Please note that the dimension of

triple junction mobility and its driving force, respectively, are diﬀerent from

the respective quantities for grain boundary migration.) P

eﬀ

can be written

eﬀ

= P − Γ



f (4.34)

where f is the attraction force between the triple junction and an impurity

atom, Γ



is the adsorption on the triple junction.

On the other hand the velocity of an impurity atom and, therefore, also

of the triple junction in the case that both move together reads with the

Nernst-Einstein relation

(4.35)

where m

is the mobility of an impurity atom

v = m

f (4.36)

342 4 Thermodynamics and Kinetics of Connected Grain Boundaries

From Eq. (4.33)-(4.36) we ﬁnd

v =

1+Γ



(4.37)

or, for Γ



 1

∼



−



(4.38)

where D

is the pre-exponential factor and H

the activation enthalpy of

impurity diﬀusion, respectively.

Correspondingly, the triple junction mobility, with regard to the impurity

eﬀect, can be deﬁned as



−



(4.39)

Eqs. (4.37) and (4.39) describe the triple junction motion in the presence

of impurities in the L¨ucke-Detert approximation [193] (see Chapter 3). In

analogy to grain boundary motion in this approximation, an impurity atom

can move together with a triple junction at a velocity not exceeding

∗

−



(4.40)

where r

is the smallest dimension of the defect (grain boundary or triple

junction width), and the ratio U

is an estimate of the maximum force

of interaction between the impurity atom and the triple junction. (It should

be remembered that the dimension of U

for a triple junction line is [en-

ergy/length].) If the velocity exceeds v

∗

, the triple junction detaches from the

impurities.

However, apart from the inﬂuence of the impurity atoms on triple junction

motion, there is also an inﬂuence of these atoms on the motion of the adjoin-

ing grain boundaries. As mentioned, the motion of a grain boundary system

with a triple junction is akin to the motion of a grain boundary quarter-loop.

Therefore, the general solution for Eq. (4.25) is in accordance with [243, 244]

y = y

mγ

arc cos



−

(x−x

mγ



(4.41a)

where y

and x

are integration constants.

The grain boundary velocity V is at a maximum at the triple junction and

reduces to zero at the straight, immobile sections. The critical velocity v

∗

can

be described as the velocity of such point on the grain boundary system with

a triple junction (Fig. 4.5) which separates two boundary segments, namely

a segment detached from the impurity atoms (to the left in Fig. 4.5) and a

4.4 Triple Junctions Motion in the Presence of Impurities 343

segment loaded with impurities (to the right in Fig. 4.5), in other words, for

0 ≤ x ≤ x

∗

the velocity V>v

∗

and for x>x

∗

the velocity V<v

∗

(see

Chapter 3, Sec. 3.4.3).

The ﬁnal solution for the shape of a moving grain boundary half-loop with

a triple junction is quite similar to the moving grain boundary quarter-loop

[244] (Eq. (4.41b))

y(x)=

⎧

⎪

⎨

⎪

⎩

−(b

− b

)arc cos



sin Θ

∗



− b

+ b

arc cos



ln(sinΘ)−x



0 ≤ x ≤ x

∗

− b

+ b

arc cos

⎛

⎝

ln(sinΘ)−x

∗

„

−1

−x

⎞

⎠

x ≥ x

∗

(4.41b)

From the relation for b

and b



arc cos



sinΘ

∗



+Θ−



−

arc cos



sinΘ

∗



−

where b

and b

relate to the “free” and “loaded” part of the boundary

(4.42)

we obtain the mobilities of “free” and “loaded” boundary m

and m

,re-

spectively.

The angle Θ in Eq. (4.41b) is deﬁned by Eq. (4.30), in which the criterion

Λ is a function of triple junction and grain boundary mobility in the presence

of impurities.

There is a variety of possible situations. Besides the grain boundary impu-

rity attachment-detachment processes the phenomena of triple junction impu-

rity attachment-detachment have to be considered, and in addition the com-

binations “free” grain boundary — “free” triple junction, “free” grain bound-

ary — “loaded” triple junction etc. Unfortunately, little is known about triple

junction mobility, and there are no data at all about the interaction between

triple junctions and impurity atoms.

344 4 Thermodynamics and Kinetics of Connected Grain Boundaries

4.5 Experimental Investigations of Triple Junction

Motion

As mentioned above, triple junctions along with grain boundaries comprise

the main structural elements of polycrystals. In spite of its importance for mi-

crostructural evolution the inﬂuence of triple junctions on boundary migration

has not been treated at all experimentally and has been hardly investigated

theoretically, mainly by computer simulations [409]. In comparison the mo-

tion of grain boundaries has been studied actively, especially in the past 20-

30 years [410]. In all studies concerning grain boundary migration and grain

growth it was tacitly assumed that triple junctions do not have an inﬂuence

on the motion of the boundaries; rather, their role in this process was reduced

to establishing the thermodynamic equilibrium angles at the junction during

boundary motion.

The lack of experimental results for triple junction motion is mainly due to

the experimental diﬃculties of obtaining reliable data. Not only the velocity

of the junction motion must be measured, but also the shape of the inter-

secting grain boundaries during the motion, as will be discussed below. For

conducting experiments a technique had to be developed which allowed con-

tinuous monitoring of the position and the geometrical arrangement of such

boundary system during the experiment. Moreover, the steady-state motion

of a grain boundary system with a triple junction is only possible in a very

narrow range of geometrical conﬁgurations with speciﬁc restrictions imposed

on the properties of the grain boundaries in the system. In particular, it was

suggested that all grain boundaries in the system are identical. The equations

considered for the motion of the grain boundary system with a triple junction

(4.28)–(4.31) retain their validity for the following boundary system, a so-

called symmetrical boundary system, which is shown in Fig. 4.5: two identical

curved boundaries (GB I and II) and a diﬀerent straight boundary (GB III).

Such a system is much more convenient for experimental realization than a

system comprising only ideal uniform boundaries. In the following this deﬁned

boundary system will be considered [183]. The respective surface tensions and

the mobilities of the boundaries are

= γ

≡ γ = γ

(4.43)

= m

≡ m

= m

If the angles at the triple junction are in equilibrium, the driving force is equal

to zero and for a ﬁnite triple junction mobility the velocity v

should vanish

as well. Consequently, for a ﬁnite m

, a motion of the triple junction detunes

the equilibrium angles and, as a result, drags the motion of the boundaries.

For the situation given in Fig. 4.5

= m

(2γ cosΘ − γ

) (4.44)