Gockenbach M.S. Partial Differential Equations. Analytical and Numerical Methods
Подождите немного. Документ загружается.

278
Chaoter
6.
Heat flow
and
difFusior
and
graph
the
solution
at
times
0,0.02,0.04,0.06,
along with
the
steady-
state
solution. (See Exercise 6.2.1
and
Figure
C.12.)
7.
(a)
Formulate
the
weak
form
of the
following
BVP
with mixed boundary
conditions:
(b)
Show
that
the
weak
and
strong
forms
of
(6.57)
are
equivalent.
8.
Repeat Exercise
7 for the BVP
9.
Consider
a
copper
bar
(p
=
8.97g/cm
3
,c
=
0.379 J/(gK),
K
=
4.04
W/(cmK))
of
length
1 m and
cross-sectional
area
2
cm
2
.
Suppose
the bar is
heated
to a
uniform
temperature
of 8
degrees Celsius,
the
sides
and the
left
end
(a;
=
0)
are
perfectly insulated,
and the
right
end (x =
100)
is
placed
in an ice
bath.
Find
the
temperature distribution
u(x,t)
of the bar by
formulating
and
solv-
ing
an
IBVP.
Graph
u(x,
300)
(t in
seconds).
Use the
finite
element method
with
backward
Euler
time integration.
10.
Consider
a
chromium
bar of
length
25 cm. The
material constants
for
chromium
are
p
=
7.15g/cm
3
,
c =
0.439J/(gK),
«
=
0.937W/(cmK).
Suppose
that
the
left
end of the bar is
placed
in an ice
bath,
the
right
end is
held
fixed at 8
degrees Celsius,
and the bar is
allowed
to
reach
a
steady-state
temperature. Then
(at t = 0) the
right
end is
insulated. Find
the
temperature
distribution using
the finite
element method
and
backward Euler integration.
Graph
u(x,
100).
11. Let K 6
R,(
n
+
1
)
x
(
n
+
1
)
be the
stiffness
matrix
for the
Neumann problem,
and
let
K
e
R
nxn
be the
matrix obtained
by
removing
the
last
row and
column
278
Chapter
6.
Heat
flow
and diffusion
u(x,
0)
=
x(l
- x), 0 < x < 1,
au
ax
(0, t) =
0,
t >
0,
au
ax
(1, t) = 0, t > 0
and graph the solution
at
times 0,0.02,0.04,0.06, along with the steady-
state
solution. (See Exercise 6.2.1 and Figure C.12.)
7.
(a) Formulate
the
weak form of the following
BVP
with mixed boundary
conditions:
d ( dU)
- dx k(x) dx
=
I(x),
0 < x <
£,
u(O)
= 0,
~~(£)
=
O.
(b) Show
that
the weak and strong forms of (6.57) are equivalent.
8. Repeat Exercise 7 for the
BVP
d ( dU)
-dx
k(x)dx
=/(x),O<x<£,
du
(0) = 0
dx '
u(£)
=
O.
(6.57)
9.
Consider a copper
bar
(p
= 8.97
g/cm
3
,
c = 0.379J/CgK),
'"
= 4.04 W/CcmK))
of length 1 m
and
cross-sectional area 2 cm
2
•
Suppose the bar
is
heated
to
a
uniform temperature of 8 degrees Celsius,
the
sides and the left end (x =
0)
are perfectly insulated, and the right end (x = 100)
is
placed in
an
ice bath.
Find the temperature distribution
u(x, t) of the bar by formulating
and
solv-
ing
an
IBVP. Graph u(x, 300) (t in seconds). Use the finite element method
with backward Euler time integration.
10. Consider a chromium
bar
oflength
25
cm.
The
material constants for chromium
are
p = 7.15g/cm
3
,
c =
0.439J/(gK),
'"
=
0.937W/(cmK).
Suppose
that
the left end of the
bar
is
placed in
an
ice bath, the right end
is
held fixed
at
8 degrees Celsius, and the
bar
is
allowed to reach a steady-state
temperature.
Then
(at t =
0)
the right end
is
insulated. Find the temperature
distribution using
the
finite element method
and
backward Euler integration.
Graph u(x,
100).
11. Let K E
R(n+1)x(n+1)
be the stiffness matrix for
the
Neumann problem, and
let
K E R
nxn
be the matrix obtained by removing the last row and column

6.6.
Green's
functions
for the
heat equation
279
of
K.
Prove
that
K is
nonsingular.
(Hint:
K is the
stiffness
matrix
for the
BVP
with mixed boundary conditions, Neumann
at the
left
and
Dirichlet
at
the
right.)
6.6
Green's
functions
for the
heat equation
We
will
now
discuss Green's functions
for the
heat
equation.
Our
treatment
of
this
topic
is
intentionally
rather
superficial,
and our
goals
are
modest.
We
simply want
to
introduce
the
concept
of a
Green's
function
for a
time-dependent
PDE and
show
an
example.
To do
anything more would
distract
us
from
our
main topics.
Before
we
present
any
specific
Green's
functions,
we
describe
the
form
that
the
Green's
function
must take.
We
have
so far
seen
two
examples
of
Green's functions.
First
of
all,
in an
IVP
for an
ODE,
the
solution
u =
u(t)
is of the
form
where
/ is the
right-hand side
of the ODE
(see Section
4.6 for an
example). This
formula
shows
the
contribution
of the
data
at
time
s on the
solution
at
time
t—
this contribution
is
G(t]
s)f(s)
ds.
39
The
reader should notice
how the
value
u(t)
is
found
by
"adding
up" the
contributions
to
u(t)
of the
data
from
the
time interval
[to,
oo).
(The value
of
G(t;
s) is
zero when
s >
t,
so in
fact only
the
data
from
the
time interval
[tQ,t]
can
affect
the
value
of
u(t).)
We
have also seen
an
example
of a
Green's
function
for a
steady-state
BVP
(see
Section
5.7),
where
the
data
(the right-hand side
of the
differential
equation)
is
given
over
a
spatial
interval such
as
[0,1].
In
this case,
the
value
u(x)
is
determined
by
adding
up the
contributions
from
the
data
at all
points
in the
interval.
The
solution
formula
takes
the
form
In the
case
of a
time-dependent PDE,
the
data
/(x,t)
(the right-hand side
of
the
PDE)
is
prescribed over
both
space
and
time. Therefore,
we
will
have
to add
up the
contributions over both space
and
time,
and the
formula
for the
solution
will
involve
two
integrals:
39
By
this
we
mean that
the
contribution
of the
data
over
a
small interval
(s
—
e,
s +
e)
is
6.6. Green's functions for the heat equation
279
of
K.
Prove
that
K is nonsingular. (Hint: K
is
the
stiffness matrix for
the
BVP
with mixed boundary conditions, Neumann
at
the
left
and
Dirichlet
at
the
right.)
6.6 Green's functions for the heat equation
We will now discuss Green's functions for
the
heat equation.
Our
treatment
of this
topic is intentionally
rather
superficial,
and
our goals are modest.
We
simply want
to
introduce
the
concept of a Green's function for a time-dependent
PDE
and
show
an
example. To do anything more would distract us from our main topics.
Before
we
present any specific Green's functions,
we
describe
the
form
that
the
Green's function must take.
We
have so far seen two examples of Green's functions.
First
of all, in
an
IVP for
an
ODE,
the
solution u = u(t) is of
the
form
u(t) =
(JO
G(t;
s)f(y)
ds,
ito
where f is the right-hand side of
the
ODE (see Section 4.6 for
an
example). This
formula shows
the
contribution of
the
data
at
time s on the solution
at
time
t-
this contribution is G(t;
s)f(s)
ds.
39
The
reader should notice how the value u(t)
is
found by "adding up"
the
contributions
to
u(t) of
the
data
from
the
time interval
[to,
00).
(The value of G(t;
s)
is zero when s > t, so in fact only
the
data
from
the
time interval
[to,
tl
can affect the value of u(t).)
We
have also seen an example of a Green's function for a steady-state
BVP
(see Section 5.7), where
the
data
(the right-hand side of
the
differential equation)
is
given over a spatial interval such as
[0,
fl.
In
this case,
the
value u(x) is determined
by adding up the contributions from the
data
at
all points in
the
interval.
The
solution formula takes
the
form
u(x) =
10£
g(x;y)f(s)
dy.
In
the
case of a time-dependent
PDE,
the
data
!(x,
t) (the right-hand side of
the
PDE)
is prescribed over
both
space
and
time. Therefore,
we
will have
to
add
up
the
contributions over
both
space
and
time, and
the
formula for
the
solution will
involve two integrals:
u(x, t) =
roo
r
l
G(x,
t;
y,
s)f(y,
s)
dy
ds.
ito
io
39By this
we
mean
that
the
contribution
of
the
data
over a small interval
(8
-
£,8
+
£)
is
1
8+<
8-<
G(tj
s)f(s)
ds.
If
we
define
280
Chapter
6.
Heat flow
and
diffusion
6.6.1
The
Green's
function
for the
one-dimensional
heat
equation
under
Dirichlet
conditions
We
will
compute
the
Green's
function
for the
IBVP
The
solution
to
(6.58)
was
derived
in
Section 6.1;
it is
where
and
We
can
derive
an
expression
for the
Green's
functions
by
manipulating
the
formula
for
u(x,i):
then
we
have
Therefore,
G(x,t;y,s)
is the
Green's
function
for
(6.58).
280
Chapter
6.
Heat flow
and
diffusion
6.6.1
The
Green's function for the one-dimensional heat
equation under Dirichlet conditions
We
will compute
the
Green's function for
the
IBVP
au a
2
u
pc
at
- "'ax
2
=
f(x,t),
0<
x <
£,
t>
to,
u(x,
to)
= 0, ° < x <
£,
(6.58)
u(O,
t) = 0, t > to,
u(£, t) = 0, t > to.
The
solution
to
(6.58) was derived in Section 6.1;
it
is
00
u(x, t) = L an(t) sin
(n;x),
n=l
where
and
Cn(s)
=
~
1£
fey, s) sin
C?)
dy.
We
can derive
an
expression for
the
Green's functions
by
manipulating
the
formula
for u(x,
t):
If
we
define
{
~
~oo
e-t<n
2
7r
2
(t-s)/(pcP)
sin
(~)
sin
(n7rre)
G(x t
Y
s)
pel
L...n=l
£
£'
,
;,
=
0,
then
we
have
u(x,t)
= t
XJ
(t
G(x,t;y,s)f(y,s)dyds.
ltD
10
Therefore, G(x, t; y, s) is
the
Green's function for (6.58).
to
S
sst,
s > t,
(6.59)
40
That
is,
this particular Green's
function
is not
useful
as a
computational tool.
We
have encoun-
tered other Green's
functions,
particularly those
in
Section 4.6,
that
are
useful
computationally.
41
The
reader should recall
from
Section
2.1
that
each term
in the
heat equation, including
the
right-hand side,
is
multiplied
by A in the
original derivation.
42
The
interested reader
can
consult Haberman
[22],
Chapter
11.
6.6.
Green's
functions
for the
heat equation
281
Having
derived
the
Green's
function,
we
ought
to ask the
question:
What
is it
good
for?
It may be
obvious
to the
reader
that
the
formula
for u is no
more
useful,
for
computational purposes,
than
the
original Fourier series solution. Therefore,
the
value
of the
Green's
function
is not as a
computational
tool.
40
Rather,
the
Green's
function
is
valuable because
of the
insight
it
gives
us
into
the
behavior
of
solutions
to the
IBVP.
Indeed,
the
Green's
function
can be
regarded
as a
solution
to the
IBVP with
a
special right-hand side.
The
solution
to
is
The
right-hand side
6(x—yo)6(t—SQ)
represents
a
source concentrated entirely
at the
space-time point
(yo,so).
The
IBVP (6.60) describes
the
following
experiment:
A
bar of
length
t is
initially
at
temperature
0, and at
time
SQ
>
to,
A
Joules
of
energy,
where
A is the
cross-sectional
area
of the
bar,
41
are
added
to the
cross-section
at
x —
y
0
.
The
heat
energy then
flows in
accordance with
the
heat
equation.
By
examining
snapshots
of
G(x,t;yo,so),
we can get an
idea
of how the
temperature
changes with time.
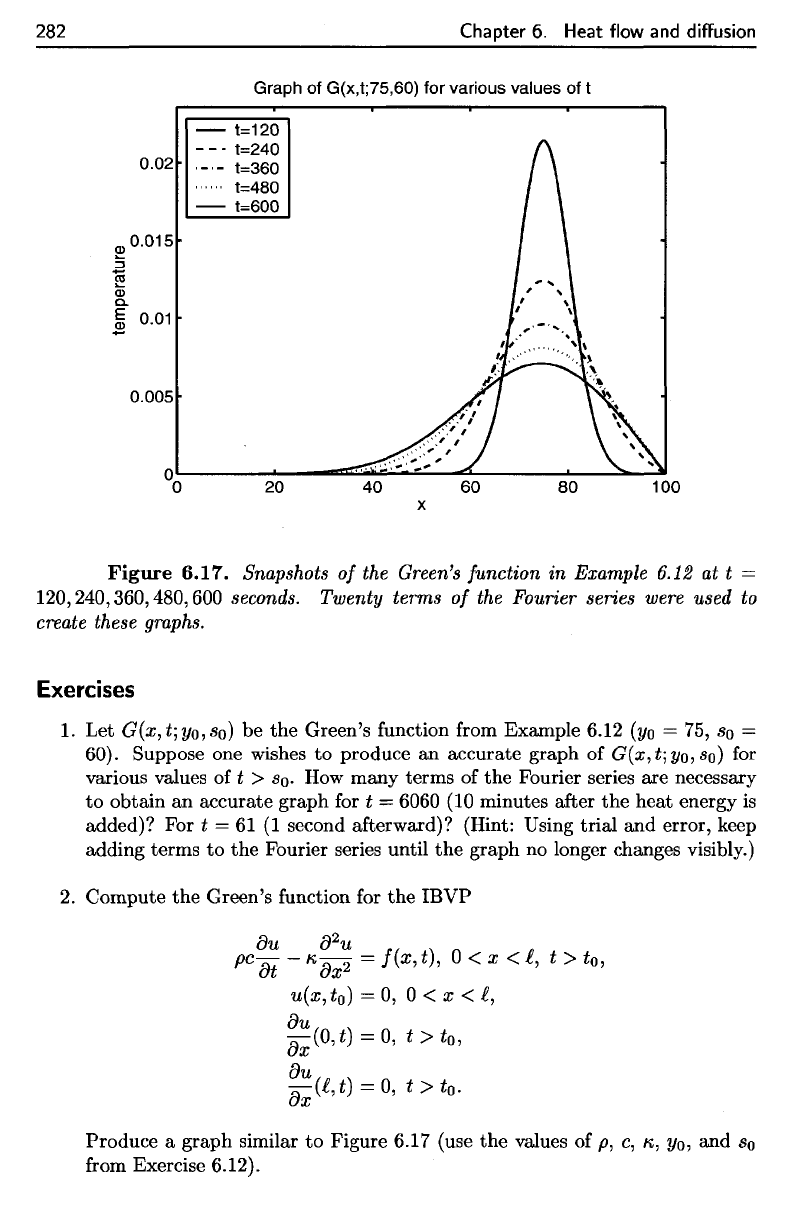
Example
6.12.
We
consider
an
iron
bar of
length
t —
100cm
(p =
7.8&g/cm
3
,
c
=
QA37J/(gK),
K
=
0.836
W/(cmK)).
We
graph
the
solution
to
(6.60)
with
to
=
0,
SQ =
60,
and
yo
= 75
(time
measured
in
seconds)
in
Figure
6.17,
which
shows
how the
spike
of
heat
energy
diffuses
over
time.
Formula (6.59)
has its
shortcomings;
for
example,
if one
wishes
to
compute
a
snapshot
of
G(x,t;y
0
,
s
0
)
for t
greater
than
but
very close
to SQ,
then many terms
of
the
Fourier series
are
required
to
obtain
an
accurate result
(see
Exercise
1).
It is
possible
to
obtain
a
more computationally
efficient
formula
for use
when
t is
very
close
to
SQ-
However,
to do so
would require
a
lengthy
digression.
42
6.6.2
Green's
functions
under
other
boundary
conditions
The
above method
can be
used
to
derive
the
Green's
function
for the
one-dimensional
heat
equation under other boundary conditions, such
as
Neumann
or
mixed bound-
ary
conditions.
The
calculations
are
left
to the
exercises.
6.6. Green's functions for the heat equation 281
Having derived
the
Green's function,
we
ought
to
ask
the
question:
What
is
it
good for?
It
may be obvious
to
the reader
that
the formula for u
is
no more useful,
for computational purposes,
than
the original Fourier series solution. Therefore,
the
value of the Green's function
is
not as a computational
too1.
40
Rather,
the
Green's function is valuable because of the insight it gives us into the behavior of
solutions to the IBVP. Indeed, the Green's function can be regarded as a solution
to
the
IBVP with a special right-hand side. The solution
to
au a
2
u
pc
at
-
~
ax
2
= 6(x - yo)6(t -
so),
0 < x <
e,
t > to,
u(x,
to)
=
0,
0 < x <
e,
(6.60)
u(O,
t) = 0, t >
to,
u(e,t) = 0,
t>
to
is
u(x, t) = G(x,
t;
Yo,
so).
The right-hand side
6(x-yo)6(t-s
o
)
represents a source concentrated entirely
at
the
space-time point
(Yo,
so).
The IBVP (6.60) describes the following experiment: A
bar
of length e
is
initially
at
temperature 0, and
at
time
So
> to, A Joules of energy,
where
A
is
the cross-sectional area of the bar,41 are added
to
the cross-section
at
x =
Yo.
The
heat energy then
flows
in accordance with the heat equation. By
examining snapshots of
G(x,
t;
Yo,
so),
we
can get
an
idea of how the temperature
changes with time.
Example
6.12.
We consider an iron bar
of
length e = 100 cm
(p
= 7.88 g/ cm
3
,
c = 0.437
J/(gK),
~
= 0.836
W/(cmK)).
We graph the solution to (6.60) with
to
= 0,
So
= 60, and
Yo
= 75 (time measured
in
seconds)
in
Figure 6.17, which
shows how the spike
of
heat energy diffuses over time.
Formula (6.59) has its shortcomings; for example, if one wishes to compute a
snapshot of
G (x,
t;
Yo,
so) for t greater
than
but
very close to so, then many terms
of the Fourier series are required
to
obtain an accurate result (see Exercise 1).
It
is
possible to obtain a more computationally efficient formula for use when t
is
very
close
to
so.
However, to do so would require a lengthy digression.
42
6.6.2 Green's functions under other boundary conditions
The above method can be used
to
derive the Green's function for the one-dimensional
heat equation under other boundary conditions, such as Neumann or mixed bound-
ary conditions. The calculations are left to the exercises.
40That
is,
this
particular
Green's
function
is
not
useful as a
computational
tool. We have encoun-
tered
other
Green's
functions,
particularly
those
in Section 4.6,
that
are useful
computationally.
41The
reader
should
recall from Section 2.1
that
each
term
in
the
heat
equation,
including
the
right-hand
side, is
multiplied
by A
in
the
original derivation.
42The
interested
reader
can
consult
Haberman
[22],
Chapter
11.
282
Chapter
6.
Heat flow
and
diffusion
Figure
6.17. Snapshots
of the
Green's
function
in
Example 6.12
at t =
120,240,360,480,600
seconds.
Twenty terms
of the
Fourier series
were
used
to
create
these
graphs.
Exercises
1. Let
G(x,t]yo,so)
be the
Green's function
from
Example 6.12
(yo
= 75,
SQ
=
60).
Suppose
one
wishes
to
produce
an
accurate graph
of
G(x,t;yo,
SQ)
for
various values
of t >
SQ.
How
many terms
of the
Fourier series
are
necessary
to
obtain
an
accurate graph
for t =
6060
(10
minutes
after
the
heat energy
is
added)?
For t = 61 (1
second
afterward)?
(Hint: Using
trial
and
error, keep
adding terms
to the
Fourier series until
the
graph
no
longer changes visibly.)
2.
Compute
the
Green's function
for the
IBVP
Produce
a
graph similar
to
Figure 6.17 (use
the
values
of p,
c,
K,
yo, and
SQ
from
Exercise
6.12).
282
Chapter
6.
Heat flow
and
diffusion
Graph of G(x,t;75,60) for various values of t
- t=120
-
_.
t=240
0.02
._.-
t=360
...... t=480
- t=600
Q)
0.015
::;
"§
Q)
c..
.ill
0.01
0.005
20
40
80
x
Figure
6.17.
Snapshots
of
the Green's function
in
Example 6.12 at t =
120,240,360,480,600 seconds. Twenty terms
of
the Fourier series were used to
create these gmphs.
Exercises
L
Let
G(x,
t;
Yo,
so) be
the
Green's function from Example 6.12
(yO
= 75,
So
=
60). Suppose one wishes
to
produce
an
accurate graph of
G(x,
t;
Yo,
so)
for
various values of
t > so. How many terms of
the
Fourier series are necessary
to
obtain
an
accurate graph for t = 6060 (10 minutes after
the
heat energy is
added)? For
t =
61
(1
second afterward)? (Hint: Using
trial
and
error, keep
adding terms
to
the
Fourier series until
the
graph no longer changes visibly.)
2.
Compute
the
Green's function for
the
IBVP
au
02u
pc
at - /'i,
ox
2
=
f(x,
t), 0 < x <
f,
t > to,
u(x,
to) =
0,
0 < x <
f,
au
ax
(0, t) =
0,
t > to,
au
ox(f,t)
=
0,
t>
to·
Produce a graph similar
to
Figure 6.17 (use
the
values of
p,
c,
/'i"
yo,
and
So
from Exercise 6.12).

6.7. Suggestions
for
further
reading
283
3.
Compute
the
Green's
function
for the
IBVP
Produce
a
graph similar
to
Figure 6.17 (use
the
values
of p,
c,
K,
yo,
and
SQ
from
Exercise
6.12).
4.
Compute
the
Green's
function
for the
IBVP
Produce
a
graph similar
to
Figure 6.17 (use
the
values
of p, c,
«,
yo
?
and
SQ
from
Exercise 6.12).
6.7
Suggestions
for
further reading
In
this section,
we
have continued
the
development, begun
in the
previous chapter,
of
both Fourier series
and finite
element methods.
The
references
noted
in
Section
5.8
are,
for the
most
part,
revelant
for
this chapter
as
well.
The
books [10,
4, 46,
22],
along with many others, introduce Fourier series
via the
method
of
separation
of
variables
briefly
described
in
Section
6.1.6.
Our
approach
is
perhaps unique among
introductory
texts.
To
gain
a
deep understanding
of
solution techniques,
it is
essential
to
know
the
qualitative theory
of
PDEs,
which
is
developed
in
several
texts.
A
basic under-
standing
can be
gained
from
introductory books such
as
Strauss [46]
or
Haberman
[22].
An
alternative
is the
older
text
by
Weinberger
[52].
For a
more advanced
and
complete study,
one
should consult
McOwen
[39]
or
Fblland [14]
or
Rauch
[41].
6.7. Suggestions for further reading
283
3. Compute
the
Green's function for
the
IBVP
au
a
2
u
pc
at
-
1£
ax
2
=
f(x,
t),
0<
x <
f,
t >
to,
u(x,
to)
= 0, ° < x < f,
u(O,
t)
= 0, t >
to,
au
ax(f,t)
= 0, t >
to·
Produce a graph similar
to
Figure 6.17 (use
the
values
of
p,
c,
1£,
Yo,
and
80
from Exercise 6.12).
4.
Compute
the
Green's function for
the
IBVP
au
a
2
u
pc
at
-
1£
ax
2
=
f(x,
t), ° < x <
f,
t >
to,
u(x,
to)
= 0, ° < x < f,
au
ax
(0, t) = 0, t >
to,
u(f,
t)
= 0, t >
to.
Produce a graph similar
to
Figure 6.17 (use
the
values of
p,
c,
1£,
Yo,
and
80
from Exercise 6.12).
6.7 Suggestions for further reading
In this section,
we
have continued
the
development, begun in
the
previous chapter,
of
both
Fourier series
and
finite element methods.
The
references noted in Section
5.8 are, for
the
most
part,
revel ant for this chapter as well.
The
books
[10,
4,
46,
22],
along with many others, introduce Fourier series via
the
method of separation of
variables briefly described in Section 6.1.6.
Our
approach is perhaps unique among
introductory texts.
To gain a deep understanding of solution techniques,
it
is
essential
to
know
the
qualitative theory of PDEs, which is developed in several texts. A basic under-
standing can be gained from introductory books such as Strauss
[46]
or Haberman
[22].
An alternative
is
the
older
text
by Weinberger
[52].
For a more advanced
and
complete study, one should consult McOwen
[39]
or Folland
[14]
or Rauch
[41].
This page intentionally left blank
This page intentionally left blank
Chapter
7
We
now
treat
the
one-dimensional wave equation, which models
the
transverse
vibrations
of an
elastic string
or the
longitudinal vibrations
of a
metal bar.
We
will
concentrate
on
modeling
a
homogeneous medium,
in
which case
the
wave equation
takes
the
form
Our
first
order
of
business
will
be to
understand
the
meaning
of the
parameter
c. We
then derive Fourier series
and finite
element methods
for
solving
the
wave equation.
It is
straightforward
to
extend
the finite
element methods
to
handle heterogeneous
media (nonconstant
coefficients
in the
PDE).
7.1 The
homogeneous wave
equation
without
boundaries
We
begin
our
study
of the
wave equation
by
supposing
that
there
are no
boundaries—
that
the
wave equation holds
for
—
oo
< x < oo.
Although this
may not
seem
to be
a
very realistic problem,
it
will
provide some
useful
information about wave motion.
We
therefore consider
the
IVP
(To
simplify
the
algebra
that
follows,
we
assume
in
this section
that
the
initial time
is
to
= 0.)
With
a
little cleverness,
it is
possible
to
derive
an
explicit formula
for the
solution
in
terms
of the
initial conditions
i^(x)
and
^(x).
The key
point
in
deriving
this
formula
is to
notice
that
the
wave operator
waves
We
now
treat
the one-dimensional wave equation, which models the transverse
vibrations of
an
elastic string or the longitudinal vibrations of a metal bar.
We
will
concentrate on modeling a homogeneous medium, in which case
the
wave equation
takes the form
8
2
u 8
2
u
8t
2
- C
2
8x
2
=
f(x,
t).
Our first order of business will be
to
understand the meaning of the parameter
c.
We
then
derive Fourier series and finite element methods for solving
the
wave equation.
It
is
straightforward
to
extend the finite element methods to handle heterogeneous
media (nonconstant coefficients in the PDE).
7.1 The homogeneous
wave
equation without
boundaries
We
begin our study
of
the
wave equation by supposing
that
there are no
boundaries-
that
the wave equation holds for
-00
< x <
00.
Although this may not seem to be
a very realistic problem, it will provide some useful information about wave motion.
We
therefore consider the IVP
8
2
u
f)
2
u
8t
2
-
c
2
f)x
2
=
0,
-00
< x <
00,
t > 0,
u(x,O) =
'l/J(x),
-00
< x <
00,
(7.1)
8u
8t
(x,O)
= ')'(x),
-00
< x <
00.
(To simplify the algebra
that
follows,
we
assume in this section
that
the
initial time
is
to
= 0.)
With
a little cleverness,
it
is
possible
to
derive an explicit formula for the
solution in terms of
the
initial conditions
'l/J(x)
and ')'(x). The key point in deriving
this formula
is
to
notice
that
the wave operator
8
2
2
8
2
f)t2
- C f)x2
285
286
Chapter
7.
Waves
can be
factored:
For
example,
The
mixed
partial
derivatives cancel because, according
to a
theorem
of
calculus,
if
the
second derivatives
of a
function
u(x,
t) are
continuous, then
It
follows
that
any
solution
w(x,
t) of
either
or
will
also solve
the
homogeneous wave equation.
If
u(x,t)
=
f(x
—
ct),
where
/ :
R
—t
R is any
twice
differentiate
function, then
Similarly,
if g
:
R
—>
R is
twice
differentiate,
then
u(x,t)
= g(x + ct)
satisfies
Thus,
by
linearity, every
function
of the
form
is
a
solution
of the
homogeneous wave equation.
We now
show
that
(7.2)
is the
general solution.
To
prove
that
(7.2) really represents
all
possible solutions
of the
homogeneous
wave
equation,
it
suffices
to
show
that
we can
always solve (7.1) with
a
function
of
286
Chapter
7.
Waves
can be factored:
For example,
The mixed partial derivatives cancel because, according
to
a theorem of calculus, if
the second derivatives of a function
u(x,
t) are continuous, then
or
8
2
u 8
2
u
8t8x
8x8t·
It
follows
that
any solution u(x,
t)
of either
8u+
c
8u=O
8t
8x
8u_
c
8u=O
8t
8x
will also solve the homogeneous wave equation.
If
u(x, t) =
f(x
- ct), where f :
R
-t
R
is
any twice differentiable function, then
8u 8u
8t
(x, t) + c
8x
(x, t) =
-c!'(x
- ct) +
c!,(x
- ct) =
O.
Similarly, if g : R
-t
R
is
twice differentiable, then u(x, t) = g(x + ct) satisfies
8u 8u
8t
(x, t) - c
8x
(x, t) = cg'(x + ct) - cg'(x + ct) =
O.
Thus, by linearity, every function of
the
form
u(x, t) =
f(x
- ct) + g(x + ct) (7.2)
is a solution of the homogeneous wave equation.
We
now show
that
(7.2)
is
the
general solution.
To prove
that
(7.2) really represents all possible solutions of the homogeneous
wave equation, it suffices
to
show
that
we
can always solve (7.1) with a function of
A
solution
to
this
is
7.1.
The
homogeneous
wave equation
without
boundaries
287
the
form
(7.2)
(since
any
solution
of the
wave equation satisfies
(7.1)
for
some
ip
and 7).
Moreover,
by
linearity
(the
principle
of
superposition),
it
suffices
to
solve
and
separately
and add the
solutions.
To
find a
solution
to
(7.3),
we
assume
that
u(x,t)
=
f(x
-
ct)
+ g(x +
ct}.
Then
u
solves
the
PDE.
We
want
to
choose
/ and g so
that
u(x,Q)
=
if)(x)
and
du/dt(x,Q}
= 0. But
and
so if we
take
the
required conditions
are
satisfied.
That
is,
is the
solution
of
(7.3).
Finding
a
solution
to
(7.4)
is a bit
harder. With
u(x,t)
=
f(x-ct}+g(x
+
ct),
we
want
u(x,0)
= 0 and
du/dt(x,0]
=
7(#)-
This yields
two
equations:
The first
equation implies
that
/(#)
=
—g(x);
substituting this into
the
second
equation
yields
7.1. The homogeneous
wave
equation without boundaries
287
the
form (7.2) (since any solution of the wave equation satisfies (7.1) for some
'ljJ
and ')'). Moreover, by linearity (the principle of superposition),
it
suffices
to
solve
fPu 2 fPu _ °
at
2
-
c ax
2
- ,
-00
< x <
00,
t > 0,
u(x,O) =
'ljJ(x),
-00
< x <
00,
(7.3)
au
at
(x,O)
= 0,
-00
< x <
00,
and
a
2
u
2a
2
U _
°
at
2
-
c ax
2
- ,
-00
< x <
00,
t > 0,
u(x,O) = 0,
-00
< x <
00,
(7.4)
au
at
(x,O) = ')'(x),
-00
< x <
00,
separately and add
the
solutions.
To find a solution to (7.3),
we
assume
that
u(x, t) =
f(x
- ct) + g(x + ct).
Then u solves the PDE.
We
want to choose f and 9 so
that
u(x,O) =
'ljJ(x)
and
au/at(x,O) =
0.
But
u(x,O) =
f(x)
+ g(x)
and
au
at
(x,O)
= c(g'(x) -
!'(x)),
so if
we
take
1 1
f(x)
=
"2'ljJ(x),
g(x) =
"2'ljJ(x),
the
required conditions are satisfied.
That
is,
1
u(x, t) =
"2
('ljJ(x
- ct) +
'ljJ(x
+ ct))
is the solution of (7.3).
Finding a solution
to
(7.4)
is
a bit harder.
With
u(x, t) =
f(x-ct)
+
g(x+ct),
we
want u(x,O) = 0 and
au/at(x,
0)
= ')'(x). This yields two equations:
f(x)
+ g(x) = 0,
-c!'(x)
+ cg'(x) = ')'(x).
The first equation implies
that
f(x)
=
-g(x);
substituting this into the second
equation yields
2cg'(x) = ')'(x).
A solution
to
this
is
1 r
g(x) =
2c
10
')'(s)
ds.
