Gockenbach M.S. Partial Differential Equations. Analytical and Numerical Methods
Подождите немного. Документ загружается.

If
possible, produce
an
accurate graph
of the
"snapshot"
w(-,
1.0).
8.
Consider
an
iron bar,
of
diameter
4cm and
length
1m,
with
specific
heat
c
=
0.437
J/(gK), density
p =
7.88g/cm
3
,
and
thermal conductivity
K
=
0.836
W/(cm
K).
Suppose
that
the bar is
insulated except
at the
ends,
is
heated
to a
constant temperature
of 5
degrees Celsius,
and the
ends
are
placed
in
an ice
bath
(0
degrees Celsius). Compute
the
temperature (accurate
to 3
digits)
at the
midpoint
of the bar
after
20
minutes. (Warning:
Be
sure
to use
consistent units.)
9.
Repeat Exercise
8
with
a
copper bar:
specific
heat
c =
0.379 J/(gK), density
p
=
8.97g/cm
3
,
thermal conductivity
K =
4.04
W/(cmK).
10.
Consider
the bar of
Exercise
8.
Suppose
the bar is
completely insulated except
at the
ends.
The bar is
heated
to a
constant temperature
of 5
degrees Celsius,
and
then
the
right
end is
placed
in an ice
bath
(0
degrees Celsius),
while
the
other
end is
maintained
at 5
degrees Celsius.
228
Chapter
6.
Heat
flow
and
diffusion
Produce
an
accurate graph
of the
"snapshot"
w(-,0.1).
5.
Consider
the
heat equation with inhomogeneous boundary conditions:
Define
6.
Suppose
V
e
C[0,4
and
(f>
e
C[0,1]
is
defined
by
(j>(y)
=
^(iy).
Show
that
the
sine
coefficients
of
<j>
on
[0,1]
are the
same
as the
sine
coefficients
of ty on
[0,4
7.
Use the
method
of
shifting
the
data
to
solve
the
following
IBVP
with inho-
mogeneous Dirichlet conditions:
and
v(x,i)
=
u(x,t)
—p(x,t).
What IBVP does
v
satisfy?
228
Chapter
6.
Heat flow
and
diffusion
u(x,O) = 0, ° < x < 1,
u(O,
t)
= 0, t > 0,
u(l,
t)
= 0, t > 0.
Produce
an
accurate graph of the "snapshot" u(·,O.I).
5.
Consider the heat equation with inhomogeneous boundary conditions:
au a
2
u
pc
at
-
~
ax
2
=
f(x,
t), ° < x < f,
t>
0,
u(x,
to)
= ¢(x), ° < x < f,
u(O,
t) = g(t), t >
to,
u(f, t) = h(t), t >
to.
Define
x
p(x, t) = g(t) + e(h(t) - g(t))
and
v(x, t) = u(x, t) - p(x, t).
What
IBVP does v satisfy?
6. Suppose
¢ E
C[O,
f), and
1>
E
C[O,
1)
is
defined by
1>(y)
= ¢(fy). Show
that
the sine coefficients of
1>
on [0,1) are the same as
the
sine coefficients of ¢ on
[0,
f).
7.
Use
the
method of shifting
the
data
to
solve the following IBVP with inho-
mogeneous Dirichlet conditions:
au _ a
2
u = ° ° < x < 1, t > 0,
at
ax
2
'
u(x,
0)
=
x,
0 < x < 1,
u(O,
t) =
0,
t > 0,
u(l,
t) =
cos
(t), t >
0.
If
possible, produce an accurate graph of the "snapshot" u(·, 1.0).
8.
Consider an iron bar, of diameter 4 cm and length 1 m, with specific heat
c
= 0.437
J/(gK),
density p = 7.88g/cm
3
,
and
thermal conductivity
~
=
0.836 W / (cm K). Suppose
that
the
bar
is
insulated except
at
the ends,
is
heated
to
a constant temperature of 5 degrees Celsius, and the ends are placed
in
an
ice
bath
(0
degrees Celsius). Compute the temperature (accurate to 3
digits)
at
the midpoint of the bar after
20
minutes. (Warning: Be sure
to
use
consistent units.)
9.
Repeat Exercise 8 with a copper bar: specific heat c =
0.379J/(gK),
density
p = 8.97
g/cm
3
,
thermal conductivity
~
= 4.04 W
/(cmK).
10. Consider the bar of Exercise
8.
Suppose
the
bar
is
completely insulated except
at
the
ends. The
bar
is
heated
to
a constant temperature of 5 degrees Celsius,
and
then the right end
is
placed in
an
ice
bath
(0
degrees Celsius), while the
other end
is
maintained
at
5 degrees Celsius.

6.2
Pure Neumann conditions
and the
Fourier cosine
series
In
this section
we
will
consider
the
effect
of
insulating
one or
both ends
of a bar in
which
heat
is flowing.
36
With
the new
boundary conditions
that
result,
we
must
use
different
eigenfunctions
in the
Fourier series method.
Of
particular
interest
is the
case
in
which
both ends
of the bar are
insulated,
as
this raises some mathematical
questions
that
we
have
not yet
encountered.
6.2.1
One end
insulated; mixed
boundary
conditions
We
begin with
the
case
in
which
one end of the bar is
insulated,
but the
other
is
not.
In
Section 2.1,
we saw
that
an
insulated boundary corresponds
to a
homogeneous
Neumann
condition, which indicates
that
no
heat energy
is flowing
through
that
end
of the
bar.
We
consider
a bar of
length
t,
perfectly
insulated
on the
sides,
and
assume
that
one end (x =
1}
is
perfectly
insulated while
the
other
end (x = 0)
36
Or
closing
one or
both ends
of a
pipe
in
which
a
chemical
is
diffusing.
6.2.
Pure
Neumann
conditions
and the
Fourier
cosine series
229
(a)
What
is the
steady-state temperature distribution
of the
bar?
(b)
How
long does
it
take
the bar to
reach steady-state
(to
within
1%)?
11.
Repeat
the
preceding exercise with
the bar of
Exercise
9.
12.
Consider
a 100 cm
circular bar, with radius
4 cm,
designed
so
that
the
thermal
conductivity
is
nonconstant
and
satisfies
K(X)
= 1 + ax
W/(cmK)
for
some
a > 0.
Assume
that
the
sides
of the bar are
completely insulated,
one end
(x
= 0) is
kept
at 0
degrees Celsius,
and
heat
is
added
to the
other
end at
a
rate
of 4 W. The
temperature
of the bar
reaches
a
steady
state,
u
=
u(x),
and
the
temperature
at the end x = 100 is
measured
to be
Estimate
a.
13.
Compute
the
Fourier sine
coefficients
of
each
of the
following
functions,
and
verify
the
statements
on
page
216
concerning
the
rate
of
decay
of the
Fourier
coefficients.
(Take
the
interval
to be
[0.11.)
6.2. Pure Neumann conditions
and
the Fourier cosine
series
229
(a)
What
is
the steady-state temperature distribution of the bar?
(b)
How
long does it take the bar to reach steady-state (to within 1
%)?
11.
Repeat
the
preceding exercise with the bar of Exercise
9.
12. Consider a 100cm circular bar, with radius 4cm, designed
so
that
the thermal
conductivity
is
nonconstant and satisfies
II;
(x ) = 1 +
o::x
W / (cm
K)
for some
0::
>
O.
Assume
that
the
sides of the bar are completely insulated, one end
(x =
0)
is
kept
at
0 degrees Celsius, and heat
is
added
to
the other end
at
a
rate
of 4 W. The temperature of the
bar
reaches a steady state, u = u(x),
and the temperature
at
the end x =
100
is
measured
to
be
u(100)
==
7.6 degrees Celsius.
Estimate
0::.
13. Compute the Fourier sine coefficients of each of
the
following functions, and
verify
the
statements on page
216
concerning the rate of decay of the Fourier
coefficients. (Take the interval to be [0,1].)
(a)
(b)
f(x)
= {
x,
2 - 2x,
Os
x <
1/2,
1/2
S x S
1.
{
X
0 s x <
1/2,
f(x)=
1'-x,I/2SxS1.
6.2 Pure Neumann conditions and the Fourier
cosine
.
series
In this section
we
will consider the effect of insulating one or
both
ends of a
bar
in
which heat
is
flowing.
36
With
the
new boundary conditions
that
result,
we
must use
different eigenfunctions in
the
Fourier series method. Of particular interest
is
the
case in which
both
ends of the bar are insulated, as this raises some mathematical
questions
that
we
have not yet encountered.
6.2.1 One
end
insulated; mixed boundary conditions
We
begin with the case in which one end of the
bar
is
insulated,
but
the other
is
not.
In Section 2.1,
we
saw
that
an insulated boundary corresponds
to
a homogeneous
Neumann condition, which indicates
that
no heat energy
is
flowing through
that
end of the bar.
We
consider a bar of length
C,
perfectly insulated on the sides, and
assume
that
one end (x =
C)
is
perfectly insulated while the other end (x =
0)
360r
closing one
or
both
ends of a pipe in which a chemical is diffusing.
then
u
will
satisfy
the
boundary conditions.
Just
as in the
previous section,
we
can
derive
an ODE
with
an
initial condition
for
each
coefficient
a
n
(t)
and
thereby
determine
u. We
will
illustrate with
an
example.
Example
6.4.
We
consider
the
temperature distribution
in the
iron
bar
of
Example
6.1,
with
the
experimental conditions unchanged
except
that
the
right
end (x =
I)
of
the bar is
perfectly
insulated.
The
temperature distribution
u(x,t)
then
satisfies
the
IBVP
We
have
230
Chapter
6.
Heat flow
and
diffusion
is
uninsulated
and
placed
in an ice
bath.
If the
initial temperature distribution
is
^(x),
then
the
temperature distribution
u(x,t)
satisfies
the
IBVP
The
eigenvalue/eigenfunctions
pairs
for
L
m
,
the
negative second derivative operator
under
the
mixed boundary conditions (Dirichlet
at the
left,
Neumann
at the
right
are
(see
Section 5.2.3).
If we
represent
the
solution
as
where
p =
7.88g/cm
3
,
c =
QA37J/(gK),
K
=
0.836
W/(cmK),
and
where
230
Chapter
6.
Heat
flow
and
diffusion
is
uninsulated and placed in
an
ice bath.
If
the
initial temperature distribution
is
'l/J(x),
then
the
temperature distribution
u(x,
t) satisfies
the
IBVP
au
a
2
u
pc
at
-
/l,
ax
2
=
f(x,
t),
° < x <
£,
t>
to,
u(x,
to) =
'l/J(x)
, ° < x <
£,
(6.8)
u(O, t) = 0, t > to,
au
ax
(£,
t) = 0, t > to·
The
eigenvalue/eigenfunctions pairs for L
m
,
the
negative second derivative operator,
under the mixed boundary conditions (Dirichlet
at
the
left, Neumann
at
the
right)
are
(2n-1)21f2 .
((2n-1)1fx)
4£2
' sm
2£
' n =
1,2,3,
...
(see Section 5.2.3).
If
we
represent the solution as
~
.
((2n
-
l)1fX)
u(x,
t) =
~
an(t) sm
2£
'
then u will satisfy the boundary conditions.
Just
as in
the
previous section,
we
can derive
an
ODE with
an
initial condition for each coefficient an(t) and thereby
determine u.
We
will illustrate with
an
example.
Example
6.4.
We consider the temperature distribution
in
the iron bar
of
Example
6.1,
with the experimental conditions unchanged except that the right end (x = £)
of
the bar is perfectly insulated. The temperature distribution
u(x,
t) then satisfies
the
IBVP
au
a
2
u
pc
at
- /l,
ax
2
=
0,
° < x < 50, t >
0,
u(x,O) =
'l/J(x),
° < x < 50,
u(O, t) = 0, t > 0,
du
dx
(50,
t)
=
0,
t >
0,
where p = 7.88
g/
cm
3
,
c = 0.437
J/
(g
K), /l, = 0.836
W/
(cm K), and
1
'l/J(x)
= 5 -
Six
-
251·
We have
~
.
((2n-1)1f)
'l/J(x)
=
~cnsm
100 '
where
_
~
(50.,,( ) .
((2n
-l)1fX)
d
c
n
-
50
J 0
'f'
X sm 100 x
80
(-\1'2
sin
(n1f
/2)
+
\1'2
cos (n1f/2) -
(-l)n)
= -
2()2
' n =
1,2,3,
....
1f
2n-1
6.2. Pure Neumann
conditions
and the
Fourier cosine series
231
We
write
the
solution
u in the
form
which
determines
u(x,t).
In
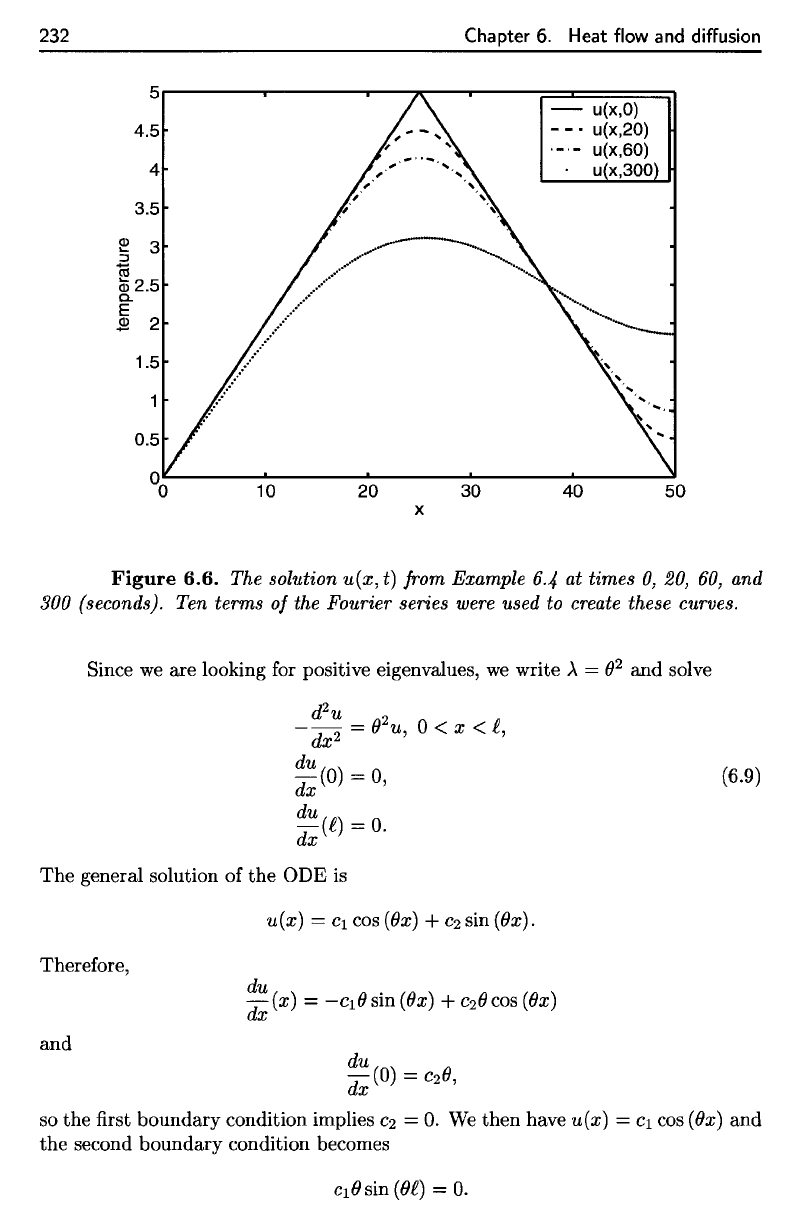
Figure 6.6,
we
show
the
temperature distributions
at
times
t =
20,
t =
60,
and
t =
300; this
figure
should
be
compared
with Figure 6.1.
The
effect
of
insulating
the
right
end is
clearly
seen.
6.2.2
Both
ends
insulated;
Neumann
boundary
conditions
In
order
to
treat
the
case
of
heat
flow in a bar
with both ends insulated,
we
must
first
analyze
the
negative second derivative operator under Neumann conditions.
We
define
Unlike
the
negative second derivative operator under
the
other sets
of
boundary
conditions
we
have considered,
LN
has a
nontrivial
null space; indeed,
L^u
= 0 if
and
only
if u is a
constant function (see Example
3.17).
Therefore,
AQ
= 0 is an
eigenvalue
of
LN
with eigenfunction
70
(x)
= 1. It is
straightforward
to
show
that
LJV
is
symmetric,
so
that
its
eigenvalues
are
real
and
eigenfunctions corresponding
to
distinct eigenvalues
are
orthogonal. Moreover,
apart
from
AQ,
the
other eigenvalues
of
Z/jv
are
positive (see Exercise
3). We now
determine these other eigenvalues
and
the
corresponding eigenfunctions.
Then,
by
almost
exactly
the
same computation
as on
page
212,
we
have
The
PDE and
initial condition
for u
then
imply
that
the
coefficient
a
n
(t)
must
satisfy
the
following
IVP:
The
solution
is
6.2. Pure Neumann conditions and the Fourier cosine series
231
We write the solution u
in
the
form
~
.
((2n
-1)1fX)
u(x,
t) =
~
an(t) sm 100 .
Then, by almost exactly the same computation
as
on page 212, we have
The
PDE
and initial condition for u then imply that the coefficient an(t)
must
satisfy
the following
IVP:
dan
~(2n
-
1)21f2
pc dt +
4.50
2
an = 0,
an(O) = c
n
·
The solution is
which determines
u(x,
t).
In
Figure 6.6, we show the temperature distributions at
times
t = 20, t =
60,
and t =
300;
this figure should
be
compared with Figure 6.1. The effect
of
insulating
the right end is clearly seen.
6.2.2 Both
ends
insulated; Neumann boundary conditions
In order to
treat
the case of heat
flow
in a
bar
with both ends insulated,
we
must
first analyze
the
negative second derivative operator under Neumann conditions.
We
define
e1[0,
e)
=
{u
E e
2
[0,
e)
:
~~(O)
=
~~(e)
= o},
and
LN
:
eF,r[o,
£)
-+
e[o,
£}
by
Unlike
the
negative second derivative operator under the other sets of boundary
conditions
we
have considered,
LN
has a nontrivial null space; indeed,
LNu
= ° if
and only if
u
is
a constant function (see Example 3.17). Therefore,
AD
= °
is
an
eigenvalue of
LN
with eigenfunction 'Yo(x) = 1.
It
is
straightforward
to
show
that
LN
is
symmetric,
so
that
its eigenvalues are real and eigenfunctions corresponding
to
distinct eigenvalues are orthogonal. Moreover,
apart
from
AD,
the other eigenvalues
of
LN
are positive (see Exercise 3).
We
now determine these other eigenvalues and
the
corresponding eigenfunctions.
232
Chapter
6.
Heat
flow
and
diffusion
Figure
6.6.
The
solution
u(x,t)
from Example
6.4 at
times
0, 20, 60, and
300
(seconds).
Ten
terms
of the
Fourier series
were
used
to
create
these curves.
Since
we axe
looking
for
positive eigenvalues,
we
write
A =
0
2
and
solve
and
so the first
boundary condition implies
C2
= 0. We
then
have
u(x]
=
c\
cos
(Ox)
and
the
second boundary condition becomes
The
general solution
of the ODE is
Therefore,
232
Chapter
6.
Heat flow
and
diffusion
5r-----~----~--~--~--~:%====~
4.5
4
3.5
~
3
::J
Cil
Q52.5
a.
E
.$
2
1.5
10
20
30
40
50
x
Figure
6.6.
The solution
u(x,
t) from Example
6.4
at times
0,
20,
60,
and
300 {seconds}. Ten terms
of
the Fourier series were used to create these curves.
Since
we
are looking for positive eigenvalues,
we
write>. =
(j2
and
solve
(6.9)
The
general solution of
the
ODE is
Therefore,
and
du
dx
(0)
= c
2
fJ,
so
the
first boundary condition implies
C2
=
O.
We
then
have
u(x)
=
Cl
cos
(Ox)
and
the
second boundary condition becomes
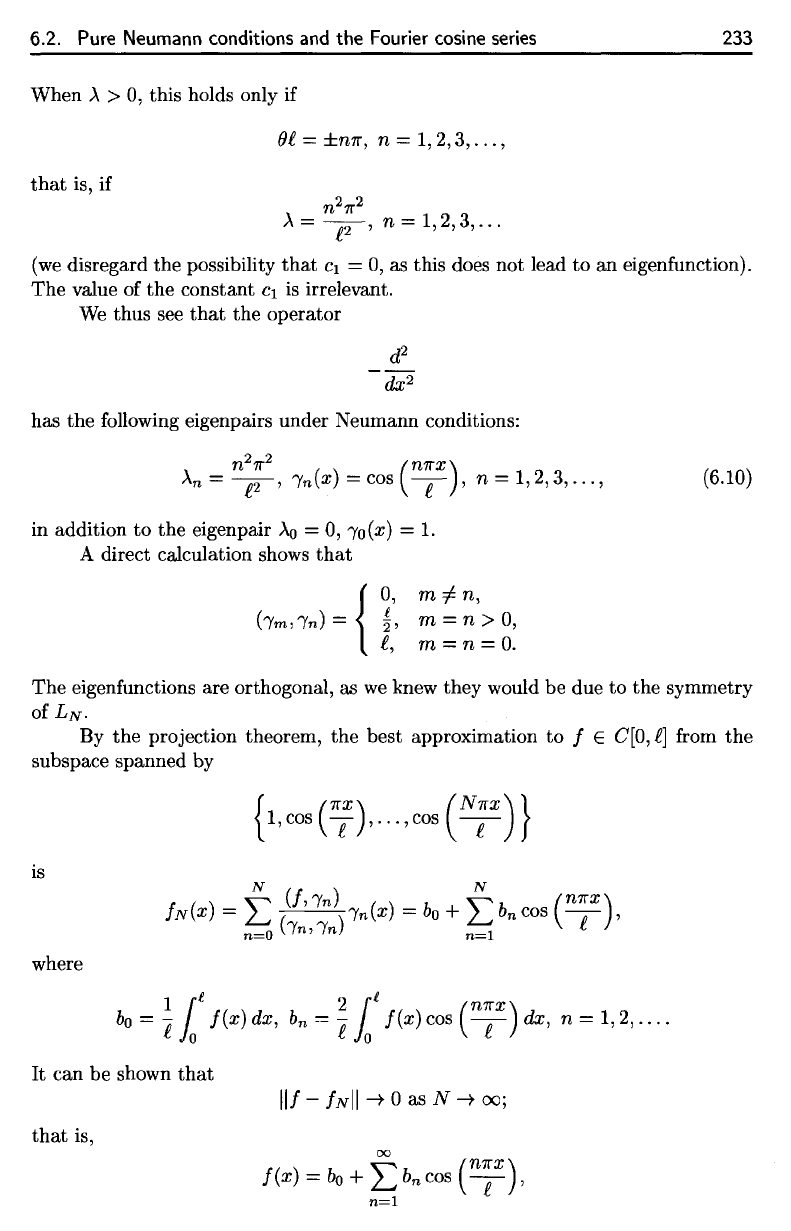
in
addition
to the
eigenpair
AQ
= 0,
7o(#)
=
1-
A
direct calculation shows
that
6.2. Pure Neumann conditions
and the
Fourier cosine
series
233
When
A > 0,
this holds only
if
that
is, if
(we
disregard
the
possibility
that
c\
= 0, as
this does
not
lead
to an
eigenfunction).
The
value
of the
constant
c\
is
irrelevant.
We
thus
see
that
the
operator
has the
following
eigenpairs under Neumann
conditions
The
eigenfunctions
are
orthogonal,
as we
knew
they would
be due to the
symmetry
of
I/AT-
By
the
projection theorem,
the
best approximation
to /
G
C*[0,£]
from
the
subspace
spanned
by
is
where
It can be
shown
that
that
is,
6.2. Pure Neumann conditions
and
the Fourier cosine
series
233
When A >
0,
this holds only if
B£=±n7l",
n=1,2,3, ... ,
that
is, if
n
2
7l"2
>..=
T'
n=
1,2,3,
...
(we
disregard the possibility
that
Cl
= 0, as this does not lead
to
an
eigenfunction).
The
value of
the
constant
Cl
is
irrelevant.
We
thus see
that
the operator
has the following eigenpairs under Neumann conditions:
(6.10)
in addition
to
the eigenpair
>"0
= 0,
'Yo(x)
=
l.
A direct calculation shows
that
{
0,
m",
n,
("(m,'Yn)
=
~,
m=n>O,
£,
m = n = 0.
The
eigenfunctions are orthogonal, as
we
knew they would be due to the symmetry
of
LN.
By
the
projection theorem, the best approximation
to
f E
e[O,
£]
from
the
subspace spanned by
{ 1, cos
(7l"£X),
... , cos (N;X) }
is
where
1 r
l
2
rl
(n7l"X)
bo
= e
10
f(x)
dx, b
n
= e
10
f(x)
cos
-£-
dx, n =
1,2,
....
It
can be shown
that
Ilf
-
fN"
-t
° as N
-t
00;
that
is,
00
f(x)
= b
o
+ L bncos
C;X),
n=l
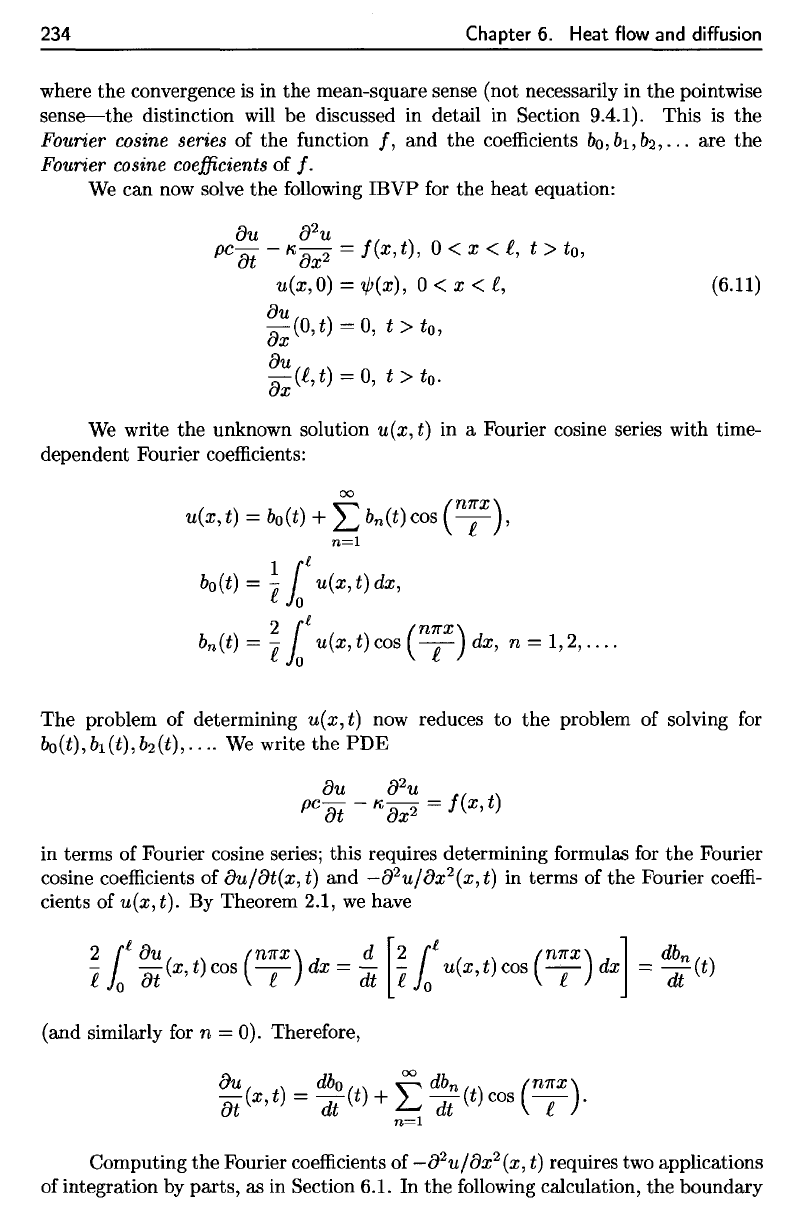
in
terms
of
Fourier cosine series; this requires determining formulas
for the
Fourier
cosine
coefficients
of
du/dt(x,t)
and
—d
2
u/dx
2
(x,t)
in
terms
of the
Fourier
coeffi-
cients
of
u(x,
t).
By
Theorem 2.1,
we
have
(and similarly
for n =
0).
Therefore,
Computing
the
Fourier
coefficients
of
—d
2
u/dx
2
(x,
t)
requires
two
applications
of
integration
by
parts,
as in
Section 6.1.
In the
following
calculation,
the
boundary
234
Chapter
6.
Heat
flow
and
diffusion
where
the
convergence
is in the
mean-square sense (not necessarily
in the
pointwise
sense—the
distinction
will
be
discussed
in
detail
in
Section
9.4.1).
This
is the
Fourier
cosine
series
of the
function
/, and the
coefficients
6
0
,&i,&2,---
are the
Fourier
cosine
coefficients
of /.
We
can now
solve
the
following
IBVP
for the
heat
equation:
The
problem
of
determining
u(x,t)
now
reduces
to the
problem
of
solving
for
b
0
(t),bi(t),b
2
(t),....
We
write
the PDE
We
write
the
unknown solution
u(x,
t) in a
Fourier cosine series with time-
dependent Fourier
coefficients:
234 Chapter
6.
Heat
flow
and diffusion
where
the
convergence is in
the
mean-square sense (not necessarily
in
the
pointwise
sense-the
distinction will
be
discussed in detail in Section 9.4.1). This is
the
Fourier cosine series of
the
function f,
and
the
coefficients b
o
, b
1
,
b
2
, •
••
are
the
Fourier cosine coefficients
of
f.
We
can now solve
the
following
IBVP
for
the
heat
equation:
oU
02U
pc
ot
-
'"
ox
2
=
f(x,
t), ° < x <
£,
t >
to,
u(x,
0)
=
'I/J(x),
° < x <
£,
OU
ox
(0,
t) =
0,
t>
to,
au
ox
(£,
t) = 0, t >
to·
(6.11)
We
write
the
unknown solution u(x, t) in a Fourier cosine series with time-
dependent Fourier coefficients:
00
u(x, t) =
bo(t)
+
2:
bn(t) cos
(n;x),
n=l
1
(-
bo(t)
= i io u(x, t) dx,
2
(l
(n7rx)
bn(t)
= i io u(x, t) cos -c- dx, n =
1,2,
....
The
problem
of
determining u(x, t) now reduces
to
the
problem
of
solving for
bo(t), b
1
(t), b
2
(t),
....
We
write
the
PDE
in terms
of
Fourier cosine series; this requires determining formulas for
the
Fourier
cosine coefficients
of
ou/ot(x,
t)
and
-o2u/ax
2
(x,
t) in terms of
the
Fourier coeffi-
cients of u(x, t).
By
Theorem 2.1,
we
have
2
{lou
(n7rx) d
[2
{l
(n7rx) 1
db
n
i
io
ot
(x, t) cos -c- dx =
dt
i
io
u(x, t) cos
-£-
dx =
dt(t)
(and
similarly for n = 0). Therefore,
au
db
o
~
db
n
(n7rx)
ot
(x, t) =
(it(t)
+
~
dt(t)
cos -c- .
n=l
Computing
the
Fourier coefficients of
-02u/ox
2
(x,
t) requires two applications
of integration by
parts,
as in Section 6.1.
In
the
following calculation,
the
boundary
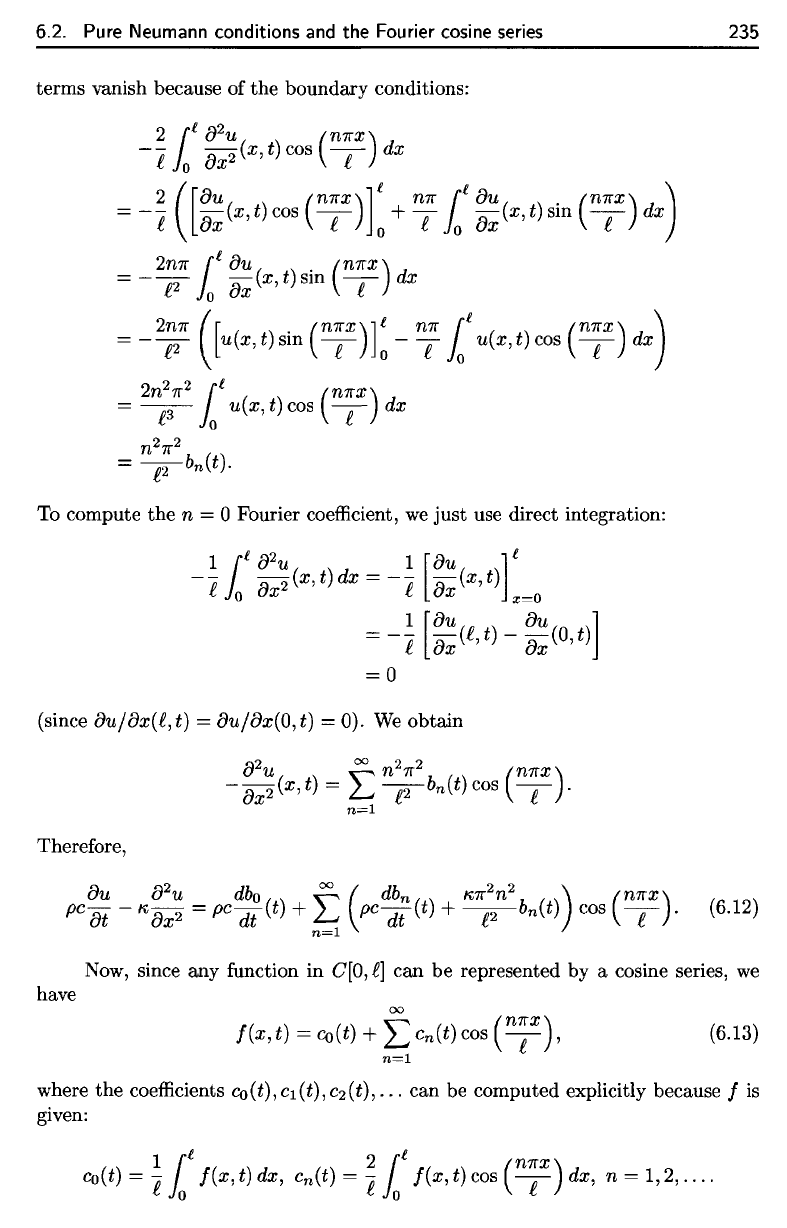
6.2. Pure Neumann conditions
and the
Fourier cosine
series
235
terms vanish because
of the
boundary conditions:
To
compute
the n = 0
Fourier
coefficient,
we
just
use
direct integration:
(since
du/dx(l,t)
—
du/dx(Q,t]
= 0). We
obtain
Therefore,
Now,
since
any
function
in
C[0,£]
can be
represented
by a
cosine series,
we
have
where
the
coefficients
CQ
(t},
c\
(t),
c^
(t),...
can be
computed explicitly because
/ is
given:
6.2. Pure Neumann conditions
and
the Fourier cosine
series
235
terms vanish because of
the
boundary conditions:
2 r
l
a
2
u
(n7rx)
-""i
io
ax2
(x,
t) cos
-e-
dx
2
([au
(n7rx)]l
n7r
(au
.
(n7rx)
)
=
-""i
ax
(x,
t) cos
-e-
0 + T
io
ax
(x,
t) sm
-e-
dx
2n7r
r
l
au
.
(n7rx)
=
-~
io
ax
(x,
t) sm
-e-
dx
2n7r
([ .
(n7rx)]l
n7r
r
l
(n7rx))
=
-~
u(x,
t) sm
-e-
0 - T
io
u(x,
t) cos
-e-
dx
2n
2
7r
2
r
l
(n7rx)
=
~
io
u(x,
t) cos
-e-
dx
n
2
7r
2
=
----g2b
n
(t).
To compute
the
n = 0 Fourier coefficient,
we
just
use direct integration:
(since
aujax(e,
t) =
aujax(O,
t) = 0).
We
obtain
Therefore,
(6.12)
Now, since any function in
C[O,e]
can be represented by a cosine series,
we
have
=
f(x,
t) = eo(t) + L cn(t) cos (n;x),
n=l
(6.13)
where
the
coefficients cO(t),Cl(t),C2(t),
...
can be computed explicitly because f
is
given:
1 r
l
2 r
l
(n7rx)
co(t) =
""i
io
f(x,
t)
dx,
cn(t) =
""i
io
f(x,
t) cos
-e-
dx,
n =
1,2,
....
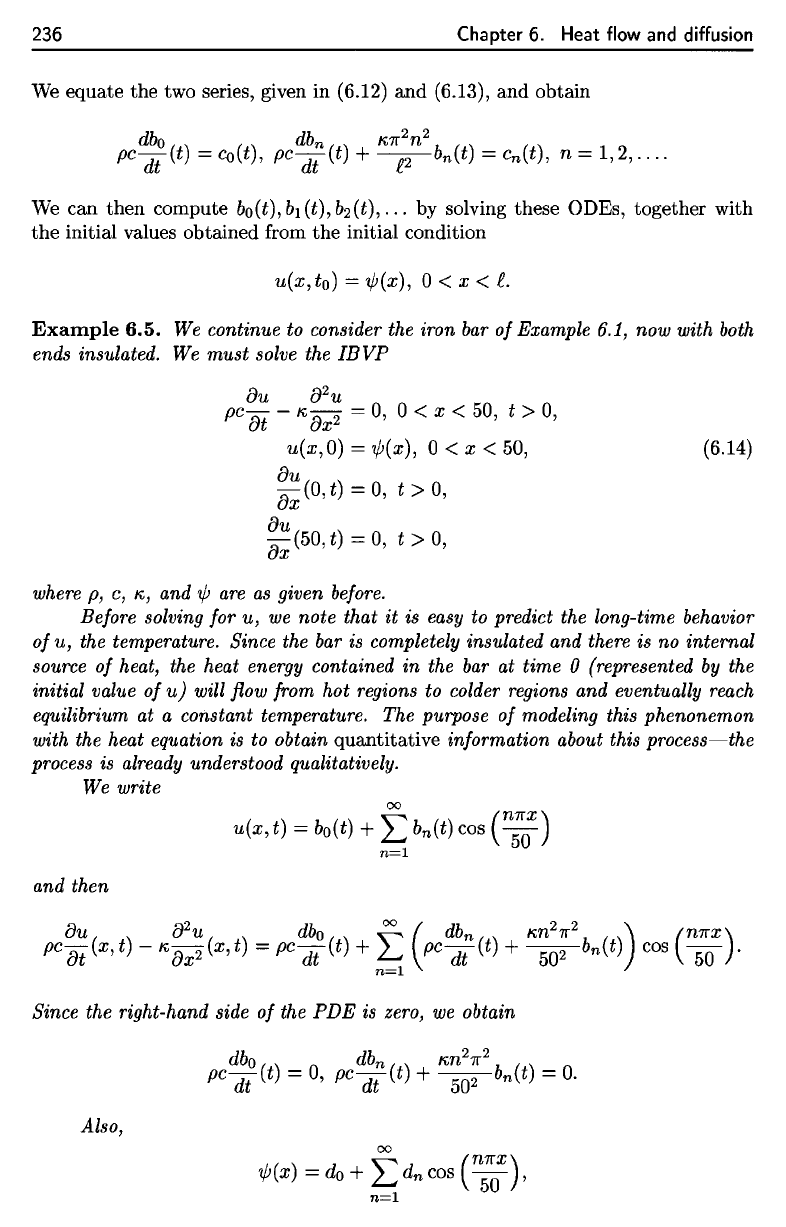
where
p, c,
K,
and
ip
are as
given
before.
Before
solving
for
u,
we
note that
it is
easy
to
predict
the
long-time behavior
ofu,
the
temperature. Since
the bar is
completely insulated
and
there
is no
internal
source
of
heat,
the
heat
energy
contained
in the bar at
time
0
(represented
by the
initial value
of
u)
will
flow
from
hot
regions
to
colder
regions
and
eventually reach
equilibrium
at a
constant temperature.
The
purpose
of
modeling this
phenonemon
with
the
heat equation
is to
obtain
quantitative
information about this
process—the
process
is
already
understood qualitatively.
We
write
and
then
Since
the
right-hand side
of the PDE is
zero,
we
obtain
Also,
Example
6.5.
We
continue
to
consider
the
iron
bar
of
Example 6.1,
now
with
both
ends
insulated.
We
must solve
the
IBVP
We
can
then compute
bo(t),b\(t),b<2,(t),...
by
solving these ODEs,
together
with
the
initial values
obtained
from
the
initial condition
We
equate
the two
series, given
in
(6.12)
and
(6.13),
and
obtain
236
Chapter
6.
Heat
flow
and
diffusion
236
Chapter
6.
Heat
flow
and
diffusion
We
equate
the
two series, given in (6.12)
and
(6.13), and obtain
We
can then compute bo(t), b
l
(t), b
2
(t),
...
by solving these ODEs, together with
the
initial values obtained from the initial condition
u(x,
to) = ,¢(x),
0<
x <
e.
Example
6.5.
We continue to consider the iron
bar
of
Example 6.1, now with
both
ends insulated. We
must
solve the
IB
VP
au
a
2
u
pc
at
- K,
ax
2
=
0,
° < x < 50, t > 0,
u(x,O) = ,¢(x), ° < x < 50,
(6.14)
au
ax
(0, t) =
0,
t >
0,
au
ax
(50, t) =
0,
t >
0,
where
p,
c,
K"
and
'¢
are
as
given before.
Before solving for u,
we
note that
it
is easy to predict the long-time behavior
of
u,
the temperature. Since the
bar
is
completely insulated and there is no internal
source
of
heat, the heat energy contained in the
bar
at time 0 (represented
by
the
initial value
of
u)
will flow from hot regions to colder regions and eventually reach
equilibrium at a constant temperature. The purpose
of
modeling this phenonemon
with the heat equation
is to obtain quantitative information about this
process-the
process is already understood qualitatively.
We write
00
u(x,
t) = bo(t) +
2..:
bn(t) cos
(n:ox)
n=l
and then
Since the right-hand side
of
the
PDE
is zero,
we
obtain
Also,
00
,¢(x) =
do
+
2..:
d
n
cos
(n:ox)
,
n=1
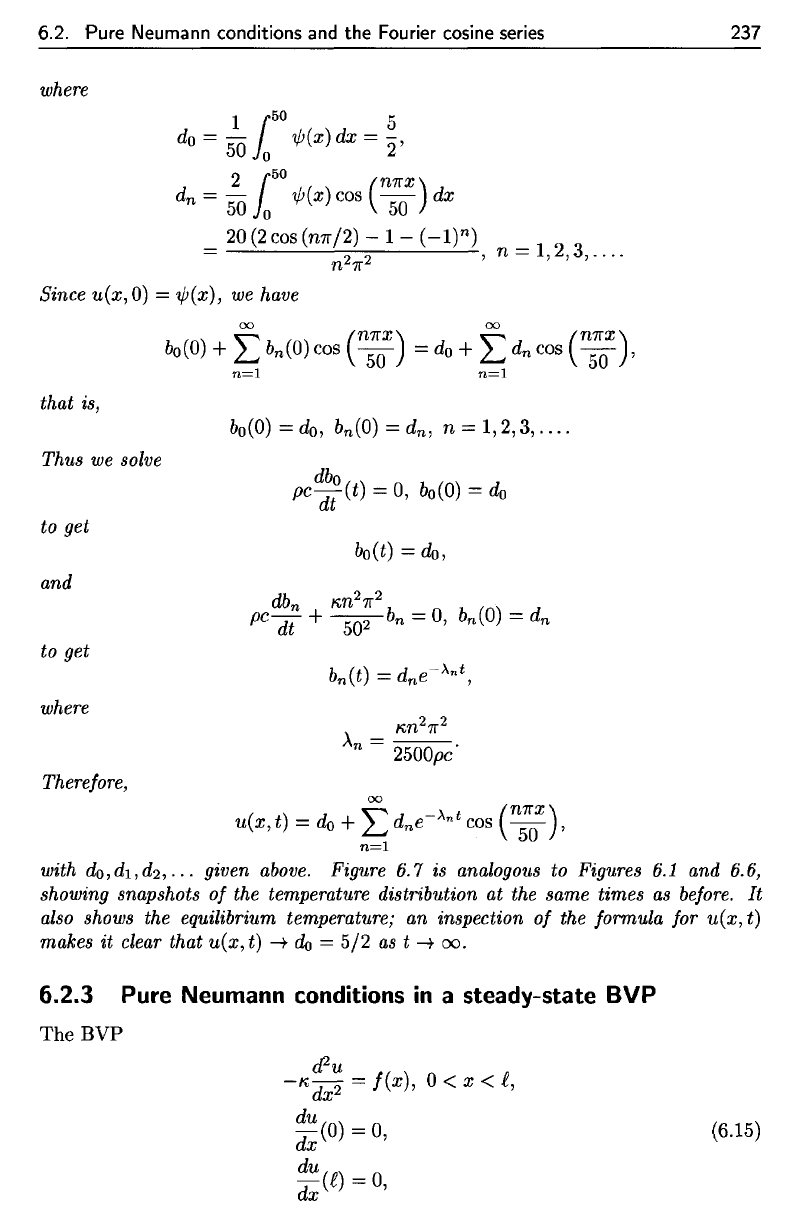
with
^0,^1,^2,...
given above. Figure
6.7 is
analogous
to
Figures
6.1 and
6.6,
showing
snapshots
of the
temperature distribution
at the
same
times
as
before.
It
also
shows
the
equilibrium temperature;
an
inspection
of the
formula
for
u(x,t)
makes
it
clear that
u(x,t}
->
do = 5/2 as t
—>
oo.
6.2.
Pure
Neumann
conditions
and the
Fourier cosine series
237
where
Since
w(#,0)
=
i£>(x),
we
have
that
is,
Thus
we
solve
to get
and
to get
where
Therefore,
6.2.3 Pure
Neumann
conditions
in a
steady-state
BVP
The BVP
6.2. Pure Neumann conditions
and
the Fourier cosine
series
237
where
1
(50
5
do
= 5010
'l/J(x)
dx
= 2'
2
(50
(n1fX)
d
n
= 5010
'l/J(x)
cos
50
dx
20
(2
cos (n1f
/2)
- 1 -
(-1)n)
=
22
,n=1,2,3,
....
n1f
Since
u(x,O)
= 'l/J(x), we have
that
is,
bo(O)
= do,
bn(O)
= d
n
, n =
1,2,3,
....
Thus we solve
to get
bo(t)
=d
o
,
and
to
get
where
Therefore,
00
u(x,t)
=
do
+
2:
dne->'nt cos
(n1fx)
,
n=l
50
with d
o
,d
1
,d
2
,
...
given above. Figure 6.7 is analogous to Figures
6.1
and 6.6,
showing snapshots
of
the temperature distribution
at
the
same
times
as before.
It
also shows the equilibrium temperature; an inspection
of
the formula for
u(x,
t)
makes
it
clear
that
u(x,
t)
--+
do
=
5/2
as t
--+
00.
6.2.3 Pure Neumann conditions
in
a steady-state BVP
The
BVP
~u
-r;,
dx
2
=
f(x),
0 < x <
e,
~~
(0) = 0,
~~(e)
= 0,
(6.15)
