Gibilisco S. Everyday Math Demystified: A Self-Teaching Guide

Подождите немного. Документ загружается.

or, rearranged to get rid of the need for parentheses:

r ¼ 2=p

PROBLEM 11-6

What is the value of the constant, a, in the cardioid shown in Fig. 11-17?

What is the equation of this cardioid? Assume that each radial division

represents 1 unit.

SOLUTION 11-6

Note that if  ¼ 0, then r ¼ 4. We can solve for a by substituting this number

pair in the general equation for the cardioid. We know that (, r) ¼ (0, 4).

Proceed like this:

r ¼ 2að1 þ cos Þ

4 ¼ 2að1 þ cos 0Þ

4 ¼ 2að1 þ 1Þ

4 ¼ 4a

a ¼ 1

This means that the equation of the cardioid is:

r ¼ 2ð1 þ cos Þ

or, rearranged to get rid of the need for parentheses:

r ¼ 2 þ 2 cos 

Quiz

Refer to the text in this chapter if necessary. A good score is eight correct.

Answers are in the back of the book.

1. What is the distance between the points (3,4) and (–3,4) in Cartesian

coordinates?

(a) 10

(b) 8

(d) 5

2. Suppose a straight line in Cartesian coordinates is represented by the

following equation:

3x  6y þ 2 ¼ 0

CHAPTER 11 Graphing It 271

What is the slope of this line?

(a) 2

(b) 3/2

(d) 1/2

3. What is the distance between the origin and the point (8, 8) in

Cartesian coordinates?

(a) 16

(b) 8

1/2

(d) 8

1/2

4. Suppose the equation of a line in Cartesian coordinates is speciﬁed as:

y  3 ¼ 7ðx þ 2Þ

From this equation, we know that the line passes through the point

(a) (2,0)

(b) (0, 3)

(d) none of the above

5. What does the graph of  ¼ 0 look like in polar coordinates?

(a) A point.

(b) A straight line.

(d) A circle.

6. The term ordinate in Cartesian coordinates refers to

(a) the direction

(b) the value of the dependent variable

(d) the angle

7. A radian is equivalent to approximately

(a) 114.68

(b) 908

(d) 28.78

8. The direction of a point, relative to the origin, in conventional polar

coordinates is expressed as an angle going

(a) counterclockwise from the axis running from the origin

upwards

PART 3 Shapes and Places

272

(b) counterclockwise from the axis running from the origin towards

the left

downwards

(d) counterclockwise from the axis running from the origin towards

the right

9. What is the distance between the origin and (r, ) ¼ (4, 1358) in polar

coordinates?

(a) 0

(b) 4

(d) It is impossible to determine this without more information.

10. A straight line has a slope of –3 in Cartesian coordinates, and

runs through the point (0,2). The equation of this line is

(a) y ¼ –3x þ 2

(b) y ¼ 2x  3

(d) impossible to determine without more information

CHAPTER 11 Graphing It 273

CHAPTER

A Taste of

Trigono metry

Trigonometry (or ‘‘trig’’) involves angles and distances. Trigonometry scares

some people because of the Greek symbology, but the rules are clear-cut.

Once you can get used to the idea of Greek letters representing angles, and

if you’re willing to draw diagrams and use a calculator, basic trigonometry

loses most of its fear-inspiring qualities. You might even ﬁnd yourself having

fun with it.

More about Circles

Circles are deﬁned by equations in which either x or y can be considered

the dependent variable. We’ve already looked at the graphs of some simple

circles in the xy-plane. Let’s look more closely at this special species of

geometric ﬁgure.

274

GENERAL EQUATION OF A CIRCLE

The equation in the xy-plane that represents a circle depends on two things:

the radius of the circle, and the location of its center point.

Suppose r is the radius of a circle, expressed in arbitrary units. Imagine

that the center point of the circle in Cartesian coordinates is located at

the point x ¼ a and y ¼ b, represented by the ordered pair (a, b). Then the

equation of that circle is:

ðx  aÞ

þðy  bÞ

¼ r

If the center of the circle happens to be at the origin, that is, at (0, 0) on the

coordinate plane, then the general equation is simpler:

þ y

¼ r

THE UNIT CIRCLE

Consider a circle in rectangular coordinates with the following equation:

þ y

¼ 1

This is called the unit circle because its radius is 1 unit, and it is centered

at the origin (0, 0). This circle gives us a good way to deﬁne the common

trigonometric functions, which are sometimes called circular functions.

IT’S GREEK TO US

In trigonometry, mathematicians and scientists have acquired the habit of

using Greek letters to represent angles. The most common symbol for this

purpose is an italic, lowercase Greek theta (pronounced ‘‘THAY-tuh’’).

It looks like an italic numeral 0 with a horizontal line through it ().

When writing about two diﬀerent angles, a second Greek letter is used

along with . Most often, it is the italic, lowercase letter phi (pronounced

‘‘FIE’’ or ‘‘FEE’’). This character looks like an italic lowercase English

letter o with a forward slash through it ().

Sometimes the italic, lowercase Greek alpha (‘‘AL-fuh’’), beta (‘‘BAY-

tuh’’), and gamma (‘‘GAM-uh’’) are used to represent angles. These,

respectively, look like this: , , . When things get messy and there are a

lot of angles to talk about, numeric subscripts are sometimes used with

Greek letters, so don’t be surprised if you see text in which angles are denoted



, 

, and so on.

CHAPTER 12 A Taste of Trigonometry 275

ANGULAR UNITS

There are two main units by which the measures of angles in a ﬂat plane

can be speciﬁed: the radian and the degree. The radian was deﬁned in the

previous chapter. A quarter circle is p/2 rad, a half circle is p rad, and

three quarters of a circle is 3p/2 rad. Mathematicians prefer to use the radian

when working with trigonometric functions, and the abbreviation ‘‘rad’’ is

left out. So if you see something like 

¼ p/4, you know the angle 

expressed in radians.

The angular degree (8), also called the degree of arc, is the unit of angular

measure most familiar to lay people. One degree (18) is 1/360 of a full circle.

An angle of 908 represents a quarter circle, 1808 represents a half circle, 2708

represents three quarters of a circle, and 3608 represents a full circle. A right

angle has a measure of 908, an acute angle has a measure of more than 08

but less than 908, and an obtuse angle has a measure of more than 908 but

less than 1808.

To denote the measures of tiny angles, or to precisely denote the measures

of angles in general, smaller units are used. One minute of arc or arc minute,

symbolized by an apostrophe or accent (

) or abbreviated as m or min, is 1/60

of a degree. One second of arc or arc second, symbolized by a closing

quotation mark (

) or abbreviated as s or sec, is 1/60 of an arc minute or

1/3600 of a degree. An example of an angle in this notation is 308 15

which is read as ‘‘30 degrees, 15 minutes, 0 seconds.’’

Alternatively, fractions of a degree can be denoted in decimal form. You

might see, for example, 30.258. This is the same as 308 15

. Decimal frac-

tions of degrees are easier to work with than the minute/second scheme when

angles must be added and subtracted, or when using a conventional calcu-

lator to work out trigonometry problems. Nevertheless, the minute/second

system, like the English system of measurements, remains in widespread use.

PROBLEM 12-1

A text discussion tells you that 

¼ p/4. What is the measure of 

in degrees?

SOLUTION 12-1

There are 2p rad in a full circle of 3608. The value p/4 is equal to 1/8 of 2p.

Therefore, the angle 

is 1/8 of a full circle, or 458.

PROBLEM 12-2

Suppose your town is listed in an almanac as being at 408 20

north latitude

and 938 48

west longitude. What are these values in decimal form? Express

your answers to two decimal places.

PART 3 Shapes and Places

276

SOLUTION 12-2

There are 60 minutes of arc in one degree. To calculate the latitude, note that

¼ (20/60)8 ¼ 0.338; that means the latitude is 40.338 north. To calculate

the longitude, note that 48

¼ (48/60)8 ¼ 0.808; that means the longitude is

93.808 west.

Primary Circular Functions

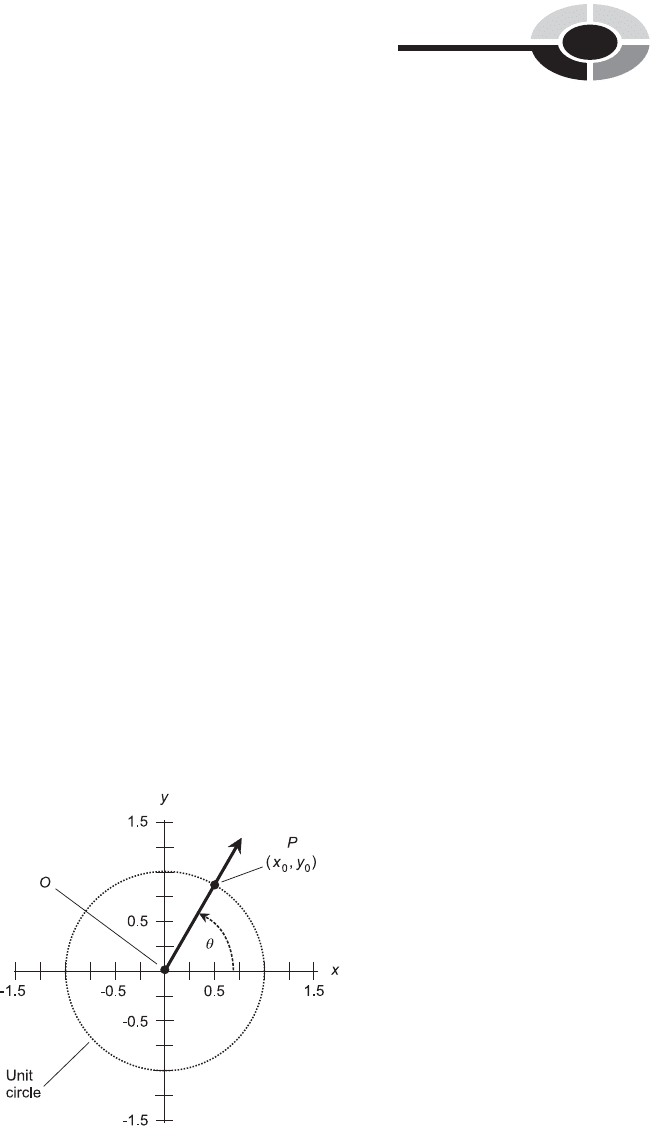
Consider a circle in the Cartesian xy-plane with the following equation:

þ y

¼ 1

This equation, as deﬁned earlier in this chapter, represents the unit circle.

Let  be an angle whose apex is at the origin, and that is measured counter-

clockwise from the x axis, as shown in Fig. 12-1. Suppose this angle corre-

sponds to a ray that intersects the unit circle at some point P ¼ (x

, y

). We

can deﬁne the three basic circular functions, also called the primary

circular functions, of the angle  in an elegant way. But before we get into

this, let’s extend our notion of angles to cover negative values, and also to

cover values more than 3608 (2p rad).

OFFBEAT ANGLES

In trigonometry, any direction angle expressible as a real number, no matter

how extreme, can always be reduced to something that is at least 08 (0 rad)

Fig. 12-1. The unit circle is the basis for the trigonometric functions.

CHAPTER 12 A Taste of Trigonometry 277

but less than 3608 (2p rad). If you look at Fig. 12-1, you should be able to

envision how this works. Even if the ray OP makes more than one complete

revolution counterclockwise from the x axis, or if it turns clockwise instead,

its direction can always be deﬁned by some counterclockwise angle of least 08

(0 rad) but less than 3608 (2p rad) relative to the x axis.

Any oﬀbeat direction angle such as 7308 or 9p/4 rad can be reduced

to a direction angle that measures at least 08 (0 rad) but less than 3608

(2p rad) by adding or subtracting some whole-number multiple of 3608

(2p rad). But you have to be careful about this. A direction angle speciﬁes

orientation only. The orientation of the ray OP is the same for an angle of

5408 (3p rad) as for an angle of 1808 (p rad), but the larger value carries

with it the insinuation that the ray (also called a vector) OP has revolved

1.5 times around, while the smaller angle implies that it has undergone

less than one complete revolution. Sometimes this doesn’t matter, but often

it does!

Negative angles are encountered in trigonometry, especially in graphs

of functions. Multiple revolutions of objects are important in physics

and engineering. So if you ever hear or read about an angle such as 908, p/

2 rad, 9008,or5p rad, you can be conﬁdent that it has meaning. The negative

value indicates clockwise rotation. An angle that is said to measure more

than 3608 (2p rad) indicates more than one complete revolution counter-

clockwise. An angle that is said to measure less than 3608 (2p rad)

indicates more than one revolution clockwise.

THE SINE FUNCTION

In Fig. 12-1, imagine ray OP pointing along the x axis, and then starting

to rotate counterclockwise on its end point O, as if point O is a mechanical

bearing. The point P, represented by coordinates (x

, y

), thus revolves

around point O, following the perimeter of the unit circle.

Imagine what happens to the value of y

(the ordinate of point P) during

one complete revolution of ray OP. The ordinate of P starts out at y

¼ 0,

then increases until it reaches y

¼ 1 after P has gone 908 or p/2 rad around

the circle ( ¼ 908 ¼ p/2). After that, y

begins to decrease, getting back

to y

¼ 0 when P has gone 1808 or p rad around the circle ( ¼ 1808 ¼ p).

As P continues on its counterclockwise trek, y

keeps decreasing until,

at  ¼ 2708 ¼ 3p/2, the value of y

reaches its minimum of 1. After that, the

value of y

rises again until, when P has gone completely around the circle,

it returns to y

¼ 0 for  ¼ 3608 ¼ 2p.

PART 3 Shapes and Places

278

The value of y

is deﬁned as the sine of the angle . The sine function is

abbreviated sin, so we can state this simple equation:

sin  ¼ y

CIRCULAR MOTION

Imagine that you attach a ‘‘glow-in-the-dark’’ ball to the end of a string, and

then swing the ball around and around at a rate of one revolution per second.

The ball describes a circle as viewed from high above, as shown in Fig. 12-2A.

Suppose you make the ball circle your head so the path of the ball lies in a

perfectly horizontal plane. Imagine that you are in the middle of a ﬂat,

open ﬁeld on a perfectly dark night. If a friend stands far away with his or

her eyes exactly in the plane of the ball’s orbit and looks at you and the

ball through a pair of binoculars, what does your friend see?

Your friend, watching from a great distance and from a viewpoint exactly

in the plane deﬁned by the ball’s orbit, sees a point of light that oscillates

back and forth, from right-to-left and left-to-right, along what appears to be

a straight-line path (Fig. 12-2B). Starting from its extreme right-most

apparent position, the glowing point moves toward the left for half a second,

speeding up and then slowing down; then it stops and reverses direction;

then it moves toward the right for half a second, speeding up and then

slowing down; then it stops and turns around again. This goes on and on for

as long as you care to swing the ball around your head. As seen by your

friend, the ball reaches its extreme right-most position at one-second intervals

because its orbital speed is one revolution per second.

Fig. 12-2. Orbiting ball and string. At A, as seen from above; at B, as seen edge-on.

CHAPTER 12 A Taste of Trigonometry 279

THE SINE WAVE

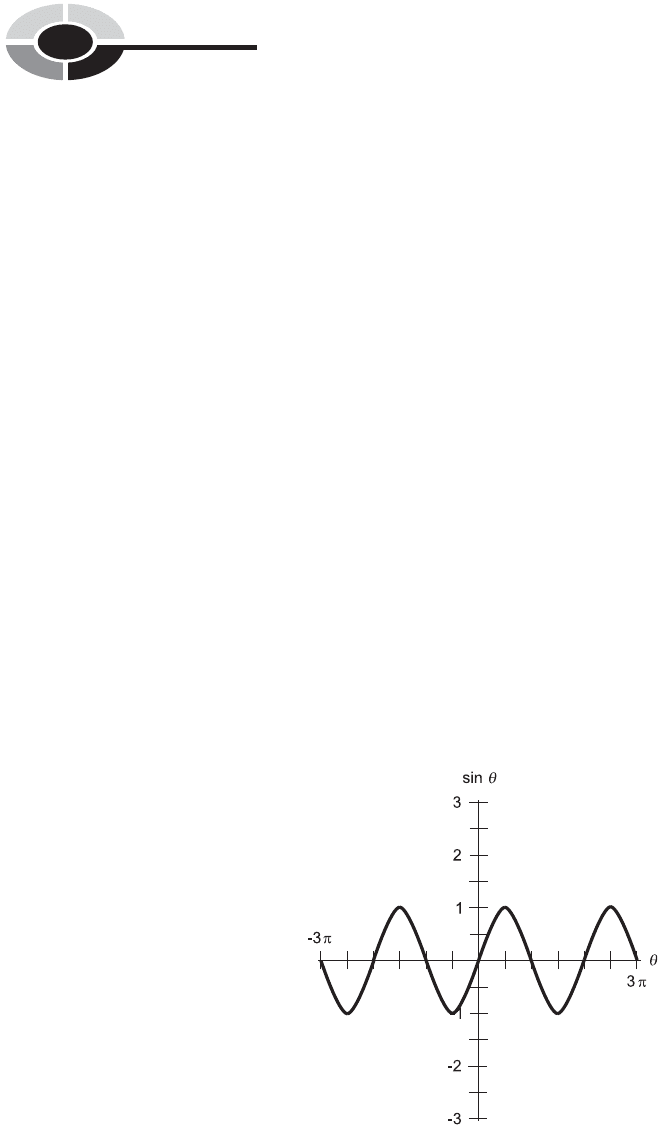
If you graph the apparent position of the ball as seen by your friend with

respect to time, the result is a sine wave, which is a graphical plot of a sine

function. Some sine waves ‘‘rise higher and lower’’ (corresponding to a longer

string), some are ‘‘ﬂatter’’ (a shorter string), some are ‘‘stretched out’’

(a slower rate of revolution), and some are ‘‘squashed’’ (a faster rate of

revolution). But the characteristic shape of the wave, known as a sinusoid,

is the same in every case.

You can whirl the ball around faster or slower than one revolution per

second. The string can be made longer or shorter. These adjustments alter

the frequency and/or the amplitude of the sine wave. But any sinusoid can be

deﬁned as the path of a point that orbits a central point in a perfect circle,

with a certain radius, at a certain rate of revolution.

Circular motion in the Cartesian plane can be deﬁned in terms of a general

formula:

y ¼ a sin b

where a is a constant that depends on the radius of the circle, and b is a

constant that depends on the revolution rate. The angle  is expressed in a

counterclockwise direction from the positive x axis. Figure 12-3 illustrates

a graph of the basic sine function: a sine wave for which a ¼ 1 and b ¼ 1,

and for which the angle is expressed in radians.

Fig. 12-3. Graph of the sine function for values of  between 3p rad and 3p rad.

PART 3 Shapes and Places

280