Gibilisco S. Everyday Math Demystified: A Self-Teaching Guide

Подождите немного. Документ загружается.

The fourth equation above is in factored form. This is a convenient form,

because a quadratic equation denoted this way can be solved without having

to do any work. Look at it closely:

ðx þ 4Þðx  5Þ¼0

The expression on the left-hand side of the equals sign is zero if either of the

two factors is zero. If x ¼4, then here is what happens when we ‘‘plug it in’’:

ð4 þ 4Þð4  5Þ¼0

0 9 ¼ 0 ðIt works!Þ

If x ¼ 5, then here’s what occurs:

ð5 þ 4Þð5  5Þ¼0

9  0 ¼ 0 ðIt works again! Þ

It is obvious which values for the variable in a factored quadratic will work as

solutions. Simply take the additive inverses (negatives) of the constants in

each factor. It’s so easy, in fact, that you must think there’s a catch. There

is, of course. Most quadratic equations are diﬃcult to get neatly into factored

form.

A COUPLE OF NITS

Here are a couple of little things that should be cleared up right away, so you

never get confused about them.

Suppose you run across a quadratic like this:

xðx þ 3Þ¼0

You might want to imagine this equation written out like this:

ðx þ 0Þðx þ 3Þ¼0

This makes it easy to see that the solutions are x ¼ 0orx ¼3. Of course,

adding 0 to a variable doesn’t change anything except to make an equation

longer. But it can clarify the process of solving some equations.

In case you forgot, at the beginning of this section it was mentioned that a

quadratic equation might have only one real-number solution. Here is an

example of the factored form of such an equation:

ðx  11Þðx  11Þ¼0

In standard form, the equation looks like this:

 22x þ 121 ¼ 0

CHAPTER 6 More Algebra 121

Some purists might say, ‘‘This equation has two real-number solutions, and

they are both equal to 11.’’

PROBLEM 6-1

Convert the following quadratic equations into factored form with

real-number coeﬃcients:

 2x  15 ¼ 0

þ 4 ¼ 0

SOLUTION 6-1

The ﬁrst equation turns out to have a ‘‘clean’’ factored equivalent:

ðx þ 3Þðx  5Þ¼0

The second equation does not have any real-numbered solutions, so the

problem, as stated, can’t be solved. Getting the second equation into factored

form requires that you know something about complex numbers, and we’ll

get into that subject in a moment. You can tell that something is peculiar

about this equation if you subtract 4 from each side:

þ 4 ¼ 0

¼4

No real number can be substituted for x in this equation in order to make it a

true statement. That is because no real number, when squared, produces

a negative real-number result.

PROBLEM 6-2

Put the following factored equations into standard quadratic form:

ðx þ 5Þðx  1Þ¼0

xðx þ 4Þ¼0

SOLUTION 6-2

Both of these can be converted to standard form by multiplying the factors.

In the ﬁrst case, it can be done in two steps:

ðx þ 5Þðx  1Þ¼0

 x þ 5x þ½5 ð1Þ ¼ 0

þ 4x  5 ¼ 0

PART 2 Finding Unknowns

122

In the second case, it can be done in a single step:

xðx þ 4Þ¼0

þ 4x ¼ 0

THE QUADRATIC FORMULA

Examine these two quadratic equations:

3x

 4x ¼ 2

¼3x þ 5

These can be reduced to standard form:

3x

 4x  2 ¼ 0

þ 3x  5 ¼ 0

You might stare at these equations for a long time before you get any ideas

about how to factor them. Eventually, you might wonder why you are wast-

ing your time. These equations do not ‘‘want’’ to be factored. Fortunately,

there is a formula you can use to solve quadratic equations in general.

Consider the general quadratic equation:

þ bx þ c ¼ 0

where a 6¼ 0. The solution(s) to this equation can be found using this formula:

x ¼½b ðb

 4acÞ

1=2

=2a

The symbol  is read ‘‘plus-or-minus’’ and is a way of compacting two

mathematical expressions into one. Written separately, the equations are:

x ¼½b þðb

 4acÞ

1=2

=2a

x ¼½b ðb

 4acÞ

1=2

=2a

The fractional exponent means the 1/2 power, another way of expressing the

square root.

Plugging in

Examine this equation once again:

3x

 4x  2 ¼ 0

CHAPTER 6 More Algebra 123

The coeﬃcients are as follows:

a ¼3

b ¼4

c ¼2

Plugging these numbers into the quadratic formula produces solutions, as

follows:

x ¼f4  ½ð4Þ

ð4 3 2Þ

1=2

g=ð2 3Þ

¼ 4 ð16  24Þ

1=2

=ð6Þ

¼ 4 ð8Þ

1=2

=ð6Þ

We are confronted with the square root of 8 in the solution. What is this?

It isn’t a real number, but how can we ignore it? We’ll look into this

matter shortly. For now, let’s work out a couple of problems with quadratics

involving only the real numbers.

THE PARABOLA

When we substitute y for 0 in the standard form of a quadratic equation,

and then graph the resulting relation with x on the horizontal axis and y

on the vertical axis, we get a curve called a parabola.

Suppose we want to plot a graph of the following equation:

þ bx þ c ¼ y

First, we determine the coordinates of the following point (x

, y

¼b=ð2aÞ

¼ c  b

=ð4aÞ

This point represents the base point of the parabola; that is, the point at

which the curvature is sharpest. Once this point is known, we must ﬁnd

four more points by ‘‘plugging in’’ values of x somewhat greater than and

less than x

, and then determining the corresponding y-values. These

x-values, call them x

2

, x

1

, x

, and x

, should be equally spaced on either

side of x

, such that:

2

< x

1

< x

1

 x

2

¼ x

 x

1

¼ x

 x

¼ x

 x

PART 2 Finding Unknowns

124

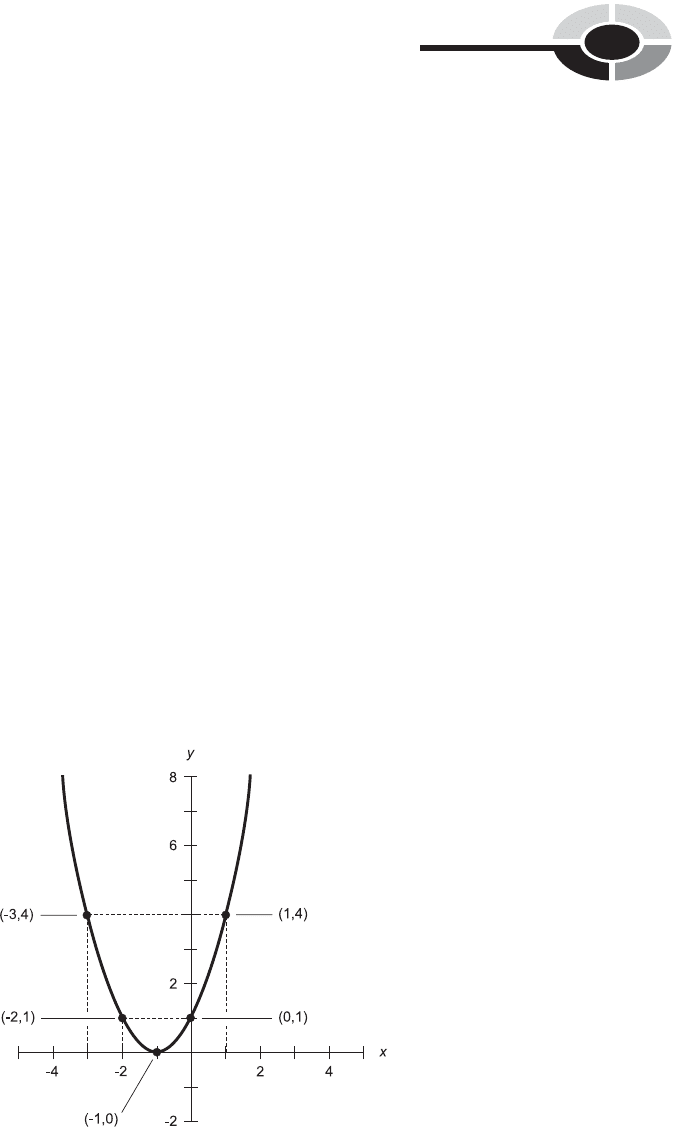
This gives us ﬁve points that lie along the parabola, and that are symmetri-

cal relative to the axis of the curve. The graph can then be inferred if the

points are wisely chosen. Some trial and error might be required. If a>0,

the parabola opens upward. If a<0, the parabola opens downward.

Plotting a parabola

Consider the following formula:

y ¼ x

þ 2x þ 1

Using the above formula to calculate the base point, we get this:

¼2=2 ¼1

¼ 1  4=4 ¼ 1  1 ¼ 0

Therefore ðx

ó y

Þ¼ð1ó 0Þ

We plot this point ﬁrst, as shown in Fig. 6-1. Next, we plot points spaced at

1-unit horizontal-axis intervals on either side of x

, as follows:

2

¼ x

 2 ¼3

2

¼ð3Þ

þ 2 ð3Þþ1 ¼ 9  6 þ 1 ¼ 4

Therefore ðx

2

ó y

2

Þ¼ð3ó 4Þ

Fig. 6-1. Graph of the quadratic equation y ¼ x

þ 2x þ 1.

CHAPTER 6 More Algebra 125

1

¼ x

 1 ¼2

1

¼ð2Þ

þ 2 ð2Þþ1 ¼ 4  4 þ 1 ¼ 1

Therefore ðx

1

ó y

1

Þ¼ð2ó 1Þ

¼ x

þ 1 ¼ 0

¼ 0

þ 2  0 þ 1 ¼ 0 þ 0 þ 1 ¼ 1

Therefore ðx

ó y

Þ¼ð0ó 1Þ

¼ x

þ 2 ¼ 1

¼ 1

þ 2  1 þ 1 ¼ 1 þ 2 þ 1 ¼ 4

Therefore ðx

ó y

Þ¼ð1ó 4Þ

From these ﬁve points, the parabola can be inferred. It is shown as a solid,

heavy curve in Fig. 6-1.

Plotting another parabola

Let’s try another example, this time with a parabola that opens downward.

Consider the following formula:

y ¼2x

þ 4x  5

The base point is:

¼4= 4 ¼ 1

¼5  16=ð8Þ¼5 þ 2 ¼3

Therefore ðx

ó y

Þ¼ð1ó  3Þ

This point is plotted ﬁrst, as shown in Fig. 6-2. Next, we plot the following

points:

2

¼ x

 2 ¼1

2

¼2 ð1Þ

þ 4 ð1Þ5 ¼2  4  5 ¼11

Therefore ðx

2

ó y

2

Þ¼ð1ó  11Þ

PART 2 Finding Unknowns

126

1

¼ x

 1 ¼ 0

1

¼2  0

þ 4  0  5 ¼5

Therefore ðx

1

ó y

1

Þ¼ð0ó  5Þ

¼ x

þ 1 ¼ 2

¼2  2

þ 4  2  5 ¼8 þ 8  5 ¼5

Therefore ðx

ó y

Þ¼ð2ó  5Þ

¼ x

þ 2 ¼ 3

¼2  3

þ 4  3  5 ¼18 þ 12  5 ¼11

Therefore ðx

ó y

Þ¼ð3ó  11Þ

From these ﬁve points, the parabola is inferred. It is the solid, heavy curve

in Fig. 6-2.

PROBLEM 6-3

Find the real-number solution(s) to the following equation by factoring:

þ 14x þ 49 ¼ 0

Fig. 6-2. Graph of the quadratic equation y ¼2x

þ 4x  5.

CHAPTER 6 More Algebra 127

SOLUTION 6-3

Assuming this equation can be put into factored form, let’s suppose the

equation looks like this:

ðx þ tÞðx þ uÞ¼0

In this case, the solutions are x ¼t and x ¼u. The trick is ﬁnding t

and u such that we get the original equation when the factors are

multiplied together. The above generalized equation looks like this when

multiplied out:

ðx þ tÞðx þ uÞ¼0

þ ux þ tx þ tu ¼ 0

þðu þ tÞx þ tu ¼ 0

Are there any numbers t and u whose sum equals 14 and their product equals

49? It should not take you long to see that the answer is yes; this works out if

t ¼ 7 and u ¼ 7. If we substitute these values into the generalized, factored

quadratic and then multiply it out, we get the original equation that we

want to solve:

ðx þ 7Þðx þ 7Þ¼0

þ 7x þ 7x þ 49 ¼ 0

þ 14x þ 49 ¼ 0

Therefore, there is a single real-number solution to this equation: x ¼7.

PROBLEM 6-4

Use the quadratic formula to solve the equation from the previous problem.

SOLUTION 6-4

Here is the equation in standard form again:

þ 14x þ 49 ¼ 0

The coeﬃcients from the general form, and which are deﬁned in the quadratic

formula above, are as follows:

a ¼ 1

b ¼ 14

c ¼ 49

PART 2 Finding Unknowns

128

Here is what happens when we plug these coeﬃcients into the quadratic

formula:

x ¼f14 ½ð14Þ

ð4  1  49Þ

1=2

g=ð2  1Þ

¼14 ð196  196Þ

1=2



¼14  0

1=2



¼14=2

¼7

Beyond Reality

Mathematicians symbolize the positive square root of 1, called the unit

imaginary number, by using the lowercase italic letter i. Scientists and engi-

neers symbolize it using the letter j, and henceforth, we will too.

IMAGINARY NUMBERS

Any imaginary number can be obtained by multiplying j by some real

number q. The real number q is customarily written after j if q is positive or

zero. If q happens to be a negative real number, then the absolute value of q is

written after j. Examples of imaginary numbers are j3, j5, j2.787, and jp.

The set of imaginary numbers can be depicted along a number line, just as

can the real numbers. The so-called imaginary number line is usually shown

standing on end, that is, vertically (Fig. 6-3). In a sense, the real-number

line and the imaginary-number line are fraternal twins. As is the case with

human twins, these two number lines, although they share similarities, are

independent. The sets of imaginary and real numbers have one value, 0, in

common. Thus:

j0 ¼ 0

COMPLEX NUMBERS

A complex number consists of the sum of some real number and some

imaginary number. The general form for a complex number k is:

k ¼ p þ jq

where p and q are real numbers.

CHAPTER 6 More Algebra 129

Mathematicians, scientists, and engineers denote the set of complex

numbers by placing the real-number and imaginary-number lines at right

angles to each other, intersecting at the points on both lines corresponding

to 0. The result is a rectangular coordinate plane (Fig. 6-4). Every point on

this plane corresponds to a unique complex number, and every complex

number corresponds to a unique point on the plane.

Now that you know a little about complex numbers, you might want to

examine the solution to the following equation again:

3x

 4x  2 ¼ 0

Recall that the solution, derived using the quadratic formula, contains

the quantity (8)

1/2

. An engineer or physicist would write this as j8

1/2

,so

the solution to the quadratic is:

x ¼ð4  j8

1=2

Þ=ð6Þ

This can be simpliﬁed to the standard form of a complex number, and then

reduced to the lowest fractional form. Step-by-step, the simpliﬁcation process

Fig. 6-3. The imaginary number line is just like the real number line, except that it is ‘‘stood

on end’’ and all the quantities are multiplied by j.

PART 2 Finding Unknowns

130