Ferrarese G. (editor) Wave Propagation
Подождите немного. Документ загружается.

44
adjacent
to a
region
of
constant
state
must be a
generalised
simple
wave
region.
This
result
which
might
have
been
conjectured
from Theorem 1
will
then
complement
the
results
of
that
theorem.
First
we
notice
that
from Theorem 1
it
follows
that
if
a
region
A
of
constant
state
exists
in
the
(x,t)-plane,
then
it
will
be bounded by a
characteristic,
say
by a
member
C
of
the
C(k)-family.
Any
region
adjacent
to
it
will
also
be
bounded by
this
same
line
C.
Now
pre-multiplication
of
system
(5)
by
the
left
eigenvector
~(j)
of
A
gives
the
system
along
the
C(j)
family
,
(16)
for
j =
1,2,
.
•.
,n.
As
the
left
and
right
eigenvectors
of
A
are
biorthogonal~
so
that
o
for
j ; k
it
follows
directly
fr~
(15)
that
the
vector
~(j)
must be
expressible
as
a
linear
combination
of
the
vectors
(VuJ
i
(k».
Accordingly,
we
set
n-l
L
sal
b
j
(V J
(k»for
j ; k •
sus
(17)
Equations
(16)
then
become
n-l
b (V J (k» dU
L
-
0
for
j
;
k ,
s"l
js
u s
dj
which by
the
chain
rule
reduces
to
n-l
dJ
(k)
L
b
j S
dj s
-
0
for
j
;
k •
sal
(18)
(19)
This
is
now a
linear
hyperbolic
system
involving
(n-l)
equations
for
the
(k)
(k) (k) (k)
(n-l)
generalised
A -Riemann
invariants
J
l
' J
2
'
•••
, I
n_
l
For any
specified
solution
vector
U
the
coefficients
b
j s
will
be known. The
condi
tion
j ; k
ensures
that
the
line
C
common
to
both
the
region
of
constant
state
and
the
generalised
simple
wave
region
will
not
be a
characteristic
of
the
new
syst
em.
Consequently
there
exists
a
unique
smooth
solution
that
can
be
continued
across
the
line
C.
Since
the
solutio
n on one
side
of
C was
the
45
Hence
constant
state
solution,
the
solution
that
is
continued
across
it
will
be
one
for
which
all
the
generalised
~(k)-Riemann
invariants
are
constant
.
from
the
nature
of
generalised
Riemann
invariants
it
may be
seen
that
the
solution
adjacent
to a
region
of
constant
state
must be a
generalised
simple
wave
region.
This
result
also
merits
a
formal
statement.
Theorem 2
(Constant
State
and
Generalised
Simple Wave)
Let
the
system
U
+AU
- 0
t x
with
U an n x 1 column
vector
and A an n x n
matrix
be
reducible
in
the
generalised
sense.
Then
if
A
is
a
region
of
constant
state
in
the
(x,t)-plane,
the
region
S
adjacent
to
it
is
a
generalised
simple
wave
region.
,
•••
t
n-l
(k)
ilJ
(k)
I
_il~
m_
m"'l ilJ
(k)
ilu
2
m
n-l
il~
(k)
l-
_1
aJ
(k)
m
Let
us now examine
further
the
implications
of
equation
(15).
Consider
the
special
case
in
which
the
eigenvalue
~(k)
is
expressible
as
a
function
of
J
l
(k),
~2
(k)
,
•••
, I
n
_
l
(k)
Then we
have
(k)
{
n- l
~,(k)
ilJ (k)
(
.V),
) '" I
_01\__
m
u m-l ar
(k)
~
m
aJm(k) )
au
n
or,
equivalently,
n-l
l
111"'1
ax
(k)
ilJ
(k)
m
V J
(k)
um
(20)
showing
that
(V
~(k»
is
a
linear
combination
of
the
gradients
of
the
u
generalised
~(k)-Riemann
invariants.
After
post-multiplication
of
(20)
by
r(k)
it
then
follows
directly
from (15)
that
(21)
In
general,
when a
quasilinear
hyperbolic
system
exists
for
which
property
(k)
(21)
is
true
with
respect
to
the
k-th
characteristic
field
C
associated
with),
=
~(k),
the
system
will
be
said
to
be
exceptional
with
respect
to
the
k-th
characteristic
field.
This
will
be
true
irrespective
of
whether
or
not
the
46
system
permits
generalised
simple
wave
solutions.
When
(21)
is
not
true,
the
system
of
equations
will
be
said
to be
genuinely
nonlinear
with
respect
to
the
k-th
characteristic
field
C(k).
Expressed
differently,
condition
(21)
asserts
that
when a
system
is
exceptional
with
respect
to
the
k-th
characteristic
field,
the
directional
derivative
of
A(k)
in
the
direction
of
the
eigenvector
r(k)
is
zero.
We
now
formulate
these
ideas
generally,
without
reference
to
generalised
simple
waves
or
to Riemann
invariants.
Definition
(Exceptional
Condition
and Genuine
Nonlinearity)
Consider
the
quasilinear
hyperbolic
system
where U
is
an n x 1 column
vector,
A ·
A(U,x,t)
is
an n x n
matrix
and
B
=
B(U,x,t)
is
an n x 1 column
vector.
Then
the
system
will
be
said
to
be:
(a)
exceptional
with
respect
to
the
k-th
characteristic
field
if
(b)
compl e t el y
exceptional
if
it
is
exceptional
with
respect
to
each
of
the
n
characteristic
fields
corresponding
to
h(l),
A(2),
.••
,
A(n);
(c)
genuinely
nonlinear
with
respect
to
the
k-th
characteristic
field
if
References
[1]
Jeffrey,
A.
Quasilinear
Hyperbolic
Systems and Waves,
Research
Note i n
Mathematics
5, Pitman
Publishing,
London, 1976.
[2J Coulson, C.
A.,
Jeffrey,
A. Waves, 2nd
Ed.,
Longman, 1977.
[3]
Friedrichs,
K. O.
Nichtlineare
Differenzialgleichungen.
Notes
of
lectures
delivered
at
GBttingen,
1955.
[4]
Lax, P. D.
Hyperbolic
Systems
of
Conservation
Laws
II,
Comm.
Pure
App1. Math. 10
(1957),
537-566.

47
Lecture
4.
The Development
of
Jump
Discontinuities
in
Nonlinear
Hyperbolic
Systems
of
Equations
1.
General
Considerations
We
shall
consider
initial
value
problems
leading
to
the
propagation
of
a
wavefront
in
quasi-linear
systems
of
equations
of
the
form
(1)
where U
is
a column
vector
with
the
n components u
l•
u
2
,
..•
,
un'
A
is
an
n
x n
matrix
and B
is
an n
element
column
vector;
A and B
are
assumed
to
depend on x, t and U. The
system
(1)
will
be
considered
to be
hyperbolic
and so
all
the
eigenvalues
of
A
are
real
and A
possesses
a
full
set
of
linearly
independent
eigenvectors.
The
left
eigenvectors
of
A,
1(i,k)
with
k •
1,2
•••.
,s
corresponding
to
the
eigenvalue
A(i)
with
multiplicity
s
satisfy
the
equations
1(i.k)
A •
A(i)1{i,k)
•
k •
1.2,
••••
8 •
(2)
They may be
used
to
display
the
equations
(1)
in
characteristic
form and
to
introduce
the
n
characteristic
curves
e(i)
as
follows.
Pre-multiply
equation
(1)
by
1(i)
and,
assuming
for
the
moment
that
the
n
eigenvalues
of
A
are
distinct,
we
obtain
n
equations
written
in
characteristic
form
which,
by
virtue
of
(2).
become
operator
a~
+
A(i)
a:
in
the
ith
equation
represents
ith
characteristic
curve
e(i)
determined
by
differentiation
along
the
1
(i)
(U + A
(i)
t
where
b(i)
•
1(i)B.
U ) +
b(i)
x
The
o
(i
1,2,
•••
,n)
(3)
C
(i ) • dx •
,(i)
•
dt
1\ •
We
shall
be
concerned
later
with
the
propagation
of
a
disturbance
or
wave
(4)
into
a
state
which
is
either
known (and
non-constant)
or
is
constant.
when
the
line
bordering
these
two
states,
the
wavefront,
is
determined
by a
relation
of
the
form
Hx.t)
•
O.
(5)
The
wavefront
~
• 0
is
assumed
here
to
be a
line
acrosa
which
the
solution

48
U
is
continuous
but
across
which
the
normal
derivative
of
U
is
discontinuous.
The
class
of
solutions
U
considered
is
thus
Lipschitz
continuous
with
exponent
unity.
2. The
Initial
Value
Problem
Consider
the
system
U +AU
+B
t x
°
(6)
subject
to
the
initial
condition
U(x,O)
t(x)
,
< x < CD
(7)
where
t(x)
is
Lipschitz
continuous.
Using
Haar's
a
priori
estimate
and a
special
iteration
scheme
it
may
be shown
that
while
the
solution
on
the
wavefront
remains
Lipschitz
continuous
the
solution
of
(6)
and
(7)
on
the
wavefront
depends
boundedly
on t he
initial
values
and on
the
inhomogeneous
term.
Accordingly,
when
the
advancing
wave-
front
ceases
to be
Lipschitz
continuous
U
will
attain
a bounded
value,
say
u
c
'
behind
the
wavefront
while
ahead
of
the
wavefront
U
will
have
a
value
appropriate
to
the
state
into
which
the
wave
is
advancing.
Thus
at
some
critical
time
t
c
and
at
some
critical
distance
x
o
the
solu
tion
ceases
to
he
Lipschitz
continuous
on
the
wavefront
and a
finite
jump
or
shock
like
discontinuity
appears
in
U
with
magnitude
U
c
- U.
We
shall
now
obtain
exact
analytical
expressions
determining
the
initial
time
t
c
and
the
critical
distance
xc.
3. Time and
Place
of
Breakdown
of
Solution
We
start
with
a
general
quasi-linear
hyperbolic
system
°
(8)
and assume
that
the
vector
B and
the
eigenvalues
and
eigenvectors
of
A
are
continuously
differentiable
with
respect
to
their
arguments.
We
also
suppose
that
A and B do
not
depend
explicitly
on x and t and so
there
exists
a
constant
solution
U
o
satisfying
the
equstion
°.
(9)
lhia
cons
taut
state
~ill
be
denoted
by
the
subscript
0 and we
shall
consider
49
a wave
advancing
into
this
constant
state
. The
solution
is
Lipschitz
continuous
normal
to
the
wavefront
and
initial
conditions
may be
prescribed
such
that
at
t =
0,
U = U
o
for
x > 0 and
such
that
U
is
continuous
at
x = O. Denote by t
c
and Xc
the
critical
time
and
critical
distance,
respectively,
at
which
the
solution
ceases
to
be
Lipschitz
continuous
on
the
wavefront.
Let
us now assume
that
there
exists
at
least
one
positive
eigenvalue
of
A so
that
the
wave
proceeds
in
the
direction
of
the
positive
x-axis.
We
identify
the
velocity
of
the
wavefront
with
one
of
the
positive
eigenvalues,
say
~~~),
and
introduce
the
curvilinear
coordinates
constant
,
through
the
equations
t'
constant
and .
t'
t
(lOa)
Ftom
equation
(lOb) we
see
that
but
along
~
=
constant
we may
write
(lOb)
(11)
o
(12)
and so from
(11)
and
(12)
we
see
that
the
~
=
constant
lines
are
characteristics
and so
dx
dt
•
~(~)
along
~
=
constant.
Thus,
since
equation
(13)
is
only
valid
along
~
=
constant
and from (lOa) we
have
t'
•
t,
equation
(13)
is
identical
with
ax
at'
which
is
a
result
that
will
be
required
later.
As
~
is
a
solution
of
(lOb) we must
specify
it
by
giving
initial
(13'
)
conditions
. These
should
reflect
the
fact
that
it
is
a
coordinate
variable
50
and so
should
be
assigned
monotonically.
We
choose
~(x,t)
by
imposing
the
initial
condition
Hx,O)
x
when
the
wavefront
is
given
by
Hx,t)
0
and,
in
the
region
of
constant
state
ahead
of
the
wavefront,
Hx,t)
> 0 •
The
transformation
introduced
through
equations
(10)
is
non-singular
provided
the
Jacobian
1
•
~x
is
non-zero
and
finite.
(14)
The
initial
condition
on
~
ensures
that
x~
is
initially
equal
to
unity
and
so we may
assume
the
non-vanishing
of
the
Jacobian
for
at
least
a
finite
time
after
t = O.
Let
us
denote
by L
the
open
region
lying
to
the
left
of
the
advancing
wavefront
(x,t)
• 0 and
bounded
on
the
left
by
the
characteristic
(x,t)
= 0
also
issuing
out
of
the
origin
and
chosen
so
that
no
other
characteristics
enter
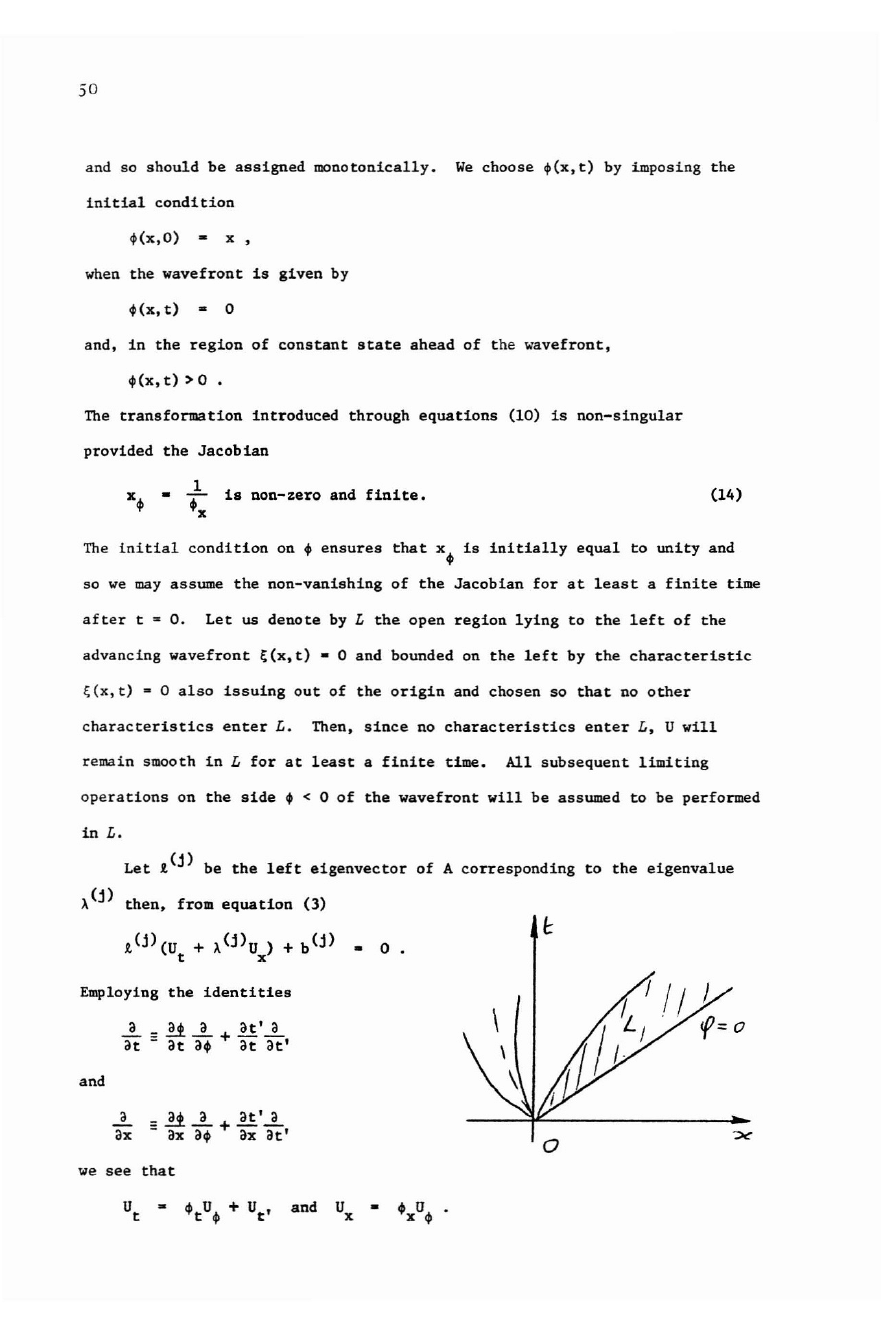
L. Then,
since
no
characteristics
enter
L, U
will
remain
smooth
in
L
for
at
least
a
finite
time.
All
subsequent
limiting
operations
on
the
side
~
< 0
of
the
wavefront
will
be
assumed
to
be
performed
in
L.
Let
l(j)
be
the
left
eigenvector
of
A
corresponding
to
the
eigenvalue
A(j)
then,
from
equation
(3)
Employing
the
identities
...!.
=
!t
...!..
+ l!'...!..
at
-
at
a~
at
at'
and
...!.
=!t...!.. +
l!
•
...!..
ax - ax
a~
ax
at'
we
see
that
o .
o

$1
Thus,
using
these
results
and
equation
(lOb)
together
with
condition
(14) to
ensure
the
non-vanishing
of
the
Jacobian,
we
obtain
R.
(j)
(x
...!.
+
(>.
(j)
-
>.
w)
...!.)
U + b
o(j)
41
at'
a41
x
41
In
particular,
if
>.(j) -
>.(41),
we
have
o .
(15)
,(4I,k)
+ b(4I,k)
..
Ut'
o ,
k -
1,2,
•••
,r
41
(16)
where r
41
is
the
multiplicity
of
the
eigenvalue
>.(41).
By
virtue
of
our
choice
of
coordinates
the
wavefront
41
- 0
is
a
characteristic,
and
since
the
solution
is
Lipschitz
con~nuous
across
the
wavefront,
jump
discontinuities
in
derivatives
with
respect
to
41
may
take
place
across
41
- O.
Accordingly
we
define
the
jumps
across
41
- 0 as
follows:
U
is
continuous
:
Ut'
is
continuous
[U ]
41-0-
- 0
or
41-0+
41=0-
[U
t']4I-O+
- 0
U(O,t')
- U
o
(constant)
and
U
4I
is
discontinuous
X
41
is
discontinuous
We
note
that
since
both
IT
and X depend on
41
they
are
not
independent
and we
shall
later
determine
their
precise
relationship
[see
equation
(21)].
From
the
definition
of
X we
see
that
X + (x
4l)4I=0+
- (x4')4I_0_,whi1e
(x4')4I-O+ -
x 0
(say)
is
finite.
Hence,
in
a
neighbourhood
of
the
wavefront
41
condition
(14)
is
seen
to be
equivalent
to
the
condition
x + x 0
is
finite
and
non-zero.
(14')
41
The
significance
of
the
non-vanishing
of
the
Jacobian
may
easily
be
seen
by
noting
that
in
L and
along
41
- 0 we have
U
x
whence
So,
if
x
41
vanishes
while
U4'
remains
finite,
U
ceases
to
be
Lipschitz
continuous
and we
have
the
gradient
catastrophe.

52
In
the
simple
case
that
U
o
m
constant
the
jump
conditions
on n and X
reduce
to
and
n(t'
)
X(t')
•
(17)
So,
since
t(j)
is
assumed
to
be
continuous
across
the
wavefront
and
Ut'
is
continuous
across
the
wavefront
with
U
t
,
= 0
in
the
constant
region
we
have,
by
using
(9)
and by
considering
equation
(15)
at
a
point
P
in
Land
letting
the
point
tend
to
a
point
on
the
wavefront,
that
j
r.p+l'
•••
, n
(18)
where aga
in
the
subscript
0
signifies
the
constant
state
appropriate
to
(9).
We
now
differentiate
equation
(16)
with
respect
to
.p
at
point
P
in
L
to
obtain
or,
where T
denotes
the
.
transpose
operation
and
where V
u
is
the
gradient
operator
with
respect
to
(u
l'
u
2,
••••
uu)-space.
Again,
letting
P
tend
to
a
point
on
the
wavefront
and
using
the
fact
that
U
t,
is
continuous
across
the
wavefront
with
(Ut').p_O+=
0 we
obtain
the
equation
k m
1,2,
•• • ,r.p
(19)
If,
now, we
differentiate
equation
(13')
with
respect
to
.p
at
a
point
P
in
L
we
obtain
0
(~~,
)
(V A
(,»
U,
~
u
and
so,
0
(x,)
(V A(.p»U
(20)
at'
u ,

53
Thus,
again
letting
P
lend
to
a
point
on
the
wavefront,
and
using
(17) we
find
that
(21)
Since
the
i~j)
are
linearly
independent
vectors
we may
use
equations
(18)
to
express
(n-r~)
components
of
TI
in
terms
of
a
certain
r~
components
of
TI.
Introducing
these
expressions
into
equations
(19)
leads
to
r~
first
order
ordinary
differential
equations
with
constant
coefficients
for
the
r~
unknowns,
say
TIl'
TI
2,
.
•.
,
rrr~
.
Introducing
TI
thus
determined
into
equation
(21) and
integrating
the
result
we may
obtain
an
expression
for
X. However,
before
doing
this
it
is
necessary
to
define
certain
limiting
operations
that
will
be
necessary
in
the
integration
process.
~
If
Q
is
a
quantity
defjned
only
in
L we
define
the
operation
Q
to
be
the
limit
Q_
lim
(Q)~~o-
For
a jump
quantity
P
depending
on
the
state
On
t'
->()
adjacent
sides
of
~
= 0 we
define
the
operation
P
to
be
the
limit
P=
lim
P
t'
->()
taken
along
'
~
~
o.
Thus,
integrating
equation
(21)
with
respect
to
t'
between 0 and T and
noting
that
X
is
a jump
quantity
defined
across
~
.. 0 we may use
the
limiting
operation
X
just
defined
to
obtain
the
result
x ..
x+ IT (V
A(~)
TIdt' •
o u 0
(22)
This
equation
describes
the
variation
of
X
along
the
wavefront
~
.. 0
with
advancing
time
and
in
writing
equation
(22) we
have
tacitly
assumed
that
the
multiplicity
r~
of
A(~)
remains
unchanged. Should
this
assumption
not
be
true
and
at
t'
.. Tl(T
l
< T)
the
multiplicity
changes,
then
TI
must be
re-determined
for
the
interval
t'
> T
l•
We
remark
here
that
although
an
initial
condition
on U
x
may be
prescribed
arbitrarily
by
specifying
lim
(Ux)t-O'
this
limiting
x->()
operation
is
not
in
L and so
in
general
U
x
is
not
equal
to
this
limit
and
although
on
the
initial
line
may
not
be
prescribed
arbitrarily.
Equation
(22) may be
displayed
in
a
slightly
different
form from which
the
critical
time
t
c
may be
determined
as
follows.
By
definition
