Ferrarese G. (editor) Wave Propagation
Подождите немного. Документ загружается.

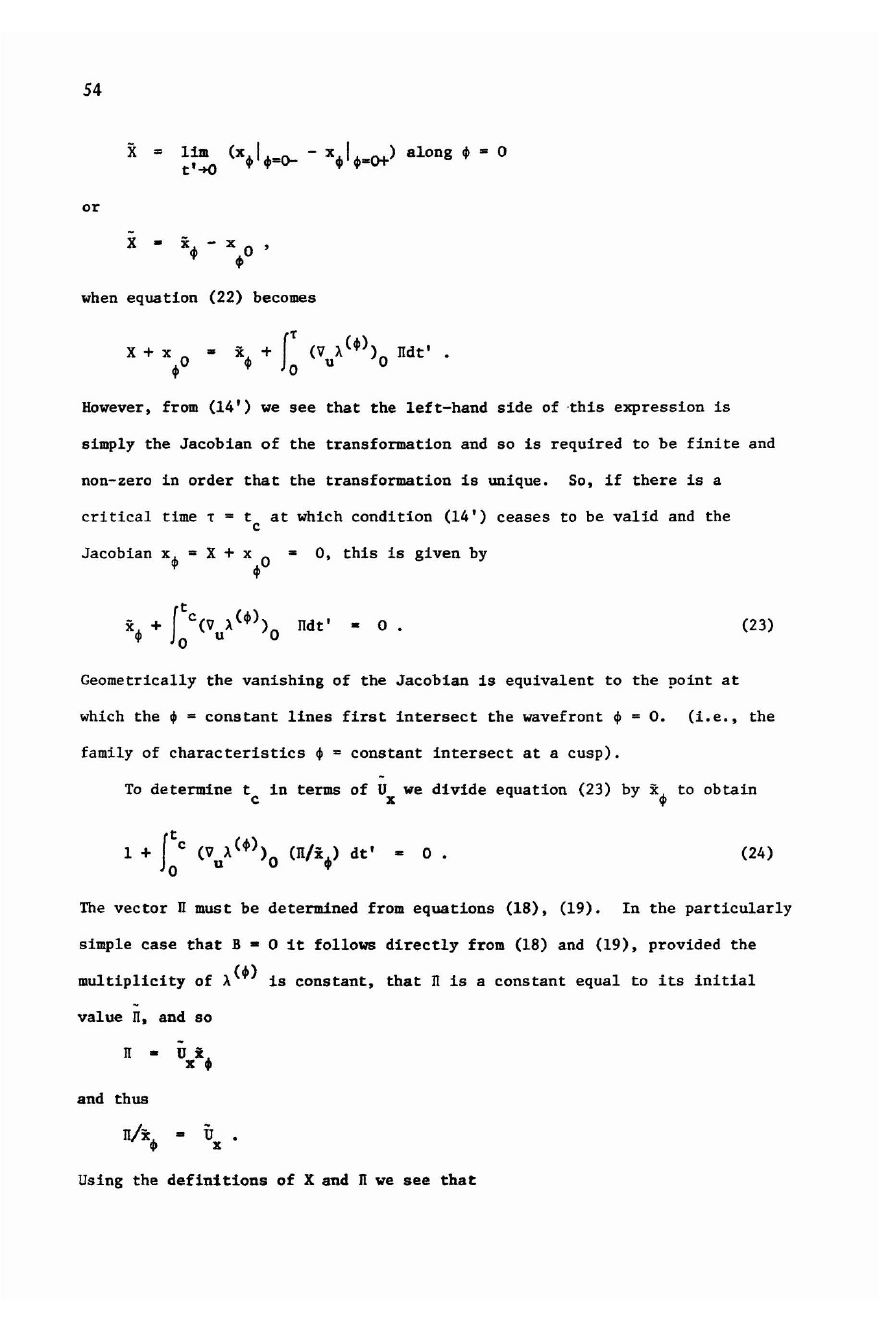
54
it =
or
when
equation
(22) becomes
However, from
(14')
we
see
that
the
left-hand
side
of
-t hi s
expression
is
simply
the
Jacobian
of
the
transformation
and so
is
required
to
be
finite
and
non-zero
in
order
that
the
transformation
is
unique.
So,
if
there
is
a
critical
time T = t
c
at
which
condition
(14')
ceases
to be
valid
and
the
Jacobian
x$ = X + x 0 -
0,
this
is
given
by
$
(23)
Geometrically
the
vanishing
of
the
Jacobian
is
equivalent
to
the
point
at
which
the
$ -
constant
lines
first
intersect
the
wavefront
$ = O.
(i.e
.,
the
fam
ily
of
characteristics
$ -
constant
intersect
at
a
cusp)
.
To
determine
t
c
in
terms
of
U
x
we
divide
equation
(23) by x$ to
obtain
o .
(24)
The
vector
n must be
determined
from
equations
(18),
(19).
In
the
particularly
simple
case
that
B • 0
it
follows
directly
from (18) and
(19),
provided
the
multiplicity
of
A($)
is
constant,
that
n
is
a
constant
equal
to
its
initial
.
value
n, and so
n •
and
thus
u
x
Using
the
definitions
of
X and n we
see
that

55
when
x
..
4
but
and so U
x
is
given
in
L by
the
expression
U UIII + (V
~(4»
Ut'}.
X X u 0 x
(25)
Thus U
x
becomes unbounded
if
the
denominator
vanishes
for
some t
c
> O.
If
(Vu~(4»o
=0
it
follows
directly
from
equation
(21)
that
X
is
a
constant
and so t
c
is
infinite.
The
discontinuity
in
this
case
is
propagated
but
remains
finite
for
all
time.
Systems
for
which
this
property
is
true
are
a
special
case
of
those
which
are
exceptional
with
respect
to
the
~(4)
characteristic
field.
The
general
case
when A, B depend on U and
also
explicitly
on
x,
t
has
been
discussed
in
detail
in
[1].
A
different
approach
to
the
problem
that
involves
three
space
dimensions
and time
has
been
described
by
Boillat
[2] and Chen
[3).
References
[1] A.
Jeffrey,
Quasilinear
Hyperbolic
Systems and Waves.
Research
Note
in
Mathematics
No
.5,
Pitman
Publishing,
London,
1976.
[2] G.
Boillat,
La
Propagation
des
Ondes.
Gauthier-Villars,
Paris,1965.
[3] P.
J.
Chen,
Selected
Topics
in
Wave
Prop~gation.
Noordhoff, Leyden, 1974.

50
Lecture
5.
The
Gradient
Catastrophe
and
the
Breaking
of
Water Waves
in
a Channel
of
Arbitrarily
Varying Depth and Width
1.
Basic
Equations
To
illustrate
the
gradient
catastrophe
in
a
physical
context,
let
us
show how
to
obtain
an
explicit
form
for
the
amplitude
of
an
acceleration
wave
that
propagates
into
water
at
rest
which
is
contained
in
a
vertical
walled
channel
with
slowly
varying
width
W(x) and an
arbitrarily
varying
depth
hex)
below
the
equilibrium
water
level.
The method we
describe
is
taken
from
the
joint
paper
submitted
for
publication
to
ZAMP
by
the
author
and
J.
Mvungi
[1].
As
usual,
let
the
x-axis
lie
in
the
equilibrium
surface
of
the
water
in
the
direction
of
propagation,
with
the
y-axis
pointing
vertically
upwards, and
write
the
equation
of
the
bottom
of
the
channel
as
y + hex) = o.
Then,
if
the
elevation
of
the
water
above
the
equilibrium
level
is
n(x,t),
g
is
the
acceleration
due to
gravity
and
the
x-component
of
the
water
velocity
is
u(x,t),
the
equation
of
motion
in
the
x-direction
is
as
derived
by
Stoker
[2],
namely
(1)
However,
the
equation
corresponding
to
the
conservation
of
mass
will
now
be
different
on
account
of
the
width
variation
of
the
channeL
To
derive
it,
all
that
is
necessary
is
to
observe
that
the
cross-sectional
area
S(x,t)
of
the
water
at
any
given
place
and
time
(x,t)
is
S(x,t)
=
W(x)(n(x,t)
+
hex»~,
and
that
the
flow
through
this
area
is
S(x,t)u(x,t).
Thus,
equating
the
time
rate
of
change
of
S
to
the
negative
flux
through
it,
we
find
-(Su)
,
x
from which
it
follows
that
(2)
o
(3)
The
governing
equations
for
flow
in
a
variable
width
channel
of
arbitrary
depth
are
thus
equations
(1)
and
(3).
The
assumption
of
a slow
variation
in
the
width
is
necessary
because
the
transverse
movement
of
the

57
water
has
been
neglected
in
these
one-dimensional
long
wave
equations,
. and
this
will
cease
to be a good
approximation
if
the
width
changes
too
rapidly.
2. The
Bernoulli
Equation
For
The
Acceleration
Wave Amplitude
Suppose
the
wave moves
in
the
direction
of
increasing
x,
starting
from x • 0
at
t • 0, and
that
it
moves
into
water
at
rest.
Then,
across
the
wavefront:
(i)
u and n
are
continuous,
with
u(x,t)
•
n(x,t)
• 0 ahead
of
the
advancing
wave,
(ii)
the
first
and
second
derivatives
of
u and n
suffer
at
most a
jump
discontinuity,
so
that
the
wavefront
being
propagated
on
the
surface
is
an
acceleration
wave.
Using a
superscript
minua
sign
to
denote
the
value
of
a
function
immediately
behind
the
advancing
wavefront
(i.e
.
at
the
edge
of
the
disturbp.d
region)
we
conclude
from
(i)
that
u
n
o
(4)
Taking
the
total
differential
of
equations
(4)
gives,
just
behind
the
wavefront,
-0
~+
u~dt
and 0
n-dx
+
n-dt
x t
or,
equivalently,
u
t
-cu
and
n
t
-c
n
xx
where c
•
dx/dt
is
the
speed
of
propagation
of
the
wavefront
which
is,
of
course,
a
characteristic
curve
for
the
system
(1),
(3) .
Immediately
behind
the
wavefront
(1) and (3) become
(5)
o and
n~
+
hu;
-
0,
(6)
where
it
is
understood
that
h •
hex)
is
the
depth
at
the
wavefront.
If
n;
; 0
equations
(5) and
(6)
imply
the
standard
result
c
2
• gh.
Now
define
the
amplitude
of
the
acceleration
wave
to
be
a
a(x)
• n
x
'
(7)

58
when (5) and
(6)
become
-
"e ,.
-ga
and u
x•
ga/c.
(8)
Now
notice
that
the
operation
of
differentiation
with
respect
to x
along
the
characteristic
followed
by
the
wavefront,
behind
which
u~
and
u~
are
defined,
takes
the
form
It
then
follows
immediately
from
this
that
(9)
2 -
• c U - U
xx
tt
(10)
To
obtain
the
differential
equation
governing
the
behaviour
of
the
amplitude
a
of
the
acceleration
wave we
first
differentiate
(1)
partially
with
respect
to t and
(3)
partially
with
respect
to x . Then
eliminating
n
xt
' and
using
(7) and
(8),
we
find
2
(2lh
gcW
) 3 2 2
c
u-
-
u-
+
__
x +
__
x a +.=.lL a ,.
o.
xx
tt
c W c
Combining
(10)
and (11) and
using
(8)
brings
us to
the
required
(11)
Bernoulli
da +
dx
type
equation
for
the
(
3h
W )
3a2
4hx +
2;
a +
2h
,.
amplitude
a(x),
o , (12)
in
which
use
has
been
made
of
the
fact
that,
as
c
2
gh, we
have
(dc/dx)
=
gh/2c.
3. The Amplitude
a(x)
And
Its
Implications
-1
The
standard
substitution
a • b
reduces
the
Bernoulli
equation
(12)
to
a
linear
first
order
equation,
and a
simple
calculation
then
shows
that
in
which a
O
•
a(O),
W
o
•
W(O)
and
(13)
I(x)
3h
3/4
w
1/2
o 0
2
(14)

59
A wave
of
elevation
corresponds
to a
O
< 0, and a wave
of
depression
to "o > O.
The wave
will
be
said
to
break
if
for
some x g x
the
water
c
surface
behind
the
wavefront
becomes
vertical,
so
that
the
amplitude
a(x
c)
g
m.
Since
lex)
~
0,
we
conclude
from
the
form
of
(13)
that:
(a)
A wave
of
elevation
(a
o
.
<
0)
in
a
variable
width
channel
always
breaks
in
water
of
finite
depth
provided
lex)
is
such
that
1 +
aOI(x
c)
g
0,
and Xc > 0
is
finite.
If
the
depth
of
the
water
shelves
to
zero
at
x
~
t,
say,
so
that
h(t)
•
0,
a wave
of
elevation
propagating
towards
the
shore
will
break
before
reaching
the
shore
line
if
laol
>
l/l(t),
and
at
the
shore
line
if
laol
~
let).
(b) A wave
of
depression
(a
O
>
0)
in
a
variable
width
channel
can
only
break
if
the
depth
of
the
water
shelves
to
zero,
and
then
only
at
the
shore
line
provided
let)
<
m.
When
we
set
W(x) =W
O'
these
general
conclusions
agree
with
the
special
case
of
waves
climbing
a
beach
that
was
studied
by Greenspan
[3].
This
is
because
the
one-dimensivnal
long
wave
equations
do
not
distinguish
between
flow
in
a
parallel
channel
and
unrestricted
one-dimensional
flow.
Re
sult
(14) shows
that
the
integrand
of
importance
in
th
is
case
combines
the
depth
function
hex)
and
the
width
function
W(x)
in
the
form
(h(x»-7/4(W(x»-1/2.
Thus any
modification
of
the
depth
and
width
that
leaves
this
combination
invariant
will
lead
to
the
same
conditions
for
breaking
provided
a
O'
h
O
and W
o
are
unchanged.
As
special
cases
of
results
(13) and (14) we
observe
first
that
in
a
parallel
channel
of
constant
depth
h we
obtain
Stoker's
result
[2],
that
breaking
occurs
when
x
c
2h
- 3a
O
at
a
time
t
c
2
(E.g)
1/2
- 3a
O
(
(15)
Secondly,
when hex) • h - mx so
that
the
bottom
has
a
constant
slope,
we
obtain
Jeffrey's
result
[4]
that
breaking
occurs
when

60
x
c
(16)
Result
(16)
also
shows
that
when
the
water
deepens
at
a
constant
rate
(m <
0),
then
although
a wave
of
elevation
will
normally
break,
this
will
not
occur
in
the
special
case
that
2a
O
s m.
This
was
the
result
found
in
[4J which
used
the
transport
equation
approach
that
has
been
presented
in
a
general
form
in
[5J. The
equivalence
of
the
method
used
here
and
of
the
seemingly
different
one
used
in
[4J and
generalised
in
[5J
has
been
established
by
Boillat
and
Ruggeri
[6J.
References
- - - - -
[1) A.
Jeffrey
and
J.
Mvungi, On
the
breaking
of
water
waves
in
a
channel
of
arb
itrarily
varying
depth
and
width
.
ZAMP
(submitted
for
publication
.
[2J
J . J .
Stoker,
Water Waves.
Wiley-Interscience,
New
York, 1957 .
[3) H. P.
Greenspan,
On
the
breaking
of
water
waves
of
finite
,
amplitude
on a
sloping
beach.
J.
Fluid
Mech. 4
(1958),
330-334.
(4) A.
Jeffrey,
On a
class
of
non-breaking
finite
amplitude
water
waves.
Z. angew. Math. u. Phys.
(ZAMP)
, 18
(1967),
57-65.
See
also
Addendum, A.
Jeffrey,
Z. angew. Math. u. Phys.
(ZAMP),
18
(1967),
918.
(5) A.
Jeffrey,
Quasilinear
Hyperbolic
Systems and Waves,
Research
Note
in
Mathematics
5, Pitman
Publishing,
London, 1976.
(6) G.
Boillat
and T.
Ruggeri,
On
the
evolution
law
of
weak
discontinuities
for
hyperbolic
quasilinear
systems.
Wave
Motion 1
(1979),
149-151.
Lecture
6.
Shocks And Weak
Solutions
1.
Conservation
Systems and
Conditions
Across a Shock
In
what
follows
it
will
be assumed
that
the
system
of
equations
involved
is
hyperbolic
and
capable
of
expression
in
the
generalised
conservation
form. That
is,
when
the
system
involves
n
dependent
variabJ
~s
and
is
formulated
in
]R3
x
t,
we assume
it
can be
written
in
the
divergence
form
3F
111:
+
div
G -
H,
with
U c
U(~,t),
F =
F(U,~,t)
and H =
H(U,~,t)
all
n
element
column
matrix
vectors
and G -
G(U,~,t)
an n x 3
matrix
. The
matrix
G
in
(1)
is
in
effe
ct
to be
regarded
as
a
tensor
so
that
div
G
has
the
meaning
(1)
div
G -
3 3
(s)
r
~
s-l
s
where
8(s)
is
the
s-th
column
of
G.
Systems
of
this
type
are
of
considerable
importance
because
of
their
frequent
occurrence
in
phys{cal
problems where
they
arise
from
integral
formulations
of
quantities
that
are
conserved.
Indeed,
since
an
integral
formulation
is
more
fundamental
than
the
related
differential
equation
and
it
permits
the
integrand
to be
discontinuous,
we
shall
make
use
of
it
to
discuss
discontinuous
solutions
for
system
(1).
Discontinuous
solutions
have
considerable
physical
significance,
since
they
may be
interpreted
in
terms
of
physical
phenomena
such
as
a
shock
wave
in
a
gas.
If
a
discontinuous
solution
exists
across
a
surface,
the
first
problem to be
resolved
is
how
the
solutions
on
adjacent
sides
of
the
surface
are
to be
related
one
to
the
other
and to
the
speed
of
propagation
of
the
surface
.
In
the
case
of
a
shock
wave
in
a gas
this
involves
determining
the
relationship
connecting
gas
pressures
and
densities
on
opposite
sides
of
the
shock
with
the
speed
of
propagation
of
the
shock.
Theorem 1
(Integral
Rate
of
Change Theorem)
Let
F be an n x 1 column
matrix
with
elements·
which
are
continuous
scalar
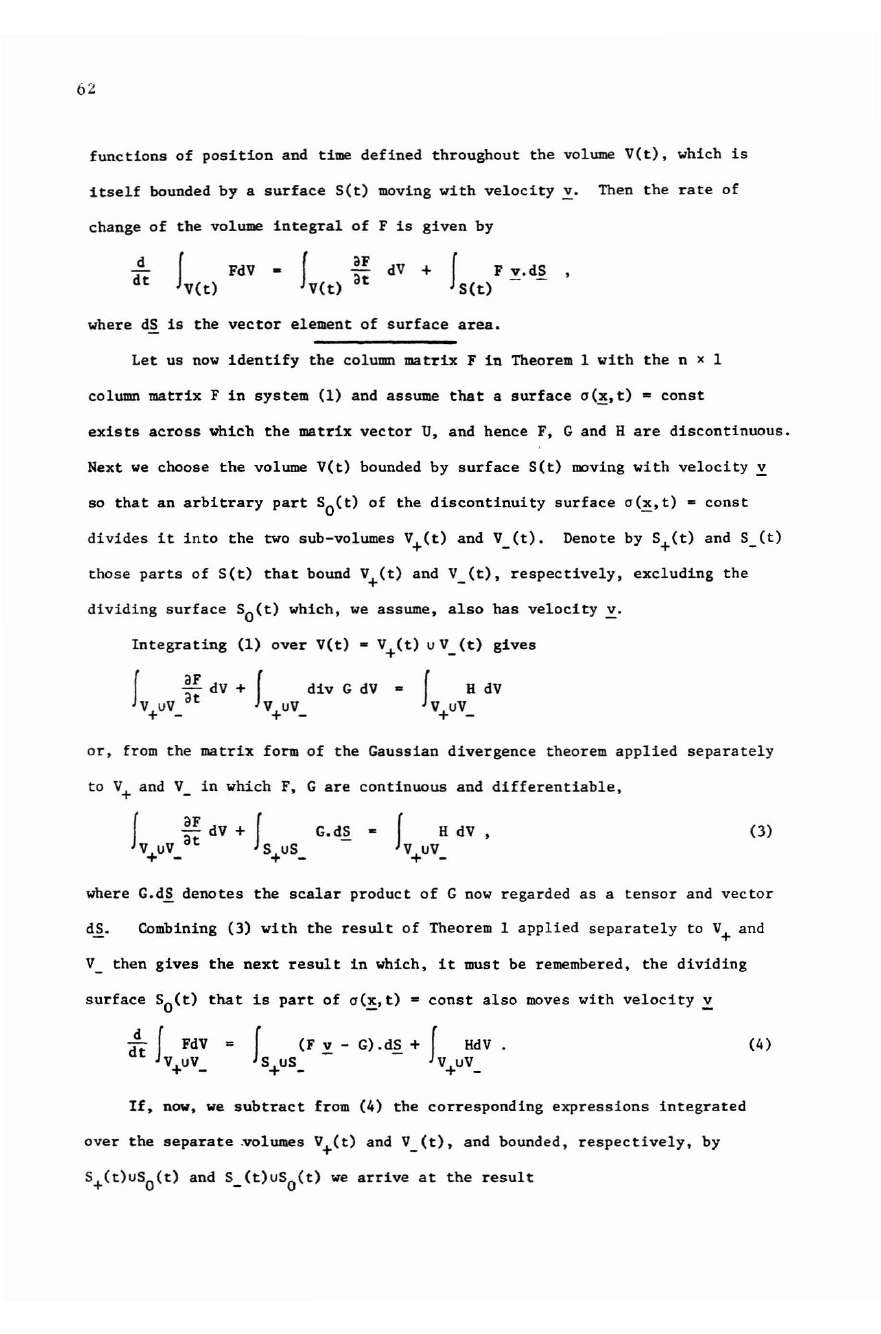
62
functions
of
position
and
time
defined
throughout
the
volume
V(t),
which
is
itself
bounded by a
surface
S(t)
moving
with
velocity~.
Then
the
rate
of
change
of
the
volume
integral
of
F
is
given
by
d
dt
I
FdV-
V(t)
J
ilF dV +
V(t)
at
I F
~.d.§.
S(t)
where
d.§.
is
the
vector
element
of
surface
area.
Let
us now
identify
the
column
matrix
F
in
Theorem 1
with
the
n x 1
column
matrix
F
in
system
(1)
and assume
that
a
surface
a(~,t)
=
const
exists
across
which
the
matrix
vector
U, and
hence
F, G and H
are
discontinuous.
Next we
choose
the
volume
V(t)
bounded by
surface
S(t)
moving
with
velocity
~
so
that
an
arbitrary
part
SO(t)
of
the
discontinuity
surface
a(~,t)
=
const
divides
it
into
the
two
sub-volumes
V+(t)
and
V_(t).
Denote
by
S+(t)
and S
(t)
those
parts
of
S(t)
that
bound
V+(t)
and
V_(t),
respectively,
excluding
the
dividing
surface
SO(t)
which,
we
assume,
also
has
velocity
~.
Integrating
(1)
over
V(t)
•
V+(t)
u V_
(t)
gives
f
~:
dV + J
div
G dV J H dV
V+UV_
V+UV_
V+uV_
or,
from
the
matrix
form
of
the
Gaussian
divergence
theorem
applied
separately
to
V+
and V
in
which
F, G
are
continuous
and
differentiable,
(3)
where
G.d.§.
denotes
the
scalar
product
of
G now
regarded
as
a
tensor
and
vector
dS. Combining
(3)
with
the
result
of
Theorem 1
applied
separately
to V+ and
V
then
gives
the
next
result
in
which,
it
must
be remembered,
the
dividing
surface
So(t)
ddt
J FdV
V+uV_
that
is
part
of
a(~,t)
=
const
also
moves
with
velocity
~
(4)
If,
now, we
subtract
from
(4)
the
corresponding
expressions
integrated
over
the
separate
.vol umes
V+(t)
and
V_(t),
and
bounded,
respectively,
by
S+(t)USO(t)
and
S_(t)USo(t)
we
arrive
at
the
result
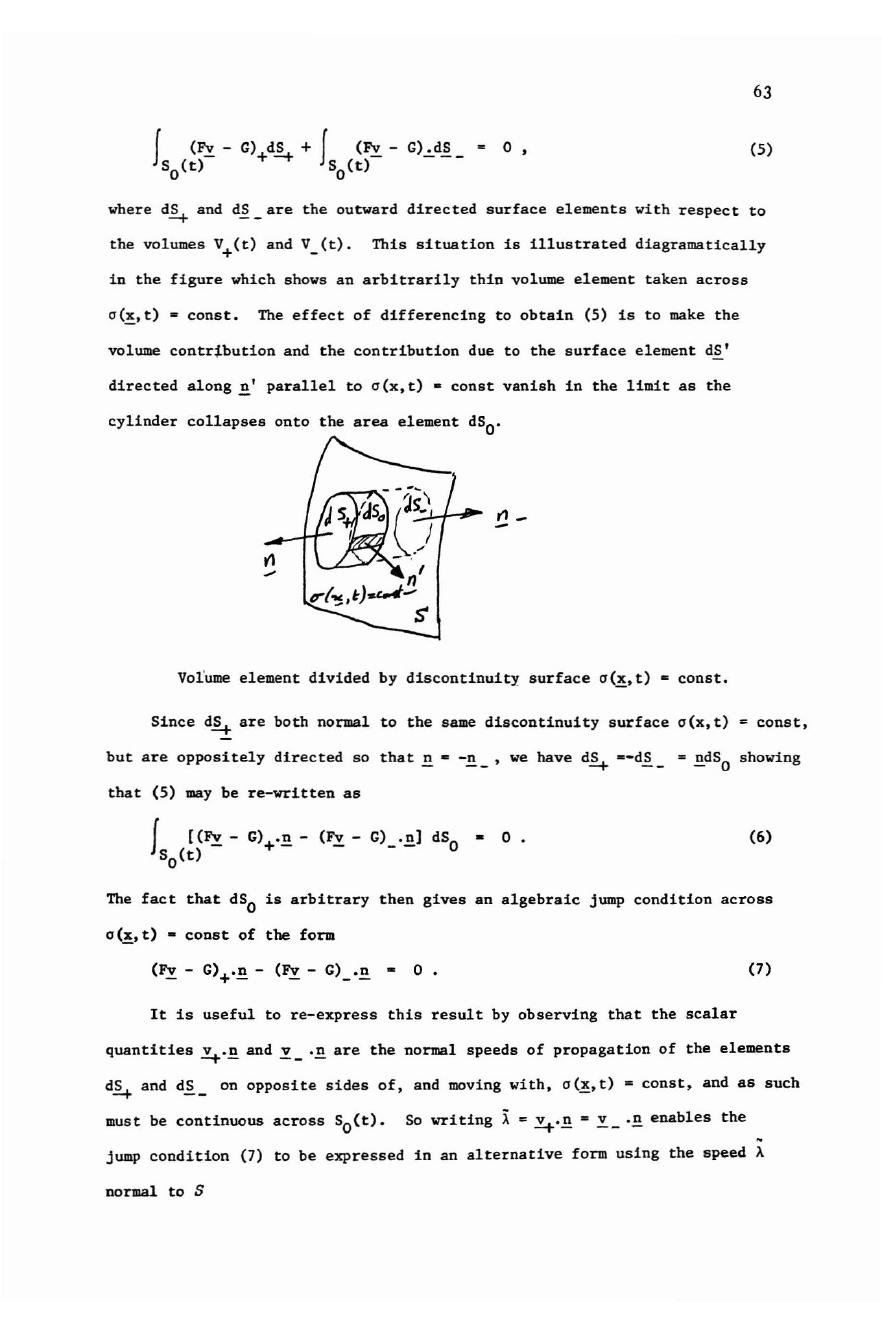
63
(5)
where
d~
and
d~
_
are
the
outward
directed
surface
elements
with
respect
to
the
volumes
V+(t)
and
V_(t).
This
situation
is
illustrated
diagramatically
in
the
figure
which
shows an
arbitrarily
thin
volume
element
taken
across
a(~,t)
=
const.
The
effect
of
differencing
to
obtain
(5)
is
to
make
the
volume
contribution
and
the
contribution
due
to
the
surface
element
dS'
directed
along
~'
parallel
to
a(x,t)
•
const
vanish
in
the
limit
as
the
cylinder
collapses
onto
the
area
element
dS
O•
r1_
-
Vofume
element
divided
by
discontinuity
surface
a(~,t)
•
const.
Since
d~
are
both
normal
to
the
same
discontinuity
surface
a(x,t)
=
const,
but
are
oppositely
directed
so
that
n =
-n
,we
have
d~
=-d~_
=
~dSo
showing
that
(5)
may be
re-written
as
(6)
The
fact
that
dS
O
is
arbitrary
then
gives
an
algebraic
jump
condition
across
a(.!.,t)
•
const
of
the
form
(7)
It
is
useful
to
re-express
this
result
by
observing
that
the
scalar
quantities
~.~
and
y_
.~
are
the
normal
speeds
of
propagation
of
the
elements
d~
and dS
on
opposite
sides
of,
and moving
with,
a(~,t)
=
const,
and
as
such
must be
continuous
across
SO(t).
So
writing
~
=
~.E.
=
y_.~
enables
the
jump
condition
(7)
to
be
expressed
in
an
alternative
form
using
the
speed
~
normal
to
S
