Farin G. Curves and Surfaces for CAGD. A Practical Guide
Подождите немного. Документ загружается.

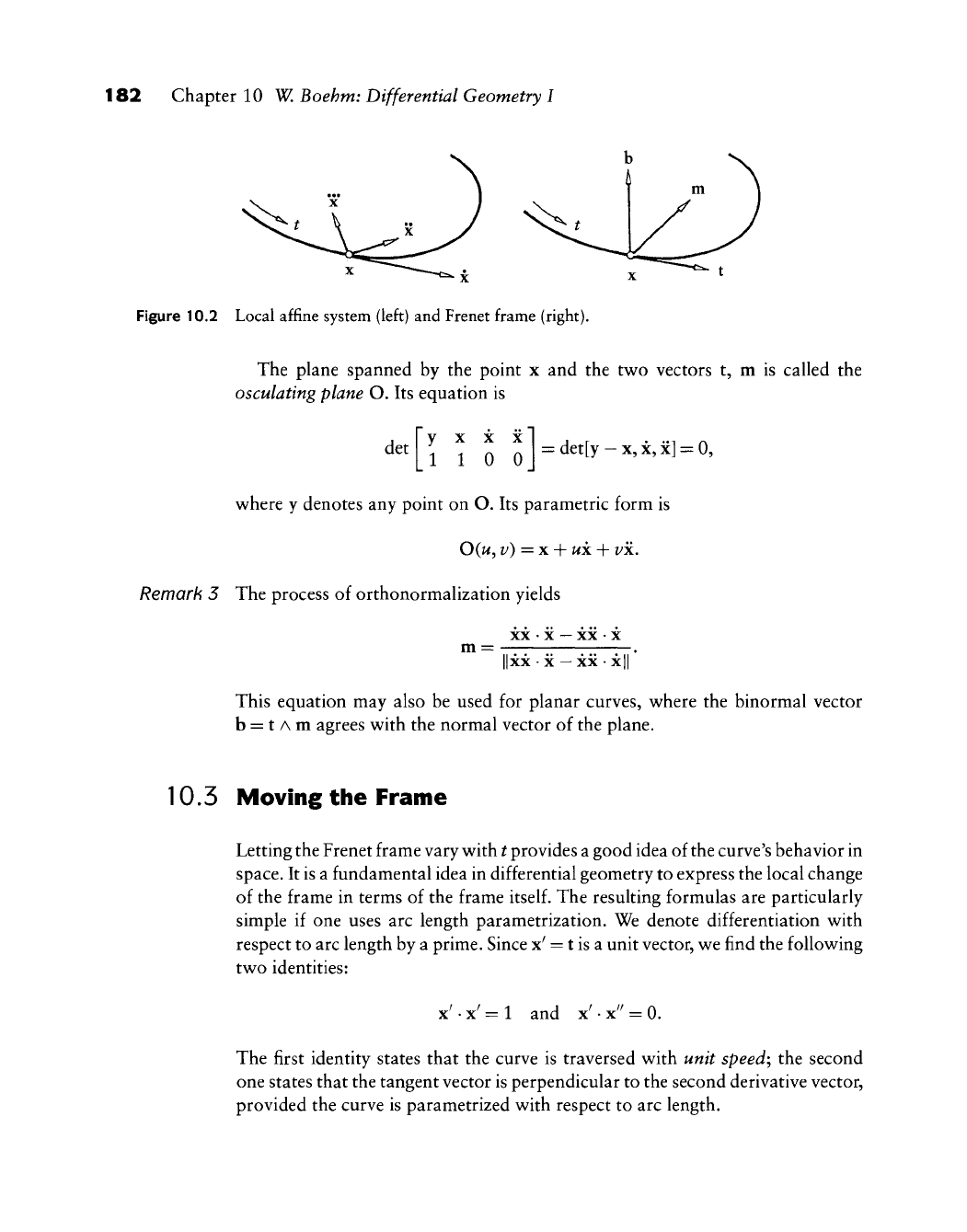
182 Chapter 10 W. Boehm: Differential Geometry I
Figure 10.2 Local affine system (left) and Frenet frame (right).
The plane spanned by the point x and the two vectors t, m is called the
osculating plane O. Its equation is
det
y X X x"j _
1 1 0 0j~
det[y
—
X,
x,x] = 0,
where y denotes any point on O. Its parametric form is
0(w, v) =x-\-ux-\- vx.
Remark 5 The process of orthonormalization yields
m =
XX
•
X — XX
•
X
XX
•
X — XX
•
X
This equation may also be used for planar curves, where the binormal vector
b = t
A
m agrees with the normal vector of the plane.
10.5 Moving the Frame
Letting the Frenet frame vary with t provides a good idea of the curve's behavior in
space. It is a fundamental idea in differential geometry to express the local change
of the frame in terms of the frame
itself.
The resulting formulas are particularly
simple if one uses arc length parametrization. We denote differentiation with
respect to arc length by a prime. Since x' = t is a unit vector, we find the following
two identities:
x^x' = l and x^x''=:0.
The first identity states that the curve is traversed with unit speedy the second
one states that the tangent vector is perpendicular to the second derivative vector,
provided the curve is parametrized with respect to arc length.
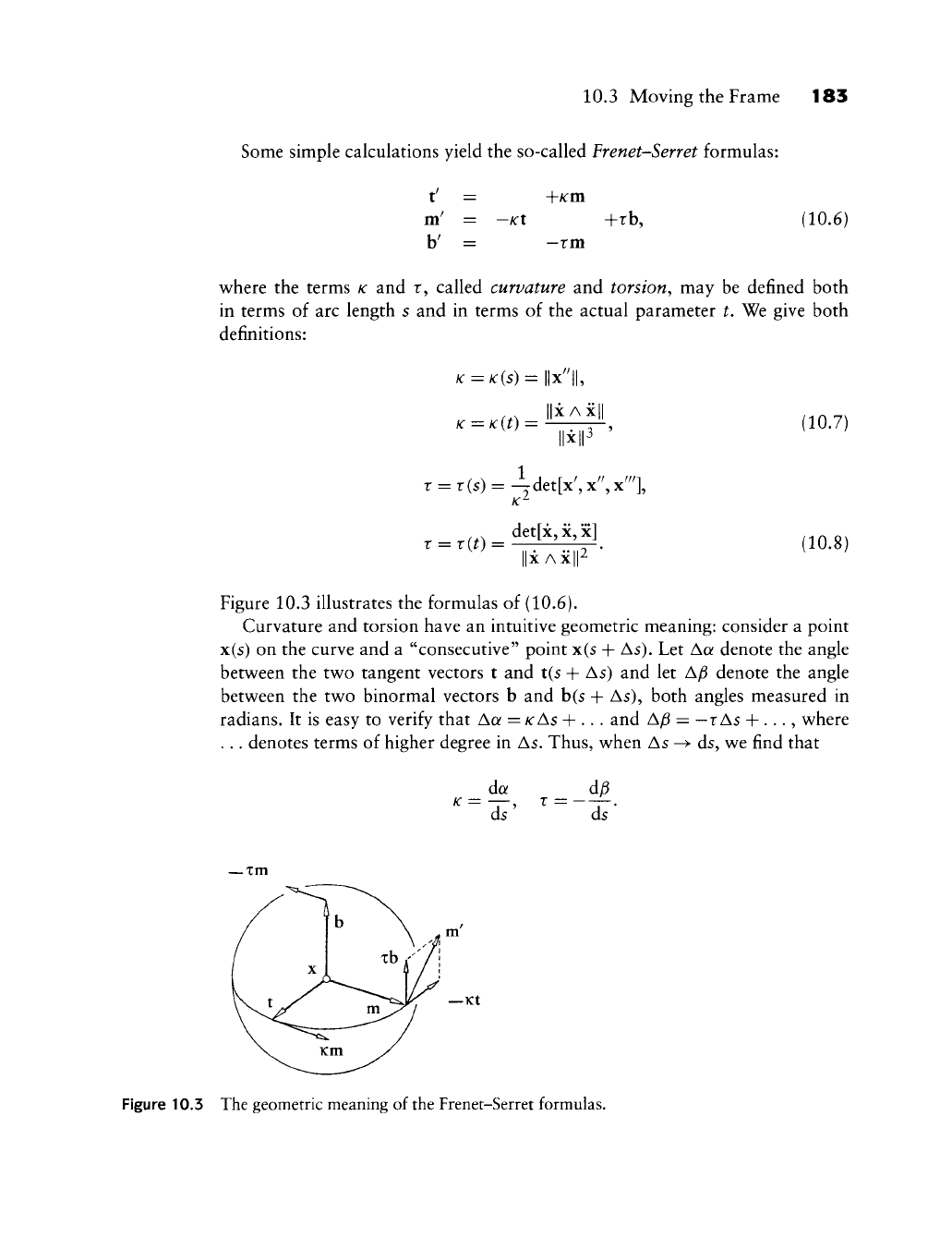
10.3 Moving the Frame 183
Some simple calculations yield the so-called Frenet-Serret formulas:
t' = +/cm
m' = —Kt +rb,
b'
= —rm
(10.6)
where the terms K and r, called curvature and torsion^ may be defined both
in terms of arc length s and in terms of the actual parameter t. We give both
definitions:
K=K{S)=\W'\1
IIXAXII
K=K(t) =
(10.7)
r = r(s) = ^det[x>^xn,
T = r(t) =
det[x,
X,
x]
X AX
|2 *
(10.8)
Figure 10.3 illustrates the formulas of (10.6).
Curvature and torsion have an intuitive geometric meaning: consider a point
x(s) on the curve and a "consecutive" point x(s + As). Let Aa denote the angle
between the two tangent vectors t and t(s + As) and let A^ denote the angle
between the two binormal vectors b and b(s + As), both angles measured in
radians. It is easy to verify that Aa = KAS -\-.. . and A^ = — r
As
+ ..., where
. . . denotes terms of higher degree in As. Thus, when As -> ds, we find that
K =
da
d7'
r =
ds'
— xm
—Kt
Figure 10.3 The geometric meaning of the Frenet-Serret formulas.
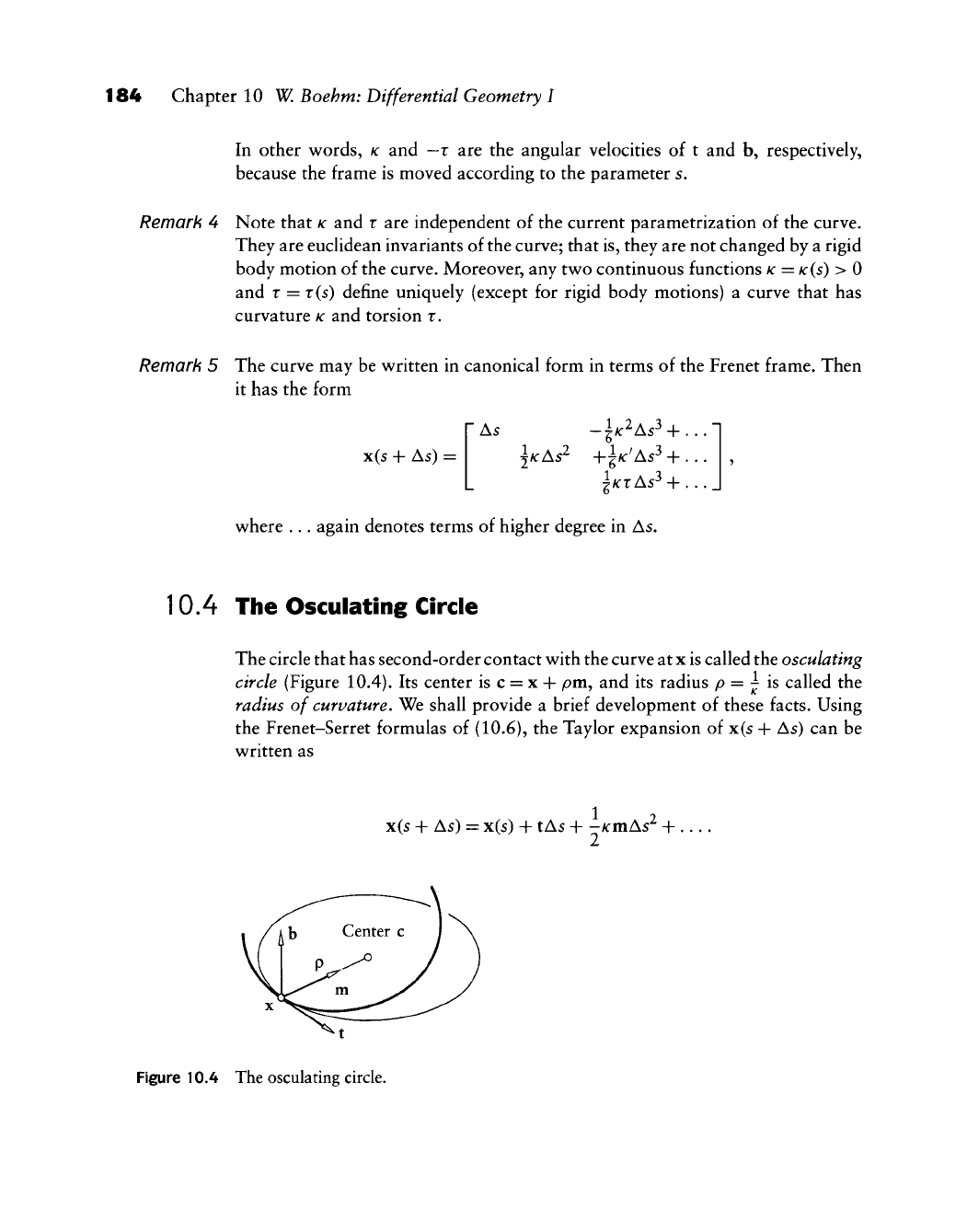
184 Chapter 10 W, Boehm: Differential Geometry I
In other words, K and — r are the angular velocities of t and b, respectively,
because the frame is moved according to the parameter 5.
Remark 4 Note that
K
and r are independent of the current parametrization of the curve.
They are euclidean invariants of the curve; that is, they are not changed by a rigid
body motion of the curve. Moreover, any two continuous functions
K
=K(S) > 0
and r = r(s) define uniquely (except for rigid body motions) a curve that has
curvature
K
and torsion r.
Remark 5 The curve may be written in canonical form in terms of the Frenet frame. Then
it has the form
x(s + As) =
As
|/crAs^
+ ...
where ... again denotes terms of higher degree in As.
10.4 The Osculating Circle
The circle that has second-order contact with the curve at x is called the osculating
circle (Figure 10.4). Its center is c = x + pm, and its radius p = ^ is called the
radius of curvature. We shall provide a brief development of these facts. Using
the Frenet-Serret formulas of (10.6), the Taylor expansion of x(s -f As) can be
written as
1 2
x(s + As) = x(s) +1As + -/cmAs + ...,
Figure 10.4 The osculating circle.
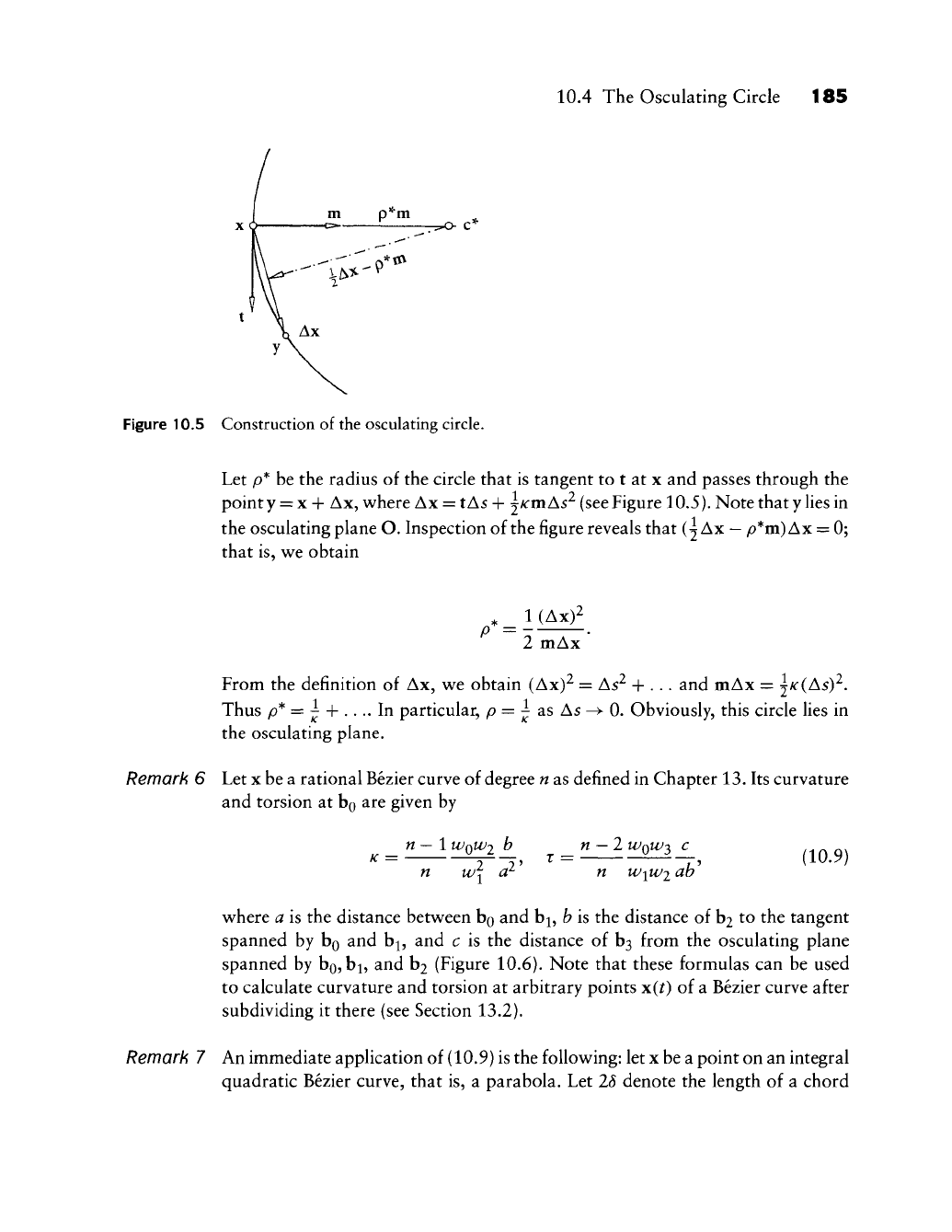
10.4 The Osculating Circle 185
m p'^m
Figure 10.5 Construction of the osculating circle.
Let p* be the radius of the circle that is tangent to t at x and passes through the
point y =
X
+ Ax, where Ax = tAs + ^/cmAs^ (see Figure 10.5). Note that y lies in
the osculating plane O. Inspection of the figure reveals that (^ Ax
—
p*m)
Ax = 0;
that is, we obtain
. ^ 1 (Ax)^
2 mAx
From the definition of Ax, we obtain (Ax)^ = As^ + ... and mAx = ^'^(As)^.
Thus p* = ^ + .... In particular, p = ^ as As ^- 0. Obviously, this circle lies in
the osculating plane.
Remark 6 Let x be a rational Bezier curve of degree n as defined in Chapter 13. Its curvature
and torsion at bo are given by
K =
_n ~ 1
WQWI
b
n
1 1'
n
—
1 WQW^ C
n WiWi ah
(10.9)
where a is the distance between bo and b^, h is the distance of h^ to the tangent
spanned by bo and b^, and c is the distance of b3 from the osculating plane
spanned by bo, bj, and b2 (Figure 10.6). Note that these formulas can be used
to calculate curvature and torsion at arbitrary points x(0 of a Bezier curve after
subdividing it there (see Section 13.2).
Remark 7 An immediate application of (10.9) is the following: let x be a point on an integral
quadratic Bezier curve, that is, a parabola. Let 28 denote the length of a chord
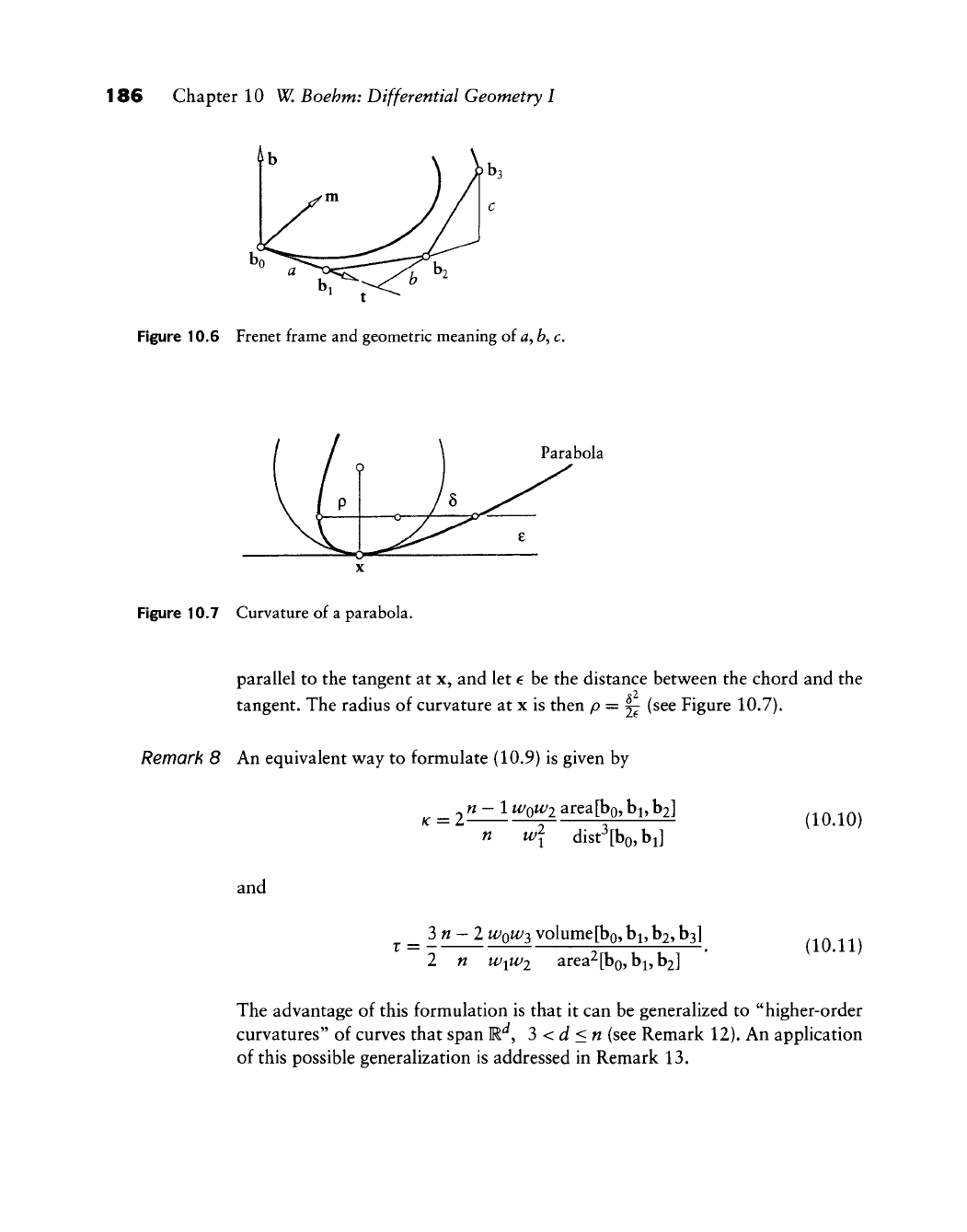
186 Chapter 10 W. Boehm: Differential Geometry I
Figure 10.6 Frenet frame and geometric meaning of
a,
b, c.
Parabola
Figure 10.7 Curvature of a parabola.
parallel to the tangent at x, and let € be the distance between the chord and the
tangent. The radius of curvature at x is then P = j^ (see Figure 10.7).
Remark 8 An equivalent way to formulate (10.9) is given by
n
—
1
WQWI
area[bo,
bj, b2]
K=l-
n w\ dist^[bo,bi]
and
_ 3 «
—
2
WQW2,
volume
[bo,
bj,
hi^
h^]
2 n wiWi area^[bo5bi,b2]
(10.10)
(10.11)
The advantage of this formulation is that it can be generalized to "higher-order
curvatures" of curves that span M^, 3 < d <n (see Remark 12). An application
of this possible generalization is addressed in Remark 13.
10.5 Nonparametric Curves 187
10.5 Nonparametric Curves
Let y = y(t); t e[a,
b]
be a function. The planar curve .
x
is called the graph
L
y{t) J
of y(t) or a nonparametric curve. From this, we derive the following:
the arc element:
the tangent vector:
the curvature:
ds
= y
1
+ y^dt,
K = —^
[l + y^J
and the center of curvature:
--¥[7]
Remark 9 Note that
K
has a sign here. Any planar parametric curve can be given a signed
curvature, for instance, by using the sign of det(x, x); see also (23.1).
Remark 10 For a nonparametric Bezier curve (see Section 6.5),
Where
U
=
UQ
+ tAu is a global parameter, we obtain
n^
n a
as illustrated in Figure 10.8.
188 Chapter 10 W, Boehm: Differential Geometry I
UQ Au/n
Figure 10.8 Curvature of nonparametric Bezier curve.
10.6 Composite Curves
A curve can be composed of several segments; we have seen sphne curves as an
example. Let x_ denote the right endpoint of
a
segment and
x_^
the left endpoint of
the adjacent segment. (We w^ill consider only continuous curves, so that x_ =
x_^
always.) Let
^
be a global parameter of the composite curve, and let dots denote
derivatives with respect to t. Obviously, the curve is tangent continuous if
x+=Qfx_. (10.12)
Moreover, it is curvature and osculating plane continuous if in addition
x_|. = Qf^x_ + a2ix_,
and it is torsion continuous if in addition
x^ = a x_ + a32X_ + 0f3ix_
(10.13)
(10.14)
and vice versa. Since we require the parametrization to be regular, it follows that
Q;
> 0, while the ajj are arbitrary parameters.
It is interesting to note that curvature and torsion continuous curves exist that
are not
K^
continuous^ (see Remark 4). Conversely,
x'^' = t'' =
K^m
+ K(-Kt + rb)
3 Recall that
K^
= d/c(s)/ds, v^here the prime denotes differentiation with respect to arc
length s of the (composite) curve. A formula for
K'
is provided by (23.2).

10.6 Composite Curves 189
implies that
x.'^'
is continuous if K' is and vice versa. To ensure
x.'^
= x^', the
coefficients a and of^y must be the result of the application of the chain rule; that
is,
with Qf2i =
)6
and a^^x^y^ one finds that
0^32
= 30?^. Now, as before, the curve
is tangent continuous if
x_^ = ax_, a > 0,
it is curvature and osculating plane continuous if in addition
..
9
.. .
X_^
=
Of
X_ + j6x_,
but it is /c' continuous if in addition
x^ = a\_ + 3a^x_ + yx_
and vice versa.
Remark / / For planar curves, torsion continuity is a vacuous condition, but
K'
continuity is
meaningful.
Remark
/
2 The preceding results may be used for the definition of higher-order geometric
continuity, A curve is said to be G^, or rth order geometrically continuous if a
regular reparametrization exists after which it is U. This definition is obviously
equivalent to the requirement of C^~^ continuity of
K
and C~^ continuity of r.
As a consequence, geometric continuity may be defined by using the chain rule,
as in the example for r = 3.
Remark 13 The geometric invariants curvature and torsion may be generalized for higher-
dimensional curves. Continuing the process mentioned in Remark 8, we find
that a J-dimensional curve has d
—
1 geometric invariants. Continuity of these
invariants makes sense only in E^, as was demonstrated for d = 2'm Remark 11.
Remark / 4 Note that although curvature and torsion are euclidean invariants, curvature and
torsion continuity (as well as the generalizations discussed in Remarks 12 and 13)
are affinely invariant properties of a curve. Both are also projectively invariant
properties; see Boehm [76] and Goldman and Micchelli
[267].
Remark 15 If two curve segments meet with a continuous tangent and have (possibly differ-
ent) curvatures K_ and
K_^
at the common point, then the ratio K_/K^ is also a
projectively invariant quantity. This is known as Memke's theorem; see Bol [88].
This Page Intentionally Left Blank
Geometric
Continuity
i4
Ns
u u
11.1 Motivation
Before we explain in detail the concept of geometric continuity, we will give
an example of a curve that is curvature continuous yet not twice differentiate.
Such curves (and, later, surfaces) are the objects that we will label geometrically
continuous.
Figure 11.1 shows three parabolas with junction points at the midpoints of
an equilateral triangle. According to (10.10), where we have to set all Wi equal
to 1, all three parabolas have the same curvature at the junction points. We thus
have a closed, curvature continuous curve. It is C^ over a uniform knot sequence.
But it is not C^ as is easily seen by sketching the second derivative vectors at the
junction points.
Differential geometry teaches us that our closed curve can be reparametrized
such that the new parameter is arc length. With that new parametrization, the
curve will actually be C^. Details are explained in Chapter 10. We shall adopt the
term G^ curves (second-order geometrically continuous) for curves that are twice
differentiable with respect to arc length but not necessarily twice differentiate
with respect to their current parametrization. Note that curves with a zero tangent
vector cannot be G^ under this definition. Planar G^ curves have continuously
varying signed curvature; G^ space curves have continuously varying binormal
vectors and continuously varying curvature.
191
