Dunajski M. Solitons, Instantons, and Twistors

Подождите немного. Документ загружается.

176 8: Symmetry reductions and the integrable chiral model

which case [193]

N = max

. (8.2.65)

The boundary conditions (8.2.16) imply that the ﬁnite-energy static solu-

tions to (8.2.13) are maps from S

(conformal compactiﬁcation of R

)into

U(n). In the moduli-space approximation we choose a class of such solutions

which are homotopic as maps of S

into U(n) and all have the same value

of potential energy. Ideally every such map ought to provide minimum of

the potential energy. This is the case on the level of the Grassmanian models

for constructions which involve (anti-)instanton solutions. For chiral models

one can show that all ﬁnite-energy static solutions are saddle points of the

potential energy functional [134]. This raises a question about stability of the

approximate solutions.

To summarize, for a given value of the topological charge, all solutions in

the class (8.2.63) can be described by a ﬁnite set of parameters which are the

positions of zeros and poles of holomorphic functions. To ensure ﬁnite values

of kinetic energy we needed to impose the base condition on the solutions by

ﬁxing their value at spatial inﬁnity. Then the parameters deﬁne a map on the

resulting moduli space.

8.2.4.2 Metric and magnetic ﬁeld

Next we allow the parameters to depend on time and so time-dependent

approximate solutions correspond to paths in the moduli space. Let us denote

the solutions contributing to the moduli space by J (γ ; x, y), where γ denote

real parameters in (8.2.64). Approximate time-dependent solutions are then of

the form J (γ (t); x, y) and time differentiation gives

= J

˙γ

, j =1,...,dim(M), where J

∂ J

∂γ

. (8.2.66)

The dynamics is governed by the action obtained as a restriction of (8.2.41) to

the moduli space





˙γ

+ A

˙γ



dt. (8.2.67)

group H

(CP

n−1

). This is isomorphic to Z.IfR : S

−→ CP

n−1

is a map from the compactiﬁed

space to CP

n−1

, representing a homology class R

∗

], we obtain the corresponding integer by

evaluating R

∗

] on a standard generator for H

(CP

n−1

) represented by the Kähler form .

In terms of differential forms, evaluating a cohomology class on a homology class just means

integrating, so the evaluation of R

∗

]on is given by the RHS of (A12). Now consider

the Hurewicz homomorphism from π

(CP

n−1

)toH

(CP

n−1

) sending the homotopy class of

R : S

−→ CP

n−1

to R

∗

], where [S

] ∈ H

) is the fundamental class. The projective space

n−1

is simply connected, so this is an isomorphism π

(CP

n−1

)=H

(CP

n−1

)=Z.

8.2 Integrable chiral model 177

The metric term can be obtained from the kinetic energy form (8.2.14) by using

(8.2.66):

T =

˙γ

, where h

= −



−1

dxdy, (8.2.68)

and the magnetic term can similarly be obtained from the WZW term, which

can be rewritten by the cyclic property of the trace as



Tr([

−1

]

−1

)dρdxdydt



˙γ

dt,

where



Tr([

−1

]

−1

)dρdxdy. (8.2.69)

Then A = A

dγ

is the magnetic one-form on the moduli space. We shall now

prove the following

Proposition 8.2.5 [56] The magnetic ﬁeld (8.2.70) vanishes on moduli spaces

constructed from embeddings (8.2.63) of Grassmanian solutions.

Proof One property of the WZW term is that its variation does not depend

on the particular choice of extension

J . We consider the variations restricted

to the moduli space δ J = J

δγ

, and ﬁnd

δS



−1

δ J, J

−1

]

dxdydt,

= −



−1

, J

−1

]

dxdy ˙γ

δγ

dt.

Comparing this expression with the variation of (8.2.69)

δS



˙γ

δγ

dt, F

= ∂

− ∂

gives

= −



−1

, J

−1

]

dxdy, (8.2.70)

where F =

(γ )dγ

∧ dγ

is the the magnetic ﬁeld. We can see that although

the magnetic one-form A in general depends on the choice of the extension

J ,

its exterior derivative F does not. Changing the extension merely corresponds

to a gauge transformation of A.

178 8: Symmetry reductions and the integrable chiral model

Note that the potential energy term has not been included in the effective

action (8.2.67). The potential is proportional to the topological charge (A12),

and does not contribute to the effective equations of motion.

Let us now consider a Grassmanian projector R depending smoothly on

some set of variables, which we shall denote by a, b, and c. From idempotency

and the Leibniz rule we deduce

= R

R + RR

and

= RR

+ R

= RR

+ R

− R

= RR

+ R

− R

+ R

R = RR

+ R

Taking the trace of the above expression gives

Tr( R

)=Tr(2RR

). (8.2.71)

If J is given by (8.2.63) then

−1

, J

−1

]

∼ Tr

(1 − 2R)R

, R

]

, (8.2.72)

where we have assumed that K

does not depend on parameters γ on the

moduli space to ensure the ﬁniteness of the kinetic energy. The RHS of (8.2.72)

vanishes because of (8.2.71), which in turn implies the vanishing of the mag-

netic ﬁeld (8.2.70). 

We deﬁned the metric (8.2.68) as a restriction of the kinetic energy to M

Its completeness is equivalent to the requirement that the kinetic energy is ﬁnite

along all curves in M

. Although the base condition was necessary to ensure

ﬁnite kinetic energies, it appears not to be sufﬁcient as the metric is complete

only on leaves of appropriate foliation of M

[172, 153] and we need to

restrict the dynamics to these leaves. These restrictions are assumed to hold

and we will use the symbol M

to denote some particular leaf.

The moduli-space metric can be obtained explicitly from (8.2.68) by using

(8.2.63), (A11), and (8.2.64). This metric is Kähler with respect to the natural

complex structure induced by map (8.2.64), with the Kähler potential





n−1



l=1

(|p

+ |r

)dxdy. (8.2.73)

Example. Consider the case G = SU(2) where the static ﬁnite-energy solu-

tions are of the form (8.2.23).

In the moduli-space approximation J stays on the equatorial S

⊂ S

, and

the lumps are located where J departs from its asymptotic value (8.2.16). In

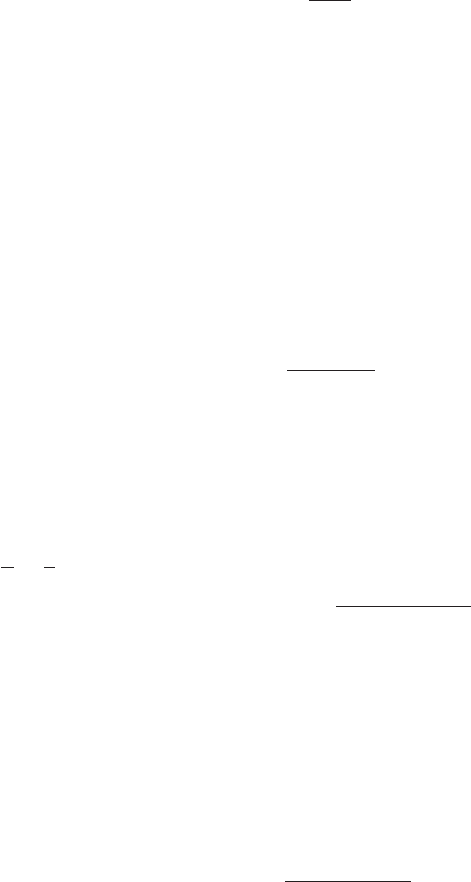
8.2 Integrable chiral model 179

these regions the energy density of (8.2.14) attains its local maxima. The

velocities of the lumps are the velocities of these local maxima.

The charge-one solution is given by

f = α +

z + γ

, (8.2.74)

and we need to ﬁx α and β in order for (8.2.68) to be well deﬁned. Choosing

α =0,β =1,γ = γ (t), and setting γ (t)=r(t)e

iθ (t)

we ﬁnd the metric and the

one-form

h =8π(dr

+ r

dθ

) and A =4π

d(r cos θ).

Therefore the metric is ﬂat, and the motion is along straight lines, γ (t)=−vt,

because dA= 0 does not contribute to the Euler–Lagrange equations. The

energy density is approximated by

E =(1+|z − vt|

)

−2

Next we look at the charge-two case:

f = α +

βz + γ

+ δz + κ

. (8.2.75)

The corresponding metric was constructed by Ward [172]. The parameters

α and β have to be ﬁxed to ensure ﬁniteness of kinetic energy, and δ can be

set to 0 by exploiting the translational invariance of (8.2.23). Moreover the

Möbius transformations can be used to ensure α =0,β ∈ R, and here Ward

makes an additional choice β = 0. The resulting metric is therefore deﬁned

on four-dimensional leaves of a foliation of M

, with local coordinates

(γ,

γ,κ,κ). The Kähler potential is given by



= −4π|κ| + π|γ |



π/2



1+|κ/γ |

sin

θdθ.

The structure is invariant under the torus action and a homothety

γ → e

iτ

γ, κ → e

iτ

κ, and |γ |

+ |κ|

→ τ

(|γ |

+ |κ|

In the next two examples we shall compare the moduli-space motion with the

limiting cases of exact soliton solutions [54]

Example. Consider the one-soliton solution (8.2.22). The energy density

E =2sin

(1 + |µ|

)

| f



|µ|

(1 + | f |

)

We remark that the boundary condition for f in these examples is f (z) −→ 0as|z|−→∞,

and hence J

= iσ

. The conclusion F = 0 does not depend on these boundary conditions.

180 8: Symmetry reductions and the integrable chiral model

has local maxima which give the locations {(x

, y

), a =1,...,N} of N

lumps. The velocities (

)=(−2|µ|cos φ/(1 + |µ|

), (1 −|µ|

)/(1 + |µ|

))

are the same for each lump so (8.2.22) should be regarded as a one-soliton

solution. To make contact with the moduli-space approximation write

= cos φ1 + ia · σ

to reveal that cos φ measures the deviation of J

from the unit sphere in the

Lie algebra su(2). If J is initially tangent to the space of static solutions, then

cos φ = 0, and we can set µ = i(1 + ε), where ε ∈ R. The solution is of the

form (8.2.23), but f is rational in

ω = z + ε(z + it)+



z −

+ it



f (ω)=

(z − Q

) ···(z − Q

)

(z − Q

N+1

) ···(z − Q

)

where the Q’s are linear functions of (ε

z,εt). The (squared) velocity is

=1− 4(1 + ε)

1+(1+ε)

so in the non-relativistic limit (which underlies the moduli-space approxima-

tion) we regard ε as small. Therefore the Q’s depend only on t, and they

all move at velocity ε. Setting N = 1, we recover the charge-one solution

(8.2.74). More generally we ﬁnd that J is given by (8.2.23) with

f = f

(z)+tf

(z),

where f

= f (ω)|

ε=0

and f

= ∂ f/∂ε|

ε=0

are rational functions of z.

Example. In Theorem 8.2.3 we have demonstrated that the total rest-frame

energy of the solution (8.2.25) is quantized in units of 8π . Solutions to

(8.2.13) obtained in the moduli-space approximation have energies close to

their potential energy as their kinetic energy is small. We should therefore

expect that some of these approximate solutions arise from (8.2.25) by a

limiting procedure.

Consider (8.2.25) and for convenience set f = −if

/2 and h = −if

/2.

To demonstrate how the limiting procedure is achieved ﬁrst observe that

solutions to (8.2.13) are deﬁned up to a multiple by a constant element of

SU(2). The static solution (8.2.23) with f = f

arises from (8.2.25) by using

this freedom and setting f

=0:



i 0

0 −i



1+| f



1 −|f

2 f

| f

− 1



= J

static

Moreover the energy density of (8.2.25) has maxima where f = f

+ tf



=0.

The lumps are located at the zeros z

= z

(t), a =1,...,deg f of f and the

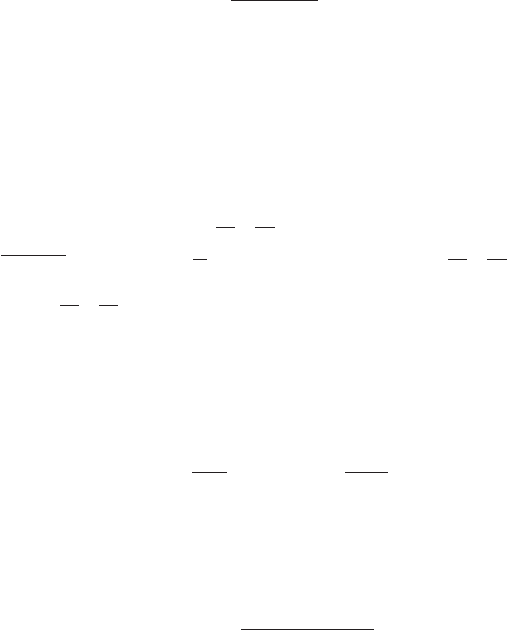
8.2 Integrable chiral model 181

squared velocity of each lump is

| f



| f



+ tf



)

z=z

so that | f



is small in the non-relativistic limit. Therefore | f

| is also small

as we choose J

to be tangent to the space of static solutions at t = 0. Keeping

only the linear terms in f

in (8.2.25) yields



i 0

0 −i



1+| f |



1 −|f |

− i( f

+ f

)2f

f | f |

− 1 − i( f

+ f

)



The term ( f

+ f

) can be dropped by rescaling the coordinates x

→

/ε.

Comparing the resulting expression with (8.2.23) will give a motion on the

moduli space of static solutions if f

, tf



, and ( f

)

lie in the common space

of rational maps of degree deg f

. To achieve this, we therefore take

p(z)

q(z)

and f

r(z)

q(z)

where r is of degree 2N and p and q are of degree at most N. The total

energy is equal to 8π deg f

. The resulting motion on the moduli space of

static solutions of charge deg f

is given by (8.2.23) with

f (z, t)=

r + t(p



q − pq



)

. (8.2.76)

This motion is restricted to a geodesic submanifold as the parameters in the

denominator of f are ﬁxed. In particular, setting q = 1, we can take f

(z)

to be a polynomial of degree 2N and f

(z) to be a polynomial of degree at

most N.

8.2.5 Mini-twistors

The geometric interpretation of the Lax representation (8.2.11) is the follow-

ing: For any ﬁxed pair of real numbers (µ, λ) the plane

µ = v + xλ + uλ

(8.2.77)

is null with respect to the Minkowski metric h = dx

− 4dudv on M = R

2,1

and conversely all null planes can be put in this form if one allows λ = ∞. The

182 8: Symmetry reductions and the integrable chiral model

two vector ﬁelds

= ∂

− λ∂

and δ

= ∂

− λ∂

(8.2.78)

span this null plane. Thus the Lax equations (8.2.11) imply that the generalized

connection (A,) is ﬂat on null planes. This underlies the twistor approach

[174, 177], where one works in a complexiﬁed Minkowski space C

, and

interprets (µ, λ) as coordinates in a patch of the mini-twistor space Z = TCP

with µ ∈ C being a coordinate on the ﬁbres and λ ∈ CP

being an afﬁne

coordinate on the base.

In this section we shall study the geometry of the mini-twistor space, and

its compactiﬁcation in some detail. It is convenient to make use of the spinor

formalism based on the isomorphism

TM = S  S,

where S is rank-two real vector bundle (spin bundle) over the (2+1)-

dimensional Minkowski space. The ﬁbre coordinates of this bundle are

denoted by

(π

,π

). Rearrange the space-time coordinates (u, x,v)asasym-

metric two-spinor



ux/2

x/2 v



such that the space-time metric and the volume form are

h = −2dx

and vol

= dx

∧ dx

As usual, two-dimensional spinor indices are raised and lowered with the

symplectic form ε

, such that ε

=1.

The mini-twistor space of R

2,1

is the two-dimensional complex manifold

Z = TCP

which is the total space of the line bundle O(2) of Chern class 2

over CP

. Points of Z correspond to null two-planes in R

2,1

via the incidence

relation

= ω. (8.2.79)

Here (ω, π

,π

) are homogeneous coordinates on Z:(ω, π

) ∼ (c

ω, cπ

where c ∈ C

∗

. In the afﬁne coordinates λ := π

/π

and µ := ω/(π

)

equation

(8.2.79) gives (8.2.77).

First ﬁx (ω, π

). If (µ, λ) are both real then (8.2.79) deﬁnes a null plane in

2,1

. If both µ and λ are complex then the solution to (8.2.79) is a time-like

curve in R

2,1

. We shall say that this curve is oriented to the future if Im(λ) > 0

Strictly speaking the spinor indices used in this section should be primed if one views the

integrable chiral model as a reduction of ASDYM. However only one type of indices will be used,

so using unprimed indices should not lead to misunderstandings.

8.2 Integrable chiral model 183

and to the past otherwise. If λ is real and µ is complex then (8.2.79) has no

solutions for ﬁnite x

An alternate interpretation of (8.2.79) is to ﬁx x

. This determines ω as

a function of π

, that is, a section of O(2) → CP

when factored out by the

relation (ω, π

) ∼ (c

ω, cπ

). These are embedded rational curves with self-

intersection number 2, as inﬁnitesimally perturbed curve µ + δµ with δµ = δv +

δxλ + δuλ

intersects (8.2.77) at two points.

Two rational curves L

and L

(corresponding to p

=(u

, x

) and p

, x

), respectively) intersect at two points

1,2

∓



h(R, R)

, where R

:= (u

− u

, x

− x

− v

Therefore the incidence of curves in Z encodes the causal structure of R

2+1

the following sense: L

and L

intersect at (a) one point, (b) two real points,

and (c) two complex points conjugates of each other, iff p

, p

are (a) null

separated, (b) space-like separated, and (c) time-like separated.

Examining the relevant cohomology groups (see formula (B5)) shows that

the moduli space of curves with self-intersection number 2 (and so the normal

bundle O(2)) in Z is C

. The real space-time R

2,1

arises as the moduli space

of curves that are invariant under the conjugation (ω, π

) → (¯ω, ¯π

), which

corresponds to real x

The correspondence space is

F = C

× CP

= {(p, Z) ∈ C

× Z|Z ∈ L

By deﬁnition, it inherits ﬁbrations over both C

and Z, and the ﬁbration of

F = C

× CP

over Z has ﬁbres spanned by the distribution δ

= π

∂

, where

∂

=1/2(ε

+ ε

). In the afﬁne coordinates where π

=(1, −λ)

this distribution is given by (8.2.78) where we have ignored the constant

factor π

8.2.5.1 Ward correspondence

There is a one-to-one correspondence between the gauge equivalence classes of

complex solutions to (8.2.8) in complexiﬁed Minkowski space, and holomor-

phic vector bundles over the mini-twistor space TCP

which are trivial on the

holomorphic sections of TCP

→ CP

. The proof is entirely analogous to that

of Theorem 7.2.2. Here we shall concentrate on the backward construction,

where the additional subtlety arises due to the choice of Ward gauge leading

to the integrable chiral model (8.2.13).

Let F be a patching matrix for a vector bundle over TCP

. Restrict F to a

section (8.2.79) where the bundle is trivial, and therefore F can be split

F =

−1

184 8: Symmetry reductions and the integrable chiral model

where H and

H are holomorphic in π

around π

= o

=(1, 0) and π

= ι

(0, 1), respectively. As a consequence of δ

F = 0, the splitting matrices satisfy

−1

H =



−1



H = π



, (8.2.80)

where



= 

(AB)

+ ε



gives a one-form A = 

and a scalar ﬁeld  on the complexiﬁed

Minkowski space, that is,





+ 

−  A



Let

h := H(x

,π

= o

) and

h :=



H(x

,π

= ι

)

so that



= h

−1

∂

h and 

−1

∂

The splitting matrices are deﬁned up to a multiple by the inverse of a non-

singular matrix g = g(x

) independent of π

H → Hg

−1

and



H →



−1

This corresponds to the gauge transformation (8.2.9) of 

We choose g such that

h = 1 so



= ι



and



= −ι

−1

∂

that is,

+  = A

=0.

This is the Ward frame with J (x

)=h. The Higgs ﬁeld is given by

 =



= V

−1

∂

where V = o

∂

= ∂

is the unit space-like vector which breaks the Lorentz

invariance. The Lax pair (8.2.11) becomes

= δ

+ H

−1

8.2 Integrable chiral model 185

where δ

= π

∂

, so that

−1

)=−H

−1

(δ

H)H

−1

+ H

−1

(δ

H)H

−1

and  = H

−1

is a solution to the Lax equations regular around λ =0.Inthe

Ward frame

J (x

)=

−1

,λ =0)

is a solution to (8.2.13). Let us show explicitly that (8.2.13) holds. Differenti-

ating both sides of (8.2.80) yields

−1

H)=−(H

−1

H)(H

−1

which holds for all π if

A(C



where D

= ∂

+ 

. This is the spinor form of the Yang–Mills–Higgs

system (8.2.8). In the Ward gauge the system reduces to

∂



which is (8.2.13).

8.2.5.2 Abelian case and the wave equation

In the abelian case j = log (J ) satisﬁes the three-dimensional wave equation

∂

∂x

−

∂

∂u∂v

=0.

Repeating the steps leading to (7.2.24) we ﬁnd the integral formula

j =





f (ω, ρ)

(ρ · ι)(ρ · o)

ρ · dρ,

where  is a real contour in a rational curve ω = x

and f is a cohomol-

ogy class homogeneous of degree zero in ρ giving rise to a patching function

F = e

. This solution is gauge dependent as the integrand depends on a choice

of the normalized spin dyad (o,ι). The abelian Higgs ﬁeld also satisﬁes the

wave equation and is given by a gauge-independent formula

 =





∂ f

∂ω

ρ · dρ.

8.2.5.3 Compactiﬁed mini-twistor space

The solutions to (8.2.8) which satisfy the trivial scattering boundary condition

(8.2.29) extend to a compactiﬁcation of the mini-twistor space. In this section