Dunajski M. Solitons, Instantons, and Twistors

Подождите немного. Документ загружается.

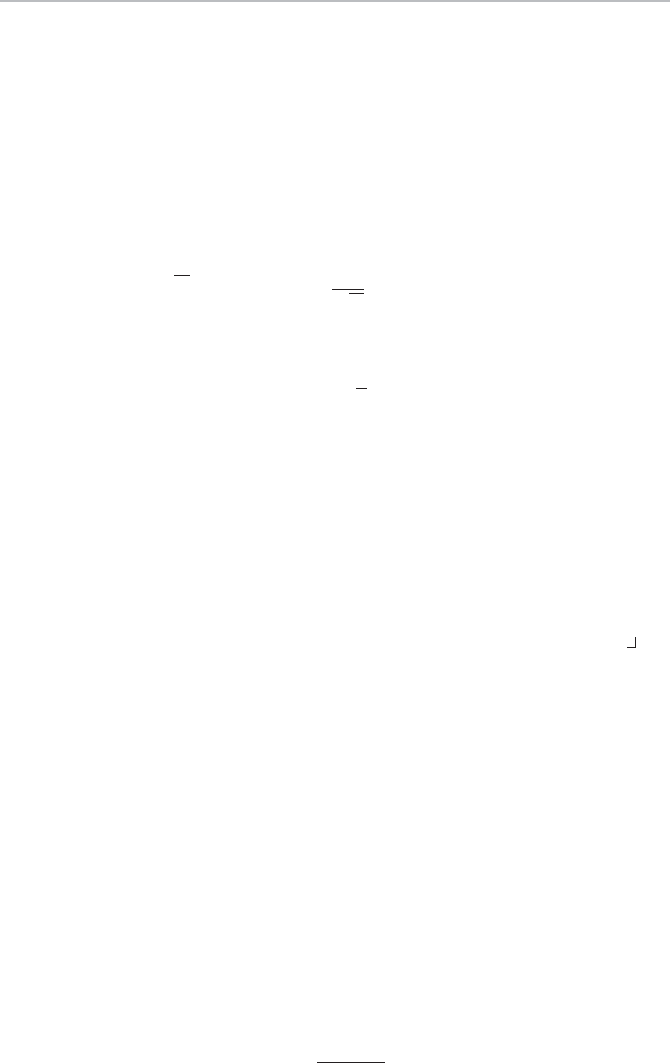
206 9: Gravitational instantons

9.4 Multi-centred gravitational instantons

We shall discuss a large class of gravitational instantons which depend on a

harmonic function on R

. Consider a metric of the form

g = V(dx

+ dx

)+V

−1

(dτ + A)

, (9.4.35)

where V = V(x

) and A = A

)dx

are a function and a one-form, respectively,

which do not depend on τ . This is known as the Gibbons–Hawking ansatz

[71]. Choosing the tetrad

√

Vdx

and e

√

(dτ + A), i =1, 2, 3

gives the basis of SD two-forms:



=(dτ + A) ∧ dx

V

ijk

∧ dx

The ASD Ricci-ﬂat equations are imposed as the closure condition (9.3.30).

This gives the monopole equation

∗

dV = dA, (9.4.36)

where ∗

is the Hodge operator on R

with its ﬂat metric. Differentiating

this equation implies that V has to be a solution to the Laplace equation

on R

The vector ﬁeld K = ∂/∂τ is Killing, that is, Lie

g = 0, which generates the

action, and x

are deﬁned up to addition of a constant by dx

= K 

In particular all SD two-forms (or equivalently the Kähler forms in the

hyper-Kähler structure) are Lie derived along K. This means that K is tri-

holomorphic. Conversely we have the following result:

Theorem 9.4.1 Any four-dimensional hyper-Kähler metric which admits a tri-

holomorphic Killing vector can be locally put in the form (9.4.35) where the

function V and the one-form A on the space of orbits of the Killing vector

satisfy (9.4.36).

Proof Any Riemannian metric which admits a Killing vector takes the form

g = h + V

−1

(dτ + A)

where τ parameterizes the orbits of K = ∂/∂τ (see Appendix C for the proof

that τ always exists locally) and (h, A, V) are a metric, a one-form, and a

function on the space of orbits of K. The function V is deﬁned by

V =

g(K, K)

. (9.4.37)

9.4 Multi-centred gravitational instantons 207

The tri-holomorphic condition Lie



= 0 gives

d

+ d(K 

)=0.

The Kähler forms are closed, thus we can use the Poincaré lemma to deduce

the local existence of functions x

, i =1, 2, 3, on M such that

= K 

. (9.4.38)

These functions are Hamiltonians (also called moment maps) for the S

action

generated by K. The metric is Hermitian with respect to all three complex

structures, thus

g(I

(K), I

(K)) = g(K, K), i =1, 2, 3 (no summation).

But g(I

(K), I

(K)) = |dx

. Therefore

= g

∇

= g

∇

= g

∇

This gives h = V(dx

+ dx

) and the metric is of the form (9.4.35).

Imposing the hyper-Kähler conditions leads, as we have already demonstrated,

to (9.4.36) which completes the proof.



Example. Consider the ﬂat metric on R

= C

with holomorphic coordinates

(w, z)

g = |dz|

+ |dw|

with the hyper-Kähler structure given by



(dz ∧ d

z + dw ∧ dw) and 

+ i

= dz ∧ dw.

(This is of the form (9.3.31) with  = |z|

+ |w|

.) Now consider an isometric

and tri-holomorphic S

action

(z,w) −→ (e

ic/2

z, e

−ic/2

w),

where c is the constant group parameter. Using the formula (4.2.4) we ﬁnd

that this action is generated by the Killing vector

K =



∂

∂z

−

∂

∂z



−



∂

∂w

−

∂

∂w



Formulae (9.4.38) give

(|z|

−|w|

) and x

+ ix

zw,

A different proof of Theorem 9.4.1 based on exterior differential systems is given in

Appendix C.

208 9: Gravitational instantons

and (9.4.37) implies

V = g(K, K)

−1

|z|

+ |w|

(9.4.39)

because r

:= x

+ x

=(|z|

+ |w|

)

/16. Thus the Gibbons–Hawking

ansatz with the simple harmonic function V = r

−1

gives ﬂat space. We also

see that the apparent singularity r = 0 in the Gibbons–Hawking metric can

be removed by a coordinate transformation. To ﬁnd the one-form A in this

case write the ﬂat metric on R

+ e

, where e

= dr, e

= rdθ, and e

= r sin θdφ.

The relation ∗

= e

∧ e

gives ∗

dr = r

sin θ dθ ∧ dφ and so

∗

dV = −

∗

dr = d(cos θ dφ).

Thus we can take

A = cos θ dφ.

Allowing V to have point singularities modelled on r

−1

and modifying V

by adding a constant leads to non-ﬂat metrics. We shall consider V of the

form

V = V



m=1

| x − x

where x

,...,x

are ﬁxed points in R

called the centres and V

= const.

If (r

,θ

,φ

) are spherical polar coordinates centred at the points x

then,

repeating the argument above, we ﬁnd that there exists a trivialization of the

bundle over R

such that A is gauge equivalent to

A =



m=1

cos θ

dφ

The behaviour of the corresponding metrics essentially depends on whether

the constant V

vanishes or not.

Example. Taub-NUT. Consider the Gibbons–Hawking metric with

V = V

, V

=0.

9.4 Multi-centred gravitational instantons 209

This is equivalent to the Taub-NUT metric (9.1.4). To see it set r = m +2

r/m

in (9.1.4). This gives

g = V



(dθ

+sin

θdφ

)



+ V

−1

(dψ + cos θ dφ)

, where

V =

which is in the Gibbons–Hawking form. In particular we have now veri-

ﬁed that the Taub-NUT metric is indeed a solution to the ASD Ricci-ﬂat

equations.

Allowing more singularities leads to large classes of multi-instantons general-

izing both the Taub-NUT and the Eguchi–Hanson solutions:

The A

N−1

ALE metrics [62, 71] are given by

V =



m=1

| x − x

We have seen that the case N = 1 is the ﬂat metric on R

. Consider N > 1.

The apparent singularities at x − x

are removable. To prove this ﬁrst analyse

the asymptotic behaviour |x|→∞. This gives

V ∼

and, by the previous calculation, A ∼ N cos θdφ. The metric is

g ∼ N



+ r

[

d(τ/N)+(A/N)

]



Setting r = ρ

/4 yields

g ∼ dρ

+ σ

[

d(τ/N) + cos θ dφ

]

(

which is regular if τ is periodic with a period 4π N. Thus asymptotically we

recover the ﬂat metric on R

Now, focusing near any of the centres x

and shifting the origin to x

gives

V ∼

Therefore we can reverse our analysis of the ﬂat metric (9.4.39) to deduce

that r = 0 is a coordinate singularity and near the origin the metric looks like

the ﬂat metric on R

The Eguchi–Hanson metric (9.1.3) can be put in this form with N =2

where the two centres lying on the x

-axis are separated by a

/4: Following

210 9: Gravitational instantons

[126] deﬁne

= |x − x

| and r

−

= |x − x

−

and set

τ =2φ, r

± a

cos θ ),φ

= φ

−

= ψ, and

cos θ

cos θ ± a

where (r

,θ

,φ

) are spherical polar coordinates around the two points x

With this ansatz the two-centre ALE multi-instanton gives (9.1.3). This also

shows that the Eguchi–Hanson metric is hyper-Kähler.

The A

N−1

ALF metrics [62, 71] are given by

V =1+



m=1

| x − x

(9.4.40)

where we rescaled the potential to set the non-zero constant V

to one.

This time we ﬁrst analyse the behaviour around the centres. Shifting the

origin to x

gives V ∼ r

−1

when r → 0. The analysis of this case is identical

to the ALE case we have just considered – we ﬁnd that the metric is ﬂat near

any of the centres. Now we consider the asymptotic region r →∞where

V ∼ 1 and A ∼ Ncos θdφ. The metric is

g ∼ dr

+ r

(dθ

+sin

θdφ

)+N

[

d(τ/N) + cos θ dφ

]

This is what we have called the ALF metric. For large r it approaches an S

bundle over S

where the radius of S

is ﬁxed.

9.4.1 Belinskii–Gibbons–Page–Pope class

In this section we shall consider hyper-Kähler metrics admitting a tri-

holomorphic action of SU(2). This assumption will reduce the hyper-Kähler

condition to the Euler equations which can be solved explicitly [13].

The hyper-Kähler metrics on M = R × SU(2) with a transitive action of

SU(2) can be put in the form

g = w

dρ

(σ

)

(σ

)

(σ

)

, (9.4.41)

where w

, and w

are functions of ρ, and σ

are left-invariant one-forms on

SU(2) which satisfy (9.1.2). The ansatz (9.4.41) is a general one. Given a four-

dimensional metric which is diagonal in the left-invariant basis one can always

deﬁne a coordinate ρ such that g is of the form (9.4.41) for some functions

. Moreover the diagonalisability in the left-invariant basis can always be

9.4 Multi-centred gravitational instantons 211

achieved if g is Ricci-ﬂat: one ﬁrst diagonalizes the metric on a surface ρ =

const and then shows that the Ricci-ﬂat condition prohibits the off-diagonal

terms. See the discussion in [160].

The SD two-forms are



= w

∧ σ

+ w

∧ dρ,



= w

∧ σ

+ w

∧ dρ, and



= w

∧ σ

+ w

∧ dρ. (9.4.42)

We assume that the SU(2) action ﬁxes all complex structures, so the invariant

frame is covariantly constant. Thus the closure condition (9.3.30) holds for

ASD Ricci-ﬂat metrics in this frame. It is equivalent to the Euler equations

˙w

= w

, ˙w

= w

, and ˙w

= w

(9.4.43)

which are integrable and admit a Lax pair (8.1.7). These equations readily

integrate to

(˙w

)

=(w

− C

)(w

− C

where C

= w

− w

and C

= w

− w

are constants. The Belinskii–

Gibbons–Page–Pope (BGPP) metric (9.4.41) is ﬂat if C

= C

= 0, and is never

complete if C

− C

) = 0. The remaining cases correspond to the Eguchi–

Hanson solution (9.1.3).

Now choose a one-dimensional subgroup U(1) ⊂ SU(2). Any hyper-Kähler

metric with a tri-holomorphic U(1) action can be put in the Gibbons–Hawking

form (9.4.35). We shall now characterize the harmonic functions V for which

(9.4.35) belongs to the BGPP class (9.4.41). Let g be such a metric and let K

be the corresponding Killing vector which puts g in the Gibbons–Hawking

form. We expand K in a left-invariant basis of SU(2), eliminate the Euler

angles and ρ in favour of (x

,τ) and observe that V

−1

= g(K, K), where g is

given by (9.4.41). The details can be found in [73] and [50], where it is shown

that

V(x

, x



i=1

(β − β

)



−1/2





i=1

(β − β

)



−1

where β is an algebraic root of



i=1

β − β

= C,

and C; β

are constants. Equivalently it can be shown [50] that the Gibbons–

Hawking metric (9.4.35) belongs to the BGPP class (9.4.41) iff V = r ·∇

V, and

212 9: Gravitational instantons

V is a harmonic function constant on a central quadric, that is,

(

V)x

= const

for some symmetric matrix B

= B

(

V).

Example. Consider the Eguchi–Hanson metric which corresponds to the

harmonic function

V = |r + a|

−1

+ |r − a|

−1

, where a =(0, 0, a).

We verify that r ·∇

V = V, where the harmonic function

V = −

arccoth



|r + a| + |r − a|



is constant on the ellipsoid

+ x

[coth (a

V/2)]

− 1

[coth (a

V/2)]

=1.

9.5 Other gravitational instantons

The positive action theorem [183] states that Ricci-ﬂat manifolds (M, g) which

have the topology of R

at inﬁnity and approach the ﬂat Euclidean metric

µν

, where η = diag(1, 1, 1, 1)

sufﬁciently fast, in the sense that

µν

= η

µν

+ O(r

−4

), (∂

)

νλ

)=O(r

−4−p

), and r

= η

µν

, (9.5.44)

have to be ﬂat. A weaker asymptotic condition one can impose on g is asymp-

totically locally Euclidean as in Deﬁnition 9.1.1. Globally the neighbourhood

of inﬁnity must look like S

/ × R, where  ⊂ SO(4) is a ﬁnite group of

isometries acting freely on S

(a Kleinian group).

In the following we shall use the isomorphism (9.2.14) and consider  as

a ﬁnite subgroup of SU(2). Finite subgroups of  ⊂ SU(2) correspond to

Platonic solids in R

. They are the cyclic groups, and the binary dihedral,

tetrahedral, octahedral, and icosahedral groups (one can think about the last

three as Möbius transformations of S

= CP

which leave the points cor-

responding to vertices of a given Platonic solid ﬁxed). All Kleinian groups

act on C

, and the ‘inﬁnity’ S

⊂ C

. Let (z

, z

) ∈ C

. For each  there

exist three invariants x, y, and z which are polynomials in (z

, z

) invari-

ant under . These invariants satisfy some algebraic relations which we list

9.5 Other gravitational instantons 213

below:

Group Relation F



(x, y, z)=0

Cyclic xy − z

Dihedral x

+ y

z + z

Tetrahedral x

+ y

+ z

Octahedral x

+ y

+ yz

Icosahedral x

+ y

+ z

In each case

/ ⊂ C

= {(x, y, z) ∈ C

, F



(x, y, z)=0}.

The manifold M on which an ALE metric is deﬁned is obtained by mini-

mally resolving the singularity at the origin of C

/. This desingularization

is achieved by taking M to be the zero set of





(x, y, z)=F



(x, y, z)+



i=1

(x, y, z),

where f

span the ring of polynomials in (x, y, z) which do not vanish when

∂



= ∂



= ∂



= 0. The dimension r of this ring is equal to the number of

non-trivial conjugacy classes of  which is k − 1, k +1, 6, 7, and 8, respectively

[22]. Kronheimer [99, 100] proved that for each  a unique hyper-Kähler

metric exists on a minimal resolution M, and that this metric is precisely the

ALE metric with R

/ as its inﬁnity.

Example. Consider the cyclic group  of matrices of order two generated by





and



−10

0 −1



This subgroup of SU(2) acts linearly on C

.If(z

, z

) ∈ C

then the mono-

mials

x =(z

)

, y =(z

)

, and z = z

are invariant under . These monomials satisfy the algebraic relation

xy = z

which gives the function F



(x, y, z) in this case. The quotient C

/ is singu-

lar, but this singularity can be resolved by adding lower order terms

xy =(z − p

)(z − p

)

which gives





(x, y, z). The non-singular zero set of





in C

is the Eguchi–

Hanson manifold.

214 9: Gravitational instantons

An example of an ALF gravitational instanton (see Deﬁnition 9.1.2) is the

Atiyah–Hitchin metric [11]. Consider the metric of the form (9.4.41). The

action of SU(2) lifts to the bundle of SD two-forms 

. If the action is

trivial, so that all SD two-forms are Lie-derived along the Killing vector ﬁelds

generating the action, the ASD Ricci-ﬂat equations reduce to the Euler system

(9.4.43). Here we assume that the action is non-trivial and instead rotates

the SD two-forms. This means that the two-forms 

given by (9.4.42) are

not in the covariantly constant spin-frame, and the connection one-form





does not vanish. Thus instead of the closure condition one has d



2



∧ 



)



= 0 or, equivalently, d

= α

∧ 

where α

= −α

are one-

forms constructed out of 



. The equations resulting from the ASD vacuum

condition R



=0are

˙w

= w

− w

+ w

˙w

= w

− w

+ w

), and (9.5.45)

˙w

= w

− w

+ w

Following [11] this system can be solved in fairly closed-form using elliptic

functions. Redeﬁning the coordinate ρ one has

g =

dρ

(σ

)

(σ

)

(σ

)

where

= −W

dρ

−

cosec(ρ),

= −W

dρ

cot (ρ), and

= −W

dρ

cosec(ρ),

and W satisﬁes the ODE

dρ

Wcosec

(ρ)=0.

The solution corresponding to the complete metric is

W =



sin ρ K



sin

(ρ/2)



, where K(k)=



π/2

dφ



1 − ksin

The importance of the Atiyah–Hitchin metric is that it arises as a natural metric

on the moduli space of charge-two non-abelian magnetic SU(2) monopole and

its geodesics describe low-energy monopole scattering [11].

9.5 Other gravitational instantons 215

9.5.1 Compact gravitational instantons and K 3

Compact solutions to Einstein equations with non-zero cosmological constant

are relatively easy to ﬁnd: the conformally ﬂat metric on S

and the Fubini–

Study metric on CP

are two examples. The ﬁrst one arises as the conformal

rescaling of the ﬂat metric on R

. The metric on S

with radius r

g =

1+(ρ/r

)



dρ



+ σ





is conformally ﬂat, and so obviously ASD. The second one is the standard

Kähler metric on the complex projective space. It is given by the expression

(9.3.31) with the Kähler potential

 = log (1 + |z|

+ |w|

where (z,w) are holomorphic coordinates on CP

deﬁned by

w =

and z =

in the neighbourhood of Z

= 0 in terms of the homogeneous coordinates

, Z

). Notice that  does not satisfy the Monge–Ampere equation

(9.3.34) as the metric is not Ricci-ﬂat. This is the one example when one needs

to be careful about the choice of orientation. The Kähler metric on CP

have just constructed is SD (rather that ASD) with respect to the orientation

picked by the SD Kähler two-form. Setting

z = r cos





exp



(ψ + φ)



and w = r sin





exp



(ψ − φ)



yields

g =

(1 + r

)

(1 + r

)

1+r

(σ

+ σ

The apparent singularity at r = 0 is a NUT singularity resulting from using

spherical polars. To analyse the behaviour of the metric when r →∞set r =

−1

. Fixing (θ,φ) gives

g ∼ du

dψ

near u = 0. Thus u = 0 is a bolt singularity which can be removed if 0 ≤ ψ ≤

4π.

In view of these two simple compact gravitational instantons it may there-

fore seem natural to look for compact Ricci-ﬂat gravitational instantons.

The ﬂat four-torus T

= S

× S

is a trivial example, but no other

examples are explicitly known. The point is that Ricci-ﬂat metrics on compact