Dunajski M. Solitons, Instantons, and Twistors

Подождите немного. Документ загружается.

216 9: Gravitational instantons

spaces cannot admit Killing vectors, except the trivial cases with S

factors. To

see this consider a Killing vector K

. The Killing equations ∇

= 0 imply

the identity

∇

= R

bca

Contracting this identity on the (ab) indices and contracting the resulting

formula with K

yields

− K

K

=0.

Integrating this by parts over a compact manifold M gives



+ |∇

)

√

x =0.

If the metric on g is Ricci-ﬂat R

= 0 then we necessarily have ∇

=0,as

in the Riemannian case there are no non-zero tensors with zero norm. This

condition can only hold if the components of the Riemann tensor vanish along

. If that is the case then g is of the form dτ

+ g

, where K = ∂/∂τ and

is a Ricci-ﬂat metric on R

. Thus g

is necessarily ﬂat, and so is the four-

dimensional metric.

A Ricci-ﬂat metric on a simply connected compact manifold is known to

exist on the so-called K3 surface.

The Riemann tensor of this metric is ASD

and the metric is Kähler and does not admit any Killing vectors. The existence

of this metric follows from Yau’s proof [189] of the Calabi conjecture, but ﬁnd-

ing the explicit expression for the metric is one of the biggest outstanding open

problems in Riemannian geometry and the theory of gravitational instantons.

9.6 Einstein–Maxwell gravitational instantons

In this section we shall discuss the Einstein–Maxwell theory which admits

regular multi-centred instantons where the geometry is not determined by

ﬁxing the asymptotics – there exists non-ﬂat, but asymptotically ﬂat solutions.

These solutions have been studied in [53, 190, 182]. Our presentation follows

[53].

The Einstein–Maxwell gravitational instantons on a four-dimensional man-

ifold M are solutions to the Einstein–Maxwell equations with Riemannian

Named after three geometers: Kummer, Kähler, and Kodaira. The metric on K3 has so far

remained even more elusive than the notoriously difﬁcult mountain K2 in the Karakorum range

of the Himalayas.

9.6 Einstein–Maxwell gravitational instantons 217

signature

−

= T

, (9.6.46)

where the Einstein–Maxwell energy–momentum tensor is given by

=2F

−

and the Maxwell equations

∇

hold for the closed two-form F

. The metric is given by

g =



(dτ + ω)

+ U



Udx

, (9.6.47)

where the functions U,



U and the one-form ω depend on x =(x

, x

) and

satisfy

∇

U = ∇



U = 0 and

∇×ω =



U∇U − U∇



U. (9.6.48)

We will work with the orthonormal tetrad



1/2

(dτ + ω) and e

=(U



1/2

. (9.6.49)

With respect to this tetrad the electromagnetic ﬁeld strength may now be

written as

∂

−1

−



−1

and

ijk

∂

−1



−1

, (9.6.50)

where the derivatives are partial derivatives with respect to the corresponding

space-time indices. One can check that this ﬁeld strength satisﬁes the Bianchi

identities, and thus locally at least we can write F = dA. The metrics with

U =



U have purely magnetic ﬁeld strength F = −2∗

dU and are the Rieman-

nian analogues of the Majumdar–Papapetrou solutions [152] of the Einstein–

Maxwell equations.

The solutions (9.6.47) were ﬁrst found in the Lorentzian regime by Israel

and Wilson [89] and by Perjés [133] as a stationary generalization of the static

Majumdar–Papapetrou multi-black-hole solutions. However, it was shown by

Hartle and Hawking that all the non-static solutions suffered from naked

singularities [33, 76]. With Riemannian signature however, regular solutions

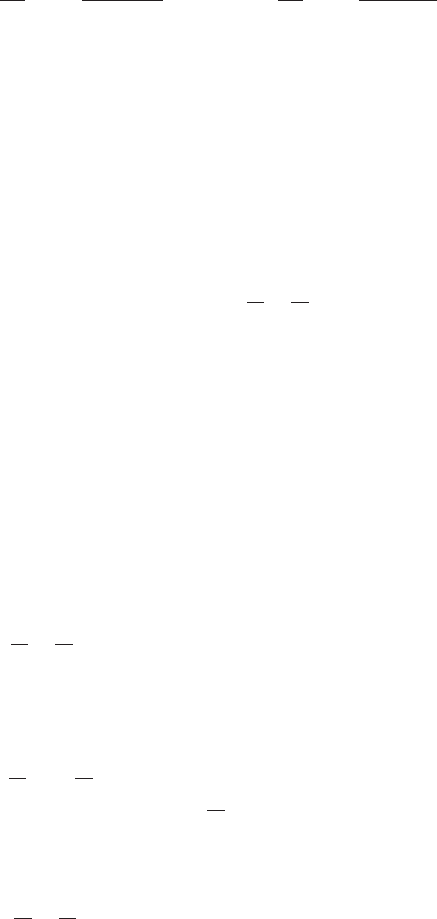
218 9: Gravitational instantons

exist [190, 182]. We can take

U =

4π



m=1

| x − x

and



U =

4π





n=1

| x −

, (9.6.51)

in these expressions β,

β,a

, x

, N, and



N are constants. For the sig-

nature to remain positive throughout we can require U,



U > 0 which in turn

requires a

> 0.

If there is at least one non-coincident centre, x

=

, regularity requires

that τ is identiﬁed with period 4π and that the constants satisfy the following

constraints at all the non-coincident centres

)

= 1 and



U(x

=1, ∀m, n. (9.6.52)

Given the locations of the centres {x

}, these constraints may be solved

uniquely for the {a

} [190]. When

4π

= 0 the solution is only unique

up to the overall scaling

U → e

U and



U → e

−s



U. (9.6.53)

In general this scaling leaves the metric invariant and induces a linear duality

transformation on the Maxwell ﬁeld mapping solutions to solutions:

E → cosh s E + sinh s B and B → sinh s E + cosh s B. (9.6.54)

The rescaling does not leave the action and other properties of the solutions

invariant.

The constants β and

β determine the asymptotics of the solution. There are

three possibilities:

The case

4π

= 0 gives an ALF metric, tending to an S

bundle over S

at inﬁnity, with ﬁrst Chern number N −



N. Without loss of generality we

have rescaled the harmonic functions using (9.6.53) so that β =

β. Equations

(9.6.52) now imply that



− N =



−



N.IfN =



N the asymptotic

bundle is trivial and we obtain asymptotically ﬂat (∼ R

× S

) solutions.

The case

4π

=0,

4π

= 1 gives an ALE metric, tending to R

|N−



. We have

used the rescaling (9.6.53) to set

4π

= 1 without loss of generality. In this case

the constraints (9.6.52) require that



= N −



N. Of course we can reverse

the roles of β and

β.IfN =



N + 1 the solution is asymptotically Euclidean

(∼ R

The case

4π

= 0 leads to an asymptotically locally Robinson–Bertotti

metric, tending to Ad S

× S

or Ad S

/Z × S

The former case only arises if all of the centres are coincident, so that

U =



U, and τ need not be made periodic. For both these asymptotics, the

9.6 Einstein–Maxwell gravitational instantons 219

constraints (9.6.52) require that N =



N. We may further use the rescaling

(9.6.53) to set



As Riemannian solutions, the metrics are naturally thought of as general-

izations of the Gibbons–Hawking multi-centre metrics (9.4.35) which in fact

they include as the special case



U = 1, albeit with an additional ASD Maxwell

ﬁeld. A crucial new aspect of the ALE and ALF Israel–Wilson–Perjés solutions

is that when

N =



N ± 1 (for ALE) or N =



N (for ALF),

the ﬁbration of the τ circle over S

at inﬁnity is trivial and the metrics do

not require the Z

identiﬁcations at inﬁnity that are needed in the Gibbons–

Hawking case. The space-times are therefore strictly asymptotically Euclidean

and asymptotically ﬂat, respectively, in these cases.

We shall now use the arguments of Tod given in the Lorentzian setting

[157] and show that the solution (9.6.47) and (9.6.50) with the harmonic

functions described by (9.6.51) and satisfying the constraints (9.6.52) is the

most general regular Einstein–Maxwell instanton with a ‘charged’ covariantly

constant spinor.

Theorem 9.6.1 [53] Let (g, A) be a regular solution to the Riemannian

Einstein–Maxwell equations such that there exist spinors (α

,β



) (which do

not vanish identically) satisfying

∇



− i

√

2φ



= 0 and ∇



+ i

√



=0, (9.6.55)

where the spinors φ and

φ are symmetric in their respective indices and give

the ASD and SD parts of the electromagnetic ﬁeld

= φ



. (9.6.56)

Then the metric g admits a Killing vector and (g, A) can be put in the form

(9.6.47) where U and



U are harmonic functions of the form (9.6.51) and ω is

a one-form which satisﬁes (9.6.48).

Proof We shall use the conjugation properties of Euclidean spinors (9.2.17)

to deﬁne

U =(α

ˆα

)

−1

and



U =(β



)

−1

. (9.6.57)

In the positive-deﬁnite case U and



U are bounded unless α or β have zeros.

In the Lorentzian case their possible vanishing leads to plane wave space-times

[157]. Now deﬁne a (complex) null tetrad

= α



, X

=ˆα



, Y

= α



, and Y

= −ˆα



(9.6.58)

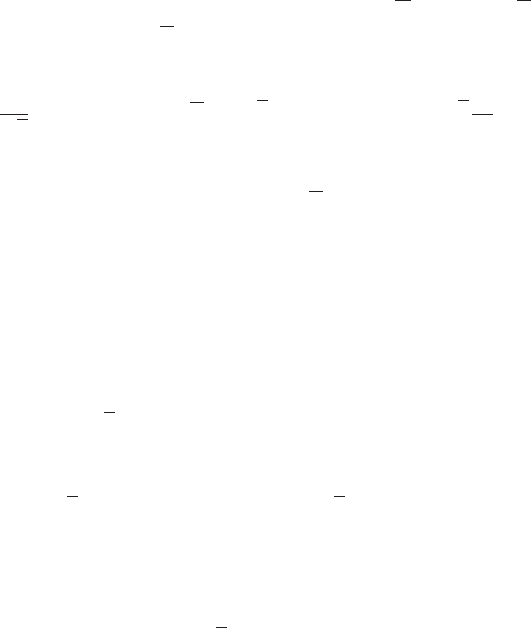
220 9: Gravitational instantons

We can check that ( ˆα



) is also a solution to the Killing spinor equation

(9.6.55) (note that

= −φ

so that F is real when expanded in a complex

null tetrad). It therefore follows from (9.6.55) that X

, X

, and Y

− Y

are

gradients and that K

= Y

+ Y

is a Killing vector. Deﬁne local coordinates

,τ)=(x, y, z,τ)by

X =

√

(dx + idy), (Y −

Y)=i

√

2dz, and K

∇

√

∂

∂τ

(9.6.59)

where X = X

= X



and similarly for Y, Y. The vector K Lie derives the

spinors (α

,β



), implying that U and



U are independent of τ .

The metric is now given by g = ε



. This expression may be

evaluated by noting that from (9.6.57) we have ε

= U(α

ˆα

− α

ˆα

) and

similarly for ε



. Using the fact that from the above deﬁnitions K

2(U



−1

, we ﬁnd that the metric takes the form (9.6.47) for some one form ω.

The next step is to ﬁnd ω .

The deﬁnitions of U,



U, and K together with (9.6.55) imply

∇

= i

√



−1



+ U

−1



, (9.6.60)

and

∇

−1

= i

√

2φ



and ∇



−1

= −i

√



. (9.6.61)

The formulae in (9.6.61) may be inverted to ﬁnd expressions for φ

and



using



Substituting the result into (9.6.60) yields the expression (9.6.48) for ∇×ω.

Finally, differentiating the relations (9.6.55) shows that the energy–

momentum tensor is that of Einstein–Maxwell theory: T

=2φ



.The

Maxwell equations

∇



= 0 and ∇



= 0 (9.6.62)

now imply that U and



U are harmonic on R

. This completes the local

reconstruction of the solution from the Killing spinors.

So far everything has proceeded as in [157] with minor differences in signs

and the reality conditions. The main difference arises in global regularity

considerations which lead us to consider the invariant

| = |2(φ



= |∇U

−1

+ |∇



−1

, (9.6.63)

9.7 Kaluza–Klein monopoles 221

where the norm of the gradients is taken with respect to the ﬂat metric on

, and we have used (9.6.61). Regularity requires this invariant be bounded.

Therefore both |∇U

−1

| and |∇



−1

| must be bounded. The various boundary

conditions we have described imply that U and



U are regular as |x|→∞.In

particular, they are both regular outside a ball B

of sufﬁciently large radius

R in R

The coordinates {x,τ} cover R × (R

\ S), where S is the compact subset

of B

on which U or



U blow up. A theorem from [32] can now be applied

separately to both harmonic functions to prove that S consists of a ﬁnite

number of points. In fact

#S < max{|∇U

−1

|, |∇



−1

|}|U( p)+



U(p)| R +1, (9.6.64)

where p is any point in B

which does not belong to S. This combined with

the maximum principle shows that (9.6.51) are the most general harmonic

functions leading to regular metrics. It also follows from (9.6.57) and the

positivity of the spinor inner product that a

and

in (9.6.51) are all

non-negative. 

9.7 Kaluza–Klein monopoles

In the gauge-theoretic context, instantons in D dimensions can be interpreted

as solitons in (D + 1) dimensions. This remains true in the context of gravity.

The point is that if g is a Riemannian Ricci-ﬂat metric in D dimensions

then the product metric −dt

+ g is a static Lorentizian Ricci-ﬂat metric in

(D + 1) dimensions. To construct gravitational solitons – static, non-singular

solutions – to Lorentzian Einstein equations one of course has to worry about

the asymptotic behaviour. The simplest example of such soliton is the ﬁve-

dimensional Kaluza–Klein monopole

of Gross–Perry [74] and Sorkin [148].

In its original version the Kaluza–Klein theory is ﬁve-dimensional general

relativity such that the ﬁfth, space-like, dimension is compactiﬁed on a circle.

The Kaluza–Klein monopole is given by

(5)

= −dt

+ V(dx

+ dx

)+V

−1

(dτ + A)

(9.7.65)

where we have taken the gravitational instanton in four dimensions to be the

multi-Taub-NUT gravitational solution (9.4.40).

From the (3+1)-dimensional perspective the solutions (9.7.65) give rise to

solutions of Einstein–Maxwell theory with a dilaton. This is the standard

The existence of solitons in (3+1)-dimensions is ruled out by the Birkhoff theorem which

states that every non-ﬂat static solutions to vacuum Einstein equations is diffeomorphic to the

Schwarzchild black-hole.

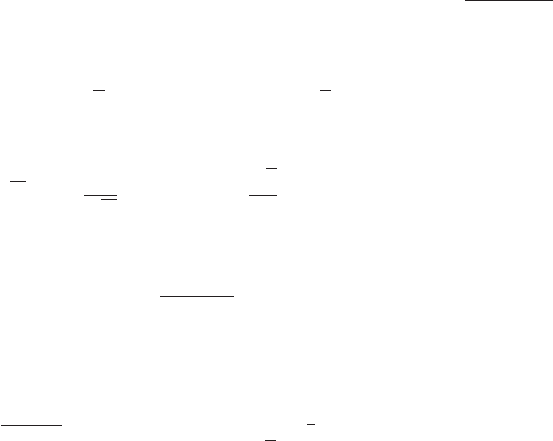
222 9: Gravitational instantons

Kaluza–Klein reduction where the ﬁfth dimension compactiﬁes to a circle

of small radius R. This corresponds to τ in (9.4.35) being periodic. If the

radius is sufﬁciently small then low-energy experiments will average over the

ﬁfth dimension thus leading to an effective four-dimensional theory with the

Maxwell potential and a scalar ﬁeld.

We perform the Kaluza–Klein reduction with respect to the space-like Killing

vector ∂/∂τ. The four-dimensional theory is invariant under the general coordi-

nate transformations independent of τ . The translation of the ﬁbre coordinate

τ → τ + (x

) where x

=(x, t) induces the U(1) gauge transformations of the

Maxwell one-form. The scaling symmetry

τ −→ cτ, [g

(5)

]

µτ

−→ c

−2

(5)

]

µτ

is spontaneously broken by the Kaluza–Klein vacuum, since τ is a coordinate

on a circle with a ﬁxed radius. The scalar ﬁeld corresponding to this symmetry

breaking is called the dilaton.

It is the usual practice to conformally rescale the resulting (3 + 1)-

dimensional metric, and the dilaton so that the multiple of the Ricci scalar of

the Lorentzian metric G

µν

in the reduced Lagrangian is equal to



|det(G

µν

)|.

The corresponding Maxwell ﬁeld is F = dA, and the physical metric G

µν

(3 + 1) signature is given by

(5)

= exp (−2φ/

√

3)G

µν

+ exp (4φ/

√

3)(dτ + A)

, (9.7.66)

where the triple

G =

√

Vdx

−

√

,φ= −

√

log V, and F = ∗

satisﬁes the Einstein–Maxwell dilaton equations. These equations arise from

the Einstein–Hilbert Lagrangian in ﬁve dimensions:





|det g

(5)

dτ d

where R

(5)

is the Ricci scalar of g

(5)

. Substituting the anzatz (9.7.66) into this

Lagrangian yields the four-dimensional Lagrangian

2πR





|det G|



R − 2G

µν

∇

φ ∇

φ −

√

3φ

µν



x, (9.7.67)

where R is the radius of the Kaluza–Klein circle, F = dA, and R is the Ricci

scalar of G.

9.7.1 Kaluza–Klein solitons from Einstein–Maxwell instantons

The details of the Kaluza–Klein lift are more complicated in the case of

Einstein–Maxwell gravitational instantons (9.6.47). The Einstein–Maxwell

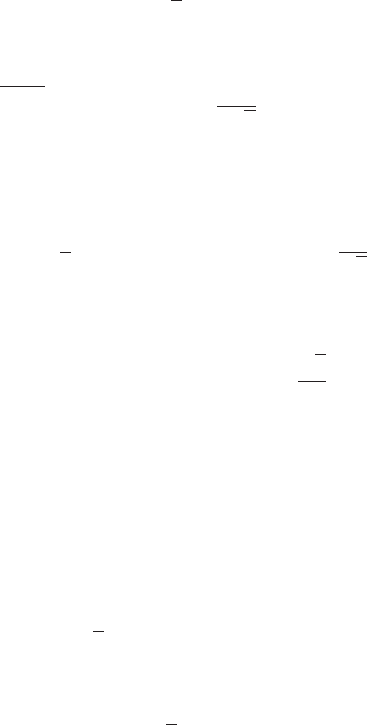
9.7 Kaluza–Klein monopoles 223

theory without a dilaton cannot be consistently lifted to pure gravity in

ﬁve dimensions. However, Einstein–Maxwell conﬁgurations may be lifted to

solutions of ﬁve-dimensional Einstein–Maxwell theory with a Chern–Simons

term. This lift is the bosonic sector of the lift from N = 2 supergravity in four

dimensions to N = 2 supergravity in ﬁve dimensions [31].

We are interested in lifting the four-dimensional Riemannian theory to a

Lorentzian theory on a ﬁve-dimensional manifold M

. The four-dimensional

action is



√

R − F

, (9.7.68)

with equations of motion given by (9.6.46). The ﬁve-dimensional action is





−g

(5)

− H

αβ

−

√



H ∧ H ∧ W, (9.7.69)

where H = dW is the ﬁve-dimensional Maxwell ﬁeld. In this section we use

Greek indices ranging from 0 to 4 in ﬁve dimensions. The equations of motion

in ﬁve dimensions are

αβ

=2H

βγ

−

(5)

αβ

γδ

and d ∗

H = −

√

H ∧ H. (9.7.70)

Given a solution, g and F = dA, to the four-dimensional equations (9.6.46),

we may lift the solution to ﬁve dimensions as follows:

(5)

= g − (dt + )

and W =

√

A, (9.7.71)

where  is a one-form determined by g and F through

d = ∗

F. (9.7.72)

One may then check that the ﬁve-dimensional conﬁguration (9.7.71) solves

the equations of motion (9.7.70). Note that solutions to (9.7.72) exist because

∗

F = 0 on shell. In the cases (9.6.47) and (9.6.50) we may solve for 

explicitly to ﬁnd

 = −

−1



−1

(dτ + ω)+χ, (9.7.73)

where χ satisﬁes

∇×χ =

∇

U −



. (9.7.74)

To investigate regularity and causality of the ﬁve-dimensional metrics we

shall analyse the behaviour near the centres where U →∞or



U →∞.Inthe

four-dimensional Riemannian Israel–Wilson–Perjés solutions these can always

be made to be regular points [190, 182] as we discussed in Section 9.6. We shall

224 9: Gravitational instantons

follow [53] and re-examine the regularity of the metric around these points and

check for the possible occurrence of closed time-like curves.

Before analysing the behaviour near the centres note the following. Firstly,

that

(5)

ττ

≡ g

(5)



∂

∂τ

∂

∂τ



= −

(U −



(2U



< 0, (9.7.75)

if U =



U. Therefore, to avoid closed time-like curves throughout the ﬁve-

dimensional space-time we must not identify τ . Secondly, possible candidates

for the location of horizons are where the metric becomes degenerate:

0=g

(5)

ττ

− [g

(5)

tτ

]

= −



. (9.7.76)

This occurs at the centres where U or



U diverge.

In order to understand the geometry near the centres, there are three dif-

ferent cases we need to consider separately. The ﬁrst is that U →∞while



remains ﬁnite. Using polar coordinates (r = ρ

/4,θ,φ) centred on the point x

and requiring that a



U(x

) = 1, the metric becomes

(5)

∼ dρ

(dτ + cos θdφ)

+ d

−

(

dt − a

dτ/2

)

(9.7.77)

as ρ → 0, with d

= dθ

+sin

θdφ

. The metric may be made regular about

this point if we identify τ with period 4π . Unfortunately this introduces closed

time-like curves as we discussed. If we choose not to identify τ we are left with

time-like naked singularities at the centres. We see that there is no horizon

at these points, but rather a (singular) origin of polar coordinates. Therefore,

metrics with this behaviour at the centres cannot lift to causal, regular solitons

in ﬁve dimensions.

The remaining two possibilities involve coincident centres where both U and



U go to inﬁnity, so that x

. One needs to treat separately the cases where

and where a

=

. In the latter case we again ﬁnd regularity at the

expense of closed time-like curves going out to inﬁnity, or alternatively naked

singularities. This leaves only the former case with a

for all m. That is,



U = U + k, with k being some constant.

By considering the asymptotic regime, one can see that in order to obtain

a regular asymptotic geometry without closed time-like curves, one requires

that either both U and



U go to a constant at inﬁnity or they both go to

zero. Rescaling the harmonic functions and performing a duality rotation on

the Maxwell ﬁeld, as we discussed in four dimensions above, implies that

without loss of generality U =



U. Therefore the only lift that leads to a globally

regular and causal ﬁve-dimensional space-time is the case U =



U, which corre-

sponds to the Euclidean Majumdar–Papapetrou metric in four dimensions. The

9.7 Kaluza–Klein monopoles 225

ﬁve-dimensional metric can be written as

(5)

= −(dt − dτ/U)

dτ

+ U

. (9.7.78)

Away from the centres, the space-times approach either R

1,4

or Ad S

× S

with U going to a constant or zero at inﬁnity, respectively.

With a rescaling of coordinates, the geometry near the centres where U →∞

may be written as

(5)

∼ a



+2rdtdτ − dt

+ d



. (9.7.79)

Calculating the curvature shows that this metric locally describes Ad S

× S

The Killing vector ∂/∂t is everywhere regular and time-like. This remains true

in the full space-time (9.7.78). There is no horizon and the degeneration of the

metric at the centres is analogous to the origin of polar coordinates.

The coordinates in (9.7.79) may be mapped to Poincaré coordinates as

follows:

Y =

1/2

cos

X =

−



− 1



tan

, and

T =

−





tan

, (9.7.80)

so that the metric becomes

(5)

−dT

+ dX

+ dY

+ a

d

. (9.7.81)

There is no singularity at t = ±π as may be checked by writing down the

embedding of Ad S

as a quadric in R

2,2

in terms of these coordinates. The map

(9.7.80) is periodic in t. Taking t with inﬁnite range corresponds to passing

to the (causal) universal cover of Ad S

. There is no need to identify τ and

therefore the space-time is causal.

The metrics (9.7.78) give causal, regular solutions to the ﬁve-dimensional

theory with an everywhere deﬁned time-like Killing vector. Writing the metric

in the form (9.7.78) suggests that the space-times should be thought of as

containing N parallel ‘solitonic strings’. The strings have world-volumes in

the t − τ plane. There is a plane-fronted wave [68] carrying momentum along

the ∂/∂τ direction of the string. We call these plane-fronted waves solitonic-

strings to emphasize that the ﬁelds are localized along strings and there are no

horizons. The strings are magnetic sources for the two-form ﬁeld strength

H = −

√

3 ∗

dU . (9.7.82)