Drake G.W.F. (editor) Handbook of Atomic, Molecular, and Optical Physics
Подождите немного. Документ загружается.

846 Part D Scattering Theory
Möller Scattering Operator.
Ω
+
=
ω
+
13
+ω
+
12
−1
+G
+
V
23
,
b
+
13
+b
+
12
+G
+
V
13
b
+
12
+V
12
b
+
13
.
(56.120)
Exact T -Matrix.
T
if
=
ψ
f
V
f
ω
+
13
+ω
+
12
−1
ψ
i
+
ψ
f
V
f
G
+
V
23
,
b
+
13
+b
+
12
ψ
i
+
ψ
f
V
f
G
+
V
13
b
+
12
+V
12
b
+
13
ψ
i
.
(56.121)
The impulse approximation to the exact T-matrix
element (56.121) is obtained by ignoring the second
term involving the commutator of V
23
.
Ψ
i
−→ Ψ
imp
i
=
ω
+
13
+ω
+
12
−1
ψ
i
. (56.122)
Impulse Approximation: Post Form.
T
imp
if
=ψ
f
|V
f
ω
+
13
+ω
+
12
−1
ψ
i
.
(56.123)
The impulse approximation can also be expressed using
incoming-wave boundary conditions by making use of
the prior operators
ω
−
ij
(m)χ
m
=
1 +
1
E
m
− H
0
−V
ij
−i
V
ij
χ
m
,
(56.124a)
ω
−
ij
=
m
ω
−
ij
(m)|χ
m
χ
m
| . (56.124b)
The impulse approximation (56.123) is exact if V
23
is
a constant since the commutator of V
23
vanishes in that
case.
Applications [56.25]
(1) Electron Capture. X
+
+H(i) → X( f ) +H
+
.
T
imp
if
=
ψ
f
V
12
+V
23
ω
+
12
+ω
+
13
−1
ψ
i
.
(56.125)
Wave functions: ψ
i
= e
ik
i
·σ
ϕ
i
(r), ψ
f
= e
ik
f
·ρ
ϕ
f
(x),
χ
m
= (2π)
−3
exp
[
i(K · x+k· ρ)
]
,wheretheϕ
n
are hy-
drogenic wave functions.
If X above is a heavy particle, the V
12
term in
(56.125) may be omitted due to the difference in mass
between the projectile 1 and the bound Rydberg elec-
tron 3. See [56.25] and references therein for details.
With the definitions
z =
4αδ
2
(T −2δ)(T −2αδ)
, T = β
2
+ P
2
,
δ = iβK − p· K ,ν= aZ
1
/K ,
t
1
= K/a+v , N(ν) = e
πν/2
Γ(1 −iν) ,
a =
M
1
M
1
+m
e
, b =
M
2
M
2
+m
e
,
k = ak
2
−(1 −a)k
f
, K =ak
1
−(1−a)k
i
,
t = (K − p)/a , p = ak
f
−k
i
,
β =aZ
1
/n ,
and n is the principal quantum number, the impulse
approximation to the T-matrix becomes, in this case,
T
imp
if
∼
ψ
f
V
23
ω
+
13
ψ
i
(56.126)
=
−1
2π
2
a
3
dK
t
2
N(ν)g
i
(t
1
)F ( f, K, p),
(56.127)
where, for a general final s-state,
F ( f, K, p) =
ϕ
∗
f
(x)e
i p·x
1
F
1
iν, 1;i
Kx
−K · x
dx , (56.128)
and g
i
(t
1
) denotes the Fourier transform of the
initial state. The normalization of the Fourier trans-
form is chosen such that momentum and coordinate
space hydrogenic wave functions are related by
ϕ
n
(r) = (2π)
−3
+
exp(it
1
· r)g
n
(t
1
) dt
1
. For the case
f = 1s,
F (1s, K, p) =−
β
3/2
√
π
∂
∂β
I(ν, 0,β,−K, p)
= 8
√
πβ
3/2
(1 −iν)β
T
2
+
iν(β −iK)
T(T −2δ)
T
T −2δ
iν
(56.129)
evaluated at β = aZ
1
. For the case f = 2s,
F (2s, K, p) =−
β
3/2
√
π
∂
∂β
+β
∂
2
∂β
2
× I
ν, 0,β,−K, p
(56.130)
evaluated at β = aZ
1
/2. For a general final ns-state f,
I(ν,α,β,K, p) =
4
√
π
T
T −2αδ
T −2δ
iν
(56.131)
× (U cosh πν ±iV sinh πν) ,
Part D 56.7
Rydberg Collisions: Binary Encounter, Born and Impulse Approximations 56.7 Impulse Approximation 847
where the complex quantity U +iV is
U +iV = (4z)
iν
Γ
1
2
+iν
Γ(1 +iν)
×
2
F
1
(−iν, −iν;1 −2iν;1/z). (56.132)
(2) Electron Impact Excitation.
e
−
+H(i) → e
−
+H( f ). (56.133)
Neglecting V
12
and exchange yields the approximate
T-matrix element
T
imp
if
∼
ψ
f
V
13
ω
+
13
ψ
i
=
−Z
1
(2πa)
3
dx
dr e
iq·σ
ϕ
∗
f
(r)
1
x
×
dK N(ν)g
i
(t
1
)e
it
1
·r
1
F
1
(iν, 1;iKx −iK · x)
(56.134)
=
−Z
1
(2πa)
3
dK N(ν)g
i
(t
1
)g
∗
f
(t
2
)
× I
ν, 0, 0, −K, −q
,
(56.135)
where
I(ν, 0, 0, −K, −q)
= lim
β→0
4π
β
2
+q
2
β
2
+q
2
β
2
+q
2
+2q· K −2iβK
iν
(56.136)
=
4π
q
2
1 +
2K
q
cos θ
−iν
A(cos θ) , (56.137)
with
A(cos θ) =
1 , cos θ>−q/2K ,
e
−πν
, cos θ<−q/2K ,
(56.138)
and cos θ =
ˆ
K ·
ˆ
q, t
2
= t
1
+bq and q = k
i
−k
f
.
(3) Heavy Particle Excitation [56.26].
H
+
+H(1s) → H
+
+H(2s). (56.139)
T
imp
if
=−
Z
1
2
15/2
b
5
πa
3
q
2
∞
0
dKN(K)K
2
1
−1
d(cos θ)
×
1 +
2K
q
cos θ
−iν
,
A(cos θ) ,
(56.140)
where
,
A(cos θ) =
2π
D
4
$
αD
D−2b
2
α
2
−β
2
3/2
+
8
3b
2
− D
α
2
−β
2
1/2
−
48γ
2
D
2
b
2
γ
2
−δ
2
5/2
+
16D
γD−
(
3γ +4α
)
b
2
γ
2
−δ
2
3/2
+
32
D−3b
2
γ
2
−δ
2
1/2
%
A(cos θ) ,
(56.141)
with A(cos θ) givenby(56.138), and
α = b
2
+v
2
+
K
2
a
2
+
K
aq
q
2
µ
+∆E
cos θ,
(56.142a)
β =
K
aq
sin θ
&
4v
2
q
2
−
q
2
µ
+∆E
2
'
1/2
, (56.142b)
δ = 4β, γ = 4α + D , (56.142c)
D =
4bq
a
(q+2K cos θ) ,
(56.142d)
while ν and N(ν) retain their meaning from (56.127).
(4) Ionization. e
−
+H(i) → e
−
+H
+
+e
−
.
T
imp
if
∼−
4π
q
2
N(ν)g
i
(k−bq)
q
2
q
2
−2q· K
iν
,
(56.143)
where K =a(k−bq−v) and q =k
i
−k
f
and exchange
and V
12
are neglected.
(5) Rydberg Atom Collisions [56.11,27].
A+ B(n) → A+ B(n
) (56.144)
→ A+B
+
+e
−
. (56.145)
Consider a Rydberg collision between a projectile (3)
and a target with an electron (1) bound in a Rydberg
orbital to a core (2) [56.11, 27].
Full T-matrix element:
T
fi
(k
3
, k
3
) (56.146)
=
φ
f
(r
1
)e
ik
3
·r
3
V(r
1
, r
3
)
Ψ
(+)
i
(r
1
, r
3
;k
3
)
,
with primes denoting quantities after the collision, and
where the potential V is
V(r
1
, r
3
) = V
13
(r) +V
3C
(r
3
+ar
1
), r = r
1
−r
3
,
(56.147)
with a = M
1
/(M
1
+M
2
), while the subscript C labels
the core. The impulse approximation to the full, outgoing
Part D 56.7

848 Part D Scattering Theory
wave function Ψ
(+)
i
is written
Ψ
imp
i
= (2π)
3/2
g
i
(k
1
)Φ(k
1
, k
3
;r
1
, r
3
)dk
1
,
(56.148)
g
i
(k
1
) =
1
(2π)
3/2
φ
i
(r
1
)e
−ik
1
·r
1
dr
1
. (56.149)
Impulse approximation:
T
imp
fi
(k
3
, k
3
) =
dk
1
dk
1
g
∗
f
(k
1
)g
i
(k
1
)T
13
(k, k
)
× δ
P−(k
1
−k
1
)
, (56.150)
where T
13
is the exact off-shell T-matrix for 1–3 scat-
tering,
T
13
(k, k
) =
exp(ik
·r)|V
13
(r)|ψ(k, r)
. (56.151)
The delta function in (56.150) ensures linear momentum,
K = k
1
+k
3
= k
1
+k
3
, is conserved in 1–3 collisions,
with
k
1
= k
1
+(k
3
−k
3
) ≡ k
1
+ P , (56.152a)
k
=
M
3
M
1
+M
3
(k
1
+k
3
) −k
3
= k+ P . (56.152b)
Elastic scattering:
T
ii
(k
3
, k
3
) =
g
∗
f
(k
1
)g
i
(k
1
)T
13
(k, k)dk
1
,
(56.153)
where k=(M
3
/M)k
1
+(M
1
/M)k
3
and M = M
1
+M
3
.
Integral cross section: for 3–(1,2) scattering,
σ
if
(k
3
) =
M
AB
M
13
2
k
3
k
3
g
f
(k
1
+ P)
× f
13
(k, k
)g
i
(k
1
)
2
d
ˆ
k
3
, (56.154)
where M
AB
is the reduced mass of the 3–(1,2) sys-
tem, M
13
the reduced mass of 1–3. The 1–3 scattering
amplitude f
13
is given by
f
13
(k, k
) =−
1
4π
2M
13
2
T
13
(k, k
). (56.155)
Six Approximations to (56.150)
(1) Optical Theorem.
σ
tot
(k
3
) =
1
k
3
2M
AB
2
T
ii
(k
3
, k
3
)
=
1
v
3
g
i
(k
1
)
2
v
13
σ
T
13
(v
13
)
dk
1
,
(56.156)
where σ
T
13
is the total cross section for 1–3 scatter-
ing at relative speed v
13
. The resultant cross section
(56.156) is an upper limit and contains no interference
terms.
(2) Plane-Wave Final State.
φ
f
(r
1
) = (2π)
−3/2
exp(iκ
1
·r
1
), (56.157)
g
f
(k
1
) = δ(k
1
−κ
1
), (56.158)
T
fi
(k
3
, k
3
) = g
i
(k
1
)T
13
(k, k
), k
1
= κ
1
− P ,
(56.159)
dσ
if
d
ˆ
k
3
dk
1
=
M
AB
M
13
2
k
3
k
3
g
i
(k
1
)
2
f
13
(k, k
)
2
.
(56.160)
(3) Closure.
f
g
f
(k
1
)g
∗
f
(k
1
) = δ(k
1
−k
1
), (56.161)
dσ
T
i
d
ˆ
k
3
=
¯
k
3
k
3
M
AB
M
13
2
g
i
(k
1
)
2
f
13
(k, k
)
2
dk
1
,
(56.162)
where k
1
= (M
3
/M)(k
1
+k
3
) −k
3
.
Conditions for validity of (56.162): (a) k
3
is high
enough to excite all atomic bound and continuum
states, and (b) k
2
3
= (k
2
3
−2ε
fi
/M
AB
) can be approxi-
mated by k
3
, or by an averaged wavenumber
¯
k
3
= (k
2
3
−
2
¯
ε
fi
/M
AB
)
1/2
, where the averaged excitation energy is
¯
ε
fi
= lnε
fi
=
j
f
ij
ln ε
ij
j
f
ij
−1
, (56.163)
and the f
ij
are the oscillator strengths.
(4) Peaking Approximation.
T
peak
fi
(k
3
, k
3
) = F
fi
(P)T
13
(k, k
), (56.164)
where F
fi
is the inelastic form factor
F
fi
(P) =
g
∗
f
(k
1
+ P)g
i
(k
1
)dk
1
(56.165)
=ψ
f
(r) exp(iP·r)|ψ
i
(r) . (56.166)
(5) T
13
= T
13
(P).
T
fi
(k
3
, k
3
) = T
13
(P)F
fi
(P). (56.167)
Part D 56.7
Rydberg Collisions: Binary Encounter, Born and Impulse Approximations 56.7 Impulse Approximation 849
(6) T
13
= constant. f
13
≡ a
s
= constant scattering
length.
σ
if
(k
3
) =
2πa
2
s
k
2
3
M
AB
M
13
2
k
3
+k
3
k
3
−k
3
F
fi
(P)
2
PdP ,
(56.168)
σ
tot
(k
3
) =
4πa
2
s
,v
3
v
1
v
1
4πa
2
s
/v
3
,v
3
v
1
.
(56.169)
Validity Criteria
(A) Intuitive Formulation [56.27]. (i) Particle 3 scatters
separately from 1 and 2, i. e., r
12
A
1,2
; the relative
separation of (1,2) the scattering lengths of 1 and 2.
(ii)
λ
13
r
12
, i. e., the reduced wavelength for 1–3 rel-
ative motion r
12
. Interference effects of 1 and 2 can
be ignored and 1, 2 treated as independent scattering
centers.
(iii) 2–3 collisions do not contribute to inelastic 1–3
scattering.
(iv) Momentum impulsively transferred to 1 during col-
lision (time τ
coll
) with 3 momentum transferred to 1
due to V
12
,i.e.,
P ψ
n
|−∇V
12
|ψ
n
τ
coll
. (56.170)
For a precise formulation of validity criteria based
upon the two-potential formula see the Appendix
of [56.27].
Two classes of interaction in A–B(n) Rydberg col-
lisions justify use of the impulse approximation for the
T-matrix for 1–3 collisions: (i) quasiclassical binding
with V
core
= const., and (ii) weak binding with
E
3
∆E
c
∼ψ
n
(r)|V
1C
(r)|ψ
n
(r) , (56.171a)
ψ
n
(r
1
)|−
2
2M
12
∇
2
1
|ψ
n
(r
1
)∼|ε
n
| , (56.171b)
where E
3
is the kinetic energy of relative motion of 3,
and ∆E
c
is the energy shift in the core. The fractional
error is [56.28]
f
13
λ
∆E
c
E
3
+τ
delay
1 ,
(56.172)
whereλ ∼ k
−1
3
is the reduced wavelength of 3, f
13
is the
scattering amplitude for 1–3 collisions and τ
delay
is the
time delay associated with 1–3 collisions.
Special Case: for nonresonant scattering with
τ
delay
= 0
f
13
λ
|ε
n
|
E
3
1 , (56.173)
which follows from (56.172) upon identifying the shift
in the core energy ∆E
c
with the binding energy |ε|.
Condition (56.173) is less restrictive than (56.171a)
or (56.171b)since f
13
can be either less than or greater
than
λ.
56.7.2 Classical Impulse Approximation
(A) Ionization. For electron impact on heavy par-
ticles [56.29], the cross section for ionization of
a particle moving with velocity t by a projectile with
velocity s is
Q(s, t) =
1
u
2
2s
m
2
s
2
1
dz
z
2
A(z)
z
+ B(z)
,
(56.174)
where
A(z) =
1
2st
3
1
3
x
3/2
02
−x
3/2
01
−2
s
2
+t
2
x
1/2
02
−x
1/2
01
−
s
2
−t
2
2
2ts
3
x
−1/2
02
−x
−1/2
01
,
(56.175a)
B(z) =
1
2m
2
st
3
(m
1
+m
2
)
s
2
−t
2
x
−1/2
02
−x
−1/2
01
−(m
2
−m
1
)
x
1/2
02
−x
1/2
01
.
(56.175b)
For electron impact, (56.175b) is evaluated at
m
1
= 1. The remaining terms above are given by
s
2
= v
2
2
/v
2
0
, t
2
= v
2
1
/v
2
0
= E
1
/u ,
E
2
= m
2
v
2
2
,
u = v
2
0
= Ionization potential of target ,
x
0i
= (s
2
+t
2
−2st cos θ
i
), i = 1, 2 ,
cos θ
i
=
κ
0
±κ
1
, |κ
0
±κ
1
|≤1
1 ,κ
0
±κ
1
> 1
−1 ,κ
0
±κ
1
< −1
,
κ
0
±κ
1
=−
1
2
1 −
m
1
m
2
z
st
±
-
1 +
z
t
2
1 −
z
s
2
.
Equal Mass Case: (m
1
= m
2
)
Q(s, t) =
4
3s
2
1
u
2
2(s
2
−1)
3/2
t
, 1 ≤ s
2
≤ t
2
+1 ,
4
3s
2
1
u
2
2t
2
+3 −
3
s
2
−t
2
, s
2
≥ t
2
+1 .
(56.176)
Part D 56.7
850 Part D Scattering Theory
Integrating over the speed distribution (Sect. 56.4),
Q(s) =
32
π
1
u
2
∞
0
Q(s, t)t
2
dt
(t
2
+1)
4
, (56.177)
which is then numerically evaluated. For electrons, the
integral can be done analytically with the result
Q(y) =
8
3πy
2
(y+1)
4
×
5y
4
+15y
3
−3y
2
−7y+6
(y−1)
1/2
+
5y
5
+17y
4
+15y
3
−25y
2
+20y
×tan
−1
(y−1)
1/2
−24y
3/2
ln
√
y+
√
y−1
√
y−
√
y−1
,
(56.178)
with y =s
2
.
Thomson’s Result:
Q
T
(y) =
4
y
1
u
2
1 −
1
y
.
(56.179)
(B) Electron Loss Cross Section [56.18].
A(V ) + B(u) → A
+
+ e
−
+ B( f ), (56.180)
where B( f ) denotes that the target B is left in any state
(either bound or free) after the collision with the pro-
jectile A. The initial velocity of the projectile is V
while the velocity of the Rydberg electron relative to
thecoreisu, and the ionization potential of the target B
is I.
σ
loss
=
1
3πν
2
∞
τ/4ν
dx σ
T
(x
¯
u)
$
8νx −1 −(ν −x)
2
−2τ
1 +(ν −x)
2
3
+
1
1 +(ν +x)
2
−τ
2
%
,
(56.181)
where ν = V/
¯
u, τ = I/
1
2
m
e
¯
u
2
,
¯
u =
√
2I/m
e
,andσ
T
is
the total electron scattering cross section at speed x
¯
u.
The cross section (56.181) is valid only for particles
being stripped (or lost from the projectile) which are
not strongly bound. See [56.18, 30, 31] for details and
numerous results.
(C) Capture Cross Section from Shell i [56.18].
σ
i
capture
(V)
=
2
5/2
N
i
π
3V
7
r
i
0
dr
C(+1)
C(−1)
d(cos η
)
[
P
i
(r)
]
2
×
1 +y
2
4ε
2
−
ε
2
−y
2
1 +ε
2
+a
2
−y
2
r
3/2
ε
9/2
1 +a
2
3
y
2
−a
2
,
(56.182)
where C denotes that the integration range
[
−1, +1
]
is
restricted such that the integrand is real and positive and
that |1 −ε| <
y
2
−a
2
. The dimensionless variables a
and y above are defined as
y
2
=
2
m
e
|V(R)|V
2
, a
2
=
2
m
e
I
i
V
2
, (56.183)
and with P
i
(r)/r representing the Hartree–Fock–Slater
radial wave function for shell i, with normalization
r
i
0
[
P
i
(r)
]
2
dr = 1 . (56.184)
The ionization potential and number of electrons in
shell i are denoted above, respectively, by I
i
and N
i
.
(D) Total Capture Cross Section [56.18].
σ
total
capture
(V) =
i
σ
i
capture
. (56.185)
(E) Universal Capture Cross Section [56.18]. A uni-
versal curve independent of projectile mass M and
charge Z is obtained from the above expressions for
the capture cross section by plotting the scaled cross
section
,σ
total
capture
=
E
11/4
M
11/4
Z
7/2
λ
3/4
σ
total
capture
(56.186)
versus the scaled energy
,
E =
m
e
M
E
I
,
(56.187)
where m
e
is the mass of the particle captured, which
is usually taken to be a single electron, and I is the
ionization potential of the target. The term λ in (56.186)
is the coupling constant in the target potential, V(R) =
m
e
λ/R
2
, which the electron being captured experiences
during the collision. See Fig. 11 of [56.18] for details.
Part D 56.7
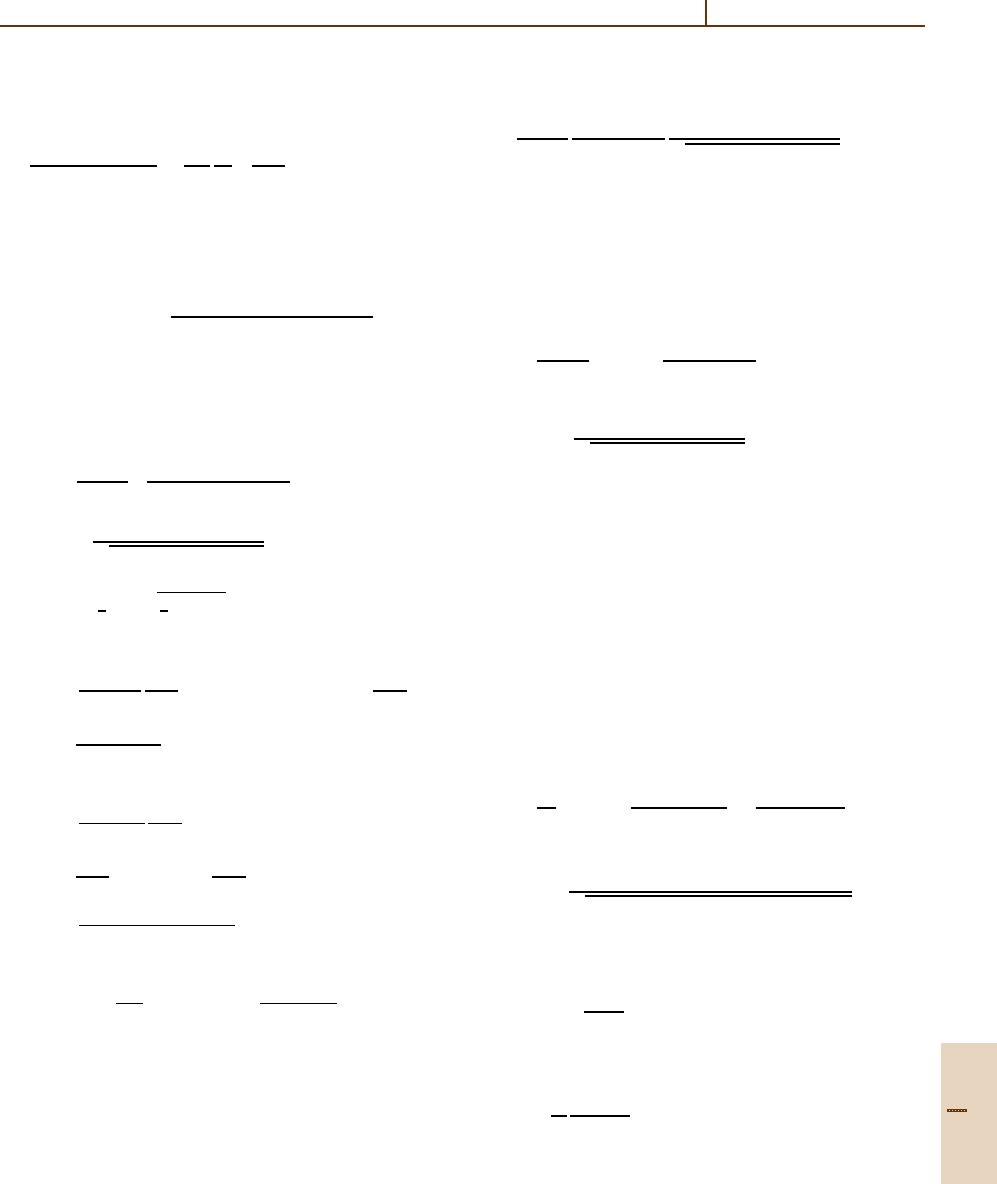
Rydberg Collisions: Binary Encounter, Born and Impulse Approximations 56.7 Impulse Approximation 851
56.7.3 Semiquantal Impulse Approximation
Basic Expression [56.27,32, 33].
dσ
dεdP dk
1
dkdφ
1
=
k
2
1
J
55
k
3
k
3
M
3
M
13
2
g
i
(k
1
)
2
×
f
13
(k, k
)
2
. (56.188)
J
55
is the 5-dimensional Jacobian of the transformation
(P,ε,k
1
, k,φ
1
) →
ˆ
k
3
, k
1
,
(56.189a)
J
55
=
∂
(
P,ε,k
1
, k,φ
1
)
∂
cos θ
3
φ
3
, k
1
, cos θ
1
,φ
1
.
(56.189b)
Expression for Elemental Cross Section [56.27]. In the
(P,ε,k
1
, k,φ
1
) representation,
dσ =
dεdP
M
2
13
v
2
3
&
|g
i
(k
1
)|
2
k
2
1
dk
1
dφ
1
v
1
'
×
| f
13
(k, k
)|
2
dg
2
g
2
+
−g
2
g
2
−g
2
−
,
(56.190)
where g
2
±
=
1
2
B±
1
4
B
2
−C,and
B = B(ε, P,v
1
;v
3
)
=
a
(1 +a)
2
P
2
M
2
13
+
v
2
1
+v
2
1
+v
2
3
+v
2
3
+
2∆
3
M
13
−
4ε(ε +∆
3
)
P
2
,
C = C(ε, P,v
1
;v
3
)
=
v
2
1
+av
2
3
1 +a
P
2
M
2
13
+
v
2
1
−v
2
3
v
2
1
−v
2
3
+
2∆
3
M
13
v
2
1
+v
2
3
+
4∆
3
P
2
v
2
1
(ε +∆
3
) −εv
2
3
,
a =
M
2
M
3
M
1
(M
1
+M
2
+M
3
)
,
,
M
1
= M
1
(1 +M
1
/M
2
),
v
2
1
= v
2
1
+
2ε
,
M
1
,v
2
3
= v
2
3
−
2(ε +∆
3
)
M
AB
,
and ∆
3
is the change in internal energy of particle 3,
while ε denotes the energy change in the target 1 −2.
Hydrogenic Systems g
i
(k
1
) = g
n‘
(k
1
)Y
‘m
(θ
1
‚φ
1
). The
g
n
are the hydrogenic wave functions in momentum
space. See Chapt. 9 for details on hydrogenic wave
functions.
Elemental Cross Sections (m-Averaged and φ
1
-
Integrated).
dσ =
dεdP
M
2
13
v
2
3
W
n
(v
1
)dv
1
2v
1
| f
13
(P, g)|
2
dg
2
g
2
+
−g
2
g
2
−g
2
−
,
(56.191)
where the speed distribution W
n
is given by (56.57).
Two Representations for 1–3 Scattering Ampli-
tude [56.27].
(i) f
13
= f
13
(P, g) is a function of
momentum transferred and relative speed. Then
σ(v
3
) =
1
M
2
13
v
2
3
ε
2
ε
1
dε
∞
v
10
W
n
(v
1
)dv
1
v
1
P
+
P
−
dP
×
g
+
g
−
| f
13
(P, g)|
2
dg
2
g
2
+
−g
2
g
2
−g
2
−
,
(56.192)
where v
2
10
(ε) = max
[
0,(2ε/M)
]
, and the limits to the
P integral are
P
+
= P
+
(
ε, v
1
;v
3
)
= min
M(v
1
+v
1
), M
AB
(v
3
+v
3
)
,
(56.193a)
P
−
= P
−
(
ε, v
1
;v
3
)
= max
M
v
1
−v
1
, M
AB
v
3
−v
3
,
(56.193b)
and unless P
+
> P
−
,theP integral is zero.
(ii) f
13
= f
13
(g,ψ) is a function of relative speed and
scattering angle. Then
σ(v
3
) =
1
v
2
3
ε
2
ε
1
dε
∞
v
10
W
n
(v
1
) dv
1
v
1
g
+
g
−
g dg
S(v
1
, g;v
3
)
×
ψ
+
ψ
−
| f
13
(g,ψ)|
2
d(cos ψ)
cos ψ
+
−cos ψ
cos ψ −cos ψ
−
,
(56.194)
where
S(v
1
, g;v
3
) =
M
13
1 +a
(1 +a)
v
2
1
+av
2
3
−ag
2
1/2
.
Scattering angle ψ-limits,
cos ψ
±
= cos ψ
±
(ε, v
1
, g;v
3
) (56.195)
=
g
g
1
α
2
+β
2
!
α(α +
˜
ε) ±β
ω
2
α
2
+β
2
−
(
α +
˜
ε
)
2
1/2
,
(56.196)
Part D 56.7
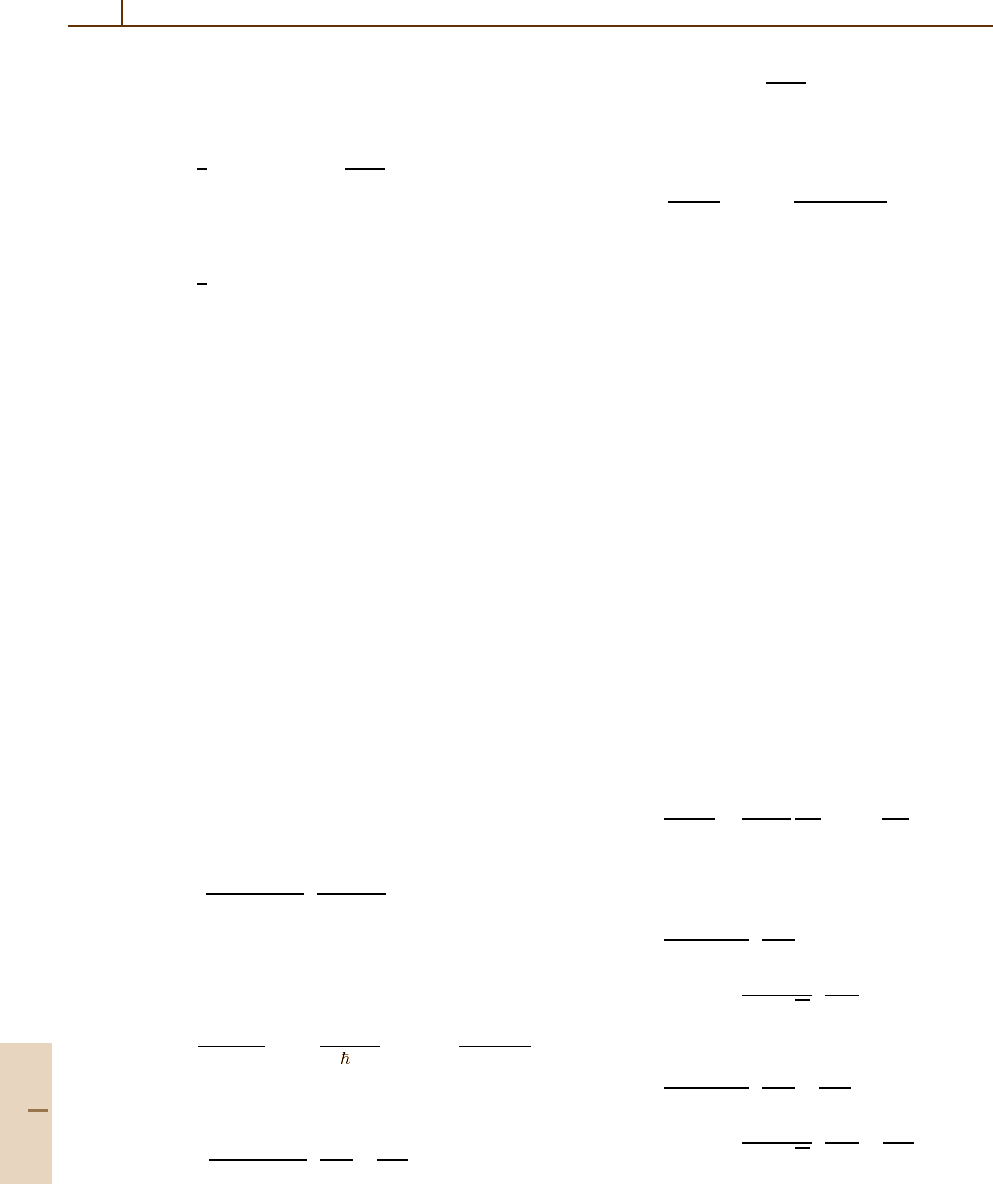
852 Part D Scattering Theory
where
α = α(v
1
, g;v
3
)
=
1
2
M
13
v
2
1
−v
2
3
+
1 −a
1 +a
g
2
,
β =β(v
1
, g;v
3
)
=
1
2
M
13
2v
2
1
+2v
2
3
−g
2
g
2
−
v
2
1
−v
2
3
2
1/2
,
ω = g
/g ,
˜
ε = ε +
a
1 +a
∆
3
.
Special Case: f
13
= f
13
(P).
σ(v
3
) =
π
M
2
13
v
2
3
ε
2
ε
1
dε
∞
v
10
W
n
(v
1
)dv
1
v
1
×
P
+
P
−
| f
13
(P)|
2
dP . (56.197)
56.8 Binary Encounter Approximation
The basic assumption of the binary encounter approx-
imation is that an excitation or ionization process is
caused solely by the interaction of the incoming char ged
or neutral projectile with the Rydberg electron bound to
its parent ion. If, for example, the cross section depends
only on the momentum transfer P to the Rydberg elec-
tron (as in the Born approximation), then the total cross
section is obtained by integrating σ
P
over the momen-
tum distribution of the Rydberg electron. The basic cross
sections required are given in the following section. For
further details see [56.34] and references therein.
56.8.1 Differential Cross Sections
Cross Section per Unit Momentum Transfer
Let the masses, velocities and charges of the particles be
(m
1
, v
1
, Z
1
, e)and(m
2
, v
2
, Z
2
, e), with v =|v
1
−v
2
|
denoting the relative velocity and quantities after the
collision are denoted by primes. Then for distinguishable
particles,
σ
P
=
8πZ
2
1
Z
2
2
e
4
P
v
2
exp(iη
P
)
P
2
2
, (56.198)
where the phase shift η
P
is
η
P
=−2γ ln(P/2µv) +2η
0
+π, (56.199)
and with
µ =
m
1
m
2
m
1
+m
2
,γ=
Z
1
Z
2
e
2
v
, e
2iη
0
=
Γ(1 +iγ)
Γ(1 −iγ)
.
(56.200)
For identical particles,
σ
±
P
=
8πZ
2
1
Z
2
2
e
4
P
v
2
e
iη
P
P
2
±
e
iη
S
S
2
2
, (56.201)
where η
P
isgivenby(56.199)andη
S
is
η
S
=−2γ ln(S/2µv) +2η
0
+π, (56.202)
while η
0
isgivenby(56.200). The momenta P and S
transferred by direct and exchange collisions, respec-
tively, are given by
P = m
1
(v
1
−v
1
) = m
2
(v
1
−v
2
), (56.203a)
S= m
1
(v
1
−v
2
) = m
2
(v
1
−v
2
). (56.203b)
The collision rates (in cm
3
/s) are
ˆ
α
P
= v
1
σ
P
,
ˆ
α
±
P
= v
1
σ
±
P
. (56.204)
Cross Section per Unit Momentum
Transferred per Unit Steradian
Differential relationships:
α
E,P
=
d
2
α
dP dE
=
d
2
α
dPdϕ
dϕ
dE
= α
P,ϕ
dϕ
dE
.
(56.205)
For distinguishable particles,
α
P
= 2πv
1
σ
P,ϕ
= 2πα
P,ϕ
, (56.206a)
α
P,ϕ
=
4Z
2
1
Z
2
2
e
4
P
v
e
iη
P
P
2
2
, (56.206b)
α
E,P
= v
1
σ
E,P
=
8Z
2
1
Z
2
2
e
4
v
1
v
2
√
X
e
iη
P
P
2
2
. (56.206c)
For identical particles,
α
±
P,ϕ
=
4Z
2
1
Z
2
2
e
4
P
v
e
iη
P
P
2
±
e
iη
S
S
2
2
, (56.207a)
α
±
E,P
= v
1
σ
±
E,P
=
8Z
2
1
Z
2
2
e
4
v
1
v
2
√
X
e
iη
P
P
2
±
e
iη
S
S
2
2
,
(56.207b)
Part D 56.8
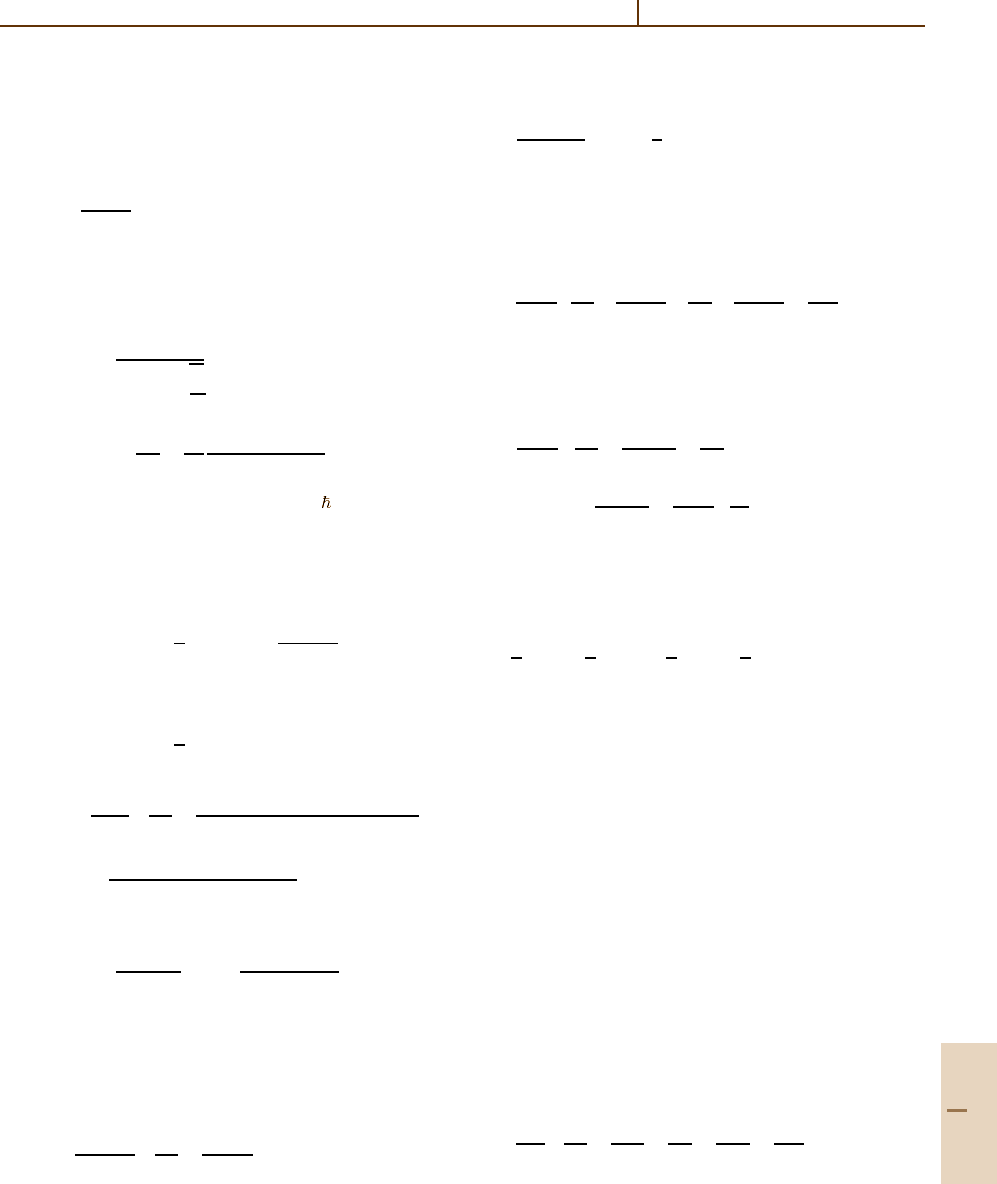
Rydberg Collisions: Binary Encounter, Born and Impulse Approximations 56.8 Binary Encounter Approximation 853
where
X =−cos
2
φ +2
ˆ
v
1
·
ˆ
P
ˆ
v
2
·
ˆ
P
cos φ +1
−
ˆ
v
1
·
ˆ
P
2
−
ˆ
v
2
·
ˆ
P
2
(56.208a)
= (cos φ
min
−cos φ)(cos φ −cos φ
max
) (56.208b)
=
v
v
1
v
2
P
2
(E
max
−E)(E − E
min
), (56.208c)
with φ being the angle between the velocity vectors v
1
and v
2
.
For the special case of electron impact, M
2
= m
e
,
Z
2
=−1, and
σ
E,P
(φ) =
8Z
2
1
e
4
v
2
1
v
2
P
4
√
X
,
(56.209)
σ
±
E,P
(φ) = 8e
4
v
2
1
v
2
√
X
×
$
1
P
4
+
1
S
4
2cos
η
P
−η
S
P
2
S
2
%
,
(56.210)
where η
P
−η
S
=−2γ ln(P/S) =
2e
2
/ v
ln(S/P),
and X isgivenby(56.208b).
Integrated Cross Sections
For incident heavy particles:
σ
E,P
=
π
0
σ
E,P
(φ)
1
2
sin φ dφ =
4πZ
2
1
e
4
v
2
1
v
2
P
4
. (56.211)
For incident electrons:
σ
±
E,P
=
π
0
σ
±
E,P
(φ)
1
2
sin φ dφ
(56.212a)
=
4πe
4
v
2
1
v
2
$
1
P
4
+
v
2
1
+v
2
2
− P
2
/2m
2
e
−2E
2
/P
2
m
4
e
v
2
1
−v
2
2
−2E/m
e
3
±
2Φ
m
2
e
P
2
v
2
1
−v
2
2
−2E/m
e
%
,
(56.212b)
where Φ can be approximated [56.35]by
Φ ∼cos
$
R
∞
E
3
−E
2
1/2
ln
E
E
3
−E
2
−E
%
.
(56.213)
and E
3
is defined in [56.35].
Cross Sections per Unit Energy
For incident heavy particles (three cases):
σ
E
=
2πZ
2
1
e
4
m
e
v
2
1
$
1
E
2
+
2m
e
v
2
2
3E
2
%
,
(56.214)
which is valid for 2v
1
≥ v
2
+v
2
, E ≤ 2m
e
v
1
(v
1
−v
2
),
or
σ
E
=
πZ
2
1
e
4
3v
2
1
v
2
E
3
4v
3
1
−
1
2
(v
2
−v
2
)
2
,
(56.215)
which is valid for v
2
−v
2
≤ 2v
1
≤ v
2
+v
2
,2m
e
v
1
(v
1
−v
2
) ≤ E ≤ 2m
e
v
1
(v
1
+v
2
),orotherwise,σ
E
= 0
for E ≥ m
e
v
1
(v
2
+v
2
).
For incident electrons (two cases):
σ
±
E
=
2πe
4
m
e
v
2
1
$
1
E
2
+
2m
e
v
2
2
3E
3
+
1
D
2
+
2m
e
v
2
2
3D
3
±
2Φ
ED
%
,
(56.216)
which is valid for m
e
(v
2
−v
2
) ≤ m
e
(v
1
−v
1
),
m
e
(v
2
+v
2
) ≤m
e
(v
1
+v
1
), D ≥0, or
σ
E
=
2πe
4
m
e
v
2
1
1
E
2
+
2m
e
v
2
1
3E
3
+
1
D
2
+
2m
e
v
2
1
3|D|
3
±
2Φ
E|D|
v
1
v
1
, (56.217)
which is valid for m
e
(v
2
−v
2
) ≤ m(v
1
−v
1
),
m
e
(v
1
+v
1
) ≤ m
e
(v
2
+v
2
), D ≤ 0. In the expressions
above, the exchange energy D transferred during the
collision is
D =
1
2
m
e
v
2
1
−
1
2
m
e
v
2
2
=
1
2
m
e
v
2
1
−
1
2
m
e
v
2
2
−E .
(56.218)
56.8.2 Integral Cross Sections
e
−
(T ) + A(E
2
) → e
−
(E) + A
+
+e
−
, (56.219)
where T is the initial kinetic energy of the projectile
electron, while the Rydberg electron, initially bound in
potential U
i
to the core A
+
, has kinetic energy E
2
.The
cross section per unit energy E is denoted below by σ
E
.
See the review by Vriens [56.34] for details.
For electron impact, there are two collision mod-
els: the unsymmetrical collision model of Thomson and
Gryzinski assumes that the incident electron has zero
potential energy, and the symmetrical collision model
of Thomas and Burgess assumes that the incident elec-
tron is accelerated initially by the target (and thereby
gains kinetic energy) while losing an equal amount of
potential energy.
Unsymmetrical model (two cases):
σ
E
=
πe
4
T
1
E
2
+
4E
2
3E
3
+
1
D
2
+
4E
2
3D
3
−
Φ
ED
,
(56.220)
Part D 56.8
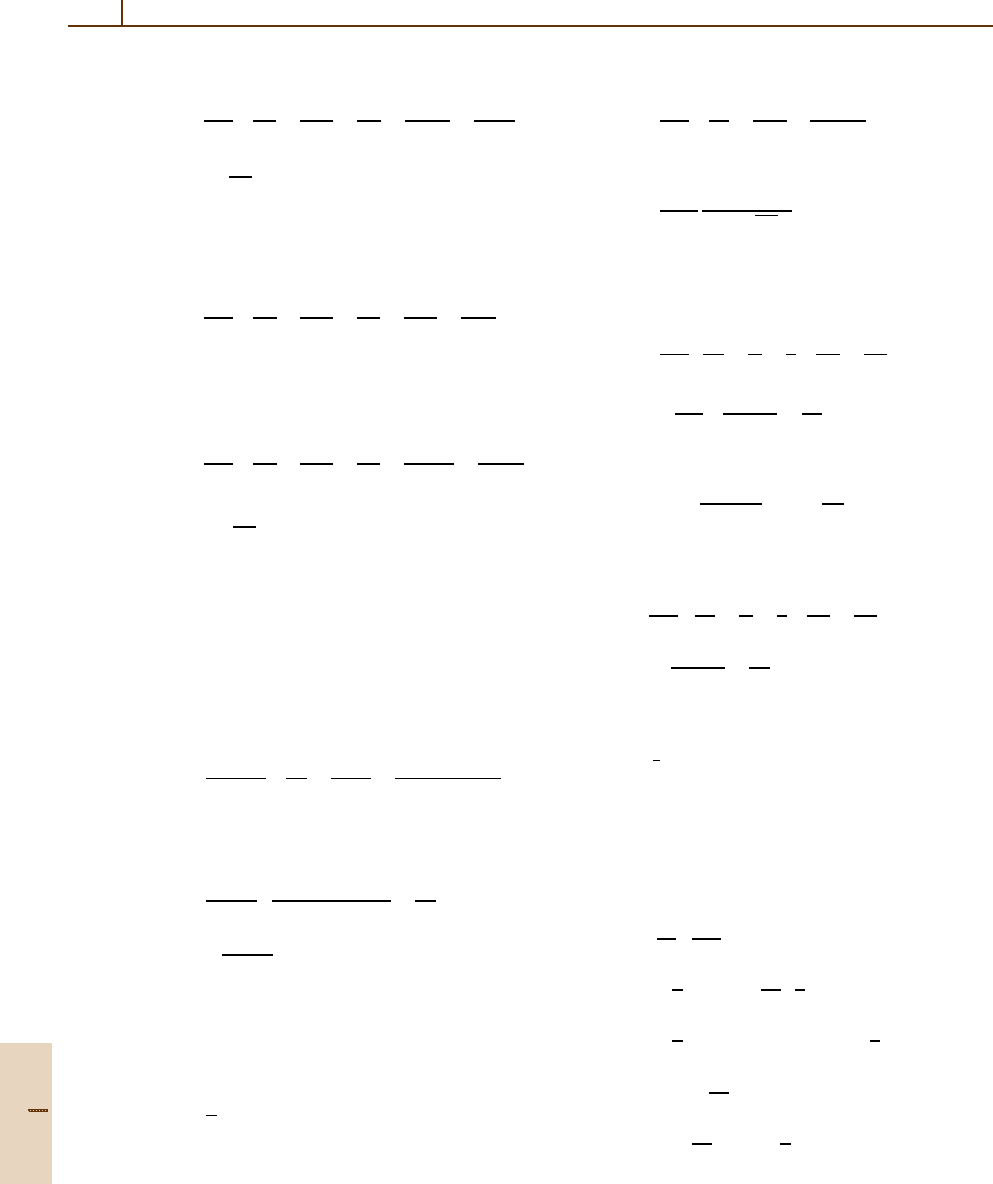
854 Part D Scattering Theory
which is valid for D = T − E
2
−E ≥ 0or,
σ
E
=
πe
4
T
1
E
2
+
4T
3E
3
+
1
D
2
+
4T
3|D|
3
−
Φ
E|D|
×
T
E
2
1/2
(56.221)
which is valid for D ≤ 0andT ≥ E;andwhereT
≡
T −E.
Symmetrical model (two cases):
σ
E
=
πe
4
T
i
$
1
E
2
+
4E
2
3E
3
+
1
X
2
i
+
4E
2
3X
3
i
−
Φ
EX
i
%
,
(56.222)
which is valid for X
i
≡ T +U
i
−E ≥ 0, with T
i
≡
T +U
i
+E
2
,and
σ
E
=
πe
4
T
i
$
1
E
2
+
4T
i
3E
3
+
1
X
2
i
+
4T
i
3|X
i
|
3
−
Φ
E|X
i
|
%
×
T
i
E
2
1/2
(56.223)
which is valid for 0 ≤ T
i
≤ E
2
, T ≥ 0, with T
i
≡ T
i
−E,
and where Φ is given by (56.213).
For incident heavy particles, the unsymmetrical
model (56.220) should be used.
Single Particle Ionization
The total ionization cross section per atomic electron for
incident heavy particles is
Q
i
=
2πZ
2
1
e
4
m
e
v
2
1
$
1
U
i
+
m
e
v
2
2
3U
2
i
−
1
2m
e
v
2
1
−v
2
2
%
,
(56.224)
which is valid for U
i
≤ 2m
e
v
1
(v
1
−v
2
),or
Q
i
=
πZ
2
1
e
4
m
e
v
2
1
1
2m
e
v
2
(v
1
+v
2
)
+
1
U
i
+
m
e
3v
2
U
2
i
2v
3
1
+v
3
2
−
2U
i
/m
e
+v
2
2
3/2
,
(56.225)
which is valid for 2m
e
v
1
(v
1
−v
2
) ≤ U
i
≤ 2m
e
v
1
(v
1
+v
2
),orotherwiseQ
i
=0forU
i
≥2m
e
v
1
(v
1
+v
2
).
For electron impact,
Q
i
=
1
2
Q
dir
i
+Q
ex
i
+Q
int
i
.
(56.226)
In the unsymmetrical model, Q
ex
i
diverges, hence the
exchange and interference terms above are omitted in
the unsymmetrical model for electrons to obtain
Q
dir
i
=
πe
4
T
$
1
U
i
+
2E
2
3U
2
i
−
1
T −E
2
%
,
(56.227)
which is valid for T ≥ E
2
+U
i
,or
Q
dir
i
=
2πe
4
3T
(T −U
i
)
3/2
U
2
i
√
E
2
, (56.228)
which is valid for U
i
≤ T ≤ E
2
+U
i
.
In the symmetrical model,
Q
dir
i
= Q
ex
i
=
πe
4
T
i
&
1
U
i
−
1
T
+
2
3
$
E
2
U
2
i
−
E
2
T
2
%'
,
(56.229)
Q
int
i
=−
πe
4
T
i
2Φ
T +U
i
ln
T
U
i
,
(56.230)
where Φ
can be approximated by [56.35]
Φ
= cos
&
R
∞
E
1
+U
i
1/2
ln
E
1
U
i
'
.
(56.231)
and E
1
is defined in [56.35].
The sum of (56.229)and(56.230) yields
Q
i
=
πe
4
T
i
1
U
i
−
1
T
+
2
3
E
2
U
i
−
E
2
T
2
−
Φ
T +U
i
ln
T
U
i
,
(56.232)
which is also obtained by integrating the expression
(56.223)forσ
E
,
Q
i
=
1
2
(T+U
i
)
U
i
σ
E
dE . (56.233)
Ionization Rate Coefficients. For heavy particle im-
pact [56.29],
Q
=
a
2
0
κ
2
128
9
κ
3
b
3
−b
3/2
+
1
3
λb
35 −
58
3
b
8
3
b
2
+
2
3
κab
5 −4κ
2
3a
2
+
3
2
ab+b
2
−λκ
15
2
+9a+5b
−16κa
4
ln
4κ
2
1
+θ
35
6
−κ
2
a
5
2
+3a+4a
2
+8a
3
,
(56.234)
Part D 56.8
Rydberg Collisions: Binary Encounter, Born and Impulse Approximations 56.8 Binary Encounter Approximation 855
where
κ = v
1
/v
0
,
λ = κ −(4κ)
−1
,
θ = π +2tan
−1
λ,
a = (1 +κ
2
)
−1
,
b = (1 +λ
2
)
−1
.
σ
E,P
dPdE=
64e
4
v
5
0
3v
2
1
P
4
×
$
E
P
−
P
2m
e
2
v
2
0
%
−3
dP dE ,
(56.235)
where
1
2
m
e
v
2
0
is the ionization energy of H(1s).
Scaling Laws. Given the binary encounter cross section
for ionization by protons of energy E
1
of an atom with
binding energy u
a
, the cross section for ionization of an
atom with different binding energy u
b
and scaled proton
energy E
1
can be determined to be [56.18]
σ
ion
E
1
, u
b
=
$
u
2
a
u
2
b
%
σ
ion
(E
1
, u
a
), (56.236)
E
1
= (u
b
/u
a
)E
1
, (56.237)
where σ
ion
(E, u) is the ionization cross section for re-
moval of a single electron from an atom with binding
energy u by impact with a proton with initial energy E.
Double Ionization. See [56.36] for binary encounter
cross section formulae for the direct double ionization
of two-electron atoms by electron impact.
Excitation. Excitation is generally less violent than
ionization and hence binary encounter theory is less
applicable. Binary encounter theory can be applied to ex-
change excitation transitions, e.g., e
−
+He(n
1
L) →e
−
+He(n
3
L), with the restriction of large incident electron
velocities. The cross section is
Q
ex
e
=
U
n+1
U
n
σ
E,ex
dE
=
πe
4
T
i
&
1
T
n+1
−
1
T
n
+
2
3
$
E
2
T
2
n+1
−
E
2
T
2
n
%'
,
(56.238)
valid for T ≥U
n+1
, with T
n
≡ T +U
i
−U
n
and T
n+1
≡
T +U
i
+U
n+1
,or
Q
ex
e
=
T
U
n
σ
E,ex
dE (56.239)
=
πe
4
T
i
&
1
U
i
−
1
T
n
+
2
3
$
E
2
U
2
i
−
E
2
T
2
n
%'
,
valid for U
n
≤ T ≤ U
n+1
. U
n
and U
n+1
denote the
excitation energies for levels n and n +1, respectively.
56.8.3 Classical Ionization Cross Section
Applying the classical energy-change cross section
result (56.69)ofGerjuoy [56.16] to the case of electron-
impact ionization yields the four cases [56.17]
σ
ion
(v
1
,v
2
) ∼
∆E
u
∆E
σ
eff
∆E
(v
1
,v
2
;m
1
/m
2
) d(∆E)
=
π
Z
1
Z
2
e
2
2
3v
2
1
v
2
$
−2v
3
2
(∆E)
2
−
6v
2
m
2
∆E
%
,
(56.240)
which is valid for 0 < ∆E < b,or
σ
ion
(
v
1
,v
2
)
=
π
Z
1
Z
2
e
2
2
3v
2
1
v
2
×
$
4
v
1
−2v
1
m
2
1
v
1
−v
1
2
+
4
v
2
−2v
2
m
2
2
v
2
−v
2
2
%
,
(56.241)
which is valid for b < ∆E < a,or
σ
ion
(
v
1
,v
2
)
=
π(Z
1
Z
2
e
2
)
2
3v
2
1
v
2
$
−2v
3
1
(∆E)
2
%
,
(56.242)
which is valid for ∆E > a,2m
2
v
2
> |m
1
−m
2
|v
1
,or
otherwise is zero for ∆E > a,2m
2
v
2
< |m
1
−m
2
|v
1
.
The limits ∆E
,u
to the ∆E integration in each of
the four cases is indicated in the appropriate validity
conditions. The constants a and b above are given by
a =
4m
1
m
2
(m
1
+m
2
)
2
E
1
−E
2
+
1
2
v
1
v
2
(m
1
−m
2
)
,
b =
4m
1
m
2
(m
1
+m
2
)
2
E
1
−E
2
−
1
2
v
1
v
2
(m
1
−m
2
)
.
The expressions above for σ
ion
(v
1
,v
2
) must be averaged
over the speed distribution of v
2
before comparison with
experiment. See [56.17] for explicit formulae for the
case of a delta function speed distribution.
Part D 56.8
