Drake G.W.F. (editor) Handbook of Atomic, Molecular, and Optical Physics
Подождите немного. Документ загружается.

660 Part D Scattering Theory
approach to within a distance R
X
are
b
2
X
= R
2
X
[
1 −V(R
X
)/E
]
, (45.5a)
L
2
X
= 2MR
2
X
[
E −V(R
X
)
]
. (45.5b)
The trajectory is defined by R = R(t; E, b), ψ =
ψ(t; E, b),whereR is the distance from the scattering
center O and ψ is measured with respect to the apse
line OA joining O to the pericenter R
c
.Takingt(R
c
) = 0,
(45.1) implies
t(R) =
M
2E
1/2
R
R
c
1 −
V(R)
E
−
b
2
R
2
1/2
dR ,
(45.6)
which implicitly provides R = R(t; E, b).
ψ(t; E, b) =
L
M
t
0
R
−2
(t) dt
=
2MEb
2
1/2
M
t
0
R
−2
(t) dt. (45.7)
Orbit integral (0 ≤ ψ ≤ π): ψ is symmetrical about
and measured from the apse line joining O and R
c
.
ψ(R; E, b) = b
R
R
c
dR/R
2
1 −V(R)/E −b
2
/R
2
1/2
(45.8)
=−
∂
∂b
R
R
c
1 −
V(R)
E
−
b
2
R
2
1/2
dR .
(45.9)
For large b and/or small V(R)/E 1, (45.9) reduces to
ψ(R; E, b) =
π
2
−sin
−1
b
R
+
1
2E
∂
∂b
×
R
b
V(R) dR
1 −b
2
/R
2
1/2
, (45.10)
ψ(R →∞;E, b) =
π
2
+
b
2E
×
∞
b
dV
dR
dR
R
2
−b
2
1/2
.
(45.11a)
For a straight-line path, R
2
= b
2
+ Z
2
,whereZ is the
distance along the scattering axis, and
ψ(R →∞;E, b) =
π
2
+
1
4E
∂
∂b
∞
−∞
V(b, Z) dZ .
(45.11b)
45.1.1 Deflection Functions
The deflection function χ(E, b),(−∞ ≤ χ<π), is de-
fined to be χ(E, b) = π −2ψ(R →∞;E, b).Then
χ(E, b) = π −2b
∞
R
c
dR/R
2
1 −V(R)/E −b
2
/R
2
1/2
,
(45.12)
= π −2
×
1
0
1 −V(R
c
/x)/E
1 −V(R
c
)/E
−x
2
−1/2
dx .
(45.13)
An expression which avoids spurious divergences is
χ(E, b) = π +2
∂
∂b
(45.14)
×
∞
R
c
1 −V(R)/E −b
2
/R
2
1/2
dR .
Small-angle scattering, V(R
c
)/E 1, b ∼ R
c
:
χ(E, b) =
R
c
E
∞
R
c
[
V(R
c
) −V(R)
]
R dR
R
2
− R
2
c
3/2
(45.15a)
=
1
E
1
0
[
V(R
c
) −V(R
c
/x)
]
dx
1 −x
2
3/2
, (45.15b)
where x = R
c
/R .From(45.10)–(45.11b), other forms
are
χ(E, b) =−
1
E
∂
∂b
∞
b
V(R) dR
1 −b
2
/R
2
1/2
(45.16a)
=−
b
E
∞
b
dV
dR
dR
R
2
−b
2
1/2
(45.16b)
=−
1
2E
∂
∂b
∞
−∞
V(b, Z) dZ . (45.16c)
Part D 45.1
Elastic Scattering: Classical, Quantal, and Semiclassical 45.1 Classical Scattering Formulae 661
For straight-line paths R
2
= b
2
+v
2
t
2
,(45.12)
yields the impulse-momentum result
χ(E, b) = (Mv)
−1
∞
−∞
F
⊥
(t) dt = ∆ p
⊥
/ p
∞
,
(45.17)
where ∆ p
⊥
is the momentum transferred perpendicu-
lar to the incident direction and F
⊥
=−(∂V/∂R)(b/R)
is the impulsive force causing scattering. Special cases
are
Head-on collisions (b = 0):χ(E, 0) = π.
Overall repulsion: 0 <χ≤ π.
Overall attraction: −∞ ≤ χ ≤ 0.
Forward glory: χ =−2nπ.
Backward glory: χ =−(2n −1)π,
n = 0, 1, 2,....
Rainbow scattering: ( dχ/ db) = 0atχ
r
< 0.
Deflection range: χ
r
≤ χ ≤ π.
Orbiting collisions: cf. (45.34).
Diffraction scattering: χ →0asb →∞.
The scattered particle may wind or spiral many
times around (χ →−∞) the scattering center. The
experimentally observed quantity is the scattering an-
gle θ (0 ≤ θ ≤ π) which is associated with various
deflections
χ
i
=+θ, −θ, −2π ±θ, −4π ±θ,...
(i = 1, 2,...n)
resulting from n different impact parameters b
i
.
Gauss–Mehler Quadrature Evaluation of the Deflec-
tion Function.
χ(E, b) = π
1 −
b
R
c
1
n
n
j=1
a
k
g(a
j
)
,
(45.18)
where
a
k
= cos
2 j −1
4n
π
, k = n +1 − j , and
g(x) =
1 −V(R
c
/x)/E −b
2
x
2
/R
2
c
−1/2
,
0 ≤ x ≤ 1 .
45.1.2 Elastic Scattering Cross Section
Differential cross section:
dσ(θ, E)
dΩ
≡ I(θ; E) ≡σ(θ; E),
σ(θ, E) =
n
i=1
|
b
i
db
i
d(cos χ
i
)
|=
n
i=1
I
i
(θ) . (45.19)
Integral cross section for scattering by angles θ ≥θ
0
:
σ
0
(E) = 2π
π
θ
0
I(θ; E) d(cos θ) = 2π
b
0
(θ
0
)
0
b db ,
(45.20)
where θ
0
results from one b
0
= b(θ
0
).Whenθ
0
results
from three impact parameters b
1
, b
2
, b
3
, for example,
then
σ
0
(E) = 2π
b
1
0
b db +2π
b
3
b
2
b db
= π
b
2
1
+b
2
3
−b
2
2
.
(45.21)
Diffusion (momentum-transfer) cross section:
σ
d
(E) = 2π
π
0
[
1 −cos θ(E, b)
]
I(θ) d(cos θ) ,
(45.22a)
= 2π
∞
0
[
1 −cos θ(E, b)
]
b db ,
(45.22b)
= 4π
∞
0
sin
2
1
2
θ(E, b)
b db .
(45.22c)
Viscosity cross section:
σ
v
(E) = 2π
π
0
1 −cos
2
θ(E, b)
I(θ) d(cos θ) ,
(45.23a)
= 2π
∞
0
1 −cos
2
θ(E, b)
b db , (45.23b)
= 2π
∞
0
sin
2
θ(E, b)
b db . (45.23c)
Part D 45.1
662 Part D Scattering Theory
Small-Angle Diffraction Scattering. The small-angle
scattering regime is defined by the conditions
V(R
c
)/E 1, b ≥ R
c
,whereθ =|χ|. The main con-
tribution to dσ/dΩ for small-angle scattering arises
from the asymptotic branch of the deflection func-
tion χ at large impact parameters b, and is primarily
determined by the long-range (attractive) part of the
potential V(R) (Sect. 45.3.6).
Large-Angle Scattering. The main contribution to
dσ/dΩ for large-angle scattering arises from the pos-
itive branch of χ at small b and is mainly determined by
the repulsive part of the potential.
45.1.3 Center-of-Mass to Laboratory
Coordinate Conversion
Let ψ
1
, ψ
2
be the angles for scattering and recoil, re-
spectively, of the projectile by a target initially at rest in
the lab frame. Then
σ
1
(ψ
1
)dΩ
1
= σ
2
(ψ
2
)dΩ
2
= σ
(1,2)
cm
(θ) dΩ
cm
,
0 ≤ θ ≤ π ;
(45.24)
σ
(2)
cm
(θ, φ) = σ
(1)
cm
(π −θ, φ +π) . (45.25)
(A) Two-body elastic scattering process without
conversion of translational kinetic energy into internal
energy: (1) +(2) → (1) +(2).
σ
1
(ψ
1
) = σ
cm
(θ)
1 +2x cos θ +x
2
3/2
|1 +x cos θ|
;
(45.26)
σ
2
(ψ
2
) = σ
cm
(θ)
4sin
1
2
θ
;
ψ
2
=
1
2
(π −θ) , 0 ≤ψ
2
≤
1
2
π ;
(45.27)
tan ψ
1
=
sin θ
(x +cos θ)
, x = M
1
/M
2
. (45.28)
M
1
> M
2
:As0≤ θ ≤ θ
c
= cos
−1
(−M
2
/M
1
) ,
0 ≤ ψ
1
→ ψ
max
1
= sin
−1
(M
2
/M
1
)<
1
2
π .
As θ
c
≤ θ → π, ψ
max
1
≤ ψ
1
→ 0.
θ is a double-valued function of ψ ;
M
1
= M
2
: σ
1
(ψ
1
) = (4cosψ
1
)σ
cm
(θ = 2ψ
1
) ,
0 ≤ ψ
1
≤
1
2
π, ψ
1
+ψ
2
=
1
2
π ;
no backscattering .
M
1
M
2
:σ
1
(ψ
1
) = σ
cm
(θ = ψ
1
) ;
lab and cm frames identical.
(B) Two-body elastic scattering process with con-
version of translational kinetic energy into internal
energy: (1) +(2) → (3) +(4). For conversion of inter-
nal energy ε
i
so that kinetic energy of relative motion
(in the cm frame) increases from E
i
to E
f
= E
i
+ε
i
.
For j = 3, 4,
σ
j
(ψ
j
) = σ
cm
(θ)
1 +2x
j
cos θ +x
2
j
3/2
1 +x
j
cos θ
,
(45.29)
x
3
=
M
1
M
3
E
i
M
2
M
4
E
f
1/2
,
x
4
=−
M
1
M
4
E
i
M
2
M
3
E
f
1/2
,
tan ψ
3
=
sin θ
(x
3
+cos θ)
, tan ψ
4
=
sin θ
(|x
4
|−cos θ)
.
45.1.4 Glory and Rainbow Scattering
Glory. The deflection function χ passes through −2nπ
(forward glory) or −(2n +1)π (backward glory) at finite
impact parameters b
g
.Thensinθ → 0asθ → θ
g
so that
classical cross section diverges as
σ(E,θ)=
2b
g
sin θ
db
dχ
g
as θ → θ
g
. (45.30)
Rainbow. The deflection function χ passes through
anegativeminimumatb = b
r
; (dχ/db)
r
→ 0sothat
χ(b) = χ(b
r
) +ω
r
(b −b
r
)
2
, (45.31)
ω
r
=
1
2
d
2
χ
db
2
b
r
> 0 . (45.32)
The classical cross section diverges as
σ(E,θ)=
b
r
2sinθ
[
ω
r
(θ
r
−θ)
]
−1/2
,θ<θ
r
,
(45.33)
and is augmented by the contribution from the positive
branch of χ(b).
45.1.5 Orbiting and Spiraling Collisions
Attractive interactions V(R) =−C/R
n
(n ≥2) can sup-
port quasibound states with positive energy within the
angular momentum barrier. Particles with b < b
0
spiral
towards the scattering center. Those with b = b
0
are in
Part D 45.1
Elastic Scattering: Classical, Quantal, and Semiclassical 45.1 Classical Scattering Formulae 663
unstable circular orbits of radius R
0
. The radius R
0
is
determined from the two conditions
dV
eff
dR
R
0
= 0 , E = V
eff
(R
0
), (45.34)
which, when combined, yields
E = V
eff
(R
0
) = V(R
0
) +
1
2
R
0
dV
dR
R
0
. (45.35)
The angular momentum L
0
of the circular orbit is
L
2
0
= (2ME)b
2
0
= 2MR
2
0
E −V(R
0
)
. (45.36)
Thus b
2
0
= R
2
0
F,where
F = 1 −
V(R
0
)
E
=
1
2
R
0
E
dV
dR
R
0
(45.37)
is the focusing factor. The orbiting and spiraling cross
section is then
σ
orb
(E) = πb
2
0
= πR
2
0
F . (45.38)
45.1.6 Quantities Derived
from Classical Scattering
The semiclassical phase η(E, b) ≡ η(E,λ), with
λ =
+
1
2
= kb is a function of b or λ. The quanti-
tites p(R ) ≡ p(R; E, L) and p
0
(R) ≡ p
0
(R; E, L) are
radial momenta in the presence and absence of the
potential V(R), respectively.
η
SC
(E, b) =
1
∞
R
c
p(R) dR −
∞
b
p
0
(R) dR
(45.39)
= k
∞
R
c
1 −V/E −b
2
/R
2
1/2
dR
− k
∞
b
1 −b
2
/R
2
1/2
dR . (45.40)
Asymptotic speed v: E =
1
2
Mv
2
=
2
k
2
/2M,
k/2E = 1/
v.
Jeffrey–Born phase function:
For small V/E and b ∼ R
c
,
η
JB
(E, b) =−
k
2E
∞
b
V(R) dR
1 −b
2
/R
2
1/2
. (45.41)
Eikonal phase function:
For small V/E and a linear trajectory R
2
= b
2
+ Z
2
,
η
E
(E, b) =−
k
4E
∞
−∞
V(b, Z) dZ . (45.42)
Semiclassical cross sections:
σ(E) = 8π
∞
0
sin
2
η(E, b)
b db , (45.43)
=
8π/k
2
∞
0
sin
2
η(E,λ)λdλ. (45.44)
Landau–Lifshitz cross section:
σ
LL
(E) = 8π
∞
0
sin
2
η
JB
(E, b)
b db . (45.45)
Massey–Mohr cross section:
η(E, b
0
) =
1
2
,
sin
2
η(E, b < b
0
)
=
1
2
,
(45.46)
σ
MM
(E) = 2πb
2
0
+8π
∞
b
0
η
2
JB
(E, b)b db . (45.47)
Schiff cross section:
σ
c(E)
= 4
∞
−∞
dX
∞
−∞
dY
sin
2
η
E
(X, Y )
. (45.48)
This reduces to (45.45) for spherical V(R); b ≡ (x, y).
Random-phase approximation (RPA):
For angle α,
4π
∞
0
P(b) sin
2
α(b)b db = 2π
b
c
0
P(b)b db ,
(45.49)
where α(b
c
) = 1/π.
Collision delay time function:
τ(E,λ)= 2
∂η(E,λ)
∂E
=
2
v
∂η
(k)
∂k
.
(45.50)
Deflection-angle phase function relation:
χ(E,λ)=
2
k
∂η(E, b)
∂b
= 2
∂η(E,λ)
∂λ
.
(45.51)
Part D 45.1
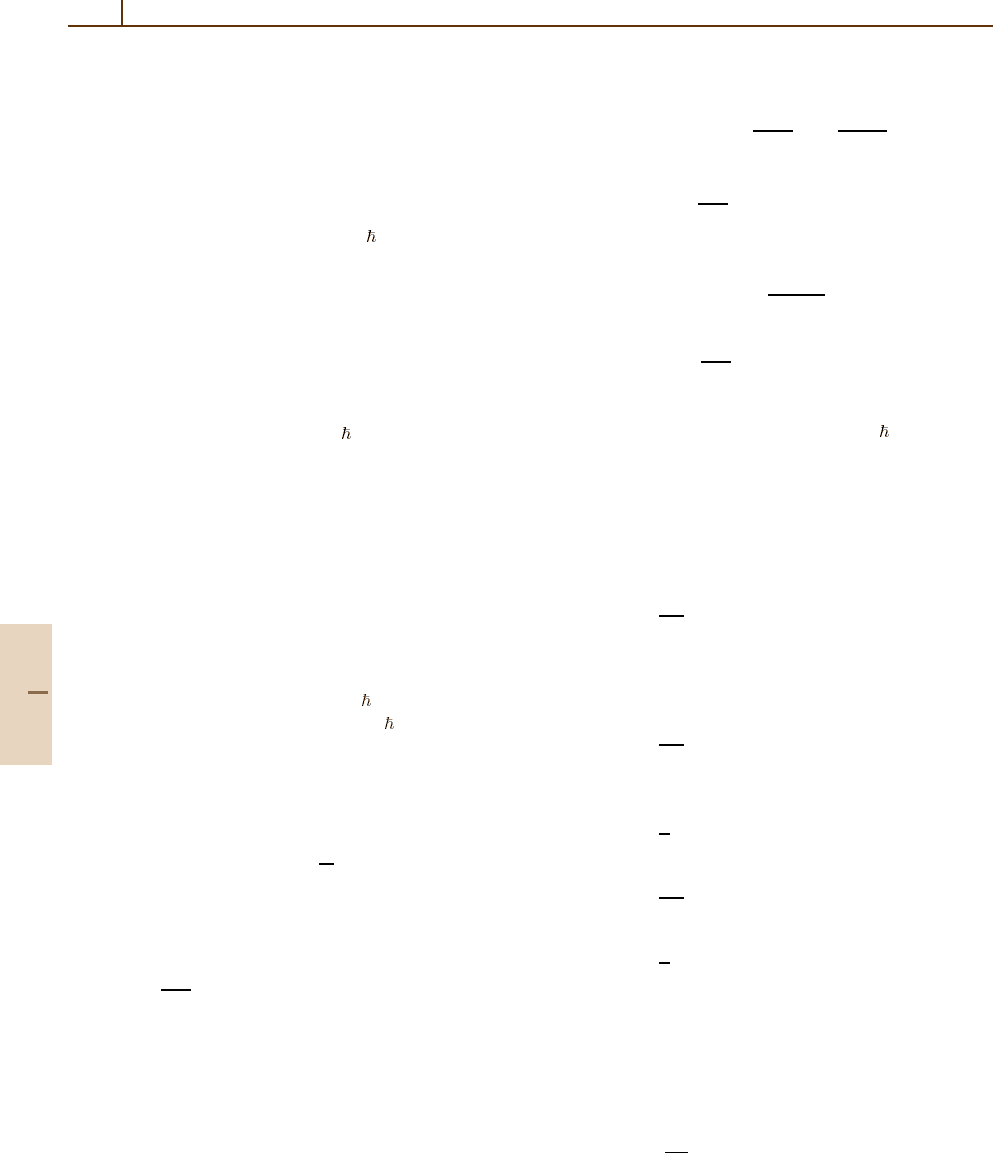
664 Part D Scattering Theory
45.1.7 Collision Action
The classical collision action along a classical path with
deflection χ =χ(E, L), measured relative to the action
along the path of the undeflected particle, is
S
C
(E, L;χ) = S
R
(E, L) − Lχ(E, L) (45.52a)
= 2η
SC
(E, L) − Lχ. (45.52b)
Radial component of collision action S
C
:
S
R
(E, L) = 2
∞
R
c
(E,L)
p(R) dR −2
∞
b(E,L)
p
0
(R) dR
(45.53a)
= 2η
SC
(E, L) (45.53b)
Collision delay time function:
τ(E, L) = 2M
∞
R
c
dR
p(R)
−
∞
b
dR
p
0
(R)
(45.54a)
=
∂S
R
∂E
L
. (45.54b)
Deflection angle function:
χ(E, L) = π −2L
∞
R
c
dR/R
2
p(R)
(45.55a)
=
∂S
R
∂L
E
. (45.55b)
Radial collision action change:
dS
R
= τ(E, L) dE +χ(E, L) dL = 2 dη
SC
(45.56)
45.2 Quantal Scattering Formulae
The basic quantity in quantal elastic scattering is the
complex scattering amplitude f(E,θ), expressed in
terms of the phase shifts η
(E) associated with scat-
tering of the th partial wave. Derived quantities are the
diagonal elements of the scattering matrix S, transition
matrix T and reactance matrix K.
Reduced Energy: k
2
=
2M/
2
E.
Reduced Potential: U(R) =
2M/
2
V(R).
45.2.1 Basic Formulae
Wave function: As R →∞,
Ψ(R) → exp(ikZ) +
1
R
f(θ) exp(ikR)
(45.57)
for symmetric interactions V = V(R).
Elastic scattering DCS:
dσ
dΩ
= I(θ) =|f(θ)|
2
. (45.58)
Scattering, transition and reactance matrix elements
in terms of η
:
S
(k) = exp(2iη
), (45.59a)
T
(k) = sin η
exp(iη
), (45.59b)
K
(k) = tan η
. (45.59c)
Scattering amplitudes f(θ):
f(θ) =
1
2ik
∞
=0
(2 +1)
exp(2iη
) −1
P
(cos θ)
=
∞
=0
f
(θ) , (45.60a)
f(θ) =
1
2ik
∞
=0
(2 +1)
[
S
(k) −1
]
P
(cos θ) ,
(45.60b)
f(θ) =
1
k
∞
=0
(2 +1)T
(k)P
(cos θ) , (45.60c)
f(θ) =
1
2ik
∞
=0
S
(k)P
(cos θ) , θ = 0 , (45.60d)
=
1
k
∞
=0
(2 +1)T
(k), θ = 0 . (45.60e)
Integral cross sections σ(E):
σ(E) = 2π
π
0
I(θ) d(cos θ) , (45.61a)
=
4π
k
2
∞
=0
(2 +1) sin
2
η
. (45.61b)
Part D 45.2
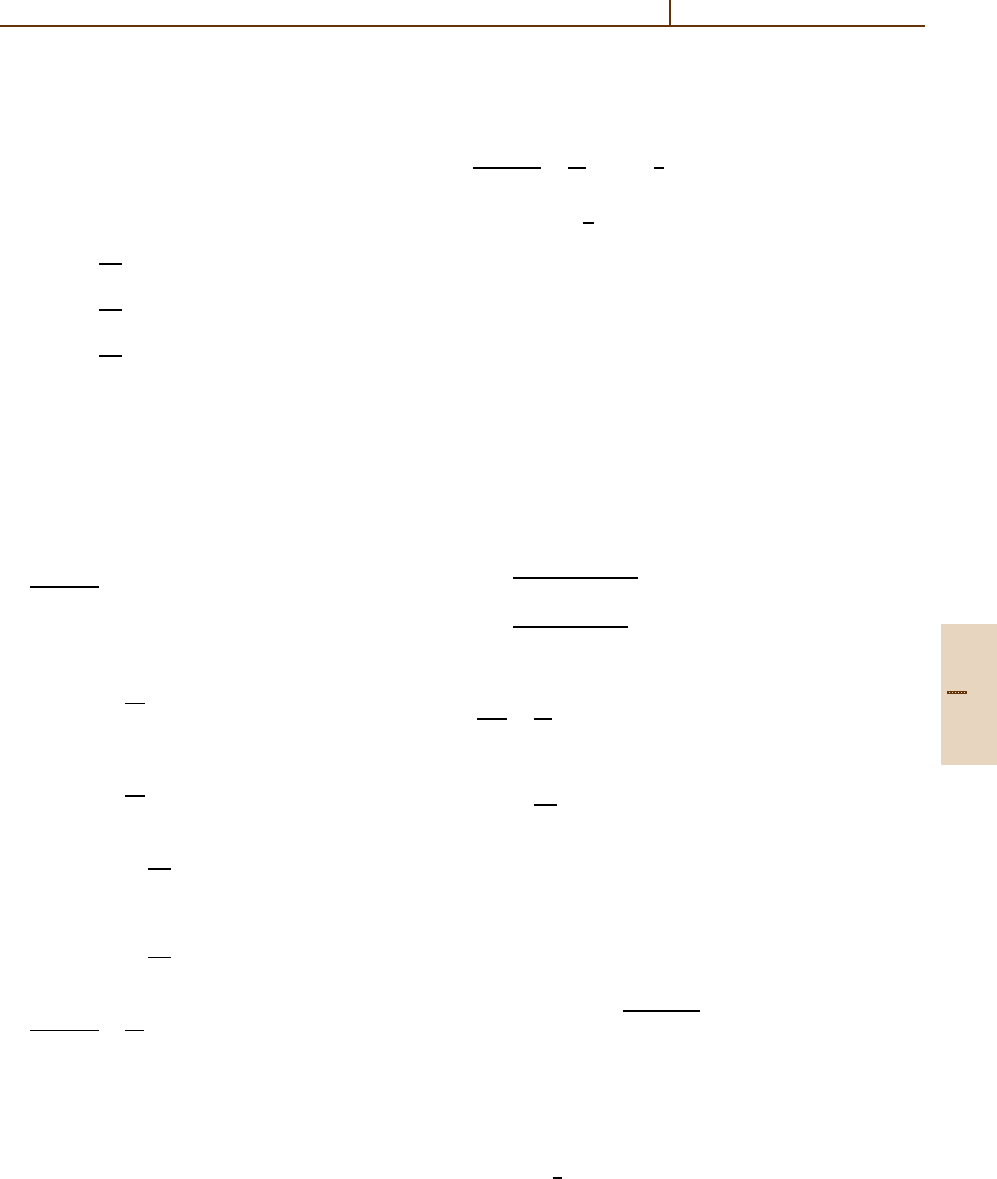
Elastic Scattering: Classical, Quantal, and Semiclassical 45.2 Quantal Scattering Formulae 665
Optical theorem:
σ(E) = (4π/k) Im[ f(0)].
(45.62)
Partial cross sections σ
(E):
σ(E) =
∞
=0
σ
(E) (45.63)
σ
(E) =
4π
k
2
(2 +1) sin
2
η
(45.64a)
=
4π
k
2
(2 +1)|T
|
2
=
2π
k
(2 +1)
[
1 −Re(S
)
]
. (45.64b)
Upper limit:
σ
(E) ≤
4π/k
2
(2 +1).
(45.65)
Unitarity, flux conservation, η
is real:
|S
|
2
= 1, |T
|
2
= Im(T
). (45.66)
Differential cross sections (DCS):
dσ(E,θ)
dΩ
=|f(θ)|
2
= I(θ) = A(θ)
2
+ B(θ)
2
,
(45.67)
A(θ) = Re[ f(θ)]
=
1
2k
∞
=0
(2 +1) sin 2η
P
(cos θ) ,
B(θ) = Im[f(θ)]
=
1
2k
∞
=0
(2 +1)
[
1 −cos 2η
]
P
(cos θ) .
A(θ)
2
dΩ =
4π
k
2
∞
=0
(2 +1) sin
2
η
cos
2
η
,
(45.68a)
B(θ)
2
dΩ =
4π
k
2
∞
=0
(2 +1) sin
4
η
, (45.68b)
dσ(E,θ)
dΩ
=
1
k
2
∞
L=0
a
L
(E)P
L
(cos θ) , (45.69a)
a
L
=
∞
=0
+L
=|−L|
(2 +1)(2
+1)
(
00 |
L0)
2
×sinη
sin η
cos(η
−η
), (45.69b)
where (
mm
|
LM) are the Clebsch–Gordan Coef-
ficients.
Three-term expansion:
dσ(E,θ)
dΩ
=
1
k
2
a
0
−
1
2
a
2
+a
1
cos θ
+
3
2
a
2
cos
2
θ
(45.70)
a
0
(E) =
∞
=0
(2 +1) sin
2
η
, (45.71a)
a
1
(E) = 6
∞
=0
( +1) sin η
sin η
+1
×cos(η
+1
−η
), (45.71b)
a
2
(E) = 5
∞
=0
b
sin
2
η
+c
sin η
sin η
+2
×cos(η
+2
−η
)
. (45.71c)
with coefficients
b
=
( +1)(2 +1)
(2 −1)(2 +3)
,
(45.72a)
c
=
3( +1)( +2)
(2 +3)
.
(45.72b)
S, P wave ( = 0, 1) net contribution:
dσ
dΩ
=
1
k
2
sin
2
η
0
+
[
6sinη
0
sin η
1
cos(η
1
−η
0
)
]
×cosθ + 9sin
2
η
1
cos
2
θ
!
, (45.73)
σ(E) =
4π
k
2
sin
2
η
0
+3sin
2
η
1
.
(45.74)
For pure S-wave scattering, the DCS is isotropic. For
pure P-wave scattering, the DCS is symmetric about θ =
π/2, where it vanishes; the DCS rises to equal maxima
at θ =0,π. For combined S-andP-wave scattering, the
DCS is asymmetric with forward-backward asymmetry.
Transport cross sections (n ≥ 1):
σ
(n)
(E) = 2π
1 −
1 +(−1)
n
2(n +1)
−1
×
π
0
1 −cos
n
θ
I(θ) d(cos θ) . (45.75)
The diffusion and viscosity cross sections (45.22a)
and (45.23a) are given by the transport cross sec-
tions σ
(1)
and
2
3
σ
(2)
, respectively.
Part D 45.2
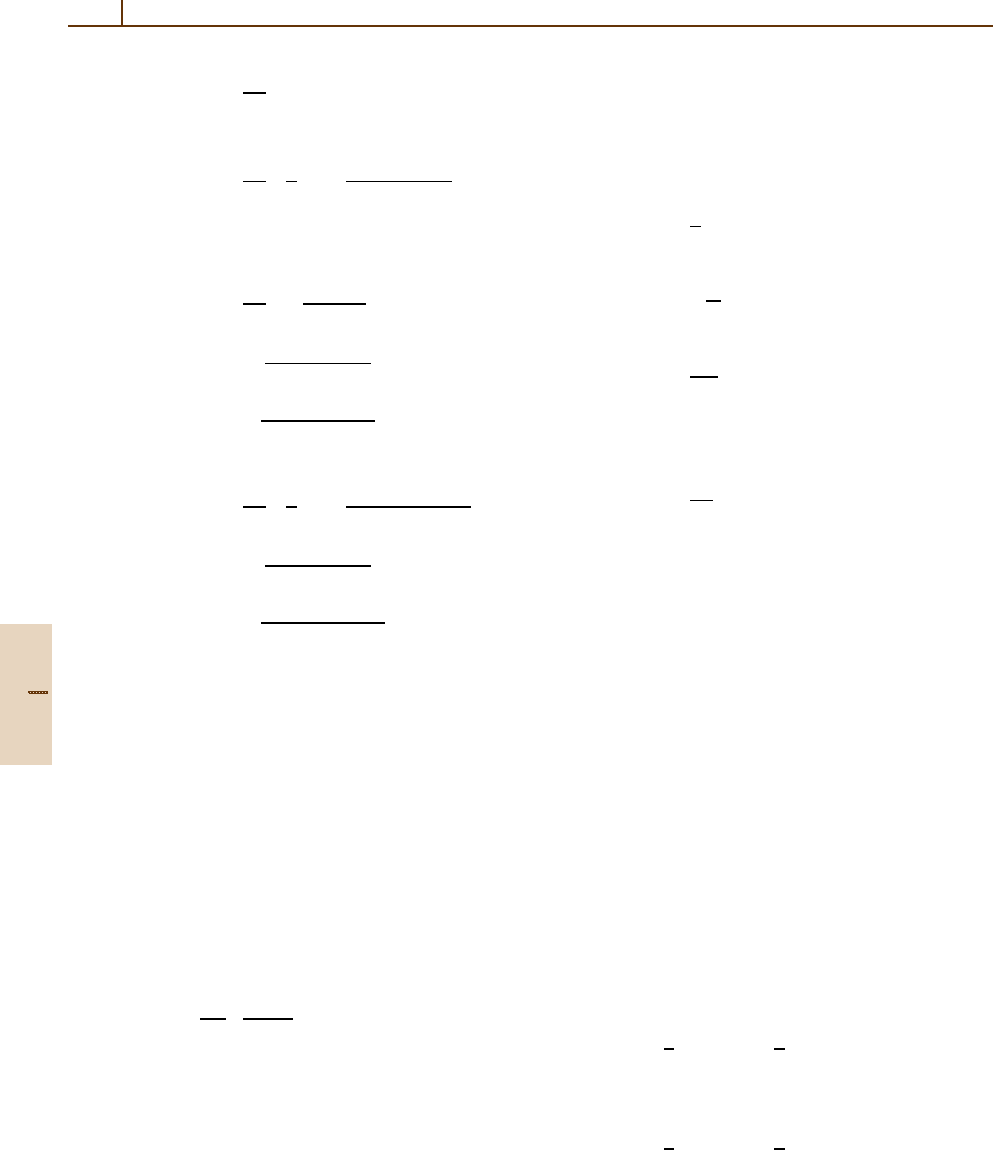
666 Part D Scattering Theory
σ
(1)
(E) =
4π
k
2
∞
=0
( +1) sin
2
(η
−η
+1
),
(45.76a)
σ
(2)
(E) =
4π
k
2
3
2
∞
=0
( +1)( +2)
(2 +3)
×sin
2
(η
−η
+2
),
(45.76b)
σ
(3)
(E) =
4π
k
2
∞
=0
( +1)
(2 +5)
×
( +2)( +3)
(2 +3)
sin
2
(η
−η
+3
)
+
3
2
+2 −1
(2 −1)
sin
2
(η
−η
+1
)
"
,
(45.76c)
σ
(4)
(E) =
4π
k
2
5
4
∞
=0
( +1)( +2)
(2 +3)(2 +7)
×
( +3)( +4)
(2 +5)
sin
2
(η
−η
+4
)
+
2
2
2
+6 −3
(2 −1)
sin
2
(η
−η
+2
)
"
.
(45.76d)
Collision integrals: Averages of σ
(n)
(E) over
a Maxwellian distribution at temperature T are
Ω
(n,s)
(T) =
(s +1)!(kT )
s+2
−1
∞
0
σ
(n)
(E)
×exp(−E/kT )E
s+1
dE . (45.77)
Normalization factors are chosen so that the above ex-
pressions for σ
(n)
and Ω
(n,s)
reduce to πd
2
for classical
rigid spheres of diameter d.
Mobility: The mobility K of ions of charge e in a gas
of density N is given by the Chapman–Enskog formula
K =
3e
8N
π
2MkT
1/2
Ω
(1,1)
(T )
−1
. (45.78)
Phase shifts η
can be determined from the numerical
solution of the radial Schrödinger equation (45.93), from
an integral equation (45.179b), from solving a nonlinear
first-order differential equation (45.179a), from Log-
arithmic Derviatives (Sect. 45.2.5) or from variational
techniques (Sect. 45.2.4).
45.2.2 Identical Particles:
Symmetry Oscillations
Colliding Particles, each with spin s, in a Total Spin S
t
Resolved State in the Range (0 → 2s). Particle inter-
change: Ψ(R) = (−1)
S
t
Ψ(−R)
I
A,S
(θ) =
1
2
| f(θ) ∓ f(π −θ)|
2
, (45.79)
Ψ
A,S
(R) →
exp(ikZ) ∓exp(−ikZ)
+
1
R
f(θ) ∓ f(π −θ)
exp(ikR),
(45.80)
I
A,S
(θ) =
1
4k
2
∞
=0
ω
(2+1)
exp 2iη
−1
× P
(cos θ)
2
, (45.81)
σ
A,S
(E) =
4π
k
2
∞
=0
ω
(2 +1) sin
2
η
, (45.82)
where A and S denote antisymmetric and symmetric
wave functions (with respect to particle interchange) for
collisions of identical particles with odd and even total
spin S
t
A: S
t
odd ω
= 0( even); ω
= 2( odd);
S: S
t
evenω
= 2( even); ω
= 0( odd).
Spin-States S
t
Unresolved. S/A combination:
I(θ) = g
A
I
A
(θ) +g
S
I
S
(θ) , (45.83)
σ(E) = g
A
σ
A
(E) +g
S
σ
S
(E), (45.84)
where g
A
and g
S
are the fractions of states with odd and
even total spins S
t
= 0, 1, 2,... ,2s. For Fermions (F)
with half integer spin s, and Bosons (B) with integral
spin s
F: g
A
= (s +1)/(2s +1) ,g
S
= s/(2s +1) ,
B: g
A
= s/(2s +1) , g
S
= (s +1)/(2s +1) ,
so that (45.83)and(45.84) have the alternative forms
I(F) =|f(θ)|
2
+|f(π −θ)|
2
−I , (45.85a)
σ(F) =
1
2
[
σ
S
+σ
A
]
−
1
2
[
σ
S
−σ
A
]
/(2s +1),
(45.85b)
I(B) =|f(θ)|
2
+|f(π −θ)|
2
+I , (45.85c)
σ(B) =
1
2
[
σ
S
+σ
A
]
+
1
2
[
σ
S
−σ
A
]
/(2s +1),
(45.85d)
Part D 45.2
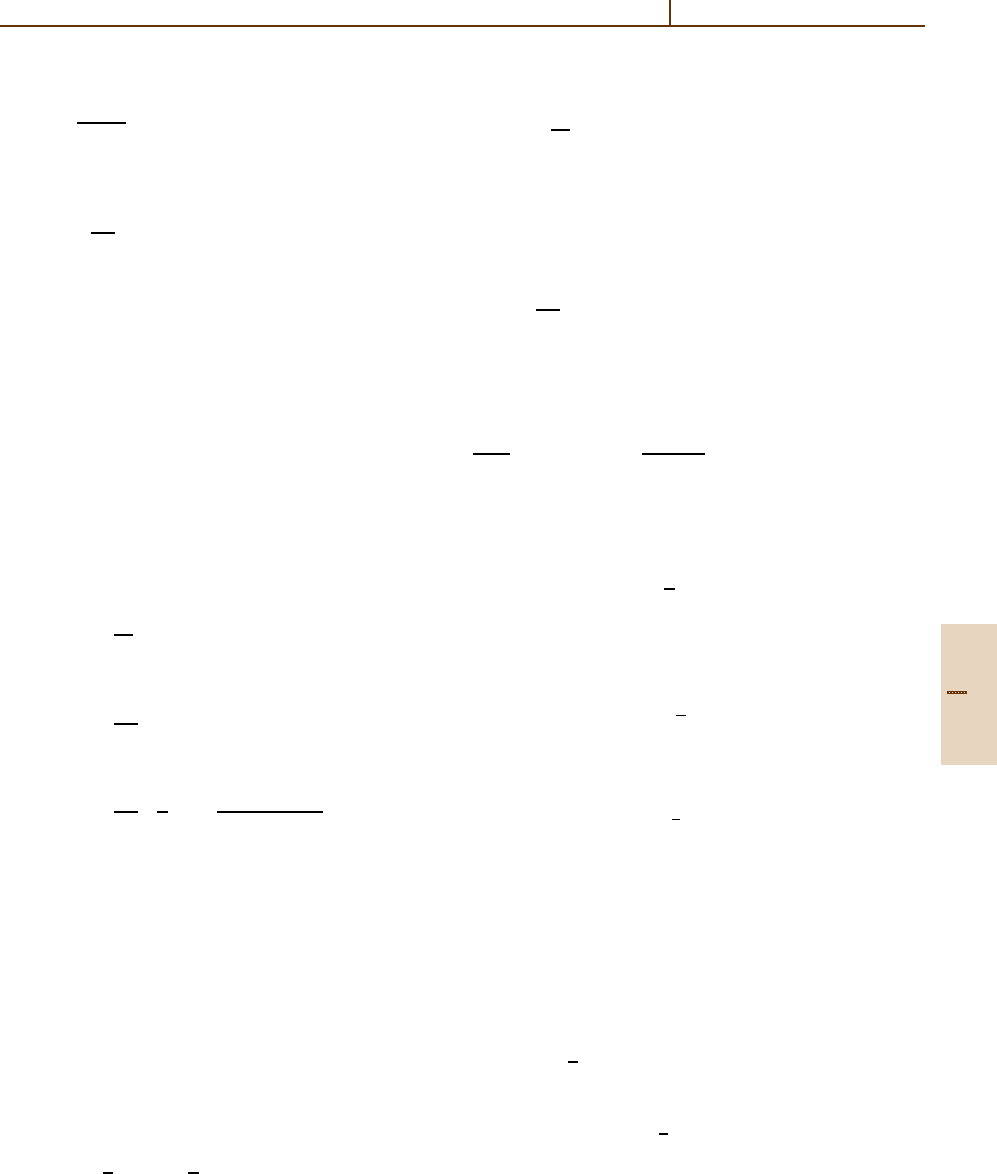
Elastic Scattering: Classical, Quantal, and Semiclassical 45.2 Quantal Scattering Formulae 667
where the interference term is
I =
2
2s +1
Re
f(θ) f
∗
(π −θ)
. (45.86)
Example: For fermions with spin 1/2,
σ(E) =
2π
k
2
∞
=even
(2 +1) sin
2
η
+3
∞
=odd
(2 +1) sin
2
η
.
(45.87)
Symmetry oscillations originate from the inter-
ference between unscattered incident particles in the
forward (θ = 0) direction and backward scattered par-
ticles (θ =π, =0). Symmetry oscillations are sensitive
to the repulsive wall of the interaction.
Resonant Charge Transfer and Transport Cross Sec-
tions for A
+
–A Collisions. The phase shifts for elastic
scattering by the gerade (g) and ungerade (u) poten-
tials of A
+
2
are, respectively, η
g
and η
u
. The charge
transfer (X) and transport cross sections are
σ
X
(E) =
π
k
2
∞
=0
(2 +1) sin
2
η
g
−η
u
,
(45.88a)
σ
(1)
A,S
(E) =
4π
k
2
∞
=0
( +1) sin
2
(β
−β
+1
),
(45.88b)
σ
(2)
A,S
(E) =
4π
k
2
3
2
∞
=0
( +1)( +2)
(2 +3)
×sin
2
(β
−β
+2
) ; (45.88c)
A: β
= η
g
( even), or η
u
( odd) ,
S: β
= η
u
( even), or η
g
( odd) .
σ
(1)
A,S
contains (g/u) interference; σ
(2)
A,S
does not.
When nuclear spin degeneracy is acknowledged, the
cross sections σ
A,S
are summed according to (45.85b)
or (45.85d).
Since there is no coupling between molecular states
of different electronic angular momentum, the scattering
by the
2
Σ
g,u
pair and the
2
Π
g,u
pair of Ne
+
2
potentials
(for example) is independent and
σ
X
(E) =
1
3
σ
Σ
(E) +
2
3
σ
Π
(E). (45.89)
Singlet–Triplet Spin Flip Cross Section.
σ
ST
(E) =
π
k
2
∞
=0
(2 +1) sin
2
η
s
−η
t
,
(45.90)
where η
S,T
are the phase shifts for individual scattering
by the singlet and triplet potentials, respectively.
45.2.3 Partial Wave Expansion
Ψ(R) =
1
kR
∞
=0
A
v
(kR)P
(cos θ) , (45.91)
A
= i
(2 +1) exp(iη
). (45.92)
Radial Schrödinger equation (RSE):
d
2
v
dR
2
+
k
2
−U(R) −
( +1)
R
2
v
(R) = 0 (45.93)
where v
is normalized so that
v
(R)
R>R
0
= cos η
F
(kR) +sin η
G
(kR) (45.94)
→ sin
kR−
1
2
π +η
as R →∞.
The regular (nonsingular) solution (zero at R =0)
of the field-free RSE (45.93) with U(R) = 0is
F
(kR) = (kR) j
(kR) =
1
2
πkR
1/2
J
+1/2
(kR)
(45.95)
→
#
(kR)
+1
/(2 +1)!!, R → 0
sin
kR−
1
2
π
, R →∞,
(45.96)
where j
is the spherical Bessel function. Equation
(45.91) with v
= F
is the partial-wave expansion for
the incident plane-wave exp(ikZ).
The irregular solution (divergent at R =0) of the
field-free RSE is
G
(kR) =−(kR)n
(kR)
=
1
2
πkR
1/2
J
−(+1/2)
(kR) (45.97)
→
#
(2 −1)!!/(kR)
, R → 0
cos
kR−
1
2
π
, R →∞,
(45.98)
where n
is the spherical Neumann function.
Part D 45.2
668 Part D Scattering Theory
The full asymptotic scattering solution is the com-
bination (45.94) of the regular and irregular solutions.
The mixture depends upon:
Forms of Normalization for v
. In (45.91), possible
choices of normalization are:
(a) A
= i
(2 +1) exp iη
, (45.99a)
v
(R) ∼ sin
kR−
1
2
π +η
;
(45.99b)
(b) A
= i
(2 +1) cos η
, (45.100a)
v
(R) ∼ sin
kR−
1
2
π
+K
cos
kR−
1
2
π
;
(45.100b)
(c) A
= i
(2 +1), (45.101a)
v
(R) ∼ sin
kR−
1
2
π
+T
e
i(kR−π/2)
;
(45.101b)
(d) A
=
1
2
i
+1
(2 +1), (45.102a)
v
(R) ∼ e
−i(kR−π/2)
−S
e
i(kR−π/2)
; (45.102b)
S
= 1 +2iT
; K
= T
/(1 +iT
) ;
(45.103)
T
= sin η
e
iη
; 1 +iT
= cos η
e
iη
.
(45.104)
Significance of η
, K
, T
,andS
. The effect of
scattering is therefore to: (1) introduce a phase shift
η
in (45.99b) to the regular standing wave, (2) leave
the regular standing wave alone and introduce ei-
ther an irregular standing wave of real amplitude K
in (45.100b) or, a spherical outgoing wave of amplitude
T
in (45.101b), and (3) to convert in (45.102b)anin-
coming spherical wave of unit amplitude to an outgoing
spherical wave of amplitude S
.
Levinson’s Theorem. A local potential U(R) can support
n
bound states of angular momentum and energy E
n
such that
lim
k→0
η
0
(k) =
n
0
π, E
n
< 0
n
0
+
1
2
π, E
n+1
= 0 ,
(45.105)
lim
k→0
η
(k) = n
π, >0 . (45.106)
45.2.4 Scattering Length
and Effective Range
Blatt–Jackson Effective Range Formula. For short-
range potentials,
k cot η
0
=−
1
a
s
+
1
2
R
e
k
2
+O
k
4
.
(45.107)
Effective range:
R
e
= 2
∞
0
u
2
0
(R) −v
2
0
(R)
dR , (45.108)
where u
0
= sin(kR+η
0
)/ sin η
0
is the k =0 limit of the
potential-free =0 radial wave function and normalized
so that u
0
(R) goes to unity as k → 0. The potential
distorted = 0 radial function v
0
is normalized at large
R to u
0
(R). The effective range is a measure of the
distance over which v
0
differs from u
0
. The outside
factor of 2 in (45.108) is chosen such that R
e
= a for
a square well of range a.
Scattering length:
a
s
=−lim
k→0
f(θ) . (45.109)
Relation with k → 0 Elastic Cross Section.
σ(k →0) =
4π
k
2
sin
2
η
0
= 4πa
2
s
'
1 −
1
2
k
2
a
s
R
e
2
+k
2
a
2
s
"
−1
(45.110)
∼ 4πa
2
s
1 +a
s
k
2
(R
e
−a
s
)
(45.111)
Relation with Bound Levels. If a = 0 bound level of
energy E
n
=−
2
k
2
n
/2M lies sufficiently near the disso-
ciation limit the effective range and scattering lengths,
R
e
and a
s
, respectively, are related by,
−
1
a
s
=−k
n
+
1
2
R
e
k
2
n
+··· (45.112)
Wigner Causality Condition. If η
provides the domi-
nant contribution to f(θ) then
∂η
(k)
∂k
≥−a
s
(45.113)
where a
s
is the scattering length ( = 0) and is a measure
of the range of the interaction.
Part D 45.2
Elastic Scattering: Classical, Quantal, and Semiclassical 45.2 Quantal Scattering Formulae 669
Effective Range Formulae. The Blatt–Jackson formula
must be modified [45.1–5] for long-range interactions
as follows.
(1) Modified Coulomb potential: V(R) ∼ Z
1
Z
2
e
2
/R
2(K/a
0
) =−(1/a
s
) +
1
2
R
e
k
2
(45.114)
K =
π cot η
0
e
2πα
−1
−ln β −0.5772
+β
2
∞
n=1
n
n
2
+β
2
−1
(45.115)
where β = Z
1
Z
2
e
2
/ v = Z
1
Z
2
/(ka
0
).
(2) Polarization potential: V(R) =−α
d
e
2
/2R
4
,
tan η
0
=−a
s
k−
π
3
C
4
k
2
−
4
3
C
4
a
s
k
3
ln(ka
0
)
+ Dk
3
+Fk
4
, (45.116)
tan η
1
=
π
15
C
4
k
2
−a
(1)
s
k
3
, (45.117)
tan η
=
πC
4
k
2
(2 +3)(2 +1)(2 −1)
+O
k
2+1
,
(45.118)
for >1, where
C
4
=
2M
2
α
d
e
2
2
=
α
d
a
0
M
m
e
.
(45.119)
Example: e
−
–Ar low energy collisions: The values
a
s
=−1.459a
0
;D = 68.93a
3
0
a
(1)
s
= 8.69a
3
0
; F =−97a
4
0
provide an accurate fit to recent measurements [45.6]of
(45.76a) for the diffusion cross section σ
d
.
(3) Van der Waals potential: V(R) =−C/R
6
k cot η
0
=−
1
a
s
+
1
2
R
e
k
2
−
π
15a
2
s
2MC
2
k
3
−
4
15a
s
2MC
2
k
4
ln(ka
0
) +O
k
4
.
(45.120)
e–Atom Collisions with Polarization Attraction. As
k →0, the differential cross section is
dσ
dΩ
= a
2
s
1 +
C
4
a
s
k sin
θ
2
+
8
3
C
4
k
2
ln(ka
0
) +···
(45.121)
and the elastic and diffusion cross sections are
σ(k →0) = 4πa
2
s
1 +
2πC
4
k
3a
s
+
8
3
C
4
k
2
ln(ka
0
) +···
(45.122)
σ
d
(k →0) = 4πa
2
s
1 +
4πC
4
k
5a
s
+
8
3
C
4
k
2
ln(ka
0
) +···
(45.123)
For e
−
–noble gas collisions, the scattering lengths
are
He Ne Ar Kr Xe
a
s
(a
0
) 1.19 0.24 -1.459 -3.7 -6.5
For atoms with a
s
< 0, a Ramsauer–Townsend min-
imum appears in both σ and σ
d
at low energies,
provided that scattering from higher partial waves
can be neglected, because from (45.116), η
0
0at
k =−3a
s
/πC
4
.
Semiclassical Scattering Lengths. For heavy particle
collisions, η
SC
(E → 0, b) tends to
η
SC
0
=
2M
2
1/2
∞
R
0
|V(R)|
1/2
dR . (45.124)
(a) Hard-core + well:
V(R) =
∞, R < R
0
−V
0
, R
0
≤ R < R
1
0, R
1
< R ,
a
s
=
1 −tan η
SC
0
/(kR
1
)
R
1
, (45.125)
η
SC
0
= k(R
1
+ R
0
), k
2
= 2MV
0
/
2
. (45.126)
The phase-averaged scattering length is a
s
=R
1
.
(b) Hard-core + power-law (n > 2):
V(R) =
#
∞ , R < R
0
±C/R
n
, R > R
0
. (45.127)
Repulsion (+): with γ
2
= 2MC/
2
,
a
(+)
s
=
γ
n −2
2/(n−2)
Γ
n −3
n −2
/Γ
n −1
n −2
.
(45.128)
Part D 45.2
